Skip to main content
SpringerLink
Log in

The Spectral Dimension of Generic Trees

  • Published:
Journal of Statistical Physics Aims and scope Submit manuscript

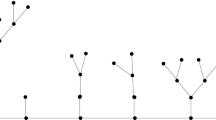
Abstract

We define generic ensembles of infinite trees. These are limits as N→∞ of ensembles of finite trees of fixed size N, defined in terms of a set of branching weights. Among these ensembles are those supported on trees with vertices of a uniformly bounded order. The associated probability measures are supported on trees with a single spine and Hausdorff dimension d h =2. Our main result is that the spectral dimension of the ensemble average is d s =4/3, and that the critical exponent of the mass, defined as the exponential decay rate of the two-point function along the spine, is 1/3.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. ben-Avraham, D., Havlin, S.: Diffusion and Reactions in Fractals and Disordered Systems. Cambridge University Press, Cambridge (2000)

    MATH  Google Scholar 

  2. Ambjørn, J., Durhuus, B., Jonsson, T.: Quantum Geometry: A Statistical Field Theory Approach. Cambridge University Press, Cambridge (1997)

    MATH  Google Scholar 

  3. Coulhon, T.: Random Walks and Geometry on Infinite Graphs. In: Ambrosio, L., Cassano, F.S. (eds.) Lecture Notes on Analysis on Metric Spaces, Trento, CIMR, 1999. Scuola Normale Superiore di Pisa (2000)

  4. Durhuus, B., Jonsson, T., Wheater, J.: Random walks on combs. J. Phys. A 39, 1009–1038 (2006)

    Article  MATH  ADS  Google Scholar 

  5. Alexander, S., Orbach, R.: Density of states on fractals: “fractons”. J. Phys. Lett. 43, L625–L631 (1982)

    Article  Google Scholar 

  6. Barlow, M.T., Járai, A.A., Kumagai, T., Slade, G.: Random walk on the incipient infinite cluster for oriented percolation in high dimensions. math.PR/0608164

  7. Ambjørn, J., Jurkiewicz, J., Watabiki, Y.: On the fractal structure of two-dimensional quantum gravity. Nucl. Phys. B 454, 313–342 (1995)

    Article  MATH  ADS  Google Scholar 

  8. Ambjørn, J., Anagnastopoulos, K.N., Ichihara, T., Jensen, T., Watabiki, Y.: Quantum geometry and diffusion. J. High Energy Phys. 11, 022 (1998)

    Article  ADS  Google Scholar 

  9. Ambjørn, J., Boulatov, D., Nielsen, J.L., Rolf, J., Watabiki, Y.: The spectral dimension of 2D quantum gravity. J. High Energy Phys. 9802, 010 (1998)

    Article  ADS  Google Scholar 

  10. Benjamini, I., Scramm, O.: Recurrence of distributional limits of finite planar graphs. Electron. J. Probab. 6, 23 (2001)

    Google Scholar 

  11. Ambjørn, J., Jurkiewicz, J., Loll, R.: Spectral dimension of the universe. Phys. Rev. Lett. 95, 171301 (2005), hep-th/0505113

    Article  ADS  Google Scholar 

  12. Ambjørn, J., Jurkiewicz, J., Loll, R.: Reconstructing the universe. Phys. Rev. D 72, 064014 (2005), hep-th/0505154

    Article  ADS  Google Scholar 

  13. Jonsson, T., Wheater, J.: The spectral dimension of the branched polymer phase of two-dimensional quantum gravity. Nucl. Phys. B 515, 549–574 (1998)

    Article  MATH  ADS  Google Scholar 

  14. Destri, C., Donetti, L.: The spectral dimension of random trees. J. Phys. A 35, 9499–9516 (2002)

    Article  MATH  ADS  Google Scholar 

  15. Correia, J.D., Wheater, J.F.: The spectral dimension of non-generic branched polymer ensembles. Phys. Lett. B 422, 76–81 (1998)

    Article  ADS  Google Scholar 

  16. Kesten, H.: Subdiffusive behavior of random walk on a random cluster. Ann. Inst. H. Poincaré Probab. Stat. 22, 425–487 (1986)

    MATH  Google Scholar 

  17. Barlow, M.T., Kumagai, T.: Random walk on the incipient infinite cluster on trees. math.PR/0503118

  18. Durhuus, B.: Probabilistic aspects of infinite trees and surfaces. Acta Phys. Pol. 34, 4795–4811 (2003)

    ADS  Google Scholar 

  19. Flajolet, Ph., Sedgewick, R.: Analytic combinatorics. Online book, available at http://algo.inria.fr/flajolet/Publications/books.html

  20. Harris, T.E.: The Theory of Branching Processes. Dover, New York (2002)

    MATH  Google Scholar 

  21. Burda, Z., Correia, J.D., Krzywicki, A.: Statistical ensemble of scale-free random graphs. Phys. Rev. E 64, 046118 (2001)

    Article  ADS  Google Scholar 

  22. Feller, W.: An Introduction to Probability Theory and Its Applications, vol. 2. Wiley, London (1968)

    MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Matematisk Institut, Universitetsparken 5, 2100, Copenhagen, Ø, Denmark

    Bergfinnur Durhuus

  2. University of Iceland, Dunhaga 3, 107, Reykjavik, Iceland

    Thordur Jonsson

  3. Rudolf Peierls Centre for Theoretical Physics, University of Oxford, 1 Keble Road, Oxford, OX13 NP, UK

    John F. Wheater

Authors
  1. Bergfinnur Durhuus
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Thordur Jonsson
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. John F. Wheater
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Thordur Jonsson.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Durhuus, B., Jonsson, T. & Wheater, J.F. The Spectral Dimension of Generic Trees. J Stat Phys 128, 1237–1260 (2007). https://doi.org/10.1007/s10955-007-9348-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10955-007-9348-3

Keywords

Search

Navigation