Skip to main content
SpringerLink
Log in

Arbitrarily High-Order Energy-Preserving Schemes for the Zakharov-Rubenchik Equations

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

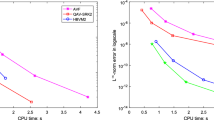
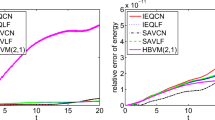
In this paper, we present a novel class of high-order energy-preserving schemes for solving the Zakharov-Rubenchik equations. The main idea of the scheme is first to introduce an quadratic auxiliary variable to transform the Hamiltonian energy into a modified quadratic energy and the original system is then reformulated into an equivalent system which satisfies the mass, modified energy as well as two linear invariants. The symplectic Runge-Kutta method in time, together with the Fourier pseudo-spectral method in space is employed to compute the solution of the reformulated system. The main benefit of the proposed schemes is that it can achieve arbitrarily high-order accurate in time and conserve the three invariants: mass, Hamiltonian energy and two linear invariants. In addition, an efficient fixed-point iteration is proposed to solve the resulting nonlinear equations of the proposed schemes. Several experiments are addressed to validate the theoretical results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13

Similar content being viewed by others

Data Availability

Enquiries about data availability should be directed to the authors.

References

  1. Barletti, L., Brugnano, L., Caccia, G.F., Iavernaro, F.: Energy-conserving methods for the nonlinear Schrödinger equation. Appl. Math. Comput. 318, 3–18 (2018)

    MATH  Google Scholar 

  2. Brugnano, L., Iavernaro, F.: Line Integral Methods for Conservative Problems. Chapman et Hall/CRC, Boca Raton (2016)

    Book  MATH  Google Scholar 

  3. Brugnano, L., Iavernaro, F., Trigiante, D.: Hamiltonian boundary value methods (energy preserving discrete line integral methods). J. Numer. Anal. Ind. Appl. Math. 5, 17–37 (2010)

    MATH  Google Scholar 

  4. Calvo, M., Iserles, A., Zanna, A.: Numerical solution of isospectral flows. Math. Comp. 66, 1461–1486 (1997)

    Article  MATH  Google Scholar 

  5. Chen, J., Qin, M.: Multi-symplectic Fourier pseudospectral method for the nonlinear Schrödinger equation. Electr. Trans. Numer. Anal. 12, 193–204 (2001)

    MATH  Google Scholar 

  6. Chen, Y., Gong, Y., Hong, Q., Wang, C.: A novel class of energy-preserving Runge-Kutta methods for the Korteweg-de Vries equation. Numer. Math. Theor. Meth. Appl. 15, 768–792 (2022)

    Article  MATH  Google Scholar 

  7. Cohen, D., Hairer, E.: Linear energy-preserving integrators for Poisson systems. BIT 51, 91–101 (2011)

    Article  MATH  Google Scholar 

  8. Cooper, G.J.: Stability of Runge-Kutta methods for trajectory problems. IMA J. Numer. Anal. 7, 1–13 (1987)

    Article  MATH  Google Scholar 

  9. Cordero, J.: Supersonic limit for the Zakharov-Rubenchik system. J. Differ. Equ. 261, 5260–5288 (2016)

    Article  MATH  Google Scholar 

  10. Cui, J., Wang, Y., Jiang, C.: Arbitrarily high-order structure-preserving schemes for the Gross-Pitaevskii equation with angular momentum rotation. Comput. Phys. Commun. 261, 107767 (2021)

    Article  Google Scholar 

  11. Gong, Y., Cai, J., Wang, Y.: Multi-symplectic Fourier pseudospectral method for the Kawahara equation. Commun. Comput. Phys. 16, 35–55 (2014)

    Article  MATH  Google Scholar 

  12. Gong, Y., Hong, Q., Wang, C., Wang, Y.: Arbitrarily high-order energy-preserving schemes for the Camassa-Holm equation based on the quadratic auxiliary variable approach. Adv. Appl. Math. Mech. (2022). https://doi.org/10.4208/aamm.OA-2022-0188

    Article  Google Scholar 

  13. Gong, Y., Zhao, J., Wang, Q.: Arbitrarily high-order linear energy stable schemes for gradient flow models. J. Comput. Phys. 419, 109610 (2020)

    Article  MATH  Google Scholar 

  14. Hairer, E.: Energy-preserving variant of collocation methods. J. Numer. Anal. Ind. Appl. Math. 5, 73–84 (2010)

    MATH  Google Scholar 

  15. Hairer, E., Lubich, C., Wanner, G.: Geometric Numerical Integration: Structure-Preserving Algorithms for Ordinary Differential Equations, 2nd edn. Springer-Verlag, Berlin (2006)

    MATH  Google Scholar 

  16. Ji, B., Zhang, L., Zhou, X.: Conservative compact difference scheme for the Zakharov-Rubenchik equations. Int. J. Comput. Math. 96, 537–556 (2019)

    Article  MATH  Google Scholar 

  17. Jiang, C., Cui, J., Qian, X., Song, S.: High-order linearly implicit structure-preserving exponential integrators for the nonlinear Schrödinger equation. J. Sci. Comput. 90, 66 (2022)

    Article  MATH  Google Scholar 

  18. Jiang, C., Qian, C., Song, S., Zheng. C.: Arbitrary high-order structure-preserving schemes for the generalized Rosenau-type equation (2022). arXiv:2205.10241

  19. Jiang, C., Wang, Y., Gong, Y.: Arbitrarily high-order energy-preserving schemes for the Camassa-Holm equation. Appl. Numer. Math. 151, 85–97 (2020)

    Article  MATH  Google Scholar 

  20. Li, H., Wang, Y., Qin, M.: A sixth order averaged vector field method. J. Comput. Math. 34, 479–498 (2016)

    Article  MATH  Google Scholar 

  21. Li, Y., Wu, X.: General local energy-preserving integrators for solving multi-symplectic Hamiltonian PDEs. J. Comput. Phys. 301, 141–166 (2015)

    Article  MATH  Google Scholar 

  22. Li, Y., Wu, X.: Functionally fitted energy-preserving methods for solving oscillatory nonlinear Hamiltonian systems. SIAM J. Numer. Anal. 54, 2036–2059 (2016)

    Article  MATH  Google Scholar 

  23. Linares, F., Matheus, C.: Well-posedness for the 1D Zakharov-Rubenchik system. Adv. Differ. Eq. 14, 261–288 (2009)

    MATH  Google Scholar 

  24. Mei, L., Huang, L., Wu, X.: Energy-preserving exponential integrators of arbitrarily high order for conservative or dissipative systems with highly oscillatory solutions. J. Comput. Phys. 442, 110429 (2021)

    Article  MATH  Google Scholar 

  25. Miyatake, Y., Butcher, J.C.: A characterization of energy-preserving methods and the construction of parallel integrators for Hamiltonian systems. SIAM J. Numer. Anal. 54, 1993–2013 (2016)

    Article  MATH  Google Scholar 

  26. Oliveira, F.: Stability of the solitons for the one-dimensional Zakharov-Rubenchik equation. Phys. D. 175, 220–240 (2003)

    Article  MATH  Google Scholar 

  27. Oliveira, F.: Adiabatic limit of the Zakharov-Rubenchik equation. Rep. Math. Phys. 61, 13–27 (2008)

    Article  MATH  Google Scholar 

  28. Oliveira, F.: Stability of solutions of the Zakharov–Rubenchik equation. In: Waves And Stability In Continuous Media (2006). https://doi.org/10.1142/9789812773616_0054

  29. Oruç, Ö.: A radial basis function finite difference (RBF-FD) method for numerical simulation of interaction of high and low frequency waves: Zakharov-Rubenchik equations. Appl. Math. Comput. 394, 125787 (2021)

    MATH  Google Scholar 

  30. Ponce, G., Saut, J.C.: Well-posedness for the Benney-Roskes/Zakharov-Rubenchik system. Discret. Contin. Dyn. Syst. 13, 818–852 (2005)

    Article  MATH  Google Scholar 

  31. Quispel, G.R.W., McLaren, D.I.: A new class of energy-preserving numerical integration methods. J. Phys. A Math. Theor. 41, 045206 (2008)

    Article  MATH  Google Scholar 

  32. Sanz-Serna, J.M.: Runge-Kutta schemes for Hamiltonian systems. BIT 28, 877–883 (1988)

    Article  MATH  Google Scholar 

  33. Sanz-Serna, J.M., Verwer, J.G.: Conservative and nonconservative schemes for the solution of the nonlinear Schrödinger equation. IMA J. Numer. Anal. 6, 25–42 (1986)

    Article  MATH  Google Scholar 

  34. Shen, J., Tang, T.: Spectral and High-Order Methods with Applications. Science Press, Beijing (2006)

    MATH  Google Scholar 

  35. Shen, J., Xu, J., Yang, J.: The scalar auxiliary variable (SAV) approach for gradient. J. Comput. Phys. 353, 407–416 (2018)

    Article  MATH  Google Scholar 

  36. Shen, J., Xu, J., Yang, J.: A new class of efficient and robust energy stable schemes for gradient flows. SIAM Rev. 61, 474–506 (2019)

    Article  MATH  Google Scholar 

  37. Tang, W., Sun, Y.: Time finite element methods: a unified framework for numerical discretizations of ODEs. Appl. Math. Comput. 219, 2158–2179 (2012)

    MATH  Google Scholar 

  38. Tapley, B.K.: Geometric integration of ODEs using multiple quadratic auxiliary variables. SIAM J. Sci. Comput. 44, A2651–A2668 (2022)

    Article  MATH  Google Scholar 

  39. Wang, B., Jiang, Y.: Optimal convergence and long-time conservation of exponential integration for schrödinger equations in a normal or highly oscillatory regime. J. Sci. Comput. 90, 1–31 (2022)

    Article  MATH  Google Scholar 

  40. Yang, X., Zhao, J., Wang, Q.: Numerical approximations for the molecular beam epitaxial growth model based on the invariant energy quadratization method. J. Comput. Phys. 333, 104–127 (2017)

    Article  MATH  Google Scholar 

  41. Zakharov, V.E., Rubenchik, A.M.: Nonlinear interaction between high and low frequency waves. Prikl. Mat. Techn. Fiz. 5, 84–89 (1972)

    Google Scholar 

  42. Zhang, F., Pérez-García, V.M., Vázquez, L.: Numerical simulation of nonlinear Schröinger systems: a new conservative scheme. Appl. Math. Comput. 71, 165–177 (1995)

    MATH  Google Scholar 

  43. Zhang, G., Jiang, C.: Arbitrary high-order structure-preserving methods for the quantum Zakharov system. (2022) arXiv:2202.13052

  44. Zhao, X., Li, Z.: Numerical methods and simulations for the dynamics of one-dimensional Zakharov-Rubenchik equations. J. Sci. Comput. 59, 412–438 (2014)

    Article  MATH  Google Scholar 

  45. Zhou, X., Wang, T., Zhang, L.: Two numerical methods for the Zakharov-Rubenchik equations. Adv. Comput. Math. 45, 1163–1184 (2019)

    Article  MATH  Google Scholar 

Download references

Acknowledgements

The authors would like to express sincere gratitude to the referees for their insightful comments and suggestions. The work is supported by the National Natural Science Foundation of China (Grant Nos. 11901513, 12261097, 12261103), and the Yunnan Fundamental Research Projects (Grant Nos. 202101AT070208, 202001AT070066, 202101AS070044), the Natural Science Foundation of Hunan (Grant No. 2021JJ40655) and Innovation team of School of Mathematics and Statistics, Yunnan University (No. ST20210104). The first author is in particular grateful to Prof. Weizhu Bao for fruitful discussions.

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics, Yunnan University, Kunming, 650504, China

    Gengen Zhang

  2. School of Statistics and Mathematics, Yunnan University of Finance and Economics, Kunming, 650221, China

    Chaolong Jiang & Hao Huang

  3. Department of Mathematics, College of Liberal Arts and Science, National University of Defense Technology, Changsha, 410073, China

    Chaolong Jiang

  4. Basic Teaching Department, Guizhou Vocational Institute of Technology, Fuquan, 550500, China

    Hao Huang

Authors
  1. Gengen Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Chaolong Jiang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Hao Huang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Chaolong Jiang.

Ethics declarations

Conflict of interest

The authors have not disclosed any competing interests.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhang, G., Jiang, C. & Huang, H. Arbitrarily High-Order Energy-Preserving Schemes for the Zakharov-Rubenchik Equations. J Sci Comput 94, 32 (2023). https://doi.org/10.1007/s10915-022-02075-4

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-022-02075-4

Keywords

Mathematics Subject Classification

Search

Navigation