Skip to main content
SpringerLink
Log in

Conforming Virtual Element Methods for Sobolev Equations

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

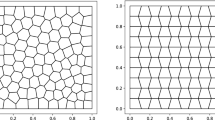
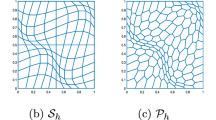
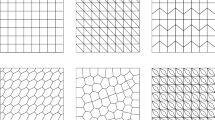
The intention of this paper is to study the \(H^1\)-conforming virtual element method for a class of Sobolev equations with variable coefficients. The semi-discrete scheme is constructed on the basis of the symmetric Nitsche’s method to impose the homogeneous and inhomogeneous Dirichlet boundary conditions in a unified way. The fully discrete scheme is obtained with the Crank–Nicolson scheme for temporal approximation. Under some assumptions about the penalty parameter in the Nitsche’s method, the existence and uniqueness of the semi-discrete and fully discrete solutions are analyzed. Furthermore, for the semi-discrete and fully discrete schemes, optimal a priori error estimates in both a mesh size dependent norm and \(L^2\)-norm are proven. Finally, some numerical experiments are carried out to illustrate the theoretical results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

Data Availability

Enquiries about data availability should be directed to the authors.

References

  1. van Duijn, C.J., Fan, Y., Peletier, L.A., Pop, I.S.: Travelling wave solutions for degenerate pseudo-parabolic equations modelling two-phase flow in porous media. Nonlinear Anal. Real World Appl. 14(3), 1361–1383 (2013)

    MathSciNet  MATH  Google Scholar 

  2. Ting, T.W.: A cooling process according to two-temperature theory of heat conduction. J. Math. Anal. Appl. 45(1), 23–31 (1974)

    MathSciNet  MATH  Google Scholar 

  3. Cao, Y., Yin, J., Wang, C.: Cauchy problems of semilinear pseudo-parabolic equations. J. Differential Equations 246(12), 4568–4590 (2009)

    MathSciNet  MATH  Google Scholar 

  4. Abreu, E., Durán, A.: Spectral discretizations analysis with time strong stability preserving properties for pseudo-parabolic models. Comput. Math. Appl. 102, 15–44 (2021)

    MathSciNet  MATH  Google Scholar 

  5. Ford, W.H., Ting, T.W.: Stability and convergence of difference approximations to pseudo-parabolic partial differential equations. Math. Comp. 27(124), 737–743 (1973)

    MathSciNet  MATH  Google Scholar 

  6. Ewing, R.E.: Numerical solution of Sobolev partial differential equations. SIAM J. Numer. Anal. 12(3), 345–363 (1975)

    MathSciNet  MATH  Google Scholar 

  7. Luo, Z., Teng, F., Chen, J.: A POD-based reduced-order Crank-Nicolson finite volume element extrapolating algorithm for 2D Sobolev equations. Math. Comput. Simulation 146, 118–133 (2018)

    MathSciNet  MATH  Google Scholar 

  8. Yang, M.: Analysis of second order finite volume element methods for pseudo-parabolic equations in three spatial dimensions. Appl. Math. Comput. 196(1), 94–104 (2008)

    MathSciNet  MATH  Google Scholar 

  9. Liu, T., Lin, Yp., Rao, M., Cannon, J.R.: Finite element methods for Sobolev equations. J. Comput. Math. 20(6), 627–642 (2002)

    MathSciNet  MATH  Google Scholar 

  10. Yang, J., Luo, Z.: A reduced-order extrapolating space-time continuous finite element method for the 2D Sobolev equation. Numer. Methods Partial Differ. Equ. 36(6), 1446–1459 (2020)

    MathSciNet  Google Scholar 

  11. Dehghan, M., Gharibi, Z.: Numerical analysis of fully discrete energy stable weak Galerkin finite element scheme for a coupled Cahn-Hilliard-Navier-Stokes phase-field model. Appl. Math. Comput. 410, 126487 (2021)

    MathSciNet  MATH  Google Scholar 

  12. Gao, F., Cui, J., Zhao, G.: Weak Galerkin finite element methods for Sobolev equation. J. Comput. Appl. Math. 317, 188–202 (2017)

    MathSciNet  MATH  Google Scholar 

  13. Gao, F., Wang, X.: A modified weak Galerkin finite element method for Sobolev equation. J. Comput. Math. 33(3), 307–322 (2015)

    MathSciNet  MATH  Google Scholar 

  14. Zhao, D., Zhang, Q.: Local discontinuous Galerkin methods with generalized alternating numerical fluxes for two-dimensional linear Sobolev equation. J. Sci. Comput. 78(3), 1660–1690 (2019)

    MathSciNet  MATH  Google Scholar 

  15. Abbaszadeh, M., Dehghan, M.: Interior penalty discontinuous Galerkin technique for solving generalized Sobolev equation. Appl. Numer. Math. 154, 172–186 (2020)

    MathSciNet  MATH  Google Scholar 

  16. Li, N., Lin, P., Gao, F.: An expanded mixed finite element method for two-dimensional Sobolev equations. J. Comput. Appl. Math. 348, 342–355 (2019)

    MathSciNet  MATH  Google Scholar 

  17. Guo, H.: A remark on split least-squares mixed element procedures for pseudo-parabolic equations. Appl. Math. Comput. 217(9), 4682–4690 (2011)

    MathSciNet  MATH  Google Scholar 

  18. Gao, F., Rui, H.: A split least-squares characteristic mixed finite element method for Sobolev equations with convection term. Math. Comput. Simulation 80(2), 341–351 (2009)

    MathSciNet  MATH  Google Scholar 

  19. Dehghan, M., Shafieeabyaneh, N., Abbaszadeh, M.: Application of spectral element method for solving Sobolev equations with error estimation. Appl. Numer. Math. 158, 439–462 (2020)

    MathSciNet  MATH  Google Scholar 

  20. Oruç, O.: A computational method based on Hermite wavelets for two-dimensional Sobolev and regularized long wave equations in fluids. Numer. Methods Partial Differ. Equ. 34(5), 1693–1715 (2018)

    MathSciNet  MATH  Google Scholar 

  21. Dehghan, M., Hooshyarfarzin, B., Abbaszadeh, M.: Proper orthogonal decomposition Pascal polynomial-based method for solving Sobolev equation. Int. J. Numer. Methods Heat Fluid Flow 32(7), 2506–2542 (2022)

    Google Scholar 

  22. Cangiani, A., Manzini, G., Sutton, O.J.: Conforming and nonconforming virtual element methods for elliptic problems. IMA J. Numer. Anal. 37(3), 1317–1354 (2017)

    MathSciNet  MATH  Google Scholar 

  23. Beirão da Veiga, L., Brezzi, F., Cangiani, A., Manzini, G., Marini, L.D., Russo, A.: Basic principles of virtual element methods. Math. Models Methods Appl. Sci. 23(1), 199–214 (2013)

    MathSciNet  MATH  Google Scholar 

  24. Vacca, G., Beirão da Veiga, L.: Virtual element methods for parabolic problems on polygonal meshes. Numer. Methods Partial Differ. Equ. 31(6), 2110–2134 (2015)

    MathSciNet  MATH  Google Scholar 

  25. Zhao, J., Zhang, B., Zhu, X.: The nonconforming virtual element method for parabolic problems. Appl. Numer. Math. 143, 97–111 (2019)

    MathSciNet  MATH  Google Scholar 

  26. Adak, D., Natarajan, E., Kumar, S.: Convergence analysis of virtual element methods for semilinear parabolic problems on polygonal meshes. Numer. Methods Partial Differ. Equ. 35(1), 222–245 (2019)

    MathSciNet  MATH  Google Scholar 

  27. Adak, D., Natarajan, S.: Virtual element methods for nonlocal parabolic problems on general type of meshes. Adv. Comput. Math. 46(5), 74 (2020)

    MathSciNet  MATH  Google Scholar 

  28. Adak, D., Natarajan, E., Kumar, S.: Virtual element method for semilinear hyperbolic problems on polygonal meshes. Int. J. Comput. Math. 96(5), 971–991 (2019)

    MathSciNet  MATH  Google Scholar 

  29. Anaya, V., Bendahmane, M., Mora, D., Sepúlveda, M.: A virtual element method for a nonlocal FitzHugh-Nagumo model of cardiac electrophysiology. IMA J. Numer. Anal. 40(2), 1544–1576 (2020)

    MathSciNet  MATH  Google Scholar 

  30. Li, M., Zhao, J., Wang, N., Chen, S.: Conforming and nonconforming conservative virtual element methods for nonlinear Schrödinger equation: a unified framework. Comput. Methods Appl. Mech. Engrg. 380, 113793 (2021)

    MATH  Google Scholar 

  31. Dehghan, M., Gharibi, Z.: Virtual element method for solving an inhomogeneous Brusselator model with and without cross-diffusion in pattern formation. J. Sci. Comput. 89(1), 16 (2021)

    MathSciNet  MATH  Google Scholar 

  32. Dehghan, M., Gharibi, Z., Eslahchi, M.R.: Unconditionally energy stable \(C^0\)-virtual element scheme for solving generalized Swift-Hohenberg equation. Appl. Numer. Math. 178, 304–328 (2022)

    MathSciNet  MATH  Google Scholar 

  33. Beirão da Veiga, L., Manzini, G.: A virtual element method with arbitrary regularity. IMA J. Numer. Anal. 34(2), 759–781 (2014)

    MathSciNet  MATH  Google Scholar 

  34. Antonietti, P.F., Beirão da Veiga, L., Scacchi, S., Verani, M.: A \(C^1\) virtual element method for the Cahn-Hilliard equation with polygonal meshes. SIAM J. Numer. Anal. 54(1), 34–56 (2016)

    MathSciNet  MATH  Google Scholar 

  35. Li, M., Zhao, J., Huang, C., Chen, S.: Nonconforming virtual element method for the time fractional reaction-subdiffusion equation with non-smooth data. J. Sci. Comput. 81(3), 1823–1859 (2019)

    MathSciNet  MATH  Google Scholar 

  36. Choudury, G.: Fully discrete approximations of parabolic boundary-value problems with nonsmooth boundary data. Appl. Math. Optim. 31(1), 41–55 (1995)

    MathSciNet  MATH  Google Scholar 

  37. Burman, E., Hansbo, P., Larson, M.G.: A cut finite element method with boundary value correction. Math. Comp. 87(310), 633–657 (2018)

    MathSciNet  MATH  Google Scholar 

  38. Ahmad, B., Alsaedi, A., Brezzi, F., Marini, L.D., Russo, A.: Equivalent projectors for virtual element methods. Comput. Math. Appl. 66(3), 376–391 (2013)

    MathSciNet  MATH  Google Scholar 

  39. Brenner, S.C., Guan, Q., Sung, L.Y.: Some estimates for virtual element methods. Comput. Methods Appl. Math. 17(4), 553–574 (2017)

    MathSciNet  MATH  Google Scholar 

  40. Chen, L., Huang, J.: Some error analysis on virtual element methods. Calcolo 55(1), 5 (2018)

    MathSciNet  MATH  Google Scholar 

  41. Rivière, B.: Discontinuous Galerkin Methods for Solving Elliptic and Parabolic Equations. Society for Industrial and Applied Mathematics (SIAM), Philadelphia (2008)

    MATH  Google Scholar 

  42. Bertoluzza, S., Pennacchio, M., Prada, D.: High order VEM on curved domains. Rend. Lincei Mat. Appl. 30(2), 391–412 (2019)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors are grateful to the referees for their valuable comments.

Funding

This work is supported by the National Natural Science Foundation of China (11771112, 12071100).

Author information

Authors and Affiliations

  1. School of Mathematics, Harbin Institute of Technology, Harbin, 150001, China

    Yang Xu, Zhenguo Zhou & Jingjun Zhao

Authors
  1. Yang Xu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zhenguo Zhou
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jingjun Zhao
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jingjun Zhao.

Ethics declarations

Competing interests

The authors have not disclosed any competing interests.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Xu, Y., Zhou, Z. & Zhao, J. Conforming Virtual Element Methods for Sobolev Equations. J Sci Comput 93, 32 (2022). https://doi.org/10.1007/s10915-022-01997-3

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-022-01997-3

Keywords

Mathematics Subject Classification

Search

Navigation