Skip to main content
SpringerLink
Log in

An Immersed Raviart–Thomas Mixed Finite Element Method for Elliptic Interface Problems on Unfitted Meshes

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

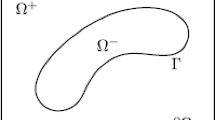
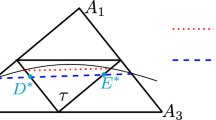
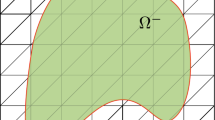
Abstract

This paper presents a lowest-order immersed Raviart–Thomas mixed triangular finite element method for solving elliptic interface problems on unfitted meshes independent of the interface. In order to achieve the optimal convergence rates on unfitted meshes, an immersed finite element (IFE) is constructed by modifying the traditional Raviart–Thomas element. Some important properties are derived including the unisolvence of IFE basis functions, the optimal approximation capabilities of the IFE space and the corresponding commuting digram. Optimal finite element error estimates are proved rigorously with the constant independent of the interface location relative to the mesh. Some numerical examples are provided to validate the theoretical analysis.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

Data Availibility

Enquiries about data availability should be directed to the authors.

References

  1. Babuška, I.: The finite element method for elliptic equations with discontinuous coefficients. Computing 5, 207–213 (1970)

    Article  MathSciNet  Google Scholar 

  2. Barrett, J.W., Elliott, C.M.: A finite-element method for solving elliptic equations with Neumann data on a curved boundary using unfitted meshes. IMA J. Numer. Anal. 4, 309–325 (1984)

    Article  MathSciNet  Google Scholar 

  3. Barrett, J.W., Elliott, C.M.: Fitted and unfitted finite-element methods for elliptic equations with smooth interfaces. IMA J. Numer. Anal. 7, 283–300 (1987)

    Article  MathSciNet  Google Scholar 

  4. Boffi, D., Brezzi, F., Fortin, M.: Mixed Finite Element Methods and Applications, vol. 44. Springer, New York (2013)

  5. Bramble, J.H., King, J.T.: A finite element method for interface problems in domains with smooth boundaries and interfaces. Adv. Comput. Math. 6, 109–138 (1996)

    Article  MathSciNet  Google Scholar 

  6. Brenner, S., Owens, L., Sung, L.: A weakly over-penalized symmetric interior penalty method. Electron. Trans. Numer. Anal. 30, 107–127 (2008)

    MathSciNet  MATH  Google Scholar 

  7. Brenner, S., Scott, L.: The Mathematical Theory of Finite Element Methods. Texts in Applied Mathematics, vol. 15. Springer, Berlin (2008)

    Google Scholar 

  8. Brezzi, F., Fortin, M.: Mixed and Hybrid Finite Element Methods. Springer, Berlin (1991)

    Book  Google Scholar 

  9. Burman, E., Guzmám, J., Sánchez, M.A., Sarkis, M.: Robust flux error estimation of an unfitted Nitsche method for high-contrast interface problems. IMA J. Numer. Anal. 38, 646–668 (2018)

    Article  MathSciNet  Google Scholar 

  10. Cao, S., Chen, L., Guo, R.: A Virtual Finite Element Method for Two Dimensional Maxwell Interface Problems with a Background Unfitted Mesh. arXiv:2103.04582 (2021)

  11. Chen, Z., Zou, J.: Finite element methods and their convergence for elliptic and parabolic interface problems. Numer. Math. 79, 175–202 (1998)

    Article  MathSciNet  Google Scholar 

  12. Chu, C.-C., Graham, I.G., Hou, T.-Y.: A new multiscale finite element method for high-contrast elliptic interface problems. Math. Comp. 79, 1915–1955 (2010)

    Article  MathSciNet  Google Scholar 

  13. Foote, R.L.: Regularity of the distance function. Proc. Am. Math. Soc. 92, 153–155 (1984)

    MathSciNet  MATH  Google Scholar 

  14. Frei, S., Richter, T.: A locally modified parametric finite element method for interface problems. SIAM J. Numer. Anal. 52, 2315–2334 (2014)

    Article  MathSciNet  Google Scholar 

  15. Fries, T., Belytschko, T.: The extended/generalized finite element method: an overview of the method and its applications. Int. J. Numer. Meth. Eng. 84, 253–304 (2010)

    Article  MathSciNet  Google Scholar 

  16. Gilbarg, D., Trudinger, N.S.: Elliptic partial differential equations of second order. Classics in Mathematics. Springer, Berlin. Reprint of the 1998 edition (2001)

  17. Guo, R.: Solving parabolic moving interface problems with dynamical immersed spaces on unfitted meshes: fully discrete analysis. SIAM J. Numer. Anal. 59, 797–828 (2021)

    Article  MathSciNet  Google Scholar 

  18. Guo, R., Lin, T.: A group of immersed finite-element spaces for elliptic interface problems. IMA J. Numer. Anal. 39, 482–511 (2019)

    Article  MathSciNet  Google Scholar 

  19. Guo, R., Lin, T.: An immersed finite element method for elliptic interface problems in three dimensions. J. Comput. Phys. 414, 109478 (2020)

    Article  MathSciNet  Google Scholar 

  20. Guo, R., Lin, T., Lin, Y.: A fixed mesh method with immersed finite elements for solving interface inverse problems. J. Sci. Comput. 79, 148–175 (2019)

    Article  MathSciNet  Google Scholar 

  21. Guo, R., Lin, T., Lin, Y.: Recovering elastic inclusions by shape optimization methods with immersed finite elements. J. Comput. Phys. 404, 109123 (2020)

    Article  MathSciNet  Google Scholar 

  22. Guo, R., Lin, Y., Zou, J.: Solving two dimensional H(curl)-elliptic interface systems with optimal convergence on unfitted meshes. arXiv:2011.11905 (2020)

  23. Guzmán, J., Sánchez, M.A., Sarkis, M.: A finite element method for high-contrast interface problems with error estimates independent of contrast. J. Sci. Comput. 73, 330–365 (2017)

    Article  MathSciNet  Google Scholar 

  24. Hansbo, A., Hansbo, P.: An unfitted finite element method, based on Nitsche’s method, for elliptic interface problems. Comput. Methods Appl. Mech. Eng. 191, 5537–5552 (2002)

    Article  MathSciNet  Google Scholar 

  25. He, X., Lin, T., Lin, Y.: Approximation capability of a bilinear immersed finite element space. Numer. Methods Partial Differ. Equ. 24, 1265–1300 (2008)

    Article  MathSciNet  Google Scholar 

  26. He, X., Lin, T., Lin, Y.: The convergence of the bilinear and linear immersed finite element solutions to interface problems. Numer. Methods Partial Differ. Equ. 28, 312–330 (2012)

    Article  MathSciNet  Google Scholar 

  27. Hou, S., Liu, X.: A numerical method for solving variable coefficient elliptic equation with interfaces. J. Comput. Phys. 202, 411–445 (2005)

    Article  MathSciNet  Google Scholar 

  28. Huang, J., Zou, J.: Some new a priori estimates for second-order elliptic and parabolic interface problems. J. Differ. Equ. 184, 570–586 (2002)

    Article  MathSciNet  Google Scholar 

  29. Huang, J., Zou, J.: Uniform a priori estimates for elliptic and static Maxwell interface problems. Discrete Contin. Dyn. Syst. Ser. B 7, 145–170 (2007)

    MathSciNet  MATH  Google Scholar 

  30. Ji, H., Wang, F., Chen, J., Li, Z.: Analysis of nonconforming IFE methods and a new scheme for elliptic interface problems. arXiv:2108.03179 (2021)

  31. Ji, H., Wang, F., Chen, J., Li, Z.: A new parameter free partially penalized immersed finite element and the optimal convergence analysis. Numer. Math. 150, 1035–1086 (2022)

  32. Kwak, D.Y., Wee, K.T., Chang, K.S.: An analysis of a broken \(P_1\)-nonconforming finite element method for interface problems. SIAM J. Numer. Anal. 48, 2117–2134 (2010)

    Article  MathSciNet  Google Scholar 

  33. Li, J., Markus, J., Wohlmuth, B., Zou, J.: Optimal a priori estimates for higher order finite elements for elliptic interface problems. Appl. Numer. Math. 60, 19–37 (2010)

    Article  MathSciNet  Google Scholar 

  34. Li, Z.: The immersed interface method using a finite element formulation. Appl. Numer. Math. 27, 253–267 (1998)

    Article  MathSciNet  Google Scholar 

  35. Li, Z., Lin, T., Lin, Y., Rogers, R.: An immersed finite element space and its approximation capability. Numer. Methods Partial Differ. Equ. 20, 338–367 (2004)

    Article  MathSciNet  Google Scholar 

  36. Li, Z., Lin, T., Wu, X.: New Cartesian grid methods for interface problems using the finite element formulation. Numer. Math. 96, 61–98 (2003)

    Article  MathSciNet  Google Scholar 

  37. Lin, T., Lin, Y., Zhang, X.: Partially penalized immersed finite element methods for elliptic interface problems. SIAM J. Numer. Anal. 53, 1121–1144 (2015)

    Article  MathSciNet  Google Scholar 

  38. Lin, T., Zhang, X.: Linear and bilinear immersed finite elements for planar elasticity interface problems. J. Comput. Appl. Math. 236, 4681–4699 (2012)

    Article  MathSciNet  Google Scholar 

  39. Peskin, C.: Numerical analysis of blood flow in the heart. J. Comput. Phys. 25, 220–252 (1977)

    Article  MathSciNet  Google Scholar 

  40. Raviart, P.A., Thomas, J.M.: A mixed finite element method for second order elliptic problems. Mathematical Aspects of the Finite Element Method, Lecture Notes in Math., 606, Springer, Berlin (1977)

  41. Wang, H., Chen, J., Sun, P., Qin, F.: A conforming enriched finite element method for elliptic interface problems. Appl. Numer. Math. 127, 1–17 (2018)

    Article  MathSciNet  Google Scholar 

  42. Wang, S., Wang, F., Xu, X.: A Rigorous Condition Number Estimate of an Immersed Finite Element Method. J. Sci. Comput. 83, 29 (2020)

    Article  MathSciNet  Google Scholar 

  43. Wu, H., Xiao, Y.: An unfitted \(hp\)-interface penalty finite element method for elliptic interface problems. J. Comput. Math. 37, 316–339 (2019)

    Article  MathSciNet  Google Scholar 

  44. Xiao, Y., Xu, J., Wang, F.: High-order extended finite element methods for solving interface problems. Comput. Methods Appl. Mech. Eng. 364, 112964 (2016)

    Article  MathSciNet  Google Scholar 

  45. Xu, J.: Error estimates of the finite element method for the 2nd order elliptic equations with discontinuous coefficients. J. Xiangtan Univ. 1, 1–5 (1982)

    Google Scholar 

  46. Zhang, S.: Robust and local optimal a priori error estimates for interface problems with low regularity: mixed finite element approximations. J. Sci. Comput. 84, 40 (2020)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

The author would like to thank the anonymous referees sincerely for their careful reading and helpful suggestions that improved the quality of the paper.

Funding

This work was partially supported by the National Natural Science Foundation of China (Grants Nos. 11701291, 12101327 and 11801281) and the Natural Science Foundation of Jiangsu Province (Grant No. BK20200848).

Author information

Authors and Affiliations

  1. School of Science, Nanjing University of Posts and Telecommunications, Nanjing, 210023, Jiangsu, China

    Haifeng Ji

Authors
  1. Haifeng Ji
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Haifeng Ji.

Ethics declarations

Conflict of interest

The authors have not disclosed any competing interests.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ji, H. An Immersed Raviart–Thomas Mixed Finite Element Method for Elliptic Interface Problems on Unfitted Meshes. J Sci Comput 91, 66 (2022). https://doi.org/10.1007/s10915-022-01839-2

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-022-01839-2

Keywords

Mathematics Subject Classification

Search

Navigation