FDTD Schemes for Maxwell’s Equations with Embedded Perfect Electric Conductors Based on the Correction Function Method

Abstract

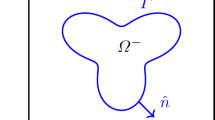
In this work, we propose staggered FDTD schemes based on the correction function method (CFM) to discretize Maxwell’s equations with embedded perfect electric conductor boundary conditions. The CFM uses a minimization procedure to compute a correction to a given FD scheme in the vicinity of the embedded boundary to retain its order. The minimization problem associated with CFM approaches is analyzed in the context of Maxwell’s equations with embedded boundaries. In order to obtain a well-posed minimization problem, we propose fictitious interfaces to fulfill the lack of information, namely the surface current and charge density, on the embedded boundary. We introduce CFM-FDTD schemes based on the well-known Yee scheme and a fourth-order staggered FDTD scheme. We investigate the stability of these CFM-FDTD schemes using long time simulations. Convergence studies are performed in 2-D for various geometries of the embedded boundary. CFM-FDTD schemes have shown high-order convergence.

Truncation Error Analysis

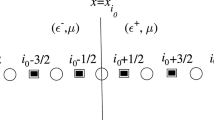
As shown in [19], the CFM can reduce the order in space of an original FD scheme for unsteady problems. Proposition 3 provides a general result on the order in space of a corrected FD scheme.

Proposition 3

Let us consider a domain $\varOmega$, a time interval I and an interface $\varGamma \subset ~\varOmega$ on which there are interface jump conditions. Assume that the correction function coming from the CFM is smooth enough and is such that

$$\begin{aligned} \partial _t \hat{\varvec{U}} + L\,(\hat{\varvec{U}}+A\,\hat{\varvec{D}}) = \varvec{F}, \end{aligned}$$

(23)

where $\hat{\varvec{U}}$ is the vector of true solution values, A is a rectangular matrix with either 0 or $\pm 1$ as components, $\hat{\varvec{D}}$ is the vector of true correction function values, L is a spatial finite difference operator of order n that approximates q-order derivatives and $\varvec{F}$ is a source term. A $(k+1)$-order approximation of the correction function leads to a corrected FD scheme of order $\min \{n,k-q+1\}$ in space.

Proof

A $(k+1)$-order approximation of $\hat{\varvec{D}}$ leads to

$$\begin{aligned} \varvec{D} = \hat{\varvec{D}} + {\mathcal {O}}(\ell _h^{k+1}), \end{aligned}$$

where $\ell _h = \beta \,h$ is the length of the space–time patch, $\beta$ is a positive constant and h is the mesh grid size. The discrete operator L, that approximates q-order derivatives, involves components scaled by a factor $\tfrac{1}{h^q}$. Hence, $L\,A\,\varvec{D} = L\,A\,\hat{\varvec{D}} + {\mathcal {O}}(\ell _h^{k+1}\,h^{-q}) = L\,A\,\hat{\varvec{D}} + {\mathcal {O}}(h^{k-q+1})$. $\square$

For problems that do not involve transient derivatives, we have

$$\begin{aligned} \hat{\varvec{U}} = L^{-1}\,\varvec{F} - A\,\hat{\varvec{D}} \end{aligned}$$

and the order of the corrected FD scheme is then $\min \{n,k+1\}$.