Hybridized Discontinuous Galerkin Method for Elliptic Interface Problems: Error Estimates Under Low Regularity Assumptions of Solutions

Abstract

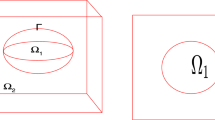
New hybridized discontinuous Galerkin (HDG) methods for the interface problem for elliptic equations are proposed. Unknown functions of our schemes are $u_h$ in elements and $\hat{u}_h$ on inter-element edges. That is, we formulate our schemes without introducing the flux variable. We assume that subdomains $\Omega _1$ and $\Omega _2$ are polyhedral domains and that the interface $\Gamma =\partial \Omega _1\cap \partial \Omega _2$ is polyhedral surface or polygon. Moreover, $\Gamma$ is assumed to be expressed as the union of edges of some elements. We deal with the case where the interface is transversely connected with the boundary of the whole domain $\overline{\Omega }=\overline{\Omega _1\cap \Omega _2}$. Consequently, the solution u of the interface problem may not have a sufficient regularity, say $u\in H^2(\Omega )$ or $u|_{\Omega _1}\in H^2(\Omega _1)$, $u|_{\Omega _2}\in H^2(\Omega _2)$. We succeed in deriving optimal order error estimates in an HDG norm and the $L^2$ norm under low regularity assumptions of solutions, say $u|_{\Omega _1}\in H^{1+s}(\Omega _1)$ and $u|_{\Omega _2}\in H^{1+s}(\Omega _2)$ for some $s\in (1/2,1]$, where $H^{1+s}$ denotes the fractional order Sobolev space. Numerical examples to validate our results are also presented.

Proof of Lemma 9

Let $s\in (1/2,1)$. Let $K\in \mathcal {T}_h$ and $e\subset \partial K$.

The fractional order Sobolev space $H^s(K)$ is defined as

$$\begin{aligned} H^{s}(K)=\{v\in L^2(K)\mid \Vert v\Vert _{H^{s}(K)}^2=\Vert v\Vert _{L^2(K)}^2+|v|_{H^{s}(K)}^2<\infty \}, \end{aligned}$$

where

$$\begin{aligned} |v|_{H^s(K)}^2=\int \ \int _{K\times K}\frac{~|v(x)-v(y)|^2}{|x-y|^{d+2s}}~dxdy. \end{aligned}$$

It suffices to prove

$$\begin{aligned} \Vert v\Vert _{L^2(e)}^2 \le C_{s,\mathrm {T}}h_e^{-1}\left( \Vert v\Vert _{L^2(K)}^2+h_K^{2s}|v|_{H^s(K)}^2\right) \qquad (v\in H^s(K)), \end{aligned}$$

(27)

since the desired inequality (20) is a direct consequence of (27).

Suppose that $\tilde{K}$ is the reference element in $\mathbb {R}^d$ with ${\text {diam}}(\tilde{K})=1$. Moreover, let $\tilde{e}\subset \partial \tilde{K}$ be a face ($d=3$)/edge ($d=2$) of $\tilde{K}$. Trace theorem implies

$$\begin{aligned} \Vert \tilde{v}\Vert _{L^2(e)}^2\le \tilde{C}\left( \Vert \tilde{v}\Vert _{L^2(\tilde{K})}^2+|\tilde{v}|_{H^{s}(\tilde{K})}^2\right) \qquad (\tilde{v}\in H^1(\tilde{K})), \end{aligned}$$

where $\tilde{C}$ denotes an absolute positive constant. See [13, Theorem 1, §V.1.1] for example.

Suppose that $\Phi (\xi )=B\xi +c$, $B\in \mathbb {R}^{d\times d}$, $c\in \mathbb {R}^d$, is the affine mapping which maps $\tilde{K}$ onto K; $K=\Phi (\tilde{K})$. We know

$$\begin{aligned} \Vert B\Vert =\sup _{|\xi |=1}|B\xi |\le \frac{h_K}{\tilde{\rho }},\quad \Vert B^{-1}\Vert \le \frac{\tilde{h}}{\rho _K},\quad d\xi =\frac{{\text {meas}}_{d}(\tilde{K})}{{\text {meas}}_{d}({K})} dx, \end{aligned}$$

where $\tilde{h}={h}_{\tilde{K}}$, $\tilde{\rho }={\rho }_{\tilde{K}}$ and ${\text {meas}}_d(K)$ denotes the $\mathbb {R}^d$-Lebesgue measure of K. Moreover,

$$\begin{aligned} \frac{|x|}{|B^{-1}x|}\le \sup _{\xi \in \mathbb {R}^d} \frac{|B\xi |}{|\xi |}=\Vert B\Vert \qquad (x\in \mathbb {R}^d,x\ne 0). \end{aligned}$$

We recall that there exists a positive constant $\nu _2$ that independent of h such that $h_K/\rho _K\le \nu _2$ ($\forall K\in \forall \mathcal {T}_h\in \{\mathcal {T}_h\}_h$) by the shape-regularity of the family of triangulations.

Now we can state the proof of (27). By the density, it suffices to consider (27) for $v\in C^1(K)$. Set $\tilde{v}=v\circ \Phi \in C^1(\tilde{K})$. Then,

$$\begin{aligned} \int _{\tilde{K}}\tilde{v}^2d\xi = \frac{{\text {meas}}_{d}(\tilde{K})}{{\text {meas}}_{d}({K})} \int _{{K}}{v}^2dx \le C \rho _K^{-d}\Vert v\Vert _{L^2(K)}^2 \end{aligned}$$

and

$$\begin{aligned} \int \int _{\tilde{K}\times \tilde{K}}\frac{~|\tilde{v}(\xi )-\tilde{v}(\eta )|^2}{|\xi -\eta |^{d+2s}}~d\xi d\eta&\le \left( \frac{{\text {meas}}_{d}(\tilde{K})}{{\text {meas}}_{d}({K})}\right) ^2 \int \int _{{K}\times {K}}\frac{~|v(x)-v(y)|^2}{~|B^{-1}x-B^{-1}y|^{d+2s}}~dxdy \\&\le C\rho _K^{-2d} \cdot \Vert B\Vert ^{d+2s}\int \int _{{K}\times {K}}\frac{~|v(x)-v(y)|^2}{~|x-y|^{d+2s}}~dxdy\\&\le Ch_K^{2s}\nu _2^d\rho _K^{-d} \int \ \int _{{K}\times {K}}\frac{~|v(x)-v(y)|^2}{~|x-y|^{d+2s}}~dxdy. \end{aligned}$$

Using those inequalities, we have

$$\begin{aligned} \Vert \tilde{v}\Vert _{L^2(e)}^2&= \frac{{\text {meas}}_{d-1}(e)}{{\text {meas}}_{d-1}(\tilde{e})} \int _{\tilde{e}}\tilde{v}(\xi )^2~d\xi \\&\le Ch_e^{d-1}\cdot \tilde{C}\left( \int _{\tilde{K}}\tilde{v}^2d\xi + \int \int _{\tilde{K}\times \tilde{K}}\frac{~|v(\xi )-v(\eta )|^2}{|\xi -\eta |^{d+2s}}~d\xi d\eta \right) .\\&\le C\nu _1^dh_e^{-1} \left( \Vert v\Vert _{L^2(K)}^2+h_K^{2s}|v|_{H^s(K)}^2 \right) , \end{aligned}$$

which completes the proof.