Three-Dimensional Lattice Boltzmann Model for the Complex Ginzburg–Landau Equation

Abstract

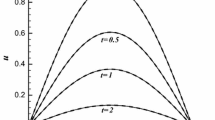
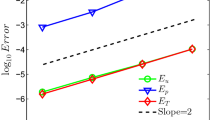
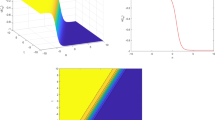
In this paper, a lattice Boltzmann model for the three-dimensional complex Ginzburg–Landau equation is proposed. The multi-scale technique and the Chapman–Enskog expansion are used to describe higher-order moments of the complex equilibrium distribution function and a series of complex partial differential equations. The modified partial differential equation of the three-dimensional complex Ginzburg–Landau equation with the third order truncation error is obtained. Based on the complex lattice Boltzmann model, some motions of the stable scroll, such as the scroll wave with a straight filament, scroll ring, and helical scroll are simulated. The comparisons between results of the lattice Boltzmann model with those obtained by the alternative direction implicit scheme are given. The numerical results show that this model can be used to simulate the three-dimensional complex Ginzburg–Landau equation.

Appendix

According to Eqs. (24a, 24b), we have

$$\begin{aligned} P_{ijk}^0&= \sum _\alpha {F_\alpha ^{eq} (\mathbf{x},t)e_{\alpha k} e_{\alpha j} e_{\alpha k} } =0,\end{aligned}$$

(31)

$$\begin{aligned} Q_{ijkm}^0&\equiv \sum _\alpha {F_\alpha ^{eq} (\mathbf{x},t)e_{\alpha i} e_{\alpha j} e_{\alpha k} e_{\alpha m} } =\frac{\lambda c^{2}}{(D+2)}(\delta _{ij} \delta _{km} +\delta _{ik} \delta _{jm} +\delta _{im} \delta _{jk} )\beta A(\mathbf{x},t).\nonumber \\ \end{aligned}$$

(32)

Denote

$$\begin{aligned} \phi ^{p}\equiv \sum _\alpha \Omega _\alpha ^{(p)} \end{aligned}$$

(33)

and

$$\begin{aligned} \psi _{k_1 ,k_2 ,\ldots ,k_n }^p \equiv \sum _\alpha {\Omega _\alpha ^{(p)} e_{\alpha k_1 } e_{\alpha k_2 } \cdots e_{\alpha k_n } },\quad n=1,2,3,\ldots ,\quad p=1,2,3,4. \end{aligned}$$

(34)

Assuming that the additional distribution $\phi ^{p}$ meets the following moments

$$\begin{aligned} \phi ^1&= H,\end{aligned}$$

(35)

$$\begin{aligned} \phi ^2&= \frac{1}{2}\frac{\partial H}{\partial t_0},\end{aligned}$$

(36)

$$\begin{aligned} \phi ^3&= (1-\tau )\frac{\partial }{\partial t_1}H + \frac{1}{6}\frac{\partial }{\partial t_0}\frac{\partial }{\partial t_0 }H,\end{aligned}$$

(37)

$$\begin{aligned} \phi ^4&= \frac{1}{2}(1-\tau )\frac{\partial }{\partial t_1}\frac{\partial H}{\partial t_0 }+\frac{1}{24}\frac{\partial ^{3}H}{\partial t_0^3}. \end{aligned}$$

(38)

We can obtain

$$\begin{aligned} \psi _i^1&= 0,\end{aligned}$$

(39)

$$\begin{aligned} \psi _i^2&= 0,\end{aligned}$$

(40)

$$\begin{aligned} \psi _i^3&= 0,\end{aligned}$$

(41)

$$\begin{aligned} \psi _{ij}^1&= -\frac{3C_3 \lambda \beta }{\tau C_2 }\delta _{ij} \frac{\partial A}{\partial t_0 },\end{aligned}$$

(42)

$$\begin{aligned} \psi _{ijk}^1&= 0, \end{aligned}$$

(43)

then

$$\begin{aligned} \Psi _{ij}^2&= -\frac{1}{\tau C_2 }\left[ \frac{3C_4 \lambda c^{2}\beta }{(D+2)}\nabla _0^2 A+\left( 6C_4 -3C_3 \frac{3C_3 }{C_2}\right) \lambda \beta \frac{\partial ^{2}}{\partial t_0^2 }A\right. \nonumber \\&\left. \qquad \qquad \,+\,(3C_3 -C_2 C_2 )\lambda \beta \frac{\partial }{\partial t_1 }A\right] \delta _{ij}. \end{aligned}$$

(44)

According to Eq. (13), the conservation law in time scale $t_0$ can be obtained as

$$\begin{aligned} \frac{\partial A}{\partial t_0 }=H. \end{aligned}$$

(45)

Also, we have

$$\begin{aligned} \frac{\partial H}{\partial t_0 }&= \frac{\partial H}{\partial u}\frac{\partial u}{\partial t_0 }+\frac{\partial H}{\partial v}\frac{\partial v}{\partial t_0 }=\hbox { Re }(H)\frac{\partial H}{\partial u}+\hbox { Im }(H)\frac{\partial H}{\partial v}\equiv \eta ,\end{aligned}$$

(46)

$$\begin{aligned} \frac{\partial ^{2}H}{\partial t_0^2}&= \frac{\partial }{\partial t_0 }\left[ \hbox {Re }(H)\frac{\partial H}{\partial u}+\hbox { Im }(H)\frac{\partial H}{\partial v}\right] =\hbox { Re }(H)\frac{\partial \eta }{\partial u}+\hbox { Im }(H)\frac{\partial \eta }{\partial v}\equiv \zeta ,\end{aligned}$$

(47)

$$\begin{aligned} \frac{\partial ^{3}H}{\partial t_0^3 }&= \frac{\partial }{\partial t_0 }\left[ \hbox {Re }(H)\frac{\partial \eta }{\partial u}+\hbox { Im }(H)\frac{\partial \eta }{\partial v}\right] =\hbox { Re }(H)\frac{\partial \zeta }{\partial u}+\hbox { Im }(H)\frac{\partial \zeta }{\partial v}. \end{aligned}$$

(48)

Summing over Eq. (14), then

$$\begin{aligned} \frac{\partial A}{\partial t_1 }\!+\!C_2 \left[ \frac{\partial }{\partial t_0 }\frac{\partial A}{\partial t_0 }\!+\!\frac{\partial }{\partial t_0 }\frac{\partial \pi _j^0 }{\partial x_{j0} }+\frac{\partial }{\partial x_{i0} }\frac{\partial \pi _i^0 }{\partial t_0 }\!+\!\frac{\partial }{\partial x_{i0} }\frac{\partial \pi _{ij}^0 }{\partial x_{j0} }\right] +\tau \frac{\partial }{\partial t_0 }\phi ^1 \!+\!\tau \frac{\partial }{\partial x_{i0} }\psi _i^1 \!=\!\phi ^2.\nonumber \\ \end{aligned}$$

(49)

According to the Eqs. (35), (36) and (39), we have

$$\begin{aligned} \frac{\partial A}{\partial t_1 }+\beta \nabla _0^2 A(\mathbf{x},t)=0. \end{aligned}$$

(50)

Taking $(13)+(14)\times \varepsilon$ and summing over $\alpha$, we have

$$\begin{aligned} \frac{\partial A}{\partial t}=\beta \nabla ^{2}A(\mathbf{x},t)+H+O(\varepsilon ^{2}), \end{aligned}$$

(51)

And other equations are

$$\begin{aligned} \frac{\partial H}{\partial t_1 }&= \frac{\partial H}{\partial u}\frac{\partial u}{\partial t_1 }+\frac{\partial H}{\partial v}\frac{\partial v}{\partial t_1 } = \hbox {Re }(\beta \nabla _0^2 A)\frac{\partial H}{\partial u} + \hbox {Im }(\beta \nabla _0^2 A)\frac{\partial H}{\partial v}\nonumber \\&\approx \hbox {Re }(\beta \nabla ^{2}A)\frac{\partial H}{\partial u} + \hbox {Im }(\beta \nabla ^{2}A)\frac{\partial H}{\partial v}. \end{aligned}$$

(52)

Summing over Eq. (15), then

$$\begin{aligned}&C_3 \left[ \frac{\partial }{\partial t_0 }\frac{\partial }{\partial t_0 }\frac{\partial A}{\partial t_0 }+3\frac{\partial }{\partial t_0 }\frac{\partial }{\partial t_0 }\frac{\partial \pi _i^0 }{\partial x_{i0} }+3\frac{\partial }{\partial t_0 }\frac{\partial }{\partial x_{i0} }\frac{\partial \pi _{ij}^0 }{\partial x_{j0} }+\frac{\partial }{\partial x_{i0} }\frac{\partial }{\partial x_{k0} }\frac{\partial \pi _{ijk}^0 }{\partial x_{j0}}\right] \nonumber \\&\quad +\, 2C_2 \frac{\partial }{\partial t_1 }\left( \frac{\partial A}{\partial t_0 }+\frac{\partial \pi _i^0 }{\partial x_{i0}}\right) +2C_2 \frac{\partial }{\partial x_{j1} }\left( \frac{\partial \pi _j^0 }{\partial t_0 }+\frac{\partial \pi _{ij}^0 }{\partial x_{i0}}\right) +\frac{\partial A}{\partial t_2 }\nonumber \\&\quad +\,\tau C_2 \left[ \frac{\partial }{\partial t_0 }\frac{\partial }{\partial t_0 }H+2\frac{\partial }{\partial t_0 }\frac{\partial }{\partial x_{j0} }\psi _j^1 +\frac{\partial }{\partial x_{i0} }\frac{\partial }{\partial x_{j0} }\psi _{ij}^1\right] \nonumber \\&\quad +\,\tau \frac{\partial }{\partial t_1 }H+\tau \frac{\partial }{\partial x_{j1} }\psi _j^1 +\tau \left( \frac{\partial }{\partial t_0 }\phi _0^2 +\frac{\partial }{\partial x_{i0}}\psi _i^2\right) =\phi ^3 \end{aligned}$$

(53)

According to the moments of the equilibrium distribution function, we have

$$\begin{aligned}&C_3 \left[ \frac{\partial }{\partial t_0 }\frac{\partial }{\partial t_0 }H + 3\lambda \beta \frac{\partial }{\partial t_0 }\frac{\partial }{\partial x_{j0} }\frac{\partial A}{\partial x_{j0} }\right] +2C_2 \frac{\partial }{\partial t_1 }H+2C_2 \lambda \beta \frac{\partial }{\partial x_{j1} }\frac{\partial A}{\partial x_{j0} }+\frac{\partial A}{\partial t_2}\nonumber \\&\quad +\,\tau C_2 \left[ \frac{\partial }{\partial t_0 }\frac{\partial }{\partial t_0 }H+\frac{\partial }{\partial x_{i0} }\frac{\partial }{\partial x_{j0} }\psi _{ij}^1\right] +\tau \frac{\partial }{\partial t_1 }H+\tau \frac{\partial }{\partial t_0 }\phi _0^2 +\tau \frac{\partial }{\partial x_{i0} }\psi _i^2 =\phi ^3\qquad \quad \end{aligned}$$

(54)

According to Eqs. (13), (37), (40) and (42), we obtain

$$\begin{aligned} \frac{\partial A}{\partial t_2 }+2C_2 \lambda \beta \frac{\partial }{\partial x_{j1} }\frac{\partial A}{\partial x_{j0}}=0. \end{aligned}$$

(55)

Taking $(13) + (14)\times \varepsilon + (15)\times \varepsilon ^{2}$ and summing over $\alpha$, we have

$$\begin{aligned} \frac{\partial A}{\partial t}=\beta \nabla ^{2}A(\mathbf{x},t)+H+O(\varepsilon ^{3}), \end{aligned}$$

(56)

and

$$\begin{aligned} \frac{\partial H}{\partial t_2 }=\frac{\partial H}{\partial u}\hbox {Re }\left( -2C_2 \beta \frac{\partial }{\partial x_{j1} }\frac{\partial A}{\partial x_{j0}}\right) +\frac{\partial H}{\partial v}\hbox {Im}\left( -2C_2 \lambda \beta \frac{\partial }{\partial x_{j1} }\frac{\partial A}{\partial x_{j0}}\right) . \end{aligned}$$

(57)

Summing over Eq. (16), then

$$\begin{aligned}&C_4\left[ \frac{\partial ^{4}A}{\partial t_0^4 }+ 4\frac{\partial ^{3}}{\partial t_0^3 }\frac{\partial }{\partial x_{i0} }\pi _i^0 +6\frac{\partial ^{2}}{\partial t_0^2 }\frac{\partial ^{2}}{\partial x_{i0} \partial x_{j0} }\pi _{ij}^0 +4\frac{\partial }{\partial t_0 }\frac{\partial ^{3}}{\partial x_{i0} \partial x_{j0} \partial x_{k0} }P_{ijk}^0\right. \nonumber \\&\left. \quad +\frac{\partial ^{4}}{\partial x_{i0} \partial x_{j0} \partial x_{k0} \partial x_{m0} }Q_{ijkm}^0\right] +3C_3 \frac{\partial }{\partial t_1 }\left[ \frac{\partial ^{2}A}{\partial t_0^2}+2\frac{\partial }{\partial t_0 }\frac{\partial }{\partial x_{i0} }M_i^0 +\frac{\partial ^{2}}{\partial x_{i0} \partial x_{j0} }\pi _{ij}^0\right] \nonumber \\&\quad +\,3C_3 \frac{\partial }{\partial x_{j1} }\left[ \frac{\partial ^{2}M_j^0 }{\partial t_0^2 }+2\frac{\partial }{\partial t_0 }\frac{\partial }{\partial x_{i0}}\pi _{ij}^0 +\frac{\partial ^{2}}{\partial x_{i0} \partial x_{k0} }P_{ijk}^0\right] +2C_2 \frac{\partial }{\partial t_2 }\left[ \frac{\partial A}{\partial t_0 }+\frac{\partial }{\partial x_{i0} }M_i^0\right] \nonumber \\&\quad +\,2C_2 \frac{\partial }{\partial x_{j2} }\left[ \frac{\partial M_j^0 }{\partial t_0 }+\frac{\partial }{\partial x_{i0}}\pi _{ij}^0 \right] +\frac{\partial A}{\partial t_3}+C_2 \left( \frac{\partial ^{2}A}{\partial t_1^2 }+2\frac{\partial }{\partial t_1 }\frac{\partial }{\partial x_{i1} }M_i^0 +\frac{\partial ^{2}}{\partial x_{i1} \partial x_{j1} }\pi _{ij}^0\right) \nonumber \\&\quad +\,\tau \left[ \frac{\partial \phi _0^1}{\partial t_2 }+\frac{\partial }{\partial x_{i2} }\psi _i^1\right] +\tau C_3 \left[ \frac{\partial ^{3}H}{\partial t_0^3 }+3\frac{\partial ^{2}}{\partial t_0^2 }\frac{\partial }{\partial x_{i0} }\psi _i^1 +3\frac{\partial }{\partial t_0 }\frac{\partial ^{2}}{\partial x_{i0} \partial x_{j0} }\psi _{ij}^1\right. \nonumber \\&\left. \quad +\frac{\partial ^{3}}{\partial x_{i0} \partial x_{j0} \partial x_{k0} }\psi _{ijk}^1\right] +2\tau C_2 \frac{\partial }{\partial t_1 }\left[ \frac{\partial H}{\partial t_0 }+\frac{\partial }{\partial x_{i0} }\psi _i^1\right] +2\tau C_2 \frac{\partial }{\partial x_{j1} }\left[ \frac{\partial \psi _i^1 }{\partial t_0 }+\frac{\partial }{\partial x_{i0} }\psi _{ij}^1\right] \nonumber \\&\quad +\,\tau C_2 \left[ \frac{\partial ^{2}}{\partial t_0^2 }\frac{1}{2}\frac{\partial H}{\partial t_0 }+2\frac{\partial }{\partial t_0 }\frac{\partial }{\partial x_{i0} }\psi _i^2 +\frac{\partial ^{2}}{\partial x_{i0} \partial x_{j0} }\psi _{ij}^2 \right] +\tau \frac{\partial }{\partial t_1 }\frac{1}{2}\frac{\partial H}{\partial t_0 }\nonumber \\&\quad +\,\tau \left[ \frac{\partial \phi _0^3 }{\partial t_0 }+\frac{\partial }{\partial x_{i0} }\psi _i^3\right] =\phi ^4. \end{aligned}$$

(58)

According to the moments of the equilibrium distribution, we have

$$\begin{aligned}&\frac{\partial A}{\partial t_3 } + C_4 \frac{\partial ^{4}}{\partial x_{i0} \partial x_{j0} \partial x_{k0} \partial x_{m0} }Q_{ijkm}^0 +\left[ 6C_4 -3C_3 \frac{3C_3 }{C_2 }\right] \lambda \beta \nabla ^{2}\frac{\partial ^{2}}{\partial t_0^2}A\nonumber \\&\quad +\,[3C_3 -C_2 C_2 ]\lambda \beta \nabla ^{2}\frac{\partial }{\partial t_1 }A +\left[ C_4 +\tau C_3 +\frac{1}{2}\tau C_2\right] \frac{\partial ^{3}H}{\partial t_0^3 }\nonumber \\&\quad +\left[ 3C_3 +2\tau C_2+\tau \frac{1}{2}\right] \frac{\partial }{\partial t_1 }\frac{\partial H}{\partial t_0 }+\tau C_2 \frac{\partial ^{2}}{\partial x_{i0} \partial x_{j0} }\psi _{ij}^2+\tau \frac{\partial \phi _0^3 }{\partial t_0} +3C_3 \frac{\partial }{\partial x_{j1} }\left[ 2\frac{\partial }{\partial t_0 }\frac{\partial }{\partial x_{i0} }\pi _{ij}^0\right] \nonumber \\&\quad +\,2C_2 \frac{\partial }{\partial t_2 }\frac{\partial A}{\partial t_0 } \!+\!\tau \frac{\partial H}{\partial t_2 }\!+\!2C_2 \frac{\partial }{\partial x_{j2} }\frac{\partial }{\partial x_{i0} }\pi _{ij}^0 \!+\!C_2 \left( \frac{\partial ^{2}}{\partial x_{i1} \partial x_{j1} }\pi _{ij}^0 \right) \!+\!2\tau C_2 \frac{\partial }{\partial x_{j1} }\frac{\partial }{\partial x_{i0} }\psi _{ij}^1\! =\!\phi ^4.\nonumber \\ \end{aligned}$$

(59)

According to Eqs. (37), (38), (42) and (44), we have

$$\begin{aligned} \frac{\partial A}{\partial t_3 }+(2C_2 +\tau )\frac{\partial H}{\partial t_2 }+2C_2 \frac{\partial }{\partial x_{j2} }\frac{\partial }{\partial x_{i0} }\pi _{ij}^0 +C_2 \left( \frac{\partial ^{2}}{\partial x_{i1} \partial x_{j1}}\pi _{ij}^0 \right) =0. \end{aligned}$$

(60)

Taking $(13) + (14)\times \varepsilon + (15)\times \varepsilon ^{2}+ (16)\times \varepsilon ^{3}$ and summing over $\alpha$, we have

$$\begin{aligned} \frac{\partial A}{\partial t}=\beta \nabla ^{2}A+H(A)+R+O(\varepsilon ^{4}), \end{aligned}$$

(61)

where

$$\begin{aligned} R&= -\varepsilon ^{3}(2C_2 +\tau )\left[ \frac{\partial H}{\partial u}\hbox {Re}\left( -2C_2 \lambda \beta \frac{\partial }{\partial x_{j1} }\frac{\partial A}{\partial x_{j0}}\right) \right. \nonumber \\&\left. +\,\frac{\partial H}{\partial v}\hbox { Im }\left( -2C_2 \lambda \beta \frac{\partial }{\partial x_{j1} }\frac{\partial A}{\partial x_{j0} }\right) \right] +O(\varepsilon ^{4}). \end{aligned}$$

(62)

Equation (62) is the complex Ginzburg–Landau equation with the third-order accuracy of truncation error and without error rebound effect, it is

$$\begin{aligned} \frac{\partial A}{\partial t}=\beta \nabla ^{2}B+H(A)+O(\varepsilon ^{3}). \end{aligned}$$

(63)