Skip to main content
SpringerLink
Log in

Optimal Error Estimates for the Fully Discrete Interior Penalty DG Method for the Wave Equation

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

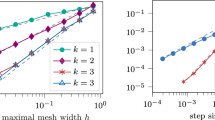
In Grote et al. (SIAM J. Numer. Anal., 44:2408–2431, 2006) a symmetric interior penalty discontinuous Galerkin (DG) method was presented for the time-dependent wave equation. In particular, optimal a-priori error bounds in the energy norm and the L 2-norm were derived for the semi-discrete formulation. Here the error analysis is extended to the fully discrete numerical scheme, when a centered second-order finite difference approximation (“leap-frog” scheme) is used for the time discretization. For sufficiently smooth solutions, the maximal error in the L 2-norm error over a finite time interval converges optimally as O(h p+1+Δt 2), where p denotes the polynomial degree, h the mesh size, and Δt the time step.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Ainsworth, M., Monk, P., Muniz, W.: Dispersive and dissipative properties of discontinuous Galerkin finite element methods for the second-order wave equation. J. Sci. Comput. 27, 5–40 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  2. Arnold, D.N., Brezzi, F., Cockburn, B., Marini, L.D.: Unified analysis of discontinuous Galerkin methods for elliptic problems. SIAM J. Numer. Anal. 39, 1749–1779 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  3. Baker, G.A.: Error estimates for finite element methods for second-order hyperbolic equations. SIAM J. Numer. Anal. 13, 564–576 (1976)

    Article  MATH  MathSciNet  Google Scholar 

  4. Baker, G.A., Dougalis, V.A., Serbin, S.M.: High order accurate two-step approximations for hyperbolic equations. RAIRO. Modél. Math. Anal. Numér. 13, 201–226 (1979)

    MATH  MathSciNet  Google Scholar 

  5. Bécache, E., Joly, P., Tsogka, C.: An analysis of new mixed finite elements for the approximation of wave propagation problems. SIAM J. Numer. Anal. 37, 1053–1084 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  6. Ciarlet, P.G.: The Finite Element Method for Elliptic Problems. North–Holland, Amsterdam (1978)

    MATH  Google Scholar 

  7. Cockburn, B.: Discontinuous Galerkin methods for convection-dominated problems. In: Barth, T., Deconink, H. (eds.) High-Order Methods for Computational Physics. Lect. Notes Comput. Sci. Engrg., vol. 9, pp. 69–224. Springer, Berlin (1999)

    Google Scholar 

  8. Cockburn, B., Karniadakis, G.E., Shu, C.-W.: The development of discontinuous Galerkin methods. In: Cockburn, B., Karniadakis, G.E., Shu, C.-W. (eds.) Discontinuous Galerkin Methods: Theory, Computation and Applications. Lect. Notes Comput. Sci. Engrg., vol. 11, pp. 3–50. Springer, Berlin (2000)

    Google Scholar 

  9. Cockburn, B., Shu, C.-W.: TVB Runge–Kutta local projection discontinuous Galerkin method for conservation laws II: General framework. Math. Comput. 52, 411–435 (1989)

    Article  MATH  MathSciNet  Google Scholar 

  10. Cockburn, B., Shu, C.-W.: Runge–Kutta discontinuous Galerkin methods for convection–dominated problems. J. Sci. Comput. 16, 173–261 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  11. Cohen, G., Joly, P., Roberts, J.E., Tordjman, N.: Higher order triangular finite elements with mass lumping for the wave equation. SIAM J. Numer. Anal. 38, 2047–2078 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  12. Cowsar, L.C., Dupont, T.F., Wheeler, M.F.: A-priori estimates for mixed finite element methods for the wave equations. Comput. Methods Appl. Mech. Eng. 82, 205–222 (1990)

    Article  MATH  MathSciNet  Google Scholar 

  13. Cowsar, L.C., Dupont, T.F., Wheeler, M.F.: A-priori estimates for mixed finite element approximations of second-order hyperbolic equations with absorbing boundary conditions. SIAM J. Numer. Anal. 33, 492–504 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  14. Diaz, J., Grote, M.J.: Energy conserving explicit local time-stepping for second-order wave equations. SIAM J. Sci. Comput. (in press)

  15. Dupont, T.: L 2-estimates for Galerkin methods for second-order hyperbolic equations. SIAM J. Numer. Anal. 10, 880–889 (1973)

    Article  MATH  MathSciNet  Google Scholar 

  16. Falk, R.S., Richter, G.R.: Explicit finite element methods for symmetric hyperbolic equations. SIAM J. Numer. Anal. 36(3), 935–952 (1999)

    Article  MathSciNet  Google Scholar 

  17. French, D.A., Peterson, T.E.: A continuous space-time finite element method for the wave equation. Math. Comput. 66, 491–506 (1996)

    MathSciNet  Google Scholar 

  18. Gekeler, E.: Linear multi-step methods and Galerkin procedures for initial boundary value problems. SIAM J. Numer. Anal. 13, 536–548 (1976)

    Article  MATH  MathSciNet  Google Scholar 

  19. Geveci, T.: On the application of mixed finite methods to the wave equation. RAIRO Modél. Math. Anal. Numér. 22, 243–250 (1988)

    MATH  MathSciNet  Google Scholar 

  20. Grote, M.J., Schneebeli, A., Schötzau, D.: Discontinuous Galerkin finite element method for the wave equation. SIAM J. Numer. Anal. 44, 2408–2431 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  21. Grote, M.J., Schneebeli, A., Schötzau, D.: Interior penalty discontinuous Galerkin method for Maxwell’s equations: Energy norm error estimates. J. Comput. Appl. Math. 204, 375–386 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  22. Grote, M.J., Schneebeli, A., Schötzau, D.: Interior penalty discontinuous Galerkin method for Maxwell’s equations: Optimal L 2-norm error estimates. IMA J. Numer. Anal. 28, 440–468 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  23. Hesthaven, J.S., Warburton, T.: Nodal high-order methods on unstructured grids I: Time-domain solution of Maxwell’s equations. J. Comput. Phys. 181, 186–221 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  24. Houston, P., Jensen, M., Süli, E.: hp-discontinuous Galerkin finite element methods with least-squares stabilization. J. Sci. Comput. 17, 3–25 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  25. Hughes, T.: The Finite Element Method: Linear Static and Dynamic Finite Element Analysis. Prentice Hall, New York (1987)

    MATH  Google Scholar 

  26. Monk, P., Richter, G.R.: A discontinuous Galerkin method for linear symmetric hyperbolic systems in inhomogeneous media. J. Sci. Comput. 22–23, 443–477 (2005)

    Article  MathSciNet  Google Scholar 

  27. Rivière, B., Wheeler, M.F.: Discontinuous finite element methods for acoustic and elastic wave problems I: Semi-discrete error estimates. Technical Report 01-02, TICAM, UT Austin (2001)

  28. Rivière, B., Wheeler, M.F.: Discontinuous finite element methods for acoustic and elastic wave problems. In: ICM2002-Beijing Satellite Conference on Scientific Computing. Contemporary Mathematics, vol. 329, pp. 271–282. Am. Math. Soc., Providence (2003)

    Google Scholar 

  29. Safjan, A., Oden, J.T.: High-order Taylor-Galerkin and adaptive h−p methods for second-order hyperbolic systems: Applications to elastodynamics. Comput. Methods Appl. Mech. Eng. 103, 187–230 (1993)

    Article  MATH  MathSciNet  Google Scholar 

  30. Shubin, G.R., Bell, J.: A modified equation approach to constructing fourth-order methods for acoustic wave propagation. SIAM J. Sci. Comput. 8, 135–151 (1987)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, University of Basel, Rheinsprung 21, 4051, Basel, Switzerland

    Marcus J. Grote

  2. Mathematics Department, University of British Columbia, 121-1984 Mathematics Road, Vancouver, V6T 1Z2, Canada

    Dominik Schötzau

Authors
  1. Marcus J. Grote
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Dominik Schötzau
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Marcus J. Grote.

Additional information

The research of M.J. Grote was supported in part by the Swiss National Science Foundation (SNF).

The research of D. Schötzau was supported in part by the Natural Sciences and Engineering Research Council of Canada (NSERC).

Rights and permissions

Reprints and permissions

About this article

Cite this article

Grote, M.J., Schötzau, D. Optimal Error Estimates for the Fully Discrete Interior Penalty DG Method for the Wave Equation. J Sci Comput 40, 257–272 (2009). https://doi.org/10.1007/s10915-008-9247-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-008-9247-z

Keywords

Search

Navigation