Unsteady triple diffusive oscillatory flow in a Voigt fluid

Abstract

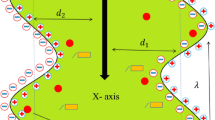
Convective energy and mass transfer in a non-Newtonian fluid layers a wide-spread physical phenomenon in natural and technical systems. Triple diffusive convection plays a crucial role in chemical engineering by enabling the understanding and optimisation of mass transfer processes involving multiple components. It is essential for designing efficient separation systems, optimising catalysts, predicting reaction kinetics, and improving environmental processes. The motivation of this paper is to explore an Oscillatory flow of a triple diffusive convection in a Voigt fluid layer. The governing partial differential equations are transformed into coupled ordinary differential equations with the help of the oscillation technique. The study emphasises the effects of known physical parameters, such as the thermal Grashof number, solutal Grashof number, Prandtl number, Lewis numbers and Voigt fluid parameters on velocity, temperature, concentrations and rate of heat and mass transfers. In particularly, the study finds that skin friction increases on both channel plates with increasing injection on the heated plate.

Appendix: Coefficients of Eqs. (19)–(22) are

$A_{0}$	$- \frac{1}{{e^{{m_{2} }} - e^{{m_{1} }} }}$
$B_{0}$	$\frac{1}{{e^{{m_{2} }} - e^{{m_{1} }} }}$
$A_{1}$	$\frac{{B_{1} \left( {\gamma m_{4} - 1} \right) + n_{1} - n_{0} }}{{1 - m_{3} \gamma }}$
$B_{1}$	$- \frac{{n_{2} (1 - m_{3} \gamma ) + \left( {n_{1} - n_{0} } \right)e^{{m_{3} }} }}{{e^{{m_{4} }} (1 - m_{3} \gamma ) + \left( {\gamma m_{4} - 1} \right)e^{{m_{3} }} }}$
$n_{0}$	$Q_{0} + Q_{1} + Q_{2} + Q_{3} + Q_{4}$
$n_{1}$	$\gamma \left( {Q_{1} m_{1} + Q_{2} m_{2} + Q_{3} m_{5} + Q_{4} m_{6} } \right)$
$n_{2}$	$Q_{0} + Q_{1} e^{{m_{1} }} + Q_{2} e^{{m_{2} }} + Q_{3} e^{{m_{5} }} + Q_{4} e^{{m_{6} }}$
$m_{1}$	$\frac{{ - s\Pr + \sqrt {\left( {s\Pr } \right)^{2} + 4\Pr i\omega } }}{2}$
$m_{2}$	$\frac{{ - s\Pr - \sqrt {\left( {s\Pr } \right)^{2} + 4\Pr i\omega } }}{2}$
$m_{3}$	$\frac{{ - s + \sqrt {s^{2} + 4\left( {1 + i\omega \,V_{f} } \right)i\omega } }}{{2\left( {1 + i\omega \,V_{f} } \right)}}$
$m_{4}$	$\frac{{ - s - \sqrt {s^{2} + 4\left( {1 + i\omega \,V_{f} } \right)i\omega } }}{{2\left( {1 + i\omega \,V_{f} } \right)}}$
$m_{5}$	$\frac{{ - sLe_{1} + \sqrt {\left( {sLe_{1} } \right)^{2} + 4Le_{1} i\omega } }}{2}$
$m_{6}$	$\frac{{ - sLe_{1} - \sqrt {\left( {sLe_{1} } \right)^{2} + 4Le_{1} i\omega } }}{2}$
$m_{7}$	$\frac{{ - sLe_{2} + \sqrt {\left( {sLe_{2} } \right)^{2} + 4Le_{2} i\omega } }}{2}$
$m_{8}$	$\frac{{ - sLe_{2} - \sqrt {\left( {sLe_{2} } \right)^{2} + 4Le_{2} i\omega } }}{2}$
$Q_{0}$	$\frac{\xi }{i\omega }$
$Q_{1}$	$- \frac{{GrtA_{0} }}{{m_{1}^{2} \left( {1 + i\omega \,V_{f} } \right) + sm_{1} - i\omega }}$
$Q_{2}$	$- \frac{{GrtB_{0} }}{{m_{2}^{2} \left( {1 + i\omega \,V_{f} } \right) + sm_{2} - i\omega }}$
$Q_{3}$	$\frac{{Grc_{1} A_{2} }}{{m_{5}^{2} \left( {1 + i\omega \,V_{f} } \right) + sm_{5} - i\omega }}$
$Q_{4}$	$\frac{{Grc_{1} B_{2} }}{{m_{6}^{2} \left( {1 + i\omega \,V_{f} } \right) + sm_{6} - i\omega }}$
$Q_{5}$	$\frac{{Grc_{2} A_{3} }}{{m_{7}^{2} \left( {1 + i\omega \,V_{f} } \right) + sm_{7} - i\omega }}$
$Q_{6}$	$\frac{{Grc_{2} B_{3} }}{{m_{8}^{2} \left( {1 + i\omega \,V_{f} } \right) + sm_{8} - i\omega }}$