1 Introduction

Owing to their exceptional characteristics and applications, nanomaterials and nanostructures have generated considerable research interest. In particular, carbon exhibits a variety of nanoscale structures including the carbon nanotube (CNT),carbon graphene sheet, carbon fullerene, carbon nanocone (CNC), and carbon torus (CT). These structures have demonstrated their applicability across a diverse range of fields, including censoring and actuation systems, energy storage, biotechnologies, composite materials, and microelectronics [1].

Furthermore, boron nitride (BN) nanoscale structures are a topic of interest because they are similar to carbon-based nanoscale structures. BN is a chemical compound that is isoelectronic to carbon. Because of the similarity between B-N and C-C bonds where both have 12 electrons on the two atoms. Boron has the atomic number 5, so it has 5 protons and 5 electrons while nitrogen has seven protons and seven electrons. Whereas carbon has six protons and six electrons. Additionally, atomic radii of carbon, boron and nitrogen are all similar and boron nitride has several structures that are like the different structures of carbon [2]. The exceptional properties of these structures contribute to their extensive applications in enhancing nanoscale devices. [3] [4]. BN has a hexagonal structure wherein nitrogen and boron atoms bound by strong covalent bonds. Other forms of BN-based nanostructures include the BN torus (BNT), BN nanotube (BNNT), BN fullerene (BN fullerene), BN graphene (BN graphene), and BN nanocone (BNNC) [5, 6].

BN nanoscale structures exhibit comparable thermal and mechanical properties, to those of carbon nanoscale structures, such as low density, high thermal conductivity, membrane stiffness, and high tensile strength. In addition, their atomic composition imbues them with certain unique features, including chemical stability at high temperatures and enhanced oxidation resistance. Consequently, BN nanostructures have exceptional applications in optoelectronics, energy storage, biomedical medicine, electronics, and nanosemiconductor devices [1, 7,8,9,10,11].

BNNTs were discovered in 1994 as tube structures of a hexagonal lattice comprising nitrogen and boron atoms. The special properties of these structures have attracted considerable scientific attention among scientists. For example, BNNTs are stable at temperatures up to \(800^{\circ }C\) in air, and function as electrical insulators with a band gap for 6 eV. In addition, they exhibit exceptional hydropbobicity, and piezoelectric properties, making them beneficial for spintronic devices. Furthermore, they may serve as excellent thermal conductors for Young’s moduli exceeding 1.3 TPa [12].

The BNT is a structure formed by bending a BNNT and connecting its ends together. Extensive research has been conducted on this nanostructure owing to its unique structure and properties. BNTs have been employed for applications such as in nano-antennas susceptible to high frequency electromagnetic signals and ultrafast optical filters [5, 13].

A conjunction between two nanoscale structures yields a new enhanced nanostructure that may feature unique properties, increasing its applicability [14,15,16,17]. These new structures may exhibit improved electrochemical and physiochemical performance over that of their constituent structures, as is the case for nanosensors and nanooscillators. Specific applications of hybrid nanostructures include probes for scanning tunnelling microscopy, energy storage, and carriers to drug delivery [4]. Consequently, various methods have been developed to model the process of conjoining nanostructures. One such method is elastic energy, wherein the curvature square is minimized to obtain a Euler-Lagrange equation, which is used to identify the area of connectivity between the two structures. Elastic energy enables the conjunction of CNTs with fulerenes, fullerenes with graphenes, CNCs with fullerenes, CNTs with graphenes, fullerenes with fullerenes, CNCs with CNCs, CNTs with CNCs and CNCs with fullerenes, as described in [18,19,20], and [21]. Another method used to link carbon nanostructures is known as the Willmore energy. This approach assumes that the rotational curvature must be considered, as it has a similar size to that of the axial curvature. As a natural generalization of elastic energy, the Willmore energy uses both curvatures to detect the surface conjunction of two nanostructures. Furthermore, this energy has been used to model surface bending in the deformation of cell membranes [32], a segmentation of spiral vertebrae [33], and behavior of red blood cells [34].This method enables the conjunction of CNTs with fullerenes, fullerenes with fullerenes, and CNTs with CTs. [13, 22].

Because BN nanostructures are similar to carbon nanoscructures they have been connected using the elastic energy method. For example, BN structures have been conjoined with graphene, and BNNTs has been conjoined with CNCs [23, 24]. Another study used the Willmore energy method to connect fullerenes with BNNTs and BNTs, as well as other fullerenes. [25].

Even though the literature has addressed a number of conjoining nanostructures, to the best of my knowledge, there is a lack of research on conjoining BN nanostructures. In the present study, we expand the use of the two mentioned methods to model the conjunction between BNNTs and BNTs. We note that the joining curve is symmetric for both models, allowing us to match gradients between the two BN nanostructures. Additionally, as demonstrated in [26], these energies were used to conjoin the corresponding carbon nanostructures. In addition, this approach could further examine other BN structures, for examples helical hexagons [27], unusual helices [28], nanoneedles, spiral graphite [29], laterally extended helices [30] and Rod-Like [31]. It also contribute to a deeper understanding of other features of boron nitride structures.

We employed classical applied mathematics to formulate an ideal structure that cannot be achieved experimentally. The objective was to accommodate the primary characteristics encapsulating the dominant physical effects. Thus, we visualized a real physical system in terms of the departures from an ideal model. In particular, we considered two models: one that depends on the axial curvature only, and another that depends on the mean curvature using the Willmore energy.

The following section presents fundamental equations that model the region of connectivity between BN nanostructures. Section 3 presents results, with Sects. 3.1 and 3.2 focusing on results obtained using the elastic and Willmore energies, respectively. Finally, Sect. 4 concludes the paper.

2 Models

The following subsections introduce the theoretical background of this study. In particular, the calculus of variations is utilized to determine the joining curve between two nanoscale BN structures, whereas the Willmore energy function is used to identify the joining surface.

2.1 Torus structure

Fig. 1
figure 1

Structure of torus

Full size image

The torus structure, illustrated in Fig. 1, can be expressed in Cartesian coordinates as

$$\begin{aligned} \left( \sqrt{x^2 + y^2}-R\right) ^2 + z^2=a^2. \end{aligned}$$

Following a transformation, we obtain

$$\begin{aligned} x(\phi , \theta )&= (R + a \cos \phi ), \cos \theta \\ y(\phi , \theta )&= (R + a \cos \phi ), \sin \theta \\ z(\phi , \theta )&= a \sin \phi , \end{aligned}$$

when \(\theta \) and \(\phi \) are the polar and azimuthal angles on the x-axis and xy-plane, respectively, R denotes the major radius of the torus, and a denotes the minor radius of the torus. Thus, the equation of the torus in cylindrical coordinates is

$$\begin{aligned} (r-R)^2 + z^2=a^2. \end{aligned}$$
(1)

2.2 Elastic energy

Fig. 2
figure 2

Joining curve in elastic energy

Full size image

We assume that a nanoscale torus is symmetric around the z-axis with a minor radius a and major radius R. In contrast, the tubular BN nanostructure is placed with its axis colinear to the y-axis with radius \(r_0\), where \(y_0\) is unknown starting distance above xz-plane. If both nanostructures are considered rotationally symmetric around the y-axis, \(y_0\) will be situated on the two-dimensional xy-plane. The joining curve joins the tube at \((r_0, y_0)\) and the torus at \((a\cos \psi ,a\sin \psi )\) with a specific arc length l,  as illustrated in Fig. 2. We find \(y=y(x)\) corresponding to an element of arc length ds,  which is the desired curve that minimizes J[y] of the form

$$\begin{aligned} J[y]=\int _0^l \kappa _a ^2 ds + \lambda \int _0 ^l ds, \end{aligned}$$

where \(\kappa _a\) is the axial curvature, \(\lambda \) is a Lagrange multiplier that corresponds to the fixed length constraint, l is the prescribed length of the curve, and the rotational curvature \(\kappa _r\) is equal to zero.

Using the same calculations as in [26], the curvature can be defined as

$$\begin{aligned} \kappa _a = \pm \left( \lambda + \frac{\alpha }{(1+y'^2)^\frac{1}{2}}\right) ^{\frac{1}{2}}. \end{aligned}$$
(2)

2.3 Willmore energy

Fig. 3
figure 3

Catenoid surface

Full size image

The mean curvature can be written in terms of the rotational curvature \(\kappa _r\) and the axial curvature \(\kappa _a\) that is called Willmore energy function, given by

$$\begin{aligned}&J[y]=\int H^2 d\mu + \lambda \int d\mu , \end{aligned}$$

where \(H=(\kappa _a + \kappa _r)\) indicates the mean curvature, obtained as the sum of two curvatures, \(\lambda \) is a Lagrange multiplier corresponding to an area constraint, and \(d\mu \) is an area element. Assuming a connection with the catenoid surface \(S=\{ (x,y,z): x = r \cos \theta , y = r \sin \theta , z = f(r)\}\) and \(0< r \le m,\) where m is a constant representing the distance from the central axis to the surface in the radial direction (see Fig. 3), the mean curvature can be expressed as

$$\begin{aligned}&H=\kappa _a+\kappa _r=-\frac{f''(r)}{(1+f'^2(r))^{\frac{3}{2}}}-\frac{f'(r)}{r\sqrt{f'^2(r)+1}}. \end{aligned}$$

In the case of \(H=0,\) this yields an absolute minimizer of the Willmore energy. Thus, the general solution can be written as

$$\begin{aligned}&f(r)= \pm \frac{\ln \left( A r +\sqrt{A^2 r^2 -1} \right) }{A}+ B, \end{aligned}$$

where A and B are arbitrary constants. Furthermore, this solution may be expressed as:

$$\begin{aligned}&f(r)= \pm \frac{\cosh ^{-1} (A r)}{A} + B. \end{aligned}$$

This function describes the connectivity surface between two nanostructures as a catenoid. Note that the positive value indicates the upper catenoid and the negative value indicates the lower catenoid. Refer to [22] for additional details pertaining to the derivation.

3 Results

3.1 Conjunction based on elastic energy

In this subsection, the joining curve between a BNNT and BNT is determined using elastic energy. By setting \(\tan \theta =y'\)in (2), we obtain \(\kappa _a=(\lambda + \alpha \cos \theta )^{1/2}.\) Because the curvature can be defined as \(\kappa _a =\frac{y''}{(1+y'^2)^{3/2}}\), we may consider \(y'\) we have

$$\begin{aligned} \frac{dy}{d\theta }=\frac{\sin \theta }{(\lambda + \alpha \cos \theta )^{1/2}}, \end{aligned}$$

and

$$\begin{aligned} \frac{dx}{d\theta }=\frac{\cos \theta }{(\lambda + \alpha \cos \theta )^{1/2}}. \end{aligned}$$

Referring to [21] and considering the boundary condition at the joining point \((r_0, y_0)\) we have

$$\begin{aligned} r_0&= a \cos \psi -\beta \{2[E(\phi _1, k)-E(\phi _0, k)]-[F(\phi _1, k)-F(\phi _0, k)]\},\nonumber \\ y_0&=2\beta k (\cos \phi _0-\cos \phi _1)+ a\sin \psi , \end{aligned}$$
(3)

where \(F(\phi ,k)\) and \(E(\phi ,k)\) are the usual Legendre incomplete elliptic integrals of the first and second kinds, respectively. Thus, we obtain \(k=[\frac{\lambda + \alpha }{2\alpha }]^{1/2}\), \(\phi _0=\sin ^{-1} (\sqrt{(1-\sin \psi )/2k^2}),\) and \(\phi _1=\sin ^{-1} (\frac{1}{\sqrt{2}k})\).

By considering the equation of the arc length constraint l

$$\begin{aligned} l=\int _{r_0}^{a \cos \psi } (1+y'^2)^{1/2}dx, \end{aligned}$$

we use \(y'=\tan \theta \) to obtain the following:

$$\begin{aligned} l=\beta [F(\phi _1,k),F(\phi _0,k)]. \end{aligned}$$
(4)

Substituting (4) into (3) yields

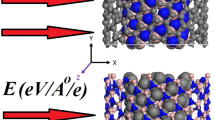
$$\begin{aligned} \mu =2\left( \frac{E(\phi _1, k)-E(\phi _0, k)}{F(\phi _1, k)-F(\phi _0, k)}\right) -1, \end{aligned}$$
(5)

where a, \(r_0\), \(\psi ,\) and l are prescribed values, as detailed in [13]. Thus, we have \(\mu =(a\cos \psi -r_0)/l\). Solving (5) for k, we can substitute the result into (4) to find \(\beta \), which in turn is substituted into (3) to find the height \(y_0\). Consequently, the joining curve between BNNT and BNT using elastic energy is obtained as shown in Fig. 4

Fig. 4
figure 4

2D joining between BN tube and torus using elastic energy

Full size image

3.2 Conjunction based on Willmore energy

Fig. 5
figure 5

Joining curve in Willmore energy

Full size image

In this subsection, the region of connectivity between a BNNT and BNT is identified by minimizing the Willmore energy. Subsequently, a section of a catenoid surface is utilized to connect the two nanostructures in a 3D model.

We consider the lower part of a catenoid to vertically join the nanostructures. The equation for this section is expressed as

$$\begin{aligned}&f(r)= - \frac{\cosh ^{-1} (A r)}{A} + B, \end{aligned}$$
(6)

where the radius \(r=r_0\) of the tube is a prescribed value. Additionally, the equation of the torus can be written as

$$\begin{aligned} z^2=a^2-(r-R)^2, \end{aligned}$$

which yields

$$\begin{aligned} z= C+\sqrt{a^2-(r-R)^2}, \end{aligned}$$
(7)

where C is a constant identified by the position of the nanotorus along the negative z-axis. We assume that the tube will join the neck of catenoid at the point \((r, z)=(r_0, 0)\), whereas the lower part of catenoid will join the torus at \((r, z)=(r_t, z_t),\) as shown in Fig. 5. The gradient at the point \((r_0,0)\) is \(\infty \) and \(f(r)=0,\) that is,

$$\begin{aligned} B = \frac{\cosh ^{-1}(A r_0)}{A}. \end{aligned}$$

Thus, the gradient of the catenoid becomes

$$\begin{aligned} \frac{d f(r)}{dr}= -\frac{1}{\sqrt{A^2 r^2 -1}}=\infty , \end{aligned}$$

so that \(A=\frac{1}{r_0}\) and \(B=0\). At the other point of connectivity \((r_t,z_t),\) we have

$$\begin{aligned} -\frac{\cosh ^{-1}(A r_t)}{A}&= C +\sqrt{a^2-(r_t-R)^2},\\ C&=-r_0 \cosh ^{-1}\left( \frac{r_t}{r_0}\right) -\sqrt{a^2-(r_t-R)^2}. \end{aligned}$$

Following gradient matching, we obtain

$$\begin{aligned} -\frac{1}{\sqrt{A^2 r_t^2 -1}}=\frac{r_t-R}{\sqrt{a^2-(r_t-R)^2}}. \end{aligned}$$

Using \(A=\frac{1}{r_0},\) and \(r_t=\frac{\sqrt{4ar_0+R}+R}{2},\) we then obtain

$$\begin{aligned} C=-r_0 \cosh ^{-1} \frac{\sqrt{4ar_0+R}+R}{2r_0}-\sqrt{a^2 - \left( \frac{\sqrt{4ar_0+R}+R}{2} \right) ^2}. \end{aligned}$$

Because a,  \(r_0,\) and R are prescribed values, the connection between the two nanostructures using a catenoid is obtained as depicted in Fig. 6

Fig. 6
figure 6

3D connection between BNNT and BNT using Willmore energy

Full size image

4 Conclusion

In this study, conventional applied mathematical modeling was employed for the essentially discrete problem of identifying the connection profiles between a BNNT and BNT. The produced nanostructures may be useful in the design of probes for scanning tunneling microscopy, as well as other nanoscale devices. Two models were used under the assumption that both nanostructures were rotationally symmetric: elastic energy and Willmore energy. Using the calculus of variations, the joining curve depends on minimization of elastic energy. Furthermore, this case can be considered as a 2D problem on the \(xy-\) plane, with the prescribed arc length assumed to connect the BN nanostructures. In addition, as the catenoid is an absolute minimizer resulting from Willmore energy, its neck is used to conjoin the BN nanostructures, yielding a 3D configuration of the hybrid structure. Using these models, we simulated the conjunction of the BNNT and BNT nanostructures. The objective of this study was to formulate the underlying axially symmetric model as a reference basis for the comparison of real physical structures. Although there are no experimental or computational results for comparison, the simple models provide meaningful approximations to complex structures, and therefore may be useful in future studies pertaining to this problem. Finally, different BN nanostructures may be modeled by considering the same methods, which is a potential future direction of research.