1 Introduction

In recent years, carbon fiber usage has grown in a variety of applications in order to meet the demand of different industries such as aerospace, sporting goods, medical, automobile, and many others. This considerable interest in carbon fibers is mainly due to its exceptional properties [1]. Their extremely high strength and stiffness, combined with excellent thermal conductivity and low density, have made carbon fibers the material of choice to use in high performance composite structures [2].

It is well known in the literature that these unique properties of carbon fibers are the result of the fiber microstructure, in both the axial and transverse directions [3,4,5,6]. However, from a more general point of view, one should also consider the organic precursors and experimental methods, since both directly influence the formation of the microstructure, hence its final properties. There are various precursors that can be used to produce carbon fibers, nevertheless, nowadays only the PAN (polyacrylonitrile), Pitch, and Rayon precursors are being employed for large-scale production of carbon fibers. The current times carbon fiber market is dominated by PAN because its resulting carbon fibers present higher tensile and compressive strengths than other classes of fiber [7, 8]. On the other hand, for applications that demand high stiffness, chemical stability, excellent thermal and electrical conductivity, the pitch-based carbon fibers are often used. The rayon-based carbon fibers possess extremely low thermal conductivity and are used in quite specific applications as rocket nozzle, heat shields and missile reentry vehicle nosecones. Although the majority of all carbon fibers used today are made from the PAN precursor, there has been a particular interest in the production of pitch-based carbon fibers owing to their excellent properties and raw material reasonable cost. The pitch-based fiber usage has been growing rapidly in recent decades, boosted by significant improvement in the performance/price ratio, which has been providing an accentuated penetration of their composites into applications formerly held by PAN fibers [1].

Actually, it is very complicated to construct theoretical models for carbon fibers within the continuum mechanics framework, because a huge number of experimental variables and boundary conditions must be taken into account for the mathematical modeling. However, there is a carbon fiber manufacturing step, called melt spinning, during which the basic characteristics of the fibers are molded, namely their microstructure and orientation of graphite crystals. In this step, the number of variables and boundary conditions for describing the fibers are reduced and hence, the mathematical modeling becomes easier [9, 10]. Particularly, the orientation of crystals is most noticeable in the melt spinning step of high-performance mesophase pitch-based carbon fibers. For producing such fibers, an anisotropic pitch (also called mesophase mixture) is used to obtain precursor fibers with specific microstructure and crystal orientation [11, 12]. In general, the precursor fibers produced during this step already exhibit prominent properties of the carbon fibers, as for example, a high tensile strength and elastic modulus, which are improved in subsequent production steps [13]. Furthermore, such properties highly depend on the crystal orientation in the mesophase pitch, as experimentally demonstrated by Duhan and Eddie [14]. Thus, by proposing a theoretical approach for mesophase pitch-based carbon fibers, first at all it is important to understand how the crystals orientation affects the properties of the precursor carbon fibers.

To control fiber properties, the importance of understanding the morphology evolution during the melt spinning process is unquestionable. However, experiments or simulations on the morphology evolution and formation are rarely reported. Moreover, investigations describing the evolution of nanometric-size microstructures, as microdomains, are almost non-existent. Therefore, in order to determine how microdomains are formed, as well as their behavior within the mesophase pitch, a mesoscopic continuum approach (coarse-grained model) is proposed in this paper. In addition, evolution equations for the density and orientation of the crystalline microdomains are also deduced. The employed approach is based on the continuum mesoscopic thermodynamics [15], developed originally for liquid crystals [16,17,18,19,20]. The idea is to take into account the mesoscopic distribution function for molecular aggregates.

The outline of the present paper is the following: Below in Sect. 2, the mesoscopic continuum approach is introduced. In Sect. 3 we develop the evolution equations for the density and orientation of the crystalline microdomains. In Sect. 4 the microdomain production is discussed in terms of the kinetic theory of crystallization. Section 5 presents some estimates obtained from the equations deduced in Sect. 3. The paper ends with a brief conclusion in Sect. 6.

2 Mesoscopic continuum approach

2.1 Mesoscopic space

Let \({\varvec{{m}}}\in {\mathcal {D}}^{i}\) be a set of additional variables in a Euclidean topological manifold \({\mathcal {D}}^{i}\) with dimension i, on which an integration can be defined. One defines the mesoscopic space \({\mathcal {M}}^{n}\) of dimension \(n=i+4\) by

$$\begin{aligned} {\mathcal {M}}^{n}={\mathcal {D}}^{i}\times {\mathbb {R}}^{3}\times {\mathbb {R}}^{1}, \quad \text {where}\quad ({\varvec{{m}}}, {\varvec{{x}}}, t)\in {\mathcal {M}}^{n}. \end{aligned}$$
(1)

Here, the manifold \({\mathcal {D}}^{i}\) is given by the domain of \({\varvec{{m}}}\), which represents the internal degrees of freedom of the system. Thus, the choice of \({\mathcal {D}}^{i}\) depends on the complex system under consideration. If the internal structure is known, then the nature of \({\mathcal {D}}^{i}\) is clear.

The mesoscopic concept also introduces a mesoscopic distribution function (MDF)

$$\begin{aligned} f({\varvec{{m}}}, {\varvec{{x}}}, t)\equiv f(\cdot ),\qquad (\cdot )\equiv ({\varvec{{m}}}, {\varvec{{x}}}, t)\in {\mathcal {M}}^{n}. \end{aligned}$$
(2)

This function gives the probability density, that the additional variable takes the value \({\varvec{{m}}}\) in the continuum element around position x at time t. The distribution function is normalized, that is,

$$\begin{aligned} \int f({\varvec{{m}}}, {\varvec{{x}}}, t)\,d{\mathcal {D}}=1, \end{aligned}$$
(3)

because it is sure, that the additional variable will take any of the possible values.

Even though the mesoscopic concept introduces a distribution function, the theory itself is not of microscopic nature, because it is not a priori assumed any kind of interaction between the particles unlike, for example, in the kinetic theory of gases.

In particular, the mesoscopic mass density \(\rho (\cdot )\) is defined by using the MDF:

$$\begin{aligned} \rho (\cdot ):=\rho ({\varvec{{x}}}, t)f({\varvec{{m}}}, {\varvec{{x}}}, t),\quad \text {where}\quad \rho ({\varvec{{x}}}, t)=\int \rho (\cdot )\,d{\mathcal {D}} \end{aligned}$$
(4)

The mesoscopic mass density considers only particles with the particular value \({\varvec{{m}}}\), whereas the macroscopic mass density ’counts’ all particles in that volume element.

Furthermore, other mesoscopic fields can be obtained in addition to the mass density, as for example, mesoscopic material velocity \({\varvec{{v}}}(\cdot )\), spin \({\varvec{{s}}}(\cdot )\), internal energy \(\varepsilon (\cdot )\) etc [21].

2.2 Orientational balances

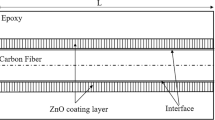
The subject of this study is the mesophase pitch whose primitive microstructural elements (crystals, microdomains, molecules, etc.) possess uniaxial symmetry. In the literature of carbon fibers [1, 7, 8], the mesophase pitch has been defined as a carbonaceous material presenting optical anisotropy and discotic liquid crystalline structural properties. Hence, the mesophase pitch can be regarded as a kind of liquid crystalline material that shows both thermotropic and lyotropic properties. According to Muschik et al. [22, 23], a liquid crystal is composed of differently orientated particles with an elongated or planar shape. In order to describe the internal order of particles with elongated or planar shape within the continuum mesoscopic framework, one defines \({\mathcal {D}}^{i}={\mathfrak {S}}^{2}\) in Eq. (1), where \({\mathfrak {S}}^{2}\) is the unit sphere manifold. As a consequence, the particle orientation will be described by a microscopic director \({\varvec{{n}}}\in {\mathfrak {S}}^{2}\), which is defined as a unit vector pointing in the temporary direction of a planar or needle-shaped rigid particle. However, in the present study, the microscopic director \({\varvec{{n}}}\) is used to describe the orientation of assemblies of molecules within a crystalline microdomain (see Fig. 1). As demonstrated experimentally by Bourrat et al. [24], these microdomains are mesophase regions with assemblies of disk-like molecules that exhibit anisotropic behavior and a long range orientation order. The assemblies of molecules gather to form microdomains, where they are aligned basically in the same direction even in the molten state. Hence, the microdomains form the textural units of domains that define the macroscopic texture.

Fig. 1
figure 1

Schematic representation of molecular assemblies with orientational order in a pitch precursor fiber. A primitive view of the mesoscopic coarse-grained model. The orientation of the microdomain is assumed to agree with the average particle orientation, and the mesoscopic potential is assumed to agree with the mean-field potential acting on the individual molecule. The macroscopic orientation in the fiber is represented by the orientations of the mesophase regions (set of microdomains). The small arrows represent the orientations of molecules, and larger blue arrows represent the orientations of the microdomains. Figure adapted from [25]

Full size image

With these assumptions on the mesophase pitch and its microdomains, one defines the mesoscopic space as

$$\begin{aligned} {\mathcal {M}}^{6}={\mathfrak {S}}^{2}\times {\mathbb {R}}^{3}\times {\mathbb {R}}^{1}. \end{aligned}$$
(5)

In the mesoscopic theory of liquid crystals [16,17,18, 21,22,23, 26, 27], this space is named nematic, and it is used to study viscosity coefficients and other properties of liquid crystals. Although the present work is based on this theory, the continuum mesoscopic approach employed here is different. In the present investigation, the mesophase pitch is regarded as a mixture formed by k chemical constituents. Strictly speaking, the pitch will be treated as a mixture constituted by k chemical species, so that the main fluid (chemical species with highest concentration) is much more dominant than all other chemical species. Under these conditions, the orientational dynamics of the mixture can be described as that of the main fluid. It is worth mentioning that such an assumption is experimentally reasonable for the mesophase pitch with 100% anisotropy, since it presents very low concentrations of impurities.

The orientational balance equations for the mesophase pitch are similar to those used in the theory of liquid crystals [16,17,18, 21,22,23, 26, 27] and in the thermodynamic investigation of mixtures with orientation [15]. These balance equations on the mesoscopic level are obtained from the general expression of balance equations [16] for an arbitrary extensive quantity:

$$\begin{aligned} \frac{\partial }{\partial t}\psi +\nabla _{{\varvec{{x}}}}\cdot [\psi {\varvec{{v}}}+\Gamma _{\psi }]+\nabla _{{\varvec{{n}}}}\cdot [\psi {\varvec{{w}}}+\Gamma ^{*}_{\psi }]={\mathfrak {s}}_{\psi }+{\mathfrak {p}}_{\psi }, \end{aligned}$$
(6)

where \(\psi \) is the mesoscopic density of the corresponding extensive quantity, \({\varvec{{v}}}\) is the mesoscopic material velocity, \({\varvec{{w}}}\) the orientation change velocity of the microscopic director \({\varvec{{n}}}\), \(\Gamma _{\psi }\) the non-convective flux of \(\psi \) on the space \({\mathbb {R}}^{3}\), \(\Gamma ^{*}_{\psi }\) the flow of \(\psi \) on the unit sphere manifold \({\mathfrak {S}}^{2}\), \({\mathfrak {s}}_{\psi }\) the supply, and \({\mathfrak {p}}_{\psi }\) is the production. Cartesian components of the gradient operators in \({\mathbb {R}}^{3}\) and \({\mathfrak {S}}^{2}\) are respectively

$$\begin{aligned} \nabla _{{\varvec{{x}}}}=\frac{\partial }{\partial x_{i}}\qquad and \qquad \nabla _{{\varvec{{n}}}}=\frac{\partial }{\partial n_{i}}-n_{i}n_{j}\frac{\partial }{\partial n_{j}}, \end{aligned}$$
(7)

where the last one is called orientational gradient operator. The orientational balances for the mesophase pitch (microdomains and ambient mesophase) are shown in Table 1. Their mathematical deduction is not displayed here, but it is similar to those deduced in [15] and [28].

Table 1 Mesoscopic field quantities that arise in the general balance equation (6) for mass \(\rho \), linear momentum \(\rho {\varvec{{v}}}\), spin \({\varvec{{s}}}\), energy \(\varepsilon \) and entropy \({\mathfrak {z}}\) of microdomain \(\alpha \) and the mesophase mixture
Full size table

3 Orientation distribution function for the microdomains

The MDF is the statistical quantity that distinguishes the mesoscopic theory from a macroscopic one. For determining the MDF, one needs a differential equation. This differential equation is often assumed in an ad-hoc form characterizing the model under consideration. Here, in the framework of the mesoscopic theory, a differential equation for the MDF is derived from the balance of mass.

Starting from Eq. (4) and the mesoscopic mass balance (see Table 1 and reference [15]), an evolution equation for the MDF of microdomain \(\alpha \) is obtained,

$$\begin{aligned} \frac{\partial }{\partial t}f_{\alpha }+\nabla _{{\varvec{{x}}}}\cdot (f_{\alpha }{\varvec{{v}}}_{\alpha })+\nabla _{{\varvec{{n}}}}\cdot (f_{\alpha }{\varvec{{w}}}_{\alpha })+f_{\alpha }\left[ \frac{\partial }{\partial t}+{\varvec{{v}}}_{\alpha }\cdot \nabla _{{\varvec{{x}}}}\right] \ln \rho _{\alpha }({\varvec{{x}}},t)=\frac{\chi _{\alpha }}{\rho _{\alpha }}. \end{aligned}$$
(8)

Here, \(\chi _{\alpha }\) is the mass production of microdomain \(\alpha \) per unit volume and time. As microdomains are formed by particles with orientation, the MDF is called an orientation distribution function (ODF). From the mathematical point of view, the Eq. (8) is an integro-differential equation due to the appearance of the macroscopic mass density \(\rho ({\varvec{{x}}},t)\), which is an integral over the mesoscopic mass density. From a physical point of view, it is a mean field equation because the ODF is also determined by the macroscopic mass density, i.e. by microdomains of any orientation. According to Eq. (4), the ODF describes the local alignment of the microdomains pertaining to a mesophase region. If there is no local alignment, that means, the microdomains are isotropically distributed, then the ODF becomes independent of n. As a consequence, the normalization condition results in \(f({\varvec{{x}}},t) = 1/(4\pi )\) and \(f({\varvec{{x}}},t) = 1/(\pi )\) for three-dimensional and two-dimensional microdomains, respectively. In the microdomain, the particle orientation has a rotational symmetry. A director n can be introduced as a unit vector pointing in the direction of the symmetry axis. In this approach, such director will be interpreted as the average orientation of the molecules within the microdomain.

Eq. (8) can be simplified by introducing the orientational material derivative [15],

$$\begin{aligned} \frac{df_{\alpha }}{dt}=\frac{\partial f_{\alpha }}{\partial t} +{\varvec{{v}}}_{\alpha }\cdot \nabla _{{\varvec{{x}}}}(f_{\alpha }) +{\varvec{{w}}}_{\alpha }\cdot \nabla _{{\varvec{{n}}}}(f_{\alpha }), \end{aligned}$$
(9)

into the mass balance equation:

$$\begin{aligned} \frac{df_{\alpha }}{dt}+f_{\alpha }\nabla _{{\varvec{{x}}}}\cdot ({\varvec{{v}}}_{\alpha })+ f_{\alpha }\nabla _{{\varvec{{n}}}}\cdot ({\varvec{{w}}}_{\alpha })=\frac{\chi _{\alpha }}{\rho _{\alpha }}-\frac{d}{dt}\mathrm {ln}(\rho _{\alpha }). \end{aligned}$$
(10)

Defining a mesoscopic operator for the microdomain \(\alpha \) by

$$\begin{aligned} \Omega =\frac{d}{dt}+\nabla _{{\varvec{{x}}}}\cdot ({\varvec{{v}}}_{\alpha })+ \nabla _{{\varvec{{n}}}}\cdot ({\varvec{{w}}}_{\alpha }), \end{aligned}$$
(11)

one can rewrite Eq. (10) in the compact form

$$\begin{aligned} \frac{d}{dt}\mathrm {ln}(\rho _{\alpha })=\frac{\chi _{\alpha }}{\rho _{\alpha }}-\Omega [f]\quad \text {or}\quad \frac{d\rho _{\alpha }}{dt}=\chi _{\alpha }-\rho _{\alpha }\Omega [f], \end{aligned}$$
(12)

where the values of \(\Omega [f]\) depend on the flow field. If the mesophase mixture is not formed by particles with orientation, \(\Omega [f]=0\), then the temporal variation of the microdomain density only depends on the production \(\chi _{\alpha }\). Note that, in Eq. (12), the temporal variation of the density is given by the difference between the microdomain mass production and the microdomain density multiplied by a mesoscopic operator \(\Omega [f]\) that depends on the ODF. Eq. (12) will be analyzed in detail in Sect. 5.

It is important to highlight that the operator \(\Omega [f]\) can be modified and extended to other systems. For example, one can extend the definition of \(\Omega [f]\) by using the molecular theory of Doi [30] in order to characterize the molecular dynamics and rheological properties of concentrated solutions of rod-like polymers in isotropic and liquid crystalline phases. Thus, depending on the model considered, the above physical interpretation of this mesoscopic operator can be drastically altered.

4 Microdomains formation thermodynamics

In order to include thermodynamic results in Eq. (12) in a form as general as possible, the recently developed generalized Gibbs’ theory [31,32,33] is employed to obtain an expression for the microdomain mass production, \(\chi _{\alpha }\).

4.1 Steady-state nucleation rate

The steady-state nucleation rate \(\zeta \) per unit volume and time is described by

$$\begin{aligned} \zeta = {\mathfrak {h}}\sqrt{\frac{{\mathfrak {d}}}{k_{B}T}}\frac{D}{a_{0}}\mathrm {exp}\left( \frac{-W_{c}}{k_{B}T}\right) , \end{aligned}$$
(13)

where \(W_{c}\) is the critical cluster formation work, \({\mathfrak {d}}\) is the specific interfacial energy, \(k_{B}\) the Boltzmann constant, T the absolute temperature, D the effective diffusion coefficient determining the processes of aggregation of ambient phase particles to crystal clusters. \(a_{0}\) is an average characteristic size parameter that is determined by the particle number density, \({\mathfrak {h}}\) of the ambient phase structural units. The value of \(a_{0}\) is commonly estimated via the crystalline molar volume, \(\upsilon _{m}\), and the Avogadro constant, \(N_{A}\), as

$$\begin{aligned} a_{0}\approx \left( \frac{\upsilon _{m}}{N_{A}}\right) ^{1/3}. \end{aligned}$$
(14)

For homogeneous nucleation \({\mathfrak {h}}\) is given by

$$\begin{aligned} {\mathfrak {h}}= \frac{1}{a_{0}^{3}}\approx \frac{n_{total}}{\upsilon }, \end{aligned}$$
(15)

where \(n_{total}\) is the total number of atoms (molecules or structural units) per cluster volume \(\upsilon \).

Assuming that the nucleation rate of microdomains is described by Eq. (13), minor conceptual adjustments for its variables have to be made. The physical meaning of the variables, \(k_{B}\), T, \(a_{0}\), \({\mathfrak {d}}\) in Eq. (13) is not changed, but for \(W_{c}\), D, \({\mathfrak {h}}\), it is slightly adapted. Thus, \(W_{c}\) is interpreted as the critical work required to form a microdomain \(\alpha \), D is the diffusion coefficient governing the aggregation processes of the mesophase mixture particles to the microdomain, and \({\mathfrak {h}}\) is the particle number density in the microdomain. The microdomain mass density \(\rho _{\alpha }\) can be defined in terms of Eq. (15) by the relation

$$\begin{aligned} {\mathfrak {h}}= \rho _{\alpha }\frac{N_{A}}{\bar{M}_{k}}, \end{aligned}$$
(16)

where \(\bar{M}_{k}\) is the average molar mass of the mesophase mixture of k components. The exact determination of the molecular mass of a mesophase pitch has been considered a difficult task, because no solvent dissolves every molecule contained in the mesophase pitch. The complexity of the molecular composition in the mesophase pitch derives from residues, which carry no monomeric units, and very high aromaticity, is the reason for such difficulty [11]. Due to this fact, one may employ \(\bar{M}_{k}\) for the microdomain, although this assumption is not a guarantee of accuracy. The most correct procedure would be to use a molecular weight distribution.

4.2 Specific interfacial energy \({\mathfrak {d}}\)

Employing the generalized Gibbs approach, the specific interfacial energy is derived via the formulation of an extension of the Stefan–Skapski–Turnbull relation [33], either in the form

$$\begin{aligned}&{\mathfrak {d}}= \gamma \frac{q}{\upsilon ^{2/3}}\nonumber \\&\quad =\frac{\gamma }{\upsilon ^{2/3}}\left\{ T_{\beta }s_{\beta }^{(particle)}-T_{\alpha }s_{\alpha }^{(particle)}+\sum _{i=1}^{k}\left[ \mu _{i\beta }(p_{\beta },T_{\beta },{x_{i\beta }})-\mu _{i\alpha }(p_{\alpha },T_{\alpha },{x_{i\alpha }})\right] x_{i\alpha }\right\} ,\nonumber \\ \end{aligned}$$
(17)

or by entropies and chemical potentials per mole, as

$$\begin{aligned}&{\mathfrak {d}}=\gamma \frac{q_{m}}{N_{A}^{1/3}\upsilon _{m}^{2/3}}\nonumber \\&\quad =\frac{\gamma }{N_{A}^{1/3}\upsilon _{m}^{2/3}}\left\{ T_{\beta }s_{\beta }^{(mol)}-T_{\alpha }s_{\alpha }^{(mol)}+\sum _{i=1}^{k}\left[ \mu _{i\beta }^{(mol)}(p_{\beta },T_{\beta },{x_{i\beta }})-\mu _{i\alpha }^{(mol)}(p_{\alpha },T_{\alpha },{x_{i\alpha }})\right] x_{i\alpha }\right\} .\nonumber \\ \end{aligned}$$
(18)

Assuming that the above relations are valid for the mesophase mixture, the variables in Eqs. (17) and (18) can be interpreted as follows:

  • \(\gamma \) is a proportionality parameter correlating the specific interfacial energy in melt crystallization with the enthalpy of melting.

  • The latent heat of melting per particle \(q=q_{m}/N_{A}\) (\(q_{m}\) is the molar heat of melting) is related to the average change of melting enthalpy by the relation

    $$\begin{aligned}&q=\frac{H_{\beta }-H_{\alpha }}{n_{\alpha }}\nonumber \\&\quad = \left\{ T_{\beta }s_{\beta }^{(particle)}-T_{\alpha }s_{\alpha }^{(particle)}+\sum _{i=1}^{k}\left[ \mu _{i\beta }(p_{\beta },T_{\beta },{x_{i\beta }})-\mu _{i\alpha }(p_{\alpha },T_{\alpha },{x_{i\alpha }})\right] x_{i\alpha }\right\} , \end{aligned}$$
    (19)

    where \(n_{\alpha }\) is the total number of particles in the microdomain \(\alpha \) and \(x_{i\alpha }=n_{i\alpha }/n_{\alpha }\) the particle number fraction of the ith component in the microdomain undergoing a phase transition to the ambient mesophase \(\beta \). The entropy s, temperature T, pressure p and chemical potential of the ith component \(\mu _{i}\) are defined in both \(\alpha \) and \(\beta \) phases.

  • \(\upsilon \) is related to the molar volume \(\upsilon _{m}\) by \(\upsilon =\upsilon _{m}/N_{A}\) and corresponds to the volume occupied by one structural unit of the mesophase mixture. According to Eq.(14), \(\upsilon \) can be defined in terms of the average characteristic size parameter \(a_{0}\),

    $$\begin{aligned} \upsilon =a_{0}^{3}. \end{aligned}$$
    (20)

    Consequently, one has

    $$\begin{aligned} {\mathfrak {h}}\approx \frac{1}{a_{0}^{3}}=\frac{1}{\upsilon }. \end{aligned}$$
    (21)

Eqs. (17) and (18) describe the dependence on the state parameters of the specific interfacial energy of both the crystalline microdomains and the ambient mesophase for the general case, that is, when both phases are not in thermodynamic equilibrium. However, if the microdomains are in equilibrium with the ambient mesophase, then the following general relations are employed,

$$\begin{aligned}&(T_{\alpha }-T_{\beta })s_{\alpha }+(p_{\beta }-p_{\alpha })+\frac{2{\mathfrak {d}}}{R_{c}}+\sum _{i=1}^{k}\varphi _{i\alpha }(\mu _{i\alpha }-\mu _{i\beta })=0, \end{aligned}$$
(22)
$$\begin{aligned}&\mu _{i\beta }-\mu _{i\alpha }=\frac{3}{R_{c}}\left( \frac{\partial {\mathfrak {d}}}{\partial \varphi _{i\alpha }}\right) _{\varphi _{i\beta },s_{\beta }}\qquad \text {for}\qquad i=1,2,...,k, \end{aligned}$$
(23)
$$\begin{aligned}&\text {and\qquad }T_{\beta }-T_{\alpha }=\frac{3}{R_{c}}\left( \frac{\partial {\mathfrak {d}}}{\partial s_{\alpha }}\right) _{\varphi _{i\beta },s_{\beta }}, \end{aligned}$$
(24)

where \(R_{c}\) is the microdomain critical radius and \(\varphi _{i\alpha }\) is a complete set of intensive variables specifying the bulk state of the microdomain phase. Note that \(s_{\alpha }\) is now interpreted as volume density of the entropy. Then, Eq. (17) can be reformulated in terms of \(\varphi _{i\alpha }\) as

$$\begin{aligned}&{\mathfrak {d}}= \gamma \frac{q}{\upsilon ^{2/3}}\nonumber \\&\quad =\frac{\gamma }{\upsilon ^{2/3}}\left\{ T_{\beta }s_{\beta }-T_{\alpha }s_ {\alpha }+\sum _{i=1}^{k}\varphi _{i\alpha }\left[ \mu _{i\beta }(p_{\beta },T_{\beta },{x_{i\beta }})-\mu _{i\alpha }(p_{\alpha },T_{\alpha },{x_{i\alpha }})\right] \right\} .\qquad \end{aligned}$$
(25)

Substituting the equilibrium conditions Eqs. (22,23,24) into the above equation, one obtains

$$\begin{aligned} {\mathfrak {d}}=\frac{\gamma }{\upsilon ^{2/3}}\left\{ T_{\beta }(s_{\beta }-s_{\alpha })+\frac{3}{R_{c}}\left[ s_{\alpha }\left( \frac{\partial {\mathfrak {d}}}{\partial s_{\alpha }}\right) _{\varphi _{i\beta },s_{\beta }}+\sum _{i=1}^{k}\varphi _{i\alpha }\left( \frac{\partial {\mathfrak {d}}}{\partial \varphi _{i\alpha }}\right) _{\varphi _{i\beta },s_{\beta }}\right] \right\} .\qquad \end{aligned}$$
(26)

It is important to emphasize that these results are succinctly presented and discussed in references [31,32,33] for general application involving crystal and melting phases. The present study just assumes its validity for an analogous system, namely a mesophase mixture with crystalline microdomain formation. In fact, as shown in [15], the usual thermodynamic relations are maintained for a system of particles with orientation. Therefore, these considerations may be applied to the mesoscopic quantities as well.

4.3 Critical work and thermodynamic driving force

The critical work \(W_{c}\) in Eq. (13) can be interpreted as the change of the Gibbs free energy connected with the formation of microdomains. Following [32], one can write the critical microdomain formation work for spherical shape nuclei as

$$\begin{aligned} W_{c}=\frac{15\pi }{3}\frac{{\mathfrak {d}}(R_{c})^3}{\left( \Delta G_{c}(p_{\alpha }, T_{\alpha };p_{\beta }, T_{\beta })\right) ^{2}}\quad \text {and}\quad R_{c}=\frac{2{\mathfrak {d}}}{\left( \Delta G_{c}(p_{\alpha }, T_{\alpha };p_{\beta }, T_{\beta })\right) },\nonumber \\ \end{aligned}$$
(27)

where \(R_{c}\) is the critical microdomain size and \(\Delta G_{c}\) the thermodynamic driving force of nucleation and microdomain growth. Although the assumption of microdomain nuclei of spherical shape is to simplify our treatment, experimental evidence [11] indicates that, in fact, they are spherical approximately.

The thermodynamic driving force for microdomain formation can be given by two approximations. First, \(\Delta G_{c}\) is given by

$$\begin{aligned} \Delta G_{c}=\sum _{i=1}^{k}{\mathfrak {h}}_{i\alpha }(\mu _{i\beta }-\mu _{i\alpha })=\frac{N_{A}}{\bar{M_{k}}}\sum _{i=1}^{k}\rho _{i\alpha }(\mu _{i\beta }-\mu _{i\alpha }), \end{aligned}$$
(28)

where Eq. (16) was used. It is possible to obtain a second approximation by performing a truncated Taylor series expansion with respect to temperature and pressure differences, as follows

$$\begin{aligned} G_{c}=\frac{N_{A}}{\bar{M_{k}}}\sum _{i=1}^{k}\rho _{i\alpha }(\mu _{i\beta }-\mu _{i\alpha })+\frac{\kappa _{\alpha }}{2}(p_{\alpha }-p_{\beta })^{2}+\frac{C_{P\alpha }}{2}\frac{(T_{\alpha }-T_{\beta })^2}{T_{\beta }} \nonumber \\ -\vartheta (p_{\alpha }-p_{\beta })(T_{\alpha }-T_{\beta }). \end{aligned}$$
(29)

Here, \(\kappa _{\alpha }\), \(C_{P\alpha }\), and \(\vartheta \) are the compressibility, specific heat, and thermal expansion coefficient of the microdomain phase \(\alpha \). Note that, for small values of pressure and temperature differences, Eq. (29) is simplified to Eq. (28).

4.4 Production of microdomain \(\alpha \) in the ambient mesophase

Since the variables in Eq. (13) are well defined, one assumes that the production of microdomain \(\alpha \) is directly related to the steady-state nucleation rate J by

$$\begin{aligned} \chi _{\alpha }=\zeta \frac{\bar{M_{k}}}{N_{A}}=\rho _{\alpha }\sqrt{\frac{{\mathfrak {d}}}{k_{B}T}}\frac{D}{a_{0}}\mathrm {exp}\left( \frac{-W_{c}}{k_{B}T}\right) , \end{aligned}$$
(30)

where Eq. (16) has been used. The above equation can be generalized by considering the nucleation-time lag,

$$\begin{aligned} \tau =\frac{16}{3}\frac{k_{B}T{\mathfrak {d}}}{\Delta G_{c}^{2}a_{0}^{2}D}, \end{aligned}$$
(31)

which is the formation time of a critical size microdomain distribution from embryo size microdomains in a stationary state. Substituting Eq. (31) in Eq. (30) and using Eqs. (16) and (21), one obtains

$$\begin{aligned} \chi _{\alpha }=\rho _{\alpha }^{2}\frac{N_{A}}{\bar{M_{k}}}\frac{{\mathfrak {d}}^{3/2}\sqrt{k_{B}T}}{\Delta G_{c}^{2}\tau }\mathrm {exp}\left( \frac{-W_{c}}{k_{B}T}\right) . \end{aligned}$$
(32)

The above equation can be simplified by using the approximation of Hoffman [34, 35] for the thermodynamic driving force

$$\begin{aligned} \Delta G_{c}=\Delta H_{m}\left( 1-\frac{T}{T_{m}}\right) =\frac{\Delta H_{m}\Delta T}{T_{m}}, \end{aligned}$$
(33)

where, \(\Delta H_{m}\) (see Eq. 19) is the melting enthalpy per microdomain, \(T_{m}\) the melting temperature of the stable microdomain and \(\Delta T\) the degree of supercooling. In this approach, the microdomains behave as crystalline regions inside the mesophase pitch, hence, \(\Delta H_{m}\) is physically interpreted as an enthalpy of phase transition. Note that substituting Eqs. (31) and (33) in (13), one obtains the nucleation rate per microdomain,

$$\begin{aligned} \zeta _{\alpha }=\frac{\zeta }{n_{total}}=\frac{16}{3}\rho _{\alpha }^{2}\left( \frac{N_{A}}{\bar{M_{k}}}\right) ^{2}\frac{{\mathfrak {d}}^{3/2}\sqrt{k_{B}T}(T_{m})^{2}}{(\Delta H_{m})^{2}(\Delta T)^{2}\tau }\mathrm {exp}\left( \frac{-W_{c}}{k_{B}T}\right) . \end{aligned}$$
(34)

Thus, by employing the definitions (26), (28) or (29) and (32) in Eq. (12), the variation of the density with changes of mechanical and thermodynamic state variables in the microdomain becomes evident. One of the greatest difficulties in using Eqs. (32) or (34) is the determination of the variables \({\mathfrak {d}}\), \(\tau \), \(\Delta G_{c}\) and \(W_{c}\), which are quite difficult to obtain experimentally. In fact, at the moment, there are no experimental data for these variables, regardless of the mesophase pitch considered.

5 Estimates for the microdomains behavior

In this section, Eq. (12) is analyzed to qualitatively describe the behavior of the microdomains. Naphthalene-derived mesophase pitch (NP) is chosen as test material in this part, since it is one of the main pitches used for the production of high-performance mesophase pitch-based carbon fibers. The physical properties of NP are shown in Table 2.

Table 2 Physical properties of Naphthalene-derived mesophase pitch
Full size table

According to Eq. (34), a nucleation barrier resulting from the interfacial energy between the ordered phase and the melt must be overcome to crystallize that phase from the amorphous melt. Such nucleation barrier is represented by the critical work \(W_{c}\) required to produce a critical-sized microdomain from the melt. Thus, by solving Eq. (34), it is possible to determine the critical work \(W_{c}\) as a function of temperature by using experimental data for the interfacial energy \({\mathfrak {d}}\), time-lag \(\tau \) and by choosing appropriate estimates of the thermodynamic driving force \(\Delta G_{c}\). Unfortunately, there are no experimental data for \(\zeta _{\alpha }\) and \(\chi _{\alpha }\). Thus, in this work their values are roughly estimated by means of the NP bulk density. In addition, based on heuristic arguments and Eq. (34), one assumes that the maximum nucleation rate for NP occurs at some temperature close to the glass-temperature \(T_{g}\), while its minimum rate can be observed at the ambient temperature and softening-temperature \(T_{s}\).

In view of the above assumptions, a linear relation between the temporal variation of the density and the mesosocopic operator is estimated from Eq. (12). For computations, a microdomain critical density is considered on the right hand side of Eq. (12), which is 15% greater than the bulk density, since according to experimental observations the crystalline regions are generally more densely packed than amorphous ones (up to 15% depending on the material). Figure (2) shows such linear relation for NP. The maximum value of \(\chi _{\alpha }\) is assumed to be equal to the bulk density per second (see Table 2). Other assumed values for \(\chi _{\alpha }\) were also considered in the calculations.

In general, it is observed that, for small values of \(\chi _{\alpha }\), by increasing the value of \(\Omega [f]\) the temporal variation of the density begins to show a negative value at some low value of \(\Omega [f]\). But, for high values of \(\chi _{\alpha }\), the temporal variation of the density begins to display negative values at \(\Omega [f]\) around 1 only. For instance, for NP with \(\chi _{\alpha }=0.65 \,{\text {g s}}^{-1} {\text {cm}}^{-3}\), the variation of the density is negative from \(\Omega [f]=0.87\) on. It is noteworthy that the linearity and parallelism of the straight lines are always observed because, for all arbitrarily fixed production values, the critical density of the microdomains is kept constant at its value for \(T_{g}\) in the calculation, even though such an assumption may not be true in experiments for isothermal processes at \(T_{g}\). This observation draw attention to the need of experimental data for \(\rho _{\alpha }\) and \(\chi _{\alpha }\), in order to verify the validity of Eq. (12).

Fig. 2
figure 2

Estimates for the temporal variation of the density of Naphthalene-derived mesophase pitch at \(T_{g}\). The points are estimated from Eq. (12) for \(\chi _{\alpha }\) values from 0.1 to \(0.65\,{\text {g s}}^{-1} {\text {cm}}^{-3}\). The critical density of the microdomain is assumed \(\rho ^{c}_{\alpha }=0.715\, {\text {g cm}}^{-3}\) (a value 15% greater than the bulk density)

Full size image

Although Fig. (2) is constructed on the basis of heuristic assumptions due to the lack of experimental data, very important qualitative conclusions can be drawn from it, namely:

  • The mesoscopic operator \(\Omega [f]\) can be physically interpreted as the rate of change of the degree of crystallinity. Generally, the degree of crystallinity for a polymer mixture can be obtained experimentally by assuming a mixture of perfect crystalline and totally disordered regions. Most of these methods include density measurement, differential scanning calorimetry (DSC), X-ray diffraction (XRD), infrared spectroscopy and nuclear magnetic resonance (NMR). The measured value depends on the method used, which would then be quoted together with the degree of crystallinity [38]. The methods used to determine the degree of crystallinity can be incorporated as a function of time to measure the kinetics of crystallization by means of the Hoffman nucleation theory [34, 35]. In fact, in Eqs. (12),  (30) and (34), the Hoffman theory is already included. Thus, if experimental data for \(\zeta _{\alpha }\) or \(\chi _{\alpha }\) exist, then \(d\rho _{\alpha }/dt\) could be estimated together with the microdomain density and the degree of crystallinity via Eq. (12). Therefore, one can conclude that Eq. (12), deduced in the present work may become a possible route to experimentally estimate the physical properties of the microdomains during the spinning step of carbon fiber production.

  • The temporal variation of the density can be approximately determined by \(\chi _{\alpha }\) or \(\zeta _{\alpha }\) when \(\Omega [f]\approx 0\), but for \(\Omega [f]>0\) its value is influenced by the flow type and orientations of the microdomains. This occurs because the distribution function f is significantly affected by the shear rate of the flow. For example, by using two-dimensional solutions of the distribution function for simple shear and planar elongational flows, it is observed that high values for \(\Omega [f]\) are related to smaller orientation angles for the microdomains in the high shear and elongation rate regime [39]. Thus, when the values of \(\Omega [f]\) increase, the orientation angles of the microdomains in relation to the fiber axis decrease. High deformation rates correspond to high values of \(\Omega [f]\).

  • At \(d\rho _{\alpha }/dt=0\) the microdomains transform into crystalline microregions and, consequently, \(\rho _{\alpha }\) at the right hand side of Eq. (12) may then be experimentally identified as the critical density \(\rho ^{c}_{\alpha }\), at which the microdomains convert to the crystalline phase by supercooling. Hence, the density \(\rho ^{c}_{\alpha }\) of a solid microdomain is given by the quite simple relation

    $$\begin{aligned} \rho ^{c}_{\alpha }=\frac{\chi _{\alpha }}{\Omega [f]}\qquad \text {for}\qquad {\left\{ \begin{array}{ll} 0<\Omega [f]\le 1 \\ \chi _{\alpha }\le \rho ^{c}_{\alpha }, \end{array}\right. } \end{aligned}$$
    (35)

    where \(\Omega [f]\) can not be null because the microdomain has an orientational order. Note that the critical density is not necessarily defined for a perfect crystalline state of microdomains (\(\Omega [f]=1\)), because fully crystalline polymers do not exist. Hence, the density of perfectly crystalline polymers cannot be measured directly, but using Eq. (35) it can be estimated. It is important to emphasize that by means of modern X-ray analysis it may be possible to determine the crystalline system of the microdomains, the dimensions of the unit cell as well as the number of the constitutional base units in the unit cell. From these data the real crystalline density can be exactly calculated.

  • In regions where the microdomains are highly aligned with respect to the fiber axis, the operator \(\Omega [f]\) has values close to 1, so that \(d\rho _{\alpha }/dt<0\), whereas in regions presenting \(\Omega [f]<<1\), generally it is observed \(d\rho _{\alpha }/dt>0\). The microdomain formation process always begins at \(d\rho _{\alpha }/dt>0\), but when \(d\rho _{\alpha }/dt<0\) two phenomena may occur simultaneously: microdomain coalescence forming larger domains and microdomain degradation. Although this statement is only an assumption to explain the negative temporal variation of the density when \(\Omega [f]\) increases, several experimental studies [3, 5, 6, 11, 13, 24, 40] show that, for high \(\Omega [f]\) values, the reduction of viscosity in the mesophase pitch could be explained in terms of deformation or degradation of the microdomains. Thus, if this explanation is proved to be true for the pitch, then Eq. (12) could be used to quantitatively describe the viscosity-shear rate correlation in terms of the temporal variation of the density of microdomains.

  • According to Eq. (12), the temporal variation of the density is determined by the product \(\rho _{\alpha }\Omega [f]\) when microdomains are not being formed. Probably, during the pitch spinning process the microdomain formation is ceased or very few of them are still produced, hence \(d\rho _{\alpha }/dt\) values will only depend on the operator \(\Omega [f]\). In this case, the quasi-static Avrami approximation [41] can be used to describe the non-isothermal crystallization rate, since \(\Omega [f]\) is assumed to be the rate of change of the degree of crystallinity.

6 Conclusions

In this paper, a simple coarse-grained model is employed to describe the behavior of microdomains in the mesophase pitch. The model can be expanded for other microstructured systems with orientation, as grains, clusters, particles, macromolecules, etc.

Starting from an orientation distribution function for the microdomains and the balance of mass of microdomains, a simple equation (see Eq. 12) for the temporal variation of the microdomain density is deduced. According to this equation, the time rate of the microdomain density is determined by three variables: the microdomain mass production, critical density and a mesoscopic operator (acting on the orientation distribution function). As assumed in the present work, the mass production can be determined applying the crystallization kinetics theory via the steady-state nucleation rate \(\zeta _{\alpha }\) for the microdomains. Unfortunately, there are no experimental data for \(\zeta _{\alpha }\), so that the validation of Eq. (12) becomes quite difficult. Experimentally, it is possible to estimate the critical density of microdomains, nevertheless there are no experimental studies for this determination in the mesophase pitch. Thus, Eq. (12) deduced in the present work provides a possible route for future estimates of microdomain density changes over time.

The deformation and orientation states of the microdomains are determined by the mesoscopic operator \(\Omega [f]\). If a solution for the orientation distribution function is found for a given type of flow, then the values of \(\Omega [f]\) can be precisely calculated. The physical meaning of \(\Omega [f]\) depends on the model equation for the orientation distribution function. In the present work, the mesoscopic mass balance equation is used, but the underlying equation may be different, however of the type of a balance equation. Note that the limit values settled down for \(\Omega [f]\) (between 0 to 1) must be adopted to the model equation.

In order to illustrate possible applications of the mesoscopic approach presented here, Eq. (12) is used to estimate the temporal variation of the microdomain density in Naphthalene-derived mesophase pitch at \(T_{g}\). In general, it is observed that, as the mass production of microdomains decreases, negative values of \(d\rho _{\alpha }/dt\) begin to occur at lower values of \(\Omega [f]\). Although these estimates are based on assumptions for the mesophase pitch, it can be explained by the experimental observation that at deformation rates high enough (high values of \(\Omega [f]\)), deformation or degradation of the microdomains occurs. The negative values of \(d\rho _{\alpha }/dt\) for NP are assigned to both microdomain degradation and coalescence phenomena. This should be investigated in detail through simulation or rheological experimental work in order to confirm this assumption. Moreover, further implementation of Eq. (12) together with Eqs. (30) and (34), for interpretation of experimental observations, should be performed.