Skip to main content
SpringerLink
Log in

Birkhoff Program for Geodesic Flows of Surfaces and Applications: Homoclinics

  • Published:
Journal of Dynamics and Differential Equations Aims and scope Submit manuscript

Abstract

We show that a Kupka–Smale riemannian metric on a closed surface contains a finite primary set of closed geodesics, i.e. they intersect any other geodesic and divide the surface into simply connected regions. From them we obtain a finite set of disjoint surfaces of section of genera 0 or 1, which intersect any orbit of the geodesic flow. As an application we obtain that the geodesic flow of a Kupka–Smale riemannian metric on a closed surface has homoclinic orbits for all branches of all of its hyperbolic closed geodesics.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22

Similar content being viewed by others

Data Availability Statement

Not applicable.

Notes

  1. After [12] was published in ArXiv, in [7] appeared another proof that generic 3 dimensional Reeb flows have a Birkhoff section. But the statement of Theorem 1.1 in [7] does not apply to geodesic flows because, for example, the density of closed geodesics in the unit tangent bundle is still not known for generic riemannian metrics.

  2. There are arbitrarily small curves whose image under the Poincaré map have infinite length and large diameter.

  3. A heteroclinic connection is the case in which two components of \(W^s({\dot{\gamma }})\setminus {\dot{\gamma }}\) and \(W^u({\dot{\eta }})\setminus {\dot{\eta }}\) are equal.

  4. This classification is the same as radial and broken binding orbits for broken book decompositions.

  5. Rotating boundary orbits can be hyperbolic or elliptic.

  6. This condition says that the flow rotates more than the surface of section when it approaches its boundary orbit \(\gamma \).

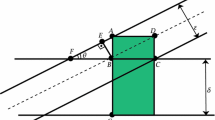
  7. In lemma 3.3 in [12] an argument of Fried is used to show that, since by Proposition 3.8 an orbit \(\kappa \in {K_{fix}}\) has homoclinics in all its branches, one can obtain a Birkhoff section \(\mathcal {B}\) which intersects \(\kappa \) in its interior.

  8. In fact the natural extension of f to a point \(x\in {K_{fix}}\) would be the whole circle of a first intersection of a component of \(W^u(\gamma )\setminus \gamma \), \(\gamma =\psi _{\mathbb R}(x)\), with S. See Fig. 4.

  9. For the boundedness of \(\tau _-\) we apply Proposition 3.12 to the inverse flow \(\psi _{-t}\).

  10. This does not happen on a contact flow but may happen for a reparametrization of the flow.

References

  1. Andersson, K.G.: Poincaré’s discovery of homoclinic points. Arch. Hist. Exact Sci. 48(2), 133–147 (1994)

    Article  MathSciNet  ADS  Google Scholar 

  2. Bangert, V.: On the existence of closed geodesics on two-spheres. Int. J. Math. 4(1), 1–10 (1993)

    Article  MathSciNet  Google Scholar 

  3. Birkhoff, G.D.: Dynamical systems with two degrees of freedom. Trans. Am. Math. Soc. 18(2), 199–300 (1917)

    Article  MathSciNet  Google Scholar 

  4. Birkhoff, G.D.: Dynamical Systems. American Mathematical Society, Providence (1966)

    Google Scholar 

  5. Cheng, C.-Q., Yan, J.: Existence of diffusion orbits in a priori unstable Hamiltonian systems. J. Differ. Geom. 67, 457–518 (2004)

    Article  MathSciNet  Google Scholar 

  6. Clarke, A.: Generic properties of geodesic flows on analytic hypersurfaces of Euclidean space. Discrete Contin. Dyn. Syst. 42(12), 5839–5868 (2022)

    Article  MathSciNet  Google Scholar 

  7. Colin, V., Dehornoy, P., Hryniewicz, U., Rechtman, A.: Generic properties of 3-dimensional reeb flows: Birkhoff sections and entropy, Preprint arXiv:2202.01506 (2022)

  8. Colin, V., Dehornoy, P., Rechtman, A.: On the existence of supporting broken book decompositions for contact forms in dimension 3. Invent. Math. 231(3), 1489–1539 (2023)

    Article  MathSciNet  ADS  Google Scholar 

  9. Contreras, G.: Geodesic flows with positive topological entropy, twist maps and hyperbolicity. Ann. Math. 172(2), 761–808 (2010)

    Article  MathSciNet  Google Scholar 

  10. Contreras, G., Knieper, G., Mazzucchelli, M., Schulz, B.H.: Surfaces of section for geodesic flows of closed surfaces, Preprint arXiv:2204.11977 (2022)

  11. Contreras, G., Mazzucchelli, M.: Proof of the \({C}^2\) stability conjecture for geodesic flows of closed surfaces, Preprint arXiv:2109.10704 (2021)

  12. Contreras, G., Mazzucchelli, M.: Existence of Birkhoff sections for Kupka–Smale Reeb flows of closed contact 3-manifolds. Geom. Funct. Anal. 32(5), 951–979 (2022)

    Article  MathSciNet  Google Scholar 

  13. Contreras-Barandiarán, G., Paternain, G.: Genericity of geodesic flows with positive topological entropy on \(S^2\). J. Differ. Geom. 61, 1–49 (2002)

    Article  Google Scholar 

  14. De Philippis, G., Marini, M., Mazzucchelli, M., Suhr, S.: Closed geodesics on reversible Finsler 2-spheres. J. Fixed Point Theory Appl. (2020) (to appear)

  15. Delshams, A., de la Llave, R., Seara, T.M.: A geometric approach to the existence of orbits with unbounded energy in generic periodic perturbations by a potential of generic geodesic flows of \({ T}^2\). Commun. Math. Phys. 209(2), 353–392 (2000)

    Article  ADS  Google Scholar 

  16. Farb, B., Margalit, D.: A Primer on Mapping Class Groups, Princeton Mathematical Series, vol. 49. Princeton University Press, Princeton (2012)

    Google Scholar 

  17. Fayad, B., Krikorian, R.: Herman’s last geometric theorem. Ann. Sci. Éc. Norm. Supér. (4) 42(2), 193–219 (2009)

    Article  MathSciNet  Google Scholar 

  18. Franks, J.: Geodesics on \(S^2\) and periodic points of annulus homeomorphisms. Invent. Math. 108(2), 403–418 (1992)

    Article  MathSciNet  ADS  Google Scholar 

  19. Franks, J.: Area preserving homeomorphisms of open surfaces of genus zero. N. Y. J. Math. 2, 1–19 (1996)

    MathSciNet  ADS  Google Scholar 

  20. Franks, J., Le Calvez, P.: Regions of instability for non-twist maps. Ergod. Theory Dyn. Syst. 23(1), 111–141 (2003)

    Article  MathSciNet  Google Scholar 

  21. Fried, D.: Transitive Anosov flows and pseudo-Anosov maps. Topology 22(3), 299–303 (1983)

    Article  MathSciNet  Google Scholar 

  22. Gage, M.E.: Curve shortening on surfaces. Ann. Sci. École Norm. Sup. (4) 23(2), 229–256 (1990)

    Article  MathSciNet  Google Scholar 

  23. Genecand, C.: Transversal homoclinic orbits near elliptic fixed points of area-preserving diffeomorphisms of the plane. In: Dynamics Reported: Expositions in Dynamical Systems (N.S.), vol. 2, pp. 1–30. Springer, Berlin (1993)

  24. Gidea, M., de la Llave, R., M-Seara, T.: A general mechanism of diffusion in Hamiltonian systems: qualitative results. Commun. Pure Appl. Math. 73(1), 150–209 (2020)

    Article  MathSciNet  Google Scholar 

  25. Grayson, M.A.: Shortening embedded curves. Ann. Math. (2) 129(1), 71–111 (1989)

    Article  MathSciNet  Google Scholar 

  26. Hofer, H., Wysocki, K., Zehnder, E.: Properties of pseudoholomorphic curves in symplectisations i: Asymptotics. Ann. Inst. Henri Poincaré Anal. Non Linéaire 13(3), 337–379 (1996), correction ibid. 15, No.4, 535–538 (1998)

  27. Hofer, H., Wysocki, K., Zehnder, E.: The dynamics on three-dimensional strictly convex energy surfaces. Ann. Math. (2) 148(1), 197–289 (1998)

    Article  MathSciNet  Google Scholar 

  28. Hofer, H., Wysocki, K., Zehnder, E.: Finite energy foliations of tight three-spheres and Hamiltonian dynamics. Ann. Math. (2) 157(1), 125–255 (2003)

    Article  MathSciNet  Google Scholar 

  29. Irie, K.: Dense existence of periodic Reeb orbits and ECH spectral invariants. J. Mod. Dyn. 9, 357–363 (2015)

    Article  MathSciNet  Google Scholar 

  30. Juvan, M., Malnič, A., Mohar, B.: Systems of curves on surfaces. J. Combin. Theory Ser. B 68(1), 7–22 (1996)

    Article  MathSciNet  Google Scholar 

  31. Knieper, G., Weiss, H.: \(C^\infty \) genericity of positive topological entropy for geodesic flows on \(S^2\). J. Differ. Geom. 62(1), 127–141 (2002)

    Article  Google Scholar 

  32. Le Calvez, P.: Étude Topologique des Applications Déviant la Verticale, Ensaios Matemáticos, 2. Sociedade Brasileira de Matemática, Rio de Janeiro (1990)

    Google Scholar 

  33. Le Calvez, P., Sambarino, M.: Homoclinic orbits for area preserving diffeomorphisms of surfaces. Ergod. Theory Dyn. Syst. 42(3), 1122–1165 (2022)

    Article  MathSciNet  Google Scholar 

  34. Mather, J.N.: Invariant subsets for area preserving homeomorphisms of surfaces. In: Mathematical Analysis and Applications, Part B, pp. 531–562. Academic Press, New York (1981)

  35. Mather, J.N.: Arnol’d diffusion I Announcement of results, Sovrem. Mat. Fundam. Napravl. 2 (2003), 116–130, translation in J. Math. Sci. (N.Y.) 124 (2004), no. 5, 5275-5289

  36. Moser, J.: Stable and Random Motions in Dynamical Systems. Princeton University Press, Princeton: With special emphasis on celestial mechanics, Hermann Weyl Lectures, the Institute for Advanced Study, p. 77. Princeton, NJ, Annals of Mathematics Studies, No (1973)

  37. Oliveira, F.: On the generic existence of homoclinic points. Ergod. Theory Dyn. Syst. 7(4), 567–595 (1987)

    Article  MathSciNet  Google Scholar 

  38. Oliveira, F., Contreras, G.: The Ideal Boundary and the Accumulation Lemma, Preprint arXiv:2205.14738 (2022)

  39. Oliveira, F., Contreras, G.: No elliptic points from fixed prime ends, Preprint arXiv:2205.14768 (2022)

  40. Pixton, D.: Planar homoclinic points. J. Differ. Equ. 44(3), 365–382 (1982)

    Article  MathSciNet  ADS  Google Scholar 

  41. Poincaré, H., Les méthodes nouvelles de la mécanique céleste. Tome I, Gauthier-Villars, Paris,: Solutions périodiques. Non-existence des intégrales uniformes, Solutions asymptotiques (1892)

  42. Poincaré, H., Les méthodes nouvelles de la mécanique céleste. Tome III, Gauthier-Villars, Paris,: Invariant intégraux. Solutions périodiques du deuxième genre, Solutions doublement asymptotiques (1899)

  43. Xia, Z., Zhang, P.: Homoclinic intersections for geodesic flows on convex spheres. In: Dynamical Systems, Ergodic Theory, and Probability: in Memory of Kolya Chernov, Contemporary Mathematics, vol. 698, pp. 221–238. American Mathematical Society, Providence (2017)

  44. Zehnder, E.: Homoclinic points near elliptic fixed points. Commun. Pure Appl. Math. 26, 131–182 (1973)

    Article  MathSciNet  Google Scholar 

Download references

Funding

Gonzalo Contreras is partially supported by CONACYT, Mexico, grant A1-S-10145.

Author information

Authors and Affiliations

  1. Centro de Investigación en Matemáticas, Guanajuato, Guanajuato, Mexico

    Gonzalo Contreras

  2. Universidade Federal de Minas Gerais, Belo Horizonte, Minas Gerais, Brazil

    Fernando Oliveira

Authors
  1. Gonzalo Contreras
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Fernando Oliveira
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

The contributions of GC happened more in the study of geodesic flows and those of FO in discrete dynamics.

Corresponding author

Correspondence to Fernando Oliveira.

Ethics declarations

Conflict of interest

Not applicable.

Ethics approval

Not applicable.

Consent to participate

Not applicable.

Consent for publication

Submission is consensual among all authors.

Code availability

Not applicable.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Gonzalo Contreras is partially supported by CONACYT, Mexico, grant A1-S-10145.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Contreras, G., Oliveira, F. Birkhoff Program for Geodesic Flows of Surfaces and Applications: Homoclinics. J Dyn Diff Equat (2024). https://doi.org/10.1007/s10884-024-10349-8

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10884-024-10349-8

Keywords

Mathematics Subject Classification

Search

Navigation