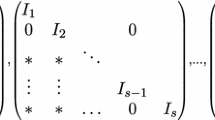Abstract
We investigate conjugacy classes of germs of hyperbolic 1-dimensional vector fields at the origin in low regularity. We show that the classical linearization theorem of Sternberg strongly fails in this setting by providing explicit uncountable families of mutually non-conjugate flows with the same multipliers, where conjugacy is considered in the bi-Lipschitz, \(C^1\) and \(C^{1+ac}\) settings.
Similar content being viewed by others
Availability of Data and Materials
Non applicable
Notes
As a straightforward computation shows, Sternberg’s germ of diffeomorphism (1) has absolutely continuous derivative as well; by [4], it embeds into a \(C^1\) flow (we do not know whether it embeds into a \(C^{1+ac}\) flow, but it follows also from [4] that it is at least \(C^1\) conjugate to a diffeomorphism that embeds in such a flow). However, Sternberg proved that it is not bi-Lipschitz conjugate to its linear part.
This example for \(\alpha = 1\) was already considered in [12, Exercise 4.1.12] However, our approach here is much more concrete (and is not based only on a “miraculous” direct integration).
References
Bonatti, C., Monteverde, I., Navas, A., Rivas, C.: Rigidity for \( C^1 \) actions on the interval arising from hyperbolicity I: solvable groups. Math. Z. 286, 919–949 (2017)
Deroin, B., Kleptsyn, V., Navas, A.: Sur la dynamique unidimensionnelle en régularité intermédiaire. Acta Math. 199(2), 199–262 (2007)
Eynard-Bontemps, H. Navas, A.: On residues and conjugacies for germs of 1-D parabolic diffeomorphisms in finite regularity. To appear in J. Inst. Math. Jussieu; arXiv: 2302.13182
Eynard-Bontemps, H., Navas, A.: The space of \(C^{1+ac}\) actions of \(\mathbb{Z}^{d}\) on a one-dimensional manifold is path-connected. Preprint (2023); arXiv:2306.17731
Cairns, G., Ghys, É.: The local linearization problem for smooth \(\rm SL (n)\)-actions. L’Enseign. Math. 43(1–2), 133–171 (1997)
Farinelli, Eglantine: Conjugacy classes of diffeomorphisms of the interval in \(C^1\) regularity Fund. Math. 237(3), 201–248 (2017)
Ghys, É., Tsuboi, T.: Différentiabilité des conjugaisons entre systèmes dynamiques de dimension 1. Ann. Inst. Fourier (Grenoble) 38, 215–244 (1988)
Kleptsyn, V., Navas, A.: A Denjoy type theorem for commuting circle diffeomorphisms with derivatives having different Hölder differentiability classes Mosc. Math. J. 8(3), 477–492 (2008)
Kopell, N.: Commuting diffeomorphisms. Global Analysis (Proc. Sympos. Pure Math., Vol. XIV, Berkeley, Calif., 1968), Amer. Math. Soc., Providence, R.I. (1970), pp 165-184
Mann, K., Wolff, M.: Reconstructing maps out of groups. Ann. Scient. École. Normale Sup. 56, 1135–1154 (2023)
Navas, A.: On centralizers of interval diffeomorphisms in critical (intermediate) regularity. J. Anal. Math. 121, 1–30 (2013)
Navas, A.: Groups of circle diffeomorphisms. Chicago Lectures in Mathematics (2011)
Sternberg, S.: Local \(C^n\) transformations of the real line. Duke Math. J. 24, 97–102 (1957)
Stowe, D.: Linearization in two dimensions. J. Differential Equ. 63(2), 183–226 (1986)
Tsuboi, T.: Homological and dynamical study on certain groups of Lipschitz homeomorphisms of the circle. J. Math. Soc. Japan 47(1), 1–30 (1995)
Yoccoz, J.-C.: Centralisateurs et conjugaison différentiable des difféomorphismes du cercle. Petits diviseurs en dimension 1. Astérisque 231, 89–242 (1995)
Zhang, W., Zhang, W.: Sharpness for \(C^1\) linearization of planar hyperbolic diffeomorphisms. J. Differential Equations 257(12), 4470–4502 (2014)
Acknowledgements
We would like to thank Étienne Ghys for having asked the question of existence of non-linearizable hyperbolic vector fields of class \(C^1\) in relation to his work with Grant Cairns on linearization of local \(\textrm{SL}(n)\)-actions [5]. We would also like to thank Jan Kiwi for his interest on the subject, as well as Frédéric Le Roux for several clever remarks. Some material of this work arose following a question of Michele Triestino about normalizers of flows near a singularity and we thank him for stimulating discussions. H. Eynard-Bontemps was detached at the CMM of University of Chile during the elaboration of this work and was partially funded by CNRS and by the IRGA project ADMIN of Grenoble INP - Université Grenoble Alpes. A. Navas was supported by the Fondecyt research project 1220032.
Funding
Hélène Eynard-Bontemps was partially funded by CNRS (Centre National de Recherche Scientifique, France), IRGA project ADMIN (Grenoble INP - Université Grenoble Alpes), Centro de Modelamiento Matemático (CMM, FB210005, BASAL funds for centers of excellence from ANID-Chile). Andrés Navas was supported by Fondecyt research project 1220032.
Author information
Authors and Affiliations
Contributions
HE-B and AN have contributed equally to the work.
Corresponding author
Ethics declarations
Conflict of interest
The authors have no relevant financial or non-financial interests to disclose.
Ethical Approval
Non applicable
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Eynard-Bontemps, H., Navas, A. On the Failure of Linearization for Germs of \(C^1\) Hyperbolic Vector Fields in Dimension One. J Dyn Diff Equat (2023). https://doi.org/10.1007/s10884-023-10330-x
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s10884-023-10330-x