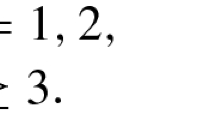Global Existence and Blow-up Solutions for a Parabolic Equation with Critical Nonlocal Interactions

Abstract

In this paper, we study the initial boundary value problem for the nonlocal parabolic equation with the Hardy–Littlewood–Sobolev critical exponent on a bounded domain. We are concerned with the long time behaviors of solutions when the initial energy is low, critical or high. More precisely, by using the modified potential well method, we obtain global existence and blow-up of solutions when the initial energy is low or critical, and it is proved that the global solutions are classical. Moreover, we obtain an upper bound of blow-up time for $J_{\mu }(u_{0})<0$ and decay rate of $H^{1}_{0}$ and $L^{2}$-norm of the global solutions. When the initial energy is high, we derive some sufficient conditions for global existence and blow-up of solutions. In addition, we are going to consider the asymptotic behavior of global solutions, which is similar to the Palais-Smale (PS for short) sequence of stationary equation.

1 Introduction and Main Results

In this paper, we consider a nonlocal parabolic initial-boundary value problem:

where $\Omega$ is a bounded domain in ${\mathbb {R}}^{N} (N\ge 3)$ and $2^{*}_{\mu }=\frac{2N-\mu }{N-2}$ is the critical exponent in the sense of the Hardy–Littlewood–Sobolev inequality. Depending on suitable properties of the initial value $u_{0}$, we are interested in the long time behaviors of solutions (global existence, blow-up in finite time) and asymptotic behavior of the global solutions.

The nonlocal parabolic equation of type (P) has important background arising from a variety of physical, chemical and biological problems. For example, problem (P) can be applied to nonlocal heat physics, where $\left( \vert x\vert ^{-\mu }*|u|^{2^{*}_{\mu }}\right) |u|^{2^{*}_{\mu }-2}u$ represents the nonlocal source, and it can also be applied to the population model with nonlocal competition, where $\left( \vert x\vert ^{-\mu }*|u|^{2^{*}_{\mu }}\right) |u|^{2^{*}_{\mu }-2}u$ models the individuals are competing not only with others at their own point in space but also with individual at other points in the domain (see [10, 18, 31, 35]), and so forth.

The following parabolic initial-boundary value problem:

$$\begin{aligned} \left\{ \begin{array}{lll} u_{t}-\Delta u=f(u), &{} x\in \Omega ,\ t>0\\ u(x,t)=0, &{} x\in \partial \Omega ,\ t>0\\ u(x,0)=u_{0}(x), &{} x\in \Omega \end{array} \right. \end{aligned}$$

(1.1)

has been extensively studied by many author with different methods. For example, critical-point theory by Ambrosetti-Rabinowitz [1], the potential well method which was constructed by Payne and Sattinger [32, 33], semigroup theory by Weissler [40, 41] and classical tools by Hoshino-Yamada [12] in a new functional analytic framework (or the monograph by Henry [11] for more detailed).

In particular, since Sattinger [33] constructed the so called stable set, the method of potential well was applied to study the existence of global solutions far and wide (see [7, 13,14,15,16, 29, 30, 38, 39] e.g.). Furthermore, Levine [19], Payne and Sattinger [32] considered blowing-up properties of solutions. When f(u) is local source term, i.e. $f(u)=\vert u\vert ^{p-1}u$, it is well known that there exist choices of initial value $u_{0}$ such that the homologous solutions global existence and the global solution tend to zero as $t\rightarrow \infty$ and there exist choices of initial value $u_{0}$ such that the homologous solutions blow-up. When the exponent is subcritical, i.e. $1<p<\frac{N+2}{N-2}$, with the help of energy functional, Nehari functional and potential well method, there exists two invariant sets W (stable set) and V (unstable set), and the long time behavior of solutions for (1.1) with low energy initial value (the energy of initial value is smaller than the depth of potential well) was described. More detailed, if initial data $u_{0}$ belongs to the stable set W, the associated solution is global, if initial data $u_{0}$ belongs to the unstable set V, the associated solution blow-up in a finite time, and blow-up in infinite time does not occur in this case (see [14, 15]). Dickstein et al. [5] generalized the above results to the critical energy level initial data. When the initial data has high energy (the energy of initial value is larger than the depth of potential well), the situation is much more complicated, since the invariance of W and V are invalid and potential well method can not be used. In [8], by using the comparison principle and variational methods, Gazzola and Weth obtained the existence of global solution and blow-up in finite time of solutions with high energy initial value. When the exponent is critical, i.e. $p=\frac{N+2}{N-2}$, by using the potential well method, Tan [36], Ikehata and Suzuki [14, 34] considered the problem (1.1), they established the existence of global solutions and blow-up of solutions in finite time, which depend on the initial value $u_{0}\in H^{1}_{0}(\Omega )$. Moreover, the asymptotic behavior of global solutions was studied. In particular, we emphasize the blow-up of case of exponent subcritical is simpler than exponent critical, since the embedding of $H^{1}_{0}(\Omega )$ into $L^{p}(\Omega )$ is compact for $p<\frac{2N}{N-2}$, while is non-compact for $p=\frac{2N}{N-2}$. From the point of view of critical point theory, the compactness condition is a sufficient and necessary condition for the PS condition to hold. Moreover, it is also a necessary condition for the nontrivial solutions existence of the stationary equation of (1.1) under conditions that do not require the geometry of the domain from the point of view of elliptic problems. In particular, contrary to the subcritical case, global unbounded solutions may exist for critical case (see [28]).

When f(u) is of a nonlocal source, i.e. $f(u)=(\vert x\vert ^{-(N-2)}*\vert u\vert ^{p})\vert u\vert ^{p-2}u$, Liu et al. considered the global existence and blow-up in finite time of solutions for problem (1.1) with $1<p<\frac{N+2}{N-2}$ by using the potential well method. In [25], they obtained a sharp threshold for global existence and finite time blow-up of solutions with lower energy initial data. In [26], they extended the results to case of critical energy initial value and obtained the asymptotic behavior of solutions. Later, they also consider the case of high energy initial value and found a criteria for global existence and blow-up in finite time of solutions respectively. Moreover, the asymptotic profile to both solutions vanishing at infinity and blowing up in finite time was established.

However, to the best of our knowledge, the nonlocal parabolic equation with critical exponent has not been studied yet. Therefore, this paper aims to study the global existence and blow-up of solution on initial value with lower energy, critical energy and high energy for problem (P). The energy functional of problem (P) is defined by

$$\begin{aligned} J_{\mu }(u)=\frac{1}{2}\int _{\Omega }|\nabla u|^{2}dx -\frac{1}{22^{*}_{\mu }} \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy. \end{aligned}$$

(1.2)

By the Hardy–Littlewood–Sobolev inequality, $J_{\mu }(u)$ is well defined in the Sobolev space $H^{1}_{0}(\Omega )$. The equation corresponds to the $L^{2}$ gradient flow associated of this energy functional. Then, along the flow generated by problem (P), we have

$$\begin{aligned} \frac{d}{dt}J_{\mu }(u)=(J_{\mu }^{\prime }(u),u_{t})=-\Vert u_{t}\Vert ^{2}_{2}. \end{aligned}$$

(1.3)

For more details, see Lemma 2.4 below.

For the Hardy–Littlewood–Sobolev critical exponent case, the corresponding functional $J_{\mu }$ does not satisfy the Palais-Smale (PS for short) condition (or $(PS)_{c}$ condition). From the critical-point theory point of view, the (PS) condition plays an important role in the proof of the existence of critical points of $J_{\mu }$, and that the stationary problem has solutions. However, by Brezis and Nirenberg [4], for $\Omega$ be a bounded domain in ${\mathbb {R}}^{N}$, any $(PS)_{c}$ sequence for $c<\frac{1}{N}S^{\frac{N}{2}}$ is relatively compact in $H^{1}_{0}(\Omega )$, where S is the best constant for the Sobolev embedding $H^{1}_{0}(\Omega )\hookrightarrow L^{2^{*}}(\Omega )$. Importantly, the Brezis-Nirenberg type critical problem for nonlinear Choquard equation was studied by Du, Gao and Yang in [6, 9], $S_{H,L}$ and the minimax level was estimated in [9] and they classify the positive solutions of this equation in [6], where $S_{H,L}$ is the best embedding constant in the sense of the Hardy–Littlewood–Sobolev inequality. In [2], Alves et al. study the singularly perturbed critical Choquard equation and establish the existence of ground states with constant coefficients. Moreover, they obtained the multiplicity of solution and the concentration behavior was characterized for perturbed problem.

Let us recall the well-known Hardy–Littlewood–Sobolev inequality, which plays a fundamental role throughout this paper.

Lemma 1.1

(Hardy–Littlewood–Sobolev inequality, see [21].) Let $t,r>1$ and $0<\mu <N$ with $1/t+\mu /N+1/r=2$. For ${\bar{f}}\in L^{t}({\mathbb {R}}^{N})$ and ${\bar{h}}\in L^{r}({\mathbb {R}}^{N})$, there exists a sharp constant $C(t,N,\mu ,r)$ independent of ${\bar{f}}$ and ${\bar{h}}$, such that

$$\begin{aligned} \int _{{\mathbb {R}}^{N}}\int _{{\mathbb {R}}^{N}}\frac{{\bar{f}}(x){\bar{h}}(y)}{|x-y|^{\mu }}dxdy\le C(t,N,\alpha ,r)\Vert {\bar{f}}\Vert _{t}\Vert {\bar{h}}\Vert _{r}. \end{aligned}$$

(1.4)

If $t=r=\frac{2N}{2N-\mu }$, then

$$\begin{aligned} C(t,N,\mu ,r)=C(N,\mu )=\pi ^{\frac{\mu }{2}}\frac{\Gamma (\frac{N}{2}-\frac{\mu }{2})}{\Gamma (N-\frac{\mu }{2})} \left\{ \frac{\Gamma (\frac{N}{2})}{\Gamma (N)}\right\} ^{-1+\frac{\mu }{N}}. \end{aligned}$$

In this case, the equality in (1.4) holds if and only if ${\bar{f}}\equiv C{\bar{h}}$ and

$$\begin{aligned} {\bar{h}}(x)=A\left( \gamma ^{2}+|x-a|^{2}\right) ^{-(2N-\mu )/2} \end{aligned}$$

for some $A\in {\mathbb {C}}$, $0\ne \gamma \in {\mathbb {R}}$ and $a\in {\mathbb {R}}^{N}$.

From the Hardy–Littlewood–Sobolev inequality, for all $u\in D^{1,2}({\mathbb R}^N)$, one has

$$\begin{aligned} {\left( \int _{{\mathbb R}^N }\int _{{\mathbb R}^N}\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\right) ^{\frac{N-2}{2N-\mu }}} \le C(N,\mu )^{\frac{N-2}{2N-\mu }}\Vert u\Vert ^{2}_{2^{*}}, \end{aligned}$$

where $C(N,\mu )$ is defined as in Lemma 1.1 and hence we call $2_{\mu }^{*}=\frac{2N-\mu }{N-2}$ the upper Hardy–Littlewood–Sobolev critical exponent. Denote best constant by

$$\begin{aligned} S_{H,L}:=\inf _{u\in H^{1}_{0}( {\mathbb R}^N )}\frac{\int _{{\mathbb R}^N}|\nabla u|^{2}dx}{\left( \int _{{\mathbb R}^N }\int _{{\mathbb R}^N }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\right) ^{\frac{N-2}{2N-\mu }}}. \end{aligned}$$

(1.5)

Lemma 1.2

[9, Lemma 1.2] The constant $S_{H,L}$ defined in (1.5) is achieved if and only if

$$\begin{aligned} U=C\left( \frac{b}{b^{2}+|x-a|^{2}}\right) ^{\frac{N-2}{2}}, \end{aligned}$$

where $C>0$ is a fixed constant, $a\in {\mathbb {R}}^{N}$ and $b\in (0,\infty )$ are parameters. Furthermore,

$$\begin{aligned} S_{H,L}=\frac{S}{C(N,\mu )^{\frac{N-2}{2N-\mu }}}, \end{aligned}$$

where S is the best Sobolev constant.

As in [42], let $U(x)=\frac{[N(N-2)]\frac{N-2}{4}}{(1+\vert x\vert ^{2})^{\frac{N-2}{2}}}$ be a minimizer for S, then

$$\begin{aligned} {\tilde{U}}=S^{\frac{(N-\mu )(2-N)}{4(N-\mu +2)}}C(N,\mu )^{\frac{2-N}{2(N-\mu +2)}}\frac{[N(N-2)]\frac{N-2}{4}}{(1+\vert x\vert ^{2})^{\frac{N-2}{2}}} \end{aligned}$$

(1.6)

is the unique positive minimizer for $S_{H,L}$ that satisfies

$$\begin{aligned} -\Delta u=\left( \vert x\vert ^{-\mu }*|u|^{2^{*}_{\mu }}\right) |u|^{2^{*}_{\mu }-2}u,\ \text {in}\ {\mathbb {R}}^{N} \end{aligned}$$

and

$$\begin{aligned} \int _{{\mathbb {R}}^{N}}\vert \nabla {\tilde{U}}\vert ^{2}dx=\int _{{\mathbb {R}}^{N}}\int _{{\mathbb {R}}^{N}}\frac{|{\tilde{U}}(x)|^{2^{*}_{\mu }}|{\tilde{U}}(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy =S^{\frac{2N-\mu }{N-\mu +2}}_{H,L}. \end{aligned}$$

Let

$$\begin{aligned} m_{\mu }:=J({\tilde{U}})=\frac{N-\mu +2}{2(2N-\mu )}S^{\frac{2N-\mu }{N-\mu +2}}_{H,L} \end{aligned}$$

(1.7)

For every open subset $\Omega$ of ${\mathbb {R}}^{N}$,

$$\begin{aligned} S_{H,L}(\Omega ):=\inf _{u\in H^{1}_{0}(\Omega )}\frac{\int _{\Omega }|\nabla u|^{2}dx}{\left( \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\right) ^{\frac{N-2}{2N-\mu }}}=S_{H,L}, \end{aligned}$$

where $S_{H,L}(\Omega )$ is never achieved except when $\Omega ={\mathbb {R}}^{N}$, see [9, Lemma 1.3].

Following [32, 36], we define stable set and unstable set as follows

$$\begin{aligned} W=\left\{ u\in H^{1}_{0}(\Omega )\ |\ J_{\mu }(u)<m_{\mu },\ I_{\mu }(u)>0\right\} \cup \left\{ 0\right\} , \end{aligned}$$

and

$$\begin{aligned} V=\left\{ u\in H^{1}_{0}(\Omega )\ |\ J_{\mu }(u)<m_{\mu },\ I_{\mu }(u)<0\right\} . \end{aligned}$$

where $I_{\mu }(u)$ is the Nehari functional for problem (P) defined by

$$\begin{aligned} I_{\mu }(u):=\int _{\Omega }|\nabla u|^{2}dx -\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy. \end{aligned}$$

(1.8)

Remark 1.3

• (i) If $0<J_{\mu }(u)<m_{\mu }$ and $I_{\mu }(u)\ge 0$, then,we have $I_{\mu }(u)>0$. Indeed, if $I_{\mu }(u)=0$, by the Hardy–Littlewood–Sobolev inequality, we have

  $$\begin{aligned} \int _{\Omega }|\nabla u|^{2}dx=\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy \le S^{-\frac{2N-\mu }{N-2}}_{H,L}\left( \int _{\Omega }|\nabla u|^{2}dx \right) ^{\frac{2N-\mu }{N-2}}, \end{aligned}$$

  which implies that $\int _{\Omega }|\nabla u|^{2}dx\ge S^{\frac{2N-\mu }{N-\mu +2}}_{H,L}$. Furthermore, by the definition of $J_{\mu }(u)$, we have $J_{\mu }(u)\ge \frac{N-\mu +2}{2(2N-\mu )}S^{\frac{2N-\mu }{N-\mu +2}}_{H,L}=m_{\mu }$, a contradiction.

• (ii) If $I_{\mu }(u)>0$, then $J_{\mu }(u)>0$. Indeed, since $I_{\mu }(u)>0$, we have

  $$\begin{aligned} \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy<\int _{\Omega }\vert \nabla u\vert ^{2}dx. \end{aligned}$$

  Furthermore, we can derive

  $$\begin{aligned} J_{\mu }(u)= & {} \frac{1}{2}\int _{\Omega }\vert \nabla u\vert ^{2}dx -\frac{1}{22^{*}_{\mu }}\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\\> & {} \frac{2^{*}_{\mu }-1}{22^{*}_{\mu }}\int _{\Omega }\vert \nabla u\vert ^{2}dx\ge 0. \end{aligned}$$

  Thus, $J_{\mu }(u)>0$.

Inspired by [36], we are going to investigate the critical parabolic equation with nonlocal interaction. By using the modified potential well method, one of the aims is to study the global existence, blow-up of solutions for problem (P) with lower energy initial value and decay rate of $H^{1}_{0}$ and $L^{2}$ norm for global solutions. Furthermore, we give an upper bound for blow-up time for $J_{\mu }(u_{0})<0$. Moreover, we also consider global existence and blow-up of solutions for problem (P) with critical energy initial value and give some sufficient conditions for the existence of global and blow-up solutions with high energy initial value. Finally, we are going to consider the asymptotic behavior of global solutions, we shall prove that there exists a sequence $\left\{ t_{n}\right\}$ such that the asymptotic behavior of $u(x,t_{n})$ as $t_{n}\rightarrow \infty$ is the PS sequence of stationary equation of problem (P).

Firstly, we state our main results about the global existence and blow-up of solutions with lower energy initial value as follows.

Theorem 1.4

If $u_{0}\in H^{1}_{0}(\Omega )$ with $J_{\mu }(u_{0})<m_{\mu }$, $I_{\mu }(u_{0})>0$, then, problem (P) has a global weak solution $u(x,t)\in L^{\infty }(0,T;H^{1}_{0}(\Omega ))$ with $u_{t}\in L^{2}(0,T;L^{2}(\Omega ))$ and $u(x,t)\in W$ for $0\le t<\infty$.

Theorem 1.5

If $u_{0}\in H^{1}_{0}(\Omega )$ with $J_{\mu }(u)<m_{\mu }$, $I_{\mu }(u)<0$, then, the weak solution u(x, t) of problem (P) blow-up in finite time. In particular, there exists a $T>0$ such that

$$\begin{aligned} \lim _{t\rightarrow T^{-}}\int ^{t}_{0}\Vert u\Vert ^{2}_{2}ds=+\infty . \end{aligned}$$

(1.9)

Moreover, for $J_{\mu }(u_{0})<0$, an upper bound for blow-up time T is given by

$$\begin{aligned} T<\frac{\Vert u_{0}\Vert ^{2}_{2}}{-42^{*}_{\mu }(2^{*}_{\mu }-1)J_{\mu }(u_{0})}. \end{aligned}$$

Furthermore, we have the decay rate of the $H^{1}_{0}$ and $L^{2}$-norm of the global solutions with lower energy initial value. Then, we state our main result of this as follows.

Theorem 1.6

Under the assumption in Theorem 1.4, for the global weak solution u(x, t) of problem (P), there exists $\alpha _{1},\alpha _{2}>0$ such that

$$\begin{aligned} \Vert \nabla u(t)\Vert ^{2}_{2}=O(e^{-\alpha _{1}t}),\ \text {as}\ t\rightarrow \infty , \end{aligned}$$

(1.10)

and

$$\begin{aligned} \Vert u(t)\Vert ^{2}_{2}=O(e^{-\alpha _{2}t}),\ \text {as}\ t\rightarrow \infty . \end{aligned}$$

(1.11)

Under the existence of global solution, we now consider the regularity of the global weak solutions with lower energy initial value, by applying a nonlocal version of the Brezis-Kato estimate, we prove that the global solutions are classical for $t\ge t_{0}>0$. The statement for more detailed as follows.

Theorem 1.7

Let u(x, t) be a global solution. Then, $u\in L^{p}(\Omega \times [t_{0},\infty ))$ for every $p\in [2,\frac{N}{N-\mu }\frac{2N}{N-2})$. In particular, u is a classical solution for $t\ge t_{0}>0$.

With the help of modified potential well method as in [24], we further study the global existence and blow-up in finite time for the case of critical energy initial value, i.e. $J_{\mu }(u_{0})= m_{\mu }$. Before state our main results of global existence and finite time blow-up of solutions, we give a remark.

Remark 1.8

If $J_{\mu }(u)= m_{\mu }$, $I_{\mu }(u)\ge 0$, then $I_{\mu }(u)>0$. Indeed, we can know that the Hardy–Littlewood–Sobolev constant is not attained on a bounded domain, and hence $E=\left\{ u\in H^{1}_{0}(\Omega )\,\ u \ \text {satisfies}\ -\Delta u=\left( \vert x\vert ^{-\mu }*|u|^{2^{*}_{\mu }}\right) |u|^{2^{*}_{\mu }-2}u\ \text {and}\ J_{\mu }(u)= m_{\mu } \right\} =\emptyset$ (see [9, Lemma 1.3]). However, the case $J_{\mu }(u)=m_{\mu }$ and $I_{\mu }(u)=0$ means means $u\in E$, this is impossible.

Theorem 1.9

Let $u_{0}(x)\in H^{1}_{0}(\Omega )$, $J_{\mu }(u_{0})= m_{\mu }$. Then,(i) If $I_{\mu }(u_{0})>0$, then the problem (P) has a global weak solution $u\in L^{\infty }(0,T;H^{1}_{0}(\Omega ))$ with $u_{t}\in L^{2}(0,T;L^{2}(\Omega ))$ and $u(x,t)\in {\bar{W}}$ for $0\le t<\infty$. Moreover, there exists $\alpha _{1},\alpha _{2}>0$ such that

$$\begin{aligned} \Vert \nabla u(t)\Vert ^{2}_{2}=O(e^{-\alpha _{1}t}),\ \text {as}\ t\rightarrow \infty , \end{aligned}$$

and

$$\begin{aligned} \Vert u(t)\Vert ^{2}_{2}=O(e^{-\alpha _{2}t}),\ \text {as}\ t\rightarrow \infty . \end{aligned}$$

(ii) If $I_{\mu }(u_{0})<0$, then the solutions of problem (P) blows up in finite time. In particular, there exists a $T>0$ such that

$$\begin{aligned} \lim _{t\rightarrow T^{-}}\int ^{t}_{0}\Vert u\Vert ^{2}_{2}ds=+\infty . \end{aligned}$$

In view of above results, for the case $J_{\mu }(u_{0})\le m_{\mu }$, whether or not the solution for problem (P) exists globally is totally determined by the Nehari functional, and it is natural to ask what will happen when $J_{\mu }(u_{0})>m_{\mu }$. However, since the invariance of W and V under the flow of (1.3) is invalid, potential well method can not be used for this case. To this end, we now introduce the following sets as in [8], define

$$\begin{aligned} {\mathcal {N}}_{+}=\{u\in H^{1}_{0}(\Omega )\ |\ I_{\mu }(u)>0\}\ \text {and}\ {\mathcal {N}}_{-}=\{u\in H^{1}_{0}(\Omega )\ |\ I_{\mu }(u)<0\}, \end{aligned}$$

and

$$\begin{aligned} J_{\mu }^{d}:=\{u\in H^{1}_{0}(\Omega )\ |\ J_{\mu }(u)<d\}. \end{aligned}$$

Furthermore, for all $d>m_{\mu }$, set

$$\begin{aligned} \lambda _{d}=\inf \{\Vert u\Vert ^{2}_{2}\ |\ u\in {\mathcal {N}}_{d}\}\ \text {and}\ \Lambda _{d}=\sup \{\Vert u\Vert ^{2}_{2}\ |\ u\in {\mathcal {N}}_{d}\}, \end{aligned}$$

Next, we also introduce the following sets

$$\begin{aligned} {\mathcal {B}}&=\left\{ u_{0}\in H^{1}_{0}(\Omega )\ |\ \text {the solution}\ u=u(t)\ \text {of } ({ P}) \text { blows up in finite time} \right\} ,\\ {\mathcal {G}}&=\left\{ u_{0}\in H^{1}_{0}(\Omega )\ |\ \text {the solution}\ u=u(t)\ \text {of } ({ P}) \text { exist for all}\ t>0 \right\} ,\\ {\mathcal {G}}_{0}&=\left\{ u_{0}\in {\mathcal {G}}\ |\ u(t)\rightarrow 0\ \text {in}\ H^{1}_{0}(\Omega )\ \text {as}\ t\rightarrow \infty \right\} . \end{aligned}$$

Then, we can characterize the sets ${\mathcal {B}}$, ${\mathcal {G}}$ and ${\mathcal {G}}_{0}$, that is, to determine the global existence and blow-up of the solution of (P) whose initial value $u_{0}$ in $H^{1}_{0}(\Omega )$. Our main results for $J_{\mu }(u_{0})>m_{\mu }$ are to show as follows.

Theorem 1.10

Assume that $J_{\mu }(u_{0})>m_{\mu }$, then the following statements hold

• (i) If $u_{0}\in {\mathcal {N}}_{+}$ and $\Vert u_{0}\Vert _{2}\le \lambda _{J(u_{0})}$, then $u_{0}\in {\mathcal {G}}_{0}$;

• (ii) If $u_{0}\in {\mathcal {N}}_{-}$ and $\Vert u_{0}\Vert _{2}\ge \Lambda _{J(u_{0})}$, then $u_{0}\in {\mathcal {B}}$.

Theorem 1.11

For $0<\mu <\min \left\{ 4,N\right\}$. If $u_{0}\in H^{1}_{0}(\Omega )$ satisfies

$$\begin{aligned} \Vert u_{0}\Vert ^{22^{*}_{\mu }}_{2}\ge \frac{22^{*}_{\mu }}{2^{*}_{\mu }-1}(r_{\Omega })^{\mu }\vert \Omega \vert ^{2^{*}_{\mu }-2}J_{\mu }(u_{0}), \end{aligned}$$

(1.12)

where $r_{\Omega }:=diam(\Omega )=\sup _{x,y\in \Omega }\vert x-y\vert <\infty$. Then, $u_{0}\in {\mathcal {N}}_{-}\cap {\mathcal {B}}$.

Theorem 1.12

For any $M>0$, there exists $u_{M}\in {\mathcal {N}}_{-}$ such that $J(u_{M})\ge M$ and $u_{M}\in {\mathcal {B}}$.

Finially, we consider the asymptotic behavior of the global solutions, which is similar to the Palais-Smale (PS for short) sequence of stationary equation.

Theorem 1.13

Let $u(x,t;u_{0})$ be a global solution of the problem (P) and uniformly bounded in $H^{1}_{0}(\Omega )$ with respect to t. Then, for any subsequence $t_{n}\rightarrow \infty$, there exists a stationary solution w such that $u(x,t_{n};u_{0})\rightarrow w$ in $H^{1}_{0}(\Omega )$.

Theorem 1.14

Let $u(x,t;u_{0})$ be a global solution of the problem (P). Then, its $\omega$-limit contains a stationary solution w.

The rest of this paper is organized as follows. In Sect. 2, we give some notations and definitions, introduce potential well sets and prove local existence theorem of the problem (P) in subsection of Sect. 2. Next, we will give global existence and blow up of the problem (P) with lower energy initial value, critical energy initial value and high energy initial value in Sects. 3, 4, 5 respectively. In Sect. 6, we prove Theorems 1.13 and 1.14.

2 Preliminaries

In this section, let us first give some definitions of the weak solution, maximal existence time and finite time blow-up. And then we introduce some functions and notations. Final, we give local existence result of solutions for problem (P). Throughout this paper, we denote $\Vert \cdot \Vert _{L^{q}(\Omega )}$ by $\Vert \cdot \Vert _{q}$ for $1\le q\le \infty$ and C is a constant that can change from one line to another.

2.1 Definitions

Definition 2.1

(Weak solution). We say that a function $u=u(x,t)$ is a weak solution of problem (P) in $Q_{T}:=\Omega \times (0,T)$ if and only if

$$\begin{aligned} u\in L^{\infty }(0,T;H^{1}_{0}(\Omega )),\ u_{t}\in L^{2}(Q_{T})=L^{2}(0,T;L^{2}(\Omega )), \end{aligned}$$

and satisfies problem (P) in the distribution sense, that is

$$\begin{aligned} (u_{t},\phi )+(\nabla u,\nabla \phi )=\left( \left( |x|^{-\mu }*|u|^{2^{*}_{\mu }}\right) |u|^{2^{*}_{\mu }-2}u,\phi \right) ,\ \forall \phi \in H^{1}_{0}(\Omega ),\ t\in (0,T), \end{aligned}$$

(2.1)

where $u(x,0)=u_{0}(x)\in H^{1}_{0}(\Omega )$ and $(\cdot ,\cdot )$ denote the $L^{2}(\Omega )$-inner product.

Definition 2.2

(Maximal existence time). Let u(x, t) be a weak solution of problem (P). We define the maximum existence time $T_{\max }$ of u(x, t) as follows:

• (i) if u(x, t) exists for all $0\le t<\infty$, then $T_{\max }=\infty$;

• (ii) if there exists $t^{*}\in (0,\infty )$ such that u(x, t) exists for all $0\le t<t^{*}$, but does not exist at $t=t^{*}$, then $T_{\max }=t^{*}$.

Definition 2.3

(Finite time blow-up). Let u(x, t) be a weak solution of problem (P). We say u(x, t) blow-up in finite time if the maximal existence time $T_{\max }$ is finite and

$$\begin{aligned} \lim _{t\rightarrow T^{-}_{\max }}\Vert u(\cdot ,t)\Vert _{L^{2}(\Omega )}=+\infty . \end{aligned}$$

Multiplying (P) by u and $u_{t}$ respectively and then integrating over $\Omega$, we can get

$$\begin{aligned} \frac{d}{dt}\left( \frac{1}{2}\int _{\Omega }u^{2}dx\right) +\int _{\Omega }|\nabla u|^{2}dx =\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy \end{aligned}$$

(2.2)

and

$$\begin{aligned} \int _{\Omega }|u_{t}|^{2}dx =-\frac{d}{dt}\left( \frac{1}{2}\int _{\Omega }|\nabla u|^{2}dx\right) +\frac{d}{dt}\left( \frac{1}{22^{*}_{\mu }} \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\right) . \qquad \end{aligned}$$

(2.3)

Then, by (2.3), we have the following Lemma.

Lemma 2.4

(Energy identity). For $0<T\le \infty$ and let u(x, t) be a weak solution of problem (P) on [0, T) with initial value $u_{0}\in H^{1}_{0}(\Omega )$. Then, $J_{\mu }(u(t))$ is non-increasing with respect to t. More precisely,

$$\begin{aligned} \int ^{t}_{s}\Vert u_{\tau }\Vert _{2}^{2}d\tau +J_{\mu }(u(t)) =J_{\mu }(u(s)), \end{aligned}$$

(2.4)

for any $0\le s\le t<T$.

2.2 Introduction of Potential Well

In this subsection, we shall introduce a class of potential wells for problem (P). Firstly, let us give some properties of $J_{\mu }(u)$ and $I_{\mu }(u)$.

Lemma 2.5

Let $u\in H^{1}_{0}(\Omega )\backslash \{0\}$. Then,

• (i) $\lim _{s\rightarrow 0}J_{\mu }(su)=0$ and $\lim _{s\rightarrow +\infty }J_{\mu }(su)=-\infty$;

• (ii) there exists a unique ${\bar{s}}=s(u)>0$ such that

  $$\begin{aligned} \frac{d}{d s}J_{\mu }(su)|_{s={\bar{s}}}=0; \end{aligned}$$

  (2.5)

• (iii) $J_{\mu }(su)$ is increasing on $0\le s\le {\bar{s}}$, decreasing on ${\bar{s}}\le s\le +\infty$ and takes the maximum at $s={\bar{s}}$.

• (iv) $I_{\mu }(su)>0$ for $0<s<{\bar{s}}$, $I_{\mu }(su)<0$ for ${\bar{s}}<s<+\infty$ and $I_{\mu }({\bar{s}} u)=0$.

Proof

(i):

By the definition of $J_{\mu }(u)$ in (1.2), we can get

$$\begin{aligned} J_{\mu }(su)= & {} \frac{1}{2}\int _{\Omega }|\nabla (su)|^{2}dx -\frac{1}{22^{*}_{\mu }} \int _{\Omega }\int _{\Omega }\frac{|su(x)|^{2^{*}_{\mu }}|su(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\nonumber \\= & {} \frac{s^{2}}{2}\int _{\Omega }|\nabla u|^{2}dx -\frac{s^{22^{*}_{\mu }}}{22^{*}_{\mu }} \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy, \end{aligned}$$

(2.6)

which implies that

$$\begin{aligned} \lim _{s\rightarrow 0}J_{\mu }(su)=0\ \text {and}\ \lim _{s\rightarrow +\infty }J_{\mu }(su)=-\infty . \end{aligned}$$

Consequently, the proof of (i) is complete.

(ii):

By (2.6), one can derive that

$$\begin{aligned} \frac{d}{ds}J_{\mu }(su)=s\left( \int _{\Omega }|\nabla u|^{2}dx -s^{22^{*}_{\mu }-2}\int _{\Omega }\int _{\Omega } \frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\right) . \end{aligned}$$

(2.7)

Therefore, there exist a unique

$$\begin{aligned} {\bar{s}}:=\left( \frac{\int _{\Omega }|\nabla u|^{2}dx}{\int _{\Omega }\int _{\Omega } \frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy}\right) ^{1/(22^{*}_{\mu }-2)} \end{aligned}$$

such that (2.5) is true.

(iii):

By (2.7), we get $\frac{d}{ds}J_{\mu }(su)\ge 0$ on $0\le s\le {\bar{s}}$ and $\frac{d}{ds}J_{\mu }(su)\le 0$ on ${\bar{s}}\le s\le +\infty$. Hence, $J_{\mu }(su)$ is increasing on $0\le s\le {\bar{s}}$, decreasing on ${\bar{s}}\le s\le +\infty$ and takes the maximum at $s={\bar{s}}$.

(iv):

By the definition of $I_{\mu }(u)$ in (1.8) and (2.7), we have

$$\begin{aligned} I_{\mu }(su)=s^{2}\int _{\Omega }|\nabla u|^{2}dx -s^{22^{*}_{\mu }}\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy =s\frac{d}{ds}J_{\mu }(su). \end{aligned}$$

Then, by (ii), the proof is complete. $\square$

Now, for $0<\delta <2^{*}_{\mu }$, we define

$$\begin{aligned} I_{\mu ,\delta }(u):=\delta \Vert \nabla u\Vert ^{2}_{2} -\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy, \end{aligned}$$

(2.8)

and

$$\begin{aligned} m_{\mu }(\delta ):=\inf _{u\in {\mathcal {N}}_{\mu ,\delta }}J_{\mu }(u), \end{aligned}$$

(2.9)

where

$$\begin{aligned} {\mathcal {N}}_{\mu ,\delta }:=\{u\in H_{0}^{1}(\Omega )\backslash \{0\}\ |\ I_{\mu ,\delta }(u)=0\}. \end{aligned}$$

Then, as in [24], we define modified potential wells by

$$\begin{aligned} W_{\delta }=\left\{ u\in H^{1}_{0}(\Omega )\ |\ J_{\mu }(u)<m_{\mu }(\delta ),\ I_{\mu ,\delta }(u)>0\right\} \cup \left\{ 0\right\} , \end{aligned}$$

and

$$\begin{aligned} V_{\delta }=\left\{ u\in H^{1}_{0}(\Omega )\ |\ J_{\mu }(u)<m_{\mu }(\delta ),\ I_{\mu ,\delta }(u)<0\right\} . \end{aligned}$$

Let

$$\begin{aligned} r(\delta ):=\left( \delta S_{H,L}^{\frac{2N-\mu }{N-2}}\right) ^{\frac{N-2}{N-\mu +2}}, \end{aligned}$$

Then, we have the following results.

Lemma 2.6

Let $u\in H^{1}_{0}(\Omega )\backslash \{0\}$.

• (i) If $0<\Vert \nabla u\Vert ^{2}_{2}<r(\delta )$, then $I_{\mu ,\delta }(u)>0$. In particular, if $0<\Vert \nabla u\Vert ^{2}_{2}<r(1)$, then $I_{\mu }(u)>0$.

• (ii) If $I_{\mu ,\delta }(u)<0$, then $\Vert \nabla u\Vert ^{2}_{2}>r(\delta )$. In particular, if $I_{\mu }(u)<0$, then $\Vert \nabla u\Vert ^{2}_{2}>r(1)$.

• (iii) If $I_{\mu ,\delta }(u)=0$, then $\Vert \nabla u\Vert ^{2}_{2}\ge r(\delta )$ or $\Vert \nabla u\Vert ^{2}_{2}=0$. In particular, if $I_{\mu }(u)=0$, then $\Vert \nabla u\Vert ^{2}_{2}\ge r(1)$ or $\Vert \nabla u\Vert ^{2}_{2}=0$.

• (iv) If $I_{\mu ,\delta }(u)=0$ and $\Vert \nabla u\Vert ^{2}_{2}\ne 0$, then $J_{\mu }(u)>0$ for $0<\delta <2^{*}_{\mu }$, $J_{\mu }(u)=0$ for $\delta =2^{*}_{\mu }$, $J_{\mu }(u)<0$ for $\delta >2^{*}_{\mu }$.

Proof

(i):

By (2.8) and the Hardy–Littlewood–Sobolev inequality, we have

$$\begin{aligned} I_{\mu ,\delta }(u)= & {} \delta \Vert \nabla u\Vert _{2}^{2} -\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\\\ge & {} \Vert \nabla u\Vert _{2}^{2}\left( \delta -S_{H,L}^{-\frac{2N-\mu }{N-2}}(\Vert \nabla u\Vert ^{2}_{2})^{\frac{N-\mu +2}{N-2}}\right) . \end{aligned}$$

Hence, we have $I_{\mu ,\delta }(u)>0$, since $0<\Vert \nabla u\Vert ^{2}_{2}<r(\delta )$.

(ii):

Since $I_{\mu ,\delta }(u)<0$, using (2.8) and the Hardy–Littlewood–Sobolev inequality again, we can get

$$\begin{aligned} \delta \Vert \nabla u\Vert _{2}^{2} <\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy \le S_{H,L}^{-\frac{2N-\mu }{N-2}}(\Vert \nabla u\Vert ^{2}_{2})^{\frac{2N-\mu }{N-2}}. \end{aligned}$$

Hence, $\Vert \nabla u\Vert ^{2}_{2}>r(\delta )$.

(iii):

Obviously, if $\Vert \nabla u\Vert ^{2}_{2}=0$, then $I_{\mu ,\delta }(u)=0$. So, we assume that $I_{\mu ,\delta }(u)=0$ and $\Vert \nabla u\Vert ^{2}_{2}\ne 0$. By (2.8) and the Hardy–Littlewood–Sobolev inequality, one has

$$\begin{aligned} \delta \Vert \nabla u\Vert _{2}^{2} =\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy \le S_{H,L}^{-\frac{2N-\mu }{N-2}}(\Vert \nabla u\Vert ^{2}_{2})^{\frac{2N-\mu }{N-2}} \end{aligned}$$

which implies that $\Vert \nabla u\Vert ^{2}_{2}\ge r(\delta )$.

(iv):

Since $I_{\mu ,\delta }(u)=0$ and $\Vert \nabla u\Vert ^{2}_{2}\ne 0$, by (iii) above, we have $\Vert \nabla u\Vert ^{2}_{2}\ge r(\delta )$. Furthermore, it follows from (1.2) that

$$\begin{aligned} J_{\mu }(u)= & {} \frac{1}{2}\int _{\Omega }|\nabla u|^{2}dx -\frac{1}{22^{*}_{\mu }} \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\\= & {} \left( \frac{1}{2}-\frac{\delta }{22^{*}_{\mu }}\right) \int _{\Omega }|\nabla u|^{2}dx \ge \left( \frac{1}{2}-\frac{\delta }{22^{*}_{\mu }}\right) r(\delta ). \end{aligned}$$

Consequently, the proof is complete. $\square$

Lemma 2.7

$m_{\mu }(\delta )$ defined in (2.9) satisfies

• (i) $m_{\mu }(\delta )\ge a(\delta )r(\delta )$ for $0<\delta <2^{*}_{\mu }$, where $a(\delta ):=\frac{1}{2}-\frac{\delta }{22^{*}_{\mu }}$;

• (ii) $\lim _{\delta \rightarrow 0}m_{\mu }(\delta )=0$, $m_{\mu }(2^{*}_{\mu })=0$ and $m_{\mu }(\delta )<0$ for $\delta >2^{*}_{\mu }$;

• (iii) $m_{\mu }(\delta )$ is increasing on $0<\delta \le 1$, decreasing in $1<\delta <2^{*}_{\mu }$ and takes the maximum $m_{\mu }(\delta )=m_{\mu }(1)$ at $\delta =1$.

Proof

(i):

For any $u\in {\mathcal {N}}_{\mu ,\delta }$, we have $I_{\mu ,\delta }(u)=0$ and $\Vert \nabla u\Vert ^{2}_{2}\ne 0$. It follows from Lemma 2.6 (iii) that $\Vert \nabla u\Vert ^{2}_{2}\ge r(\delta )$. Furthermore, we can deduce that

$$\begin{aligned} J_{\mu }(u)= & {} \frac{1}{2}\int _{\Omega }|\nabla u|^{2}dx -\frac{1}{22^{*}_{\mu }} \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\\= & {} \left( \frac{1}{2}-\frac{\delta }{22^{*}_{\mu }}\right) \int _{\Omega }|\nabla u|^{2}dx \ge \left( \frac{1}{2}-\frac{\delta }{22^{*}_{\mu }}\right) r(\delta ). \end{aligned}$$

Therefore, $m_{\mu }(\delta )\ge a(\delta )r(\delta )$, where $a(\delta ):=\frac{1}{2}-\frac{\delta }{22^{*}_{\mu }}$.

(ii):

Fix $u\in H^{1}_{0}(\Omega )$ and $\Vert \nabla u\Vert ^{2}_{2}\ne 0$ and let ${\tilde{s}}u\in {\mathcal {N}}_{\mu ,\delta }$, i.e.

$$\begin{aligned} 0=I_{\mu ,\delta }({\tilde{s}}u)=\delta \Vert \nabla ({\tilde{s}}u)\Vert _{2}^{2} -\int _{\Omega }\int _{\Omega }\frac{|{\tilde{s}}u(x)|^{2^{*}_{\mu }}|{\tilde{s}}u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy. \end{aligned}$$

Then, we can derive

$$\begin{aligned} {\tilde{s}}:=s(\delta )=\left( \frac{\delta \Vert \nabla u\Vert _{2}^{2}}{\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy}\right) ^{1/(22^{*}_{\mu }-2)}, \end{aligned}$$

(2.10)

and

$$\begin{aligned} \lim _{\delta \rightarrow 0}{\tilde{s}}=0. \end{aligned}$$

(2.11)

Furthermore, by Lemma 2.5(i) and (2.11), we can get

$$\begin{aligned} \lim _{\delta \rightarrow 0}J_{\mu }({\tilde{s}} u)=\lim _{{\tilde{s}}\rightarrow 0}J_{\mu }({\tilde{s}}u)=0. \end{aligned}$$

Hence,

$$\begin{aligned} \lim _{\delta \rightarrow 0}m_{\mu }(\delta )=0. \end{aligned}$$

Next, by Lemma 2.6 (iv), we can get $m_{\mu }(2^{*}_{\mu })=0$ and $m_{\mu }(\delta )<0$ for $\delta >2^{*}_{\mu }$.

(iii):

It is enough to prove that for any $0<\delta ^{\prime }<\delta ^{\prime \prime }<1$ or $1<\delta ^{\prime \prime }<\delta ^{\prime }<2^{*}_{\mu }$ and for any $u\in {\mathcal {N}}_{\mu ,\delta ^{\prime \prime }}$, there exist a $v\in {\mathcal {N}}_{\mu ,\delta ^{\prime }}$ and a constant $c(\delta ^{\prime },\delta ^{\prime \prime })$ such that $J_{\mu }(v)<J_{\mu }(u)-c(\delta ^{\prime },\delta ^{\prime \prime })$. Indeed, for $u\in {\mathcal {N}}_{\mu ,\delta ^{\prime \prime }}$, we define $s(\delta )$ as (2.10), then $I_{\mu ,\delta }(s(\delta )u)=0$ and $s(\delta ^{\prime \prime })=1$. Let $h(s)=J_{\mu }(su)$, we can get

$$\begin{aligned} \frac{d}{d s}h(s)= & {} s\Vert \nabla u\Vert _{2}^{2} -s^{22^{*}_{\mu }-1}\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\\= & {} \frac{1}{s}\left( (1-\delta )\Vert \nabla (su)\Vert _{2}^{2}+I_{\mu ,\delta }(su)\right) . \end{aligned}$$

Take $v=s(\delta ^{\prime })u$, then $v\in {\mathcal {N}}_{\mu ,\delta ^{\prime }}$. For $0<\delta ^{\prime }<\delta ^{\prime \prime }<1$, we have

$$\begin{aligned} J_{\mu }(u)-J_{\mu }(v)= & {} h(1)-h(s(\delta ^{\prime }))\\ {}> & {} (1-\delta ^{\prime \prime })s(\delta ^{\prime })r(\delta ^{\prime \prime })(1-s(\delta ^{\prime })) \equiv c(\delta ^{\prime },\delta ^{\prime \prime }) . \end{aligned}$$

For $1<\delta ^{\prime \prime }<\delta ^{\prime }<2^{*}_{\mu }$, we have

$$\begin{aligned} J_{\mu }(u)-J_{\mu }(v)= & {} {} h(1)-h(s(\delta ^{\prime }))\\> & {} {} (\delta ^{\prime \prime }-1)s(\delta ^{\prime \prime })r(\delta ^{\prime \prime })(s(\delta ^{\prime })-1) \equiv c(\delta ^{\prime },\delta ^{\prime \prime }) \end{aligned}$$

Therefore, the proof of (iii) is complete. $\square$

2.3 Local Existence

In this subsection, we shall give local existence result in $H^{1}_{0}(\Omega )$ for problem (P) by applying the method of [12, 14]. Denote $A=-\Delta$ with the Dirichlet null condition in $L^{2}(\Omega )$, and define the fractional powers $A^{\alpha }$ of A and the semigroup $\left\{ e^{tA}\right\} _{t\ge 0}$ generated by A as in [37]. Before state our main result, we introduce a lemma as follows.

Lemma 2.8

[12, Lemma 2.1]

• (i) For each $\theta \ge 0$, there exist a positive constant $C_{1}(\theta )$ such that

  $$\begin{aligned} \Vert A^{\theta }e^{-tA}u\Vert _{2}\le C_{1}(\theta )t^{-\theta }e^{-\lambda t}\Vert u\Vert _{2} \end{aligned}$$

  for all $u\in L^{2}(\Omega )$ and $t>0$.

• (ii) For each $0\le \theta \le 1$, there exist a positive constant $C_{2}(\theta )$ such that

  $$\begin{aligned} \Vert (e^{-tA}-I)u\Vert _{2}\le C_{2}(\theta )t^{\theta }\Vert A^{\theta }u\Vert _{2} \end{aligned}$$

  for all $u\in D(A^{\theta })$ and $t>0$.

• (iii) For each $\theta >0$ and $u\in L^{2}(\Omega )$,

  $$\begin{aligned} t^{\theta }\Vert A^{\theta }e^{-tA}u\Vert _{2}\rightarrow 0\ \text {as}\ t\rightarrow 0. \end{aligned}$$

Proposition 2.9

Suppose that $0<\mu <\min \left\{ N,4\right\}$. For each $u_{0}\in H^{1}_{0}(\Omega )$, there exists a $T>0$ such that problem (P) has a unique solution $u(t)\in C([0,T];H^{1}_{0}(\Omega ))$ satisfying:

• (i) $u(t)\in C((0,T];D(A^{\alpha }))\cap C((0,T];D(A^{\beta }))$;

• (ii) $u(t)=e^{-tA}u_{0}+\int _{0}^{t}e^{-(t-s)A}\left[ \left( \vert x\vert ^{-\mu }*|u(s)|^{2^{*}_{\mu }}\right) |u(s)|^{2^{*}_{\mu }-2}u(s)\right] ds$;

• (iii) $\lim _{t\rightarrow 0}t^{\alpha -\frac{1}{2}}\Vert A^{\alpha }u(t)\Vert _{2}=0$ and $\lim _{t\rightarrow 0}t^{\beta -\frac{1}{2}}\Vert A^{\beta }u(t)\Vert _{2}=0$.

Proof

Note that

$$\begin{aligned} \Vert f(u)-f(v)\Vert ^{2}_{2}= & {} \int _{\Omega }\left| \left( \vert x\vert ^{-\mu }*|u|^{2^{*}_{\mu }}\right) |u|^{2^{*}_{\mu }-2}u -\left( \vert x\vert ^{-\mu }*|v|^{2^{*}_{\mu }}\right) |v|^{2^{*}_{\mu }-2}v\right| ^{2}dx\nonumber \\\le & {} \int _{\Omega }\left| \left( \vert x\vert ^{-\mu }*|u|^{2^{*}_{\mu }}\right) \left( |u|^{2^{*}_{\mu }-2}u-|v|^{2^{*}_{\mu }-2}v\right) \right. \nonumber \\{} & {} \left. +\left( \vert x\vert ^{-\mu }*\left( |u|^{2^{*}_{\mu }}-|v|^{2^{*}_{\mu }}\right) \right) |v|^{2^{*}_{\mu }-2}v\right| ^{2}dx\nonumber \\\le & {} 2(I_{1}+I_{2}), \end{aligned}$$

(2.12)

where $f(u)=\left( \vert x\vert ^{-\mu }*|u|^{2^{*}_{\mu }}\right) |u|^{2^{*}_{\mu }-2}u$,

$$\begin{aligned} I_{1}:=\int _{\Omega }\left| \left( \vert x\vert ^{-\mu }*|u|^{2^{*}_{\mu }}\right) \left( |u|^{2^{*}_{\mu }-2}u-|v|^{2^{*}_{\mu }-2}v\right) \right| ^{2}dx, \end{aligned}$$

and

$$\begin{aligned} I_{2}:=\int _{\Omega }\left| \left( \vert x\vert ^{-\mu }*\left( |u|^{2^{*}_{\mu }}-|v|^{2^{*}_{\mu }}\right) \right) |v|^{2^{*}_{\mu }-2}v\right| ^{2}dx. \end{aligned}$$

By the Hölder inequality, the Hardy–Littlewood–Sobolev inequality and the mean value theorem, we have

$$\begin{aligned} I_{1}= & {} \int _{\Omega }\left| \left( \vert x\vert ^{-\mu }*|u|^{2^{*}_{\mu }}\right) \left( |u|^{2^{*}_{\mu }-2}u-|v|^{2^{*}_{\mu }-2}v\right) \right| ^{2}dx\nonumber \\\le & {} C\left\| \vert x\vert ^{-\mu }*|u|^{2^{*}_{\mu }}\right\| ^{2}_{2q}\left( \int _{\Omega }\left| |\xi |^{2^{*}_{\mu }-2}(u-v)\right| ^{2q^{\prime }}dx\right) ^{1/q^{\prime }}\nonumber \\\le & {} C\Vert u\Vert ^{22^{*}_{\mu }}_{2^{*}_{\mu }r}\left( \int _{\Omega }|\xi |^{2(2^{*}_{\mu }-2)q^{\prime }\frac{2^{*}_{\mu }-1}{2^{*}_{\mu }-2}}dx\right) ^{\frac{2^{*}_{\mu }-2}{{(2^{*}_{\mu }-1)q^{\prime }}}} \left( \int _{\Omega }\vert u-v\vert ^{2q^{\prime }(2^{*}_{\mu }-1)}dx\right) ^{\frac{1}{(2^{*}_{\mu }-1)q^{\prime }}}\nonumber \\\le & {} C\Vert u\Vert ^{22^{*}_{\mu }}_{2^{*}_{\mu }r} \left( \Vert u\Vert _{2(2^{*}_{\mu }-1)q^{\prime }}+\Vert v\Vert _{2(2^{*}_{\mu }-1)q^{\prime }}\right) ^{2(2^{*}_{\mu }-2)}\Vert u-v\Vert _{2(2^{*}_{\mu }-1)q^{\prime }} ^{2}, \end{aligned}$$

(2.13)

where $\xi (x)$ is a function between $\vert u(x)\vert$ and $\vert v(x)\vert$, C are different constants from one line to another, $q, q^{\prime }\in [1,+\infty ]$ are conjugate and $r\in (1,+\infty )$ satisfies

$$\begin{aligned} \frac{1}{r}=\frac{1}{2q}+\frac{N-\mu }{N}. \end{aligned}$$

(2.14)

Similarly, by the Hölder inequality, the Hardy–Littlewood–Sobolev inequality and the mean value theorem, we also have

$$\begin{aligned} I_{2}= & {} \int _{\Omega }\left| \left( \vert x\vert ^{-\mu }*\left( |u|^{2^{*}_{\mu }}-|v|^{2^{*}_{\mu }}\right) \right) |v|^{2^{*}_{\mu }-2}v\right| ^{2}dx\nonumber \\\le & {} \left( \int _{\Omega }\left| \vert x\vert ^{-\mu }*\left( |u|^{2^{*}_{\mu }}-|v|^{2^{*}_{\mu }}\right) \right| ^{2q}dx\right) ^{1/q} \left( \int _{\Omega }\left| |v|^{2^{*}_{\mu }-2}v \right| ^{2q\prime }dx\right) ^{1/q^{\prime }}\nonumber \\\le & {} C\left( \int _{\Omega }\left| |\xi |^{2^{*}_{\mu }-2}\xi (u-v) \right| ^{r}dx\right) ^{2/r} \Vert v\Vert ^{2(2^{*}_{\mu }-1)}_{2(2^{*}_{\mu }-1)q^{\prime }}\nonumber \\\le & {} C\Vert v\Vert ^{2(2^{*}_{\mu }-1)}_{2(2^{*}_{\mu }-1)q^{\prime }}\left( \Vert u\Vert _{2^{*}_{\mu }r}+\Vert v\Vert _{2^{*}_{\mu }r}\right) ^{2(2^{*}_{\mu }-1)}\Vert u-v\Vert ^{2}_{2^{*}_{\mu }r}, \end{aligned}$$

(2.15)

where $q, q^{\prime }$ and r were defined as above and C are different constants from one line to another. Hence, it follows from (2.12)–(2.13) and (2.15) that

$$\begin{aligned} \Vert f(u)-f(v)\Vert _{2}\le & {} C\Vert u\Vert ^{2^{*}_{\mu }}_{2^{*}_{\mu }r} \left( \Vert u\Vert _{2(2^{*}_{\mu }-1)q^{\prime }}+\Vert v\Vert _{2(2^{*}_{\mu }-1)q^{\prime }}\right) ^{2^{*}_{\mu }-2}\Vert u-v\Vert _{2(2^{*}_{\mu }-1)q^{\prime }} \nonumber \\{} & {} +C\Vert v\Vert ^{2^{*}_{\mu }-1}_{2(2^{*}_{\mu }-1)q^{\prime }}\left( \Vert u\Vert _{2^{*}_{\mu }r}+\Vert v\Vert _{2^{*}_{\mu }r}\right) ^{2^{*}_{\mu }-1}\Vert u-v\Vert _{2^{*}_{\mu }r}. \end{aligned}$$

(2.16)

Let

$$\begin{aligned} \alpha :=\frac{1}{2}+\frac{N-2}{2^{\theta }}\ \text {and}\ \beta :=\frac{2^{\theta -1}(2N-\mu )-(N-2)(2N-\mu )}{2^{\theta }(N-\mu +2)}, \end{aligned}$$

where $\theta >0$ large enough such that $\alpha >\frac{1}{2}$ and close to $\frac{1}{2}$. Clearly, $\alpha ,\beta \in (\frac{1}{2},1)$ provided that $N>2$ and $0<\mu <\min \left\{ N,4\right\}$. And taking

$$\begin{aligned} q=\frac{2^{\theta -2}N}{2^{\theta -2}\mu -(2N-\mu )},\ q^{\prime }=\frac{2^{\theta -2}N}{2^{\theta -2}(N-\mu )+(2N-\mu )},\ r=\frac{2N}{2N-\mu }\frac{2^{\theta -2}}{2^{\theta -2}-1}\,. \end{aligned}$$

Obviously, $q, q^{\prime }\in [1,+\infty ]$ are conjugate and $r\in (1,+\infty )$ satisfies (2.14).

Since $2^{*}_{\mu }r=\frac{2N}{N-4\alpha }$ and $2(2^{*}_{\mu }-1)q^{\prime }=\frac{2N}{N-4\beta }$, it follows from the Sobolev imbedding theorem (see [11]) that

$$\begin{aligned} \Vert u\Vert _{2^{*}_{\mu }r}\le C\Vert A^{\alpha }u\Vert _{2},\ \text {for all}\ u\in D(A^{\alpha }) \end{aligned}$$

(2.17)

and

$$\begin{aligned} \Vert u\Vert _{2(2^{*}_{\mu }-1)q^{\prime }}\le C\Vert A^{\beta }u\Vert _{2},\ \text {for all}\ u\in D(A^{\beta }). \end{aligned}$$

(2.18)

Hence, by (2.16)–(2.18), we can derive that

$$\begin{aligned} \Vert f(u)-f(v)\Vert _{2}\le & {} {\hat{C}}\Vert A^{\alpha }u\Vert ^{2^{*}_{\mu }}_{2} \left( \Vert A^{\beta }u\Vert _{2}+\Vert A^{\beta }v\Vert _{2}\right) ^{2^{*}_{\mu }-2}\Vert A^{\beta }(u-v)\Vert _{2} \nonumber \\{} & {} +{\bar{C}}\Vert A^{\beta }v\Vert ^{2^{*}_{\mu }-1}_{2}\left( \Vert A^{\alpha }u\Vert _{2}+\Vert A^{\alpha }v\Vert _{2}\right) ^{2^{*}_{\mu }-1}\Vert A^{\alpha }(u-v)\Vert _{2}. \qquad \end{aligned}$$

(2.19)

Next, we apply the contraction mapping argument in the set

$$\begin{aligned} Y_{T,K}&:=\left\{ u\in BC((0,T];D(A^{\alpha })\cap D(A^{\beta }))\ ,\right. \\&\quad \left. \max \left\{ \sup _{t\in (0,T]}t^{\alpha -\frac{1}{2}}\Vert A^{\alpha }u(t)\Vert _{2}, \sup _{t\in (0,T]}t^{\beta -\frac{1}{2}}\Vert A^{\beta }u(t)\Vert _{2}\right\} \le K\right\} \end{aligned}$$

endowed with the norm

$$\begin{aligned} \Vert \vert u\Vert \vert _{Y_{T,K}}:=\max \left\{ \sup _{t\in (0,T]}t^{\alpha -\frac{1}{2}}\Vert A^{\alpha }u(t)\Vert _{2}, \sup _{t\in (0,T]}t^{\beta -\frac{1}{2}}\Vert A^{\beta }u(t)\Vert _{2}\right\} , \end{aligned}$$

$(Y_{T,K},\Vert \vert u\Vert \vert _{Y_{T,K}})$ is a Banach space.

Given $u\in Y_{T,K}$, we set

$$\begin{aligned} \Phi [u](t):=e^{-tA}u_{0}+\int _{0}^{t}e^{-(t-s)A}f(u(s))ds. \end{aligned}$$

(2.20)

We shall show that $\Phi [u]$ is a strict contraction map on $Y_{T,K}$. By (2.20), we have

$$\begin{aligned} \Vert A^{\gamma }\Phi [u](t)\Vert _{2}\le \Vert A^{\gamma } e^{-tA}u_{0}\Vert _{2}+\int _{0}^{t}\Vert A^{\gamma }e^{-(t-s)A} f(u(s))\Vert _{2}ds. \end{aligned}$$

(2.21)

By Lemma 2.8 and (2.19) with $v=0$, we can estimate as follows

$$\begin{aligned} \int _{0}^{t}\Vert A^{\gamma }e^{-(t-s)A} f(u(s))\Vert _{2}ds\le & {} C_{1}(\gamma )\int _{0}^{t}(t-s)^{-\gamma }e^{-\lambda (t-s)}\Vert f(u(s))\Vert _{2}ds \nonumber \\\le & {} C_{1}\int _{0}^{t}(t-s)^{-\gamma }\Vert A^{\alpha }u(s)\Vert ^{2^{*}_{\mu }}_{2}\Vert A^{\beta }u(s)\Vert ^{2^{*}_{\mu }-1}_{2}ds \nonumber \\\le & {} C_{1}\int _{0}^{t}(t-s)^{-\gamma }s^{-(2^{*}_{\mu }-1)(\beta -\frac{1}{2})-2^{*}_{\mu }(\alpha -\frac{1}{2})}\Vert \vert u(s)\Vert \vert _{Y_{T,K}}^{22^{*}_{\mu }-1}ds \nonumber \\\le & {} C_{2}t^{1-\gamma -(2^{*}_{\mu }-1)(\beta -\frac{1}{2})-2^{*}_{\mu }(\alpha -\frac{1}{2})}K^{22^{*}_{\mu }-1}, \end{aligned}$$

(2.22)

where $\gamma \in (0,1)$ and $C_{i}>0 (i=1,2)$ are some constants.

By (2.21)–(2.22) with $\gamma =\alpha$ and $\gamma =\beta$ respectively, we can derive that

$$\begin{aligned} \Vert A^{\alpha }\Phi [u](t)\Vert _{2} \le \Vert A^{\alpha } e^{-tA}u_{0}\Vert _{2}+C_{2}t^{1-\alpha -(2^{*}_{\mu }-1)(\beta -\frac{1}{2})-2^{*}_{\mu }(\alpha -\frac{1}{2})}K^{22^{*}_{\mu }-1} \end{aligned}$$

(2.23)

and

$$\begin{aligned} \Vert A^{\beta }\Phi [u](t)\Vert _{2}\le \Vert A^{\beta } e^{-tA}u_{0}\Vert _{2}+C_{2}t^{1-\beta -(2^{*}_{\mu }-1)(\beta -\frac{1}{2})-2^{*}_{\mu }(\alpha -\frac{1}{2})}K^{22^{*}_{\mu }-1}. \end{aligned}$$

(2.24)

Hence, by (2.23) and (2.24), one has

$$\begin{aligned} \Vert \vert \Phi [u]\Vert \vert _{Y_{T,K}}\le & {} {} \max \left\{ \sup _{t\in (0,T]}t^{\alpha -\frac{1}{2}}[\Vert A^{\alpha } e^{-tA}u_{0}\Vert _{2}\!+\!C_{2}t^{1-\alpha -(2^{*}_{\mu }-1)(\beta -\frac{1}{2})-2^{*}_{\mu }(\alpha -\frac{1}{2})}K^{22^{*}_{\mu }-1}],\right. \nonumber \\{}{} & {} {} \left. \sup _{t\in (0,T]}t^{\beta -\frac{1}{2}}[\Vert A^{\beta } e^{-tA}u_{0}\Vert _{2}+C_{2}t^{1-\beta -(2^{*}_{\mu }-1)(\beta -\frac{1}{2})-2^{*}_{\mu }(\alpha -\frac{1}{2})}K^{22^{*}_{\mu }-1}] \right\} \nonumber \\ {}\le & {} {} \max \left\{ \sup _{t\in (0,T]}[t^{\alpha -\frac{1}{2}}\Vert A^{\alpha } e^{-tA}u_{0}\Vert _{2},\sup _{t\in (0,T]}[t^{\beta -\frac{1}{2}}\Vert A^{\beta } e^{-tA}u_{0}\Vert _{2}\right\} \nonumber \\{}{} & {} {} +C_{3}t^{\frac{1}{2}-(2^{*}_{\mu }-1)(\beta -\frac{1}{2})-2^{*}_{\mu }(\alpha -\frac{1}{2})}K^{22^{*}_{\mu }-1}. \end{aligned}$$

(2.25)

Note that

$$\begin{aligned} \frac{1}{2}-(2^{*}_{\mu }-1)\left( \beta -\frac{1}{2}\right) -2^{*}_{\mu }\left( \alpha -\frac{1}{2}\right) =0, \end{aligned}$$

then, we can derive that $\Vert \vert \Phi [u]\Vert \vert _{Y_{T,K}}\le K$ provided that

$$\begin{aligned} C_{2}K^{22^{*}_{\mu }-1}<K \end{aligned}$$

(2.26)

and

$$\begin{aligned} \max \left\{ \sup _{t\in (0,T]}t^{\alpha -\frac{1}{2}}\Vert A^{\alpha } e^{-tA}u_{0}\Vert _{2},\sup _{t\in (0,T]}t^{\beta -\frac{1}{2}}\Vert A^{\beta } e^{-tA}u_{0}\Vert _{2}\right\} \le K-C_{2}K^{22^{*}_{\mu }-1},\qquad \quad \end{aligned}$$

(2.27)

which implies that $\Phi$ maps $Y_{T,K}$ into itself.

Next, we show that the mapping $\Phi : Y_{T,K}\rightarrow Y_{T,K}$ is a strictly contraction. For any $u,v\in Y_{T,K}$, it follows from (2.19) and Lemma 2.8 that

$$\begin{aligned}{} & {} {} \Vert A^{\gamma }\Phi [u](t)-A^{\gamma }\Phi [v](t)\Vert _{2}\\ {}{} & {} {} \quad \le \int _{0}^{t}\Vert A^{\gamma }e^{-(t-s)A} (f(u(s))-f(v(s)))\Vert _{2}ds\\{}{} & {} {} \quad \le C_{1}(\gamma )\int _{0}^{t}(t-s)^{-\gamma }e^{-\lambda (t-s)}\Vert (f(u(s))-f(v(s)))\Vert _{2}ds\\{}{} & {} {} \quad \le C_{2}\int _{0}^{t}(t-s)^{-\gamma }\left[ C\Vert A^{\alpha }u\Vert ^{2^{*}_{\mu }}_{2} \left( \Vert A^{\beta }u\Vert _{2}+\Vert A^{\beta }v\Vert _{2}\right) ^{2^{*}_{\mu }-2}\Vert A^{\beta }(u-v)\Vert _{2}\right. \\ {}{} & {} {} \qquad \left. +C\Vert A^{\beta }v\Vert ^{2^{*}_{\mu }-1}_{2}\left( \Vert A^{\alpha }u\Vert _{2}+\Vert A^{\alpha }v\Vert _{2}\right) ^{2^{*}_{\mu }-1}\Vert A^{\alpha }(u-v)\Vert _{2}\right] ds\\{}{} & {} {} \quad \le C_{3}\int _{0}^{t}(t-s)^{-\gamma }s^{-(2^{*}_{\mu }-1)(\beta -\frac{1}{2})-2^{*}_{\mu }(\alpha -\frac{1}{2})}\Vert \vert u\Vert \vert ^{2^{*}_{\mu }}_{Y_{K,T}}\\{}{} & {} {} \qquad (\Vert \vert u\Vert \vert _{Y_{K,T}}+\Vert \vert v\Vert \vert _{Y_{K,T}})^{2^{*}_{\mu }-2}\Vert \vert u-v\Vert \vert _{Y_{K,T}}ds\\{}{} & {} {} \qquad +C_{3}\int _{0}^{t}(t-s)^{-\gamma }s^{-(2^{*}_{\mu }-1)(\beta -\frac{1}{2})-2^{*}_{\mu }(\alpha -\frac{1}{2})}\Vert \vert u\Vert \vert ^{2^{*}_{\mu }-1}_{Y_{K,T}}\\{}{} & {} {} \qquad (\Vert \vert u\Vert \vert _{Y_{K,T}}+\Vert \vert v\Vert \vert _{Y_{K,T}})^{2^{*}_{\mu }-1}\Vert \vert u-v\Vert \vert _{Y_{K,T}}ds\\{}{} & {} {} \quad \le C_{4}t^{1-\gamma -(2^{*}_{\mu }-1)(\beta -\frac{1}{2})-2^{*}_{\mu }(\alpha -\frac{1}{2})}(2K)^{2(2^{*}_{\mu }-1)}\Vert \vert u-v\Vert \vert _{Y_{K,T}}. \end{aligned}$$

Furthermore, we have

$$\begin{aligned}{} & {} {} \Vert \vert \Phi [u](t)-\Phi [v](t)\Vert \vert _{Y_{K,T}}\\{}{} & {} {} \quad \le \max \left\{ t^{\alpha -\frac{1}{2}}C_{4}t^{1-\alpha -(2^{*}_{\mu }-1)(\beta -\frac{1}{2})-2^{*}_{\mu }(\alpha -\frac{1}{2})}(2K)^{2(2^{*}_{\mu }-1)}\Vert \vert u-v\Vert \vert _{Y_{K,T}}\right. \\{}{} & {} {} \qquad \left. t^{\beta -\frac{1}{2}}C_{4}t^{1-\beta -(2^{*}_{\mu }-1)(\beta -\frac{1}{2})-2^{*}_{\mu }(\alpha -\frac{1}{2})}(2K)^{2(2^{*}_{\mu }-1)}\Vert \vert u-v\Vert \vert _{Y_{K,T}}\right\} \\{}{} & {} {} \quad \le C_{5}t^{\frac{1}{2}-(2^{*}_{\mu }-1)(\beta -\frac{1}{2})-2^{*}_{\mu }(\alpha -\frac{1}{2})}(2K)^{2(2^{*}_{\mu }-1)}\Vert \vert u-v\Vert \vert _{Y_{K,T}}. \end{aligned}$$

Note that

$$\begin{aligned} \frac{1}{2}-(2^{*}_{\mu }-1)\left( \beta -\frac{1}{2}\right) -2^{*}_{\mu }\left( \alpha -\frac{1}{2}\right) =0, \end{aligned}$$

so, we obtain that

$$\begin{aligned} \Vert \vert \Phi [u](t)-\Phi [v](t)\Vert \vert _{Y_{K,T}}\le C_{5}(2K)^{2(2^{*}_{\mu }-2)}\Vert \vert u-v\Vert \vert _{Y_{K,T}}\le \frac{1}{2}\Vert \vert u-v\Vert \vert _{Y_{K,T}},\qquad \quad \end{aligned}$$

(2.28)

provided that

$$\begin{aligned} C_{5}(2K)^{2(2^{*}_{\mu }-2)}\le \frac{1}{2}, \end{aligned}$$

(2.29)

which implies that $\Phi : Y_{K,T}\rightarrow Y_{K,T}$ is a strictly contraction mapping.

Now, we prove that there exists $K,T>0$ such that (2.26)–(2.27) and (2.29) hold. Indeed, by taking $K>0$ small enough, we can derive that (2.26) and (2.29) hold. By Lemma 2.5(iii), since $\alpha ,\beta \in (\frac{1}{2},1)$, one has

$$\begin{aligned} t^{\alpha -\frac{1}{2}}\Vert A^{\alpha }e^{-tA}u\Vert _{2} =t^{\alpha -\frac{1}{2}}\Vert A^{\alpha -\frac{1}{2}}e^{-tA}A^{\frac{1}{2}}u\Vert _{2}\rightarrow 0 \end{aligned}$$

and

$$\begin{aligned} t^{\beta -\frac{1}{2}}\Vert A^{\beta }e^{-tA}u\Vert _{2} =t^{\beta -\frac{1}{2}}\Vert A^{\beta -\frac{1}{2}}e^{-tA}A^{\frac{1}{2}}u\Vert _{2}\rightarrow 0 \end{aligned}$$

for $u\in D(A^{\frac{1}{2}})$ as $t\rightarrow 0$. Hence, we can get there exists $T>0$ small such that (2.27) holds.

Therefore, by applying Banach’s fixed point theorem, we can show that there exist a unique fixed point u in $Y_{K,T}$ and u is a mild solution of (2.20). The remainder of proof is similar to [12, 14], so we omit it here. $\square$

At the end of this section, we introduce a lemma (see [20]), which plays an important role in the proof of blow-up.

Lemma 2.10

Suppose that $0<T\le \infty$ and a non-negative function $f(t)\in C^{2}[0,T)$ satisfying

$$\begin{aligned} f^{\prime \prime }f(t)-(1+\alpha )(f^{\prime }(t))^{2}\ge 0 \end{aligned}$$

for some $\alpha >0$. If $f(0)>0$ and $f^{\prime }(0)>0$, then

$$\begin{aligned} T\le \frac{f(0)}{\alpha f^{\prime }(0)}<+\infty , \end{aligned}$$

and $f(t)\rightarrow +\infty$ as $t\rightarrow T$.

3 Lower Energy Initial Value

In this section, we establish the global existence and finite time blow-up of solution with lower energy initial value. Moreover, we derive the regularity and decay estimate of global solutions and an upper bound of blow-up time for $J_{\mu }(u_{0})<0$.

3.1 Global Existence and Blow-up of Solution

In this subsection, we give the global existence and finite time blow-up of solutions.

Proof of Theorem 1.4

From Proposition 2.9, we can derive the local existence result for problem (P) in a more general case of initial value $u_{0}\in H^{1}_{0}(\Omega )$ and $u\in C([0,T];H^{1}_{0}(\Omega ))$.

Now, we need to prove that u(t) satisfies $J_{\mu }(u(t))<m_{\mu }$ and $I_{\mu }(u(t))>0$ for any $t>0$. On the contrary, from continuity about time, there exist $t_{0}$ such that $u(x,t_{0})\in \partial W$, that is $J_{\mu }(u(t_{0}))=m_{\mu }$ or $I_{\mu }(u(t_{0}))=0, \int _{\Omega }\vert \nabla u(t_{0})\vert ^{2}dx\not =0$. From (2.4), we easily know that $J_{\mu }(u(t_{0}))\not =m_{\mu }$. If $I_{\mu }(u(t_{0}))=0, \int _{\Omega }\vert \nabla u(t_{0})\vert ^{2}dx\not =0$, by Remark 1.3, we know that $J_{\mu }(u(t_{0}))\ge m_{\mu }$, a contradiction. Therefore, u(t) satisfies $I_{\mu }(u(t))>0$ for any $t>0$.

Furthermore, we have

$$\begin{aligned} I_{\mu }(u)=\int _{\Omega }|\nabla u|^{2}dx -\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy>0. \end{aligned}$$

(3.1)

Furthermore, by (2.4) and (3.1), we have

$$\begin{aligned} \int ^{t}_{0}\Vert u_{s}\Vert _{2}^{2}ds +\frac{N-\mu +2}{2(2N-\mu )}\Vert \nabla u\Vert ^{2}_{2} <m_{\mu }=\frac{N-\mu +2}{2(2N-\mu )}S^{\frac{2N-\mu }{N-\mu +2}}_{H,L}. \end{aligned}$$

Therefore, for any $T>0$,

$$\begin{aligned} \Vert \nabla u\Vert ^{2}_{2}<S^{\frac{2N-\mu }{N-\mu +2}}_{H,L} \end{aligned}$$

and

$$\begin{aligned} \Vert u^{\prime }(s)\Vert _{L^{2}(0,T;L^{2}(\Omega ))}<\frac{N-\mu +2}{2(2N-\mu )}S^{\frac{2N-\mu }{N-\mu +2}}_{H,L}, \end{aligned}$$

hold, which implies that u(x, t) is a global solution of problem (P).

Next, we employ the classical concavity method to prove finite time blow-up for problem (P) with $J_{\mu }(u_{0})<m_{\mu }$. The idea was inspired by Levine and Payne [22, 23] and Levine [19], by constructing an auxiliary function. Here, we need the following lemma.

Lemma 3.1

Let u(x, t) is the solution for problem (P) with $u_{0}\in V$ satisfies $J_{\mu }(u_{0})>0$. Then, there exist $\rho >0$ such that

$$\begin{aligned} \int _{\Omega }\int _{\Omega }\frac{|u(x,t)|^{2^{*}_{\mu }}|u(y,t)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy \ge (1+\rho )\int _{\Omega }|\nabla u(x,t)|^{2}dx, \end{aligned}$$

(3.2)

for $t\in [0,\infty )$.

Proof

Since $u_{0}\in V$, we have $u(x,t)\in V$ for all $t>0$. Indeed, on the contrary, from continuity about time, there exist $t_{0}$ such that $u(x,t_{0})\in \partial V$, that is $J_{\mu }(u(t_{0}))=m_{\mu }$ or $I_{\mu }(u(t_{0}))=0, \int _{\Omega }\vert \nabla u(t_{0})\vert ^{2}dx\not =0$. From (2.4), we easily know that $J_{\mu }(u(t_{0}))\not =m_{\mu }$. If $I_{\mu }(u(t_{0}))=0, \int _{\Omega }\vert \nabla u(t_{0})\vert ^{2}dx\not =0$, by Remark 1.3, we know that $J_{\mu }(u(t_{0}))\ge m_{\mu }$, a contradiction. Furthermore, we have $I_{\mu }(u)<0$. Therefore, by (1.7) and the Hardy–Littlewood–Sobolev inequality, we have

$$\begin{aligned} \frac{22^{*}_{\mu }}{2^{*}_{\mu }-1}m_{\mu }=S^{\frac{2N-\mu }{N-\mu +2}}_{H,L}\le & {} \left( \frac{\int _{\Omega }|\nabla u|^{2}dx}{\left( \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\right) ^{\frac{N-2}{2N-\mu }}} \right) ^{\frac{2N-\mu }{N-\mu +2}}\nonumber \\\le & {} \int _{\Omega }|\nabla u|^{2}dx. \end{aligned}$$

(3.3)

Let

$$\begin{aligned} \rho _{0}:=1-\frac{J_{\mu }(u_{0})}{m_{\mu }}, \end{aligned}$$

we have $\rho _{0}>0$ since $J_{\mu }(u_{0})<m_{\mu }$. Furthermore, by (1.2), (1.3) and (1.8), we have

$$\begin{aligned} I_{\mu }(u)= & {} -(2^{*}_{\mu }-1)\int _{\Omega }|\nabla u|^{2}dx +22^{*}_{\mu }J_{\mu }(u)\nonumber \\\le & {} -(2^{*}_{\mu }-1)\int _{\Omega }|\nabla u|^{2}dx +22^{*}_{\mu }J_{\mu }(u_{0})\nonumber \\= & {} -(2^{*}_{\mu }-1)\int _{\Omega }|\nabla u|^{2}dx +22^{*}_{\mu }(1-\rho _{0})m_{\mu }. \end{aligned}$$

(3.4)

Next, we claim that

$$\begin{aligned} (2^{*}_{\mu }-1)\int _{\Omega }|\nabla u|^{2}dx -22^{*}_{\mu }(1-\rho _{0})m_{\mu } \ge \rho \int _{\Omega }|\nabla u|^{2}dx. \end{aligned}$$

(3.5)

where $\rho :=\frac{(2^{*}_{\mu }-1)\rho _{0}}{2}$. Indeed, since, $\rho :=\frac{(2^{*}_{\mu }-1)\rho _{0}}{2}$, we have

$$\begin{aligned}{} & {} (2^{*}_{\mu }-1)\int _{\Omega }|\nabla u|^{2}dx -22^{*}_{\mu }(1-\rho _{0})m_{\mu } -\rho \int _{\Omega }|\nabla u|^{2}dx.\\{} & {} \quad =(2^{*}_{\mu }-1-\rho )\int _{\Omega }|\nabla u|^{2}dx -22^{*}_{\mu }(1-\rho _{0})m_{\mu }\\{} & {} \quad \ge [2^{*}_{\mu }-1-\rho -(2^{*}_{\mu }-1)(1-\rho _{0})]\int _{\Omega }|\nabla u|^{2}dx\ge 0 \end{aligned}$$

Therefore, the claim is hold.

Next, by (3.4) and (3.5), we have

$$\begin{aligned} -I_{\mu }(u)\ge \rho \int _{\Omega }|\nabla u|^{2}dx, \end{aligned}$$

which implies that (3.2) is true. Consequently, the proof is complete. $\square$

Proof of Theorem 1.5(Part of finite time blow-up). We shall complete the proof by considering two separate cases.

(i):

For the case $J_{\mu }(u_{0})\le 0$. Suppose that there existence a global weak solution u(t), i.e. $T_{\max }=+\infty$, and we define a auxiliary function

$$\begin{aligned} f(t)=\int ^{t}_{0}\int _{\Omega }u(s)^{2}dxds. \end{aligned}$$

(3.6)

By (2.2), we can derive

$$\begin{aligned} f^{\prime }(t)= & {} \int _{\Omega }|u(t)|^{2}dx \nonumber \\= & {} \int _{\Omega }u_{0}^{2}dx +2\int ^{t}_{0}\left( -\int _{\Omega }|\nabla u|^{2}dx +\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\right) ds, \qquad \quad \end{aligned}$$

(3.7)

and

$$\begin{aligned} f^{\prime \prime }(t)=-2\left( \int _{\Omega }|\nabla u|^{2}dx -\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\right) =-2I_{\mu }(u). \end{aligned}$$

(3.8)

Furthermore, it follows from (2.4) and (3.8) that

$$\begin{aligned} f^{\prime \prime }(t)= & {} -2\int _{\Omega }|\nabla u|^{2}dx +2\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\nonumber \\= & {} 2\left( 2^{*}_{\mu }-1\right) \int _{\Omega }|\nabla u|^{2}dx -42^{*}_{\mu }J_{\mu }(u_{0}) +42^{*}_{\mu }\int ^{t}_{0}\int _{\Omega }|u_{s}|^{2}dxds. \end{aligned}$$

(3.9)

Since $J_{\mu }(u_{0})\le 0$, we can know that

$$\begin{aligned} 2\left( 2^{*}_{\mu }-1\right) \int _{\Omega }|\nabla u|^{2}dx -42^{*}_{\mu }J_{\mu }(u_{0})>0. \end{aligned}$$

(3.10)

Furthermore, if $T_{\max }=+\infty$, by (3.9) and (3.10), we can derive that

$$\begin{aligned} \lim _{t\rightarrow \infty }f^{\prime }(t)=\infty \ \text {and}\ \lim _{t\rightarrow \infty }f(t)=\infty . \end{aligned}$$

We also have

$$\begin{aligned} f^{\prime \prime }(t)\ge 42^{*}_{\mu }\int ^{t}_{0}\int _{\Omega }|u_{s}|^{2}dxds. \end{aligned}$$

(3.11)

By (3.6) and (3.11), making use of the Schwartz inequality, we have

$$\begin{aligned} f(t)f^{\prime \prime }(t)\ge & {} {} 42^{*}_{\mu }\left( \int ^{t}_{{0}}\int _{\Omega }u(s)^{2}dxds\right) \left( \int ^{t}_{0}\int _{\Omega }|u_{s}(s)|^{2}dxds\right) \nonumber \\ {}\ge & {} {} 42^{*}_{\mu }\left( \int ^{t}_{0}\int _{\Omega }uu_{s}dxds\right) ^{2}\nonumber \\= & {} {} 42^{*}_{\mu }\int ^{t}_{0}\int _{\Omega }\left( -\int _{\Omega }|\nabla u|^{2}dx +\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy \right) dxdt\nonumber \\ {}= & {} {} 42^{*}_{\mu }\left( f^{\prime }(t)-f^{\prime }(0)\right) ^{2}. \end{aligned}$$

(3.12)

So by (3.12), as $t\rightarrow \infty$, there exist $\alpha >0$ such that

$$\begin{aligned} f(t)f^{\prime \prime }(t)\ge (1+\alpha )\left( f^{\prime }(t)\right) ^{2}. \end{aligned}$$

(3.13)

Then, by Lemma 2.10, there exists a $T>0$ such that $\lim _{t\rightarrow T^{-}}f(t)=+\infty$, which contradicts $T_{\max }=+\infty$.

(ii):

For the case $0<J_{\mu }(u_{0})<m_{\mu }$. Similar to case (i), suppose that there existence a global weak solution u(t), i.e. $T_{\max =\infty }$ and let

$$\begin{aligned} f(t)=\int ^{t}_{0}\int _{\Omega }\vert u(s)\vert ^{2}dxds. \end{aligned}$$

By Lemma 3.1, there exist $\rho >0$ such that

$$\begin{aligned} \int _{\Omega }\int _{\Omega }\frac{|u(x,t)|^{2^{*}_{\mu }}|u(y,t)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy \ge (1+\rho )\int _{\Omega }|\nabla u(x,t)|^{2}dx. \end{aligned}$$

(3.14)

for $t\in [0,\infty )$. Hence, by (3.8) and (3.14), we have

$$\begin{aligned} f^{\prime \prime }(t)= & {} -2\left( \int _{\Omega }|\nabla u|^{2}dx -\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\right) \nonumber \\\ge & {} 2\rho \int _{\Omega }|\nabla u|^{2}dx. \end{aligned}$$

(3.15)

If $T_{\max }=\infty$, by (3.15), we can derive that

$$\begin{aligned} \lim _{t\rightarrow \infty }f^{\prime }(t)=\infty \ \text {and}\ \lim _{t\rightarrow \infty }f(t)=\infty . \end{aligned}$$

(3.16)

Next, similar to (3.9), we also have

$$\begin{aligned} f^{\prime \prime }(t)\ge 2\left( 2^{*}_{\mu }-1\right) \int _{\Omega }|\nabla u|^{2}dx -42^{*}_{\mu }J_{\mu }(u_{0}) +42^{*}_{\mu }\int ^{t}_{0}\int _{\Omega }|u_{s}|^{2}dxds. \end{aligned}$$

(3.17)

By Lemma 3.1, we have $\int _{\Omega }|\nabla u|^{2}dx <\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy$ and since $J_{\mu }(u_{0})<m_{\mu }$, then, we can derive

$$\begin{aligned}{} & {} 2\left( 2^{*}_{\mu }-1\right) \int _{\Omega }|\nabla u|^{2}dx -42^{*}_{\mu }J_{\mu }(u_{0})\nonumber \\{} & {} \quad \ge 2\left( 2^{*}_{\mu }-1\right) \int _{\Omega }|\nabla u|^{2}dx -2\left( 2^{*}_{\mu }-1\right) S^{\frac{2N-\mu }{N-\mu +2}}_{H,L} \nonumber \\{} & {} \quad >2\left( 2^{*}_{\mu }-1\right) \left[ \int _{\Omega }|\nabla u|^{2}dx-\left( \frac{\int _{\Omega }|\nabla u|^{2}dx}{\left( \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\right) ^{\frac{N-2}{2N-\mu }}} \right) ^{^{\frac{2N-\mu }{N-\mu +2}}}\right] \ge 0. \nonumber \\ \end{aligned}$$

(3.18)

Hence, by (3.17) and (3.18), one has

$$\begin{aligned} f^{\prime \prime }(t)\ge 22^{*}_{\mu }\int ^{t}_{t_{0}}\int _{\Omega }|u_{s}|^{2}dxds. \end{aligned}$$

Similar to (3.12), using the Schwartz inequality, we have

$$\begin{aligned} f(t)f^{\prime \prime }(t)\ge 2^{*}_{\mu }\left( f^{\prime }(t)-f^{\prime }(0)\right) ^{2}. \end{aligned}$$

(3.19)

Therefore, we can derive a contraction as $J_{\mu }(u_{0})\le 0$. We complete the proof.

Remark 3.2

In Theorem 1.5, we can prove a more general result that if there exists $t_{0}$ such that $u(t_{0})$ satisfies $J_{\mu }(u(t_{0}))\le 0$ or $0<J_{\mu }(u(t_{0}))\le m_{\mu }$ and $I_{\mu }(u(t_{0}))>0$, then, the weak solution u(x, t) of problem (P) blow-up in finite time. In fact, we only need to substitute initial time $t=t_{0}$ for $t=0$ in above proof.

Next, we give a different proof of Theorem 1.5, by using a modified potential well. Firstly, we give the following lemmas.

Lemma 3.3

Assume that $u\in H^{1}_{0}(\Omega )$ satisfying $0<J_{\mu }(u)<m_{\mu }$. Then, the sign of $I_{\mu ,\delta }(u)$ does not change for $\delta _{1}<\delta <\delta _{2}$, where $\delta _{1}<1<\delta _{2}$ be the two roots of $m_{\mu }(\delta )=J_{\mu }(u)$.

Proof

Obviously, $J_{\mu }(u)>0$ implies that $\Vert \nabla u\Vert ^{2}_{2}\ne 0$. On the contrary, if the sign of $I_{\mu ,\delta }(u)$ is changeable for $\delta _{1}<\delta <\delta _{2}$, then there exist a ${\bar{\delta }}\in (\delta _{1},\delta _{2})$ such that $I_{\mu ,{\bar{\delta }}}(u)=0$. Therefore, we can get $J_{\mu }(u)\ge m_{\mu }({\bar{\delta }})$. On the other hand, by Lemma 2.7(iii), we have $J_{\mu }(u)=m_{\mu }(\delta _{1})=m_{\mu }(\delta _{2})<m_{\mu }({\bar{\delta }})$, a contradiction. $\square$

Proposition 3.4

Assume that $u\in H^{1}_{0}(\Omega )$ and $0<\sigma <m_{\mu }$. Let $\delta _{1}$ and $\delta _{2}$ with $\delta _{1}<\delta _{2}$ be the two roots of $m_{\mu }(\delta )=\sigma$. Then

• (i) If $I_{\mu }(u_{0})>0$, then, all weak solutions u(x, t) of the problem (P) with $0<J_{\mu }(u_{0})\le \sigma$ belongs to $W_{\delta }$ for $\delta _{1}<\delta <\delta _{2}$, $0\le t<T$;

• (ii) If $I_{\mu }(u_{0})<0$, then, all weak solutions u(x, t) of the problem (P) with $0<J_{\mu }(u_{0})\le \sigma$ belongs to $V_{\delta }$ for $\delta _{1}<\delta <\delta _{2}$, $0\le t<T$.

Proof

(i):

Let u(x, t) be any weak solution of problem (P) with $I_{\mu }(u_{0})>0$ and $0<J_{\mu }(u_{0})\le \sigma <m_{\mu }$. It follows from Lemma 2.7(iii) that $\delta _{1}<1<\delta _{2}$. Furthermore, by Lemma 3.3 and $I_{\mu }(u_{0})>0$, we can get $I_{\mu ,\delta }(u_{0})>0$ for $\delta _{1}<\delta <\delta _{2}$. Hence, $u_{0}\in W_{\delta }$ for all $\delta _{1}<\delta <\delta _{2}$. Next, we prove $u(t)\in W_{\delta }$ for all $\delta _{1}<\delta <\delta _{2}$ and $0<t<T$. Otherwise, there exist a $t^{**}\in (0,T)$ and a $\delta ^{*}\in (\delta _{1},\delta _{2})$ such that $u(t^{**})\in \partial W_{\delta ^{*}}$. Thus, either $I_{\mu ,\delta ^{*}}(u(t^{**}))=0$, $\Vert \nabla u(t^{**})\Vert _{2}\ne 0$ or $J_{\mu }(u(t^{**}))=m_{\mu }(\delta ^{*})$. From (2.4), it follows that

$$\begin{aligned} \int ^{t}_{0}\int _{\Omega }|u_{s}|^{2}dxds+J_{\mu }(u(t)) =J_{\mu }(u_{0})<m_{\mu }(\delta ),\ \delta _{1}<\delta<\delta _{2},\ 0<t<T, \qquad \quad \end{aligned}$$

(3.20)

which implies that $J_{\mu }(u(t^{**}))\ne m_{\mu }(\delta ^{*})$. If $I_{\mu ,\delta ^{*}}(u(t^{**}))=0$, $\Vert \nabla u(t^{**})\Vert _{2}\ne 0$, then by the definition of $m_{\mu }(\delta )$, we have $J_{\mu }(u(t^{**}))\ge m_{\mu }(\delta ^{*})$, which contradicts (3.20).

(ii):

Let u(x, t) be any weak solution of the problem (P) with $I_{\mu }(u_{0})<0$ and $0<J_{\mu }(u_{0})\le \sigma <m_{\mu }$. Similar to the argument of the proof of (i), by Lemma 2.7(iii), Lemma 3.3 and $I_{\mu }(u_{0})<0$, we can get $I_{\mu ,\delta }(u_{0})<0$ for $\delta _{1}<\delta <\delta _{2}$. Hence, $u_{0}\in V_{\delta }$ for all $\delta _{1}<\delta <\delta _{2}$. Next, we prove $u(t)\in V_{\delta }$ for all $\delta _{1}<\delta <\delta _{2}$ and $0<t<T$. Otherwise, there exist a $t^{**}\in (0,T)$ and a $\delta ^{*}\in (\delta _{1},\delta _{2})$ such that $u(t^{**})\in \partial V_{\delta ^{*}}$. Thus, either $I_{\mu ,\delta ^{*}}(u(t^{**}))=0$ or $J_{\mu }(u(t^{**}))=m_{\mu }(\delta ^{*})$. By (3.20), we can get $J_{\mu }(u(t^{**}))\ne m_{\mu }(\delta ^{*})$, hence $I_{\mu ,\delta ^{*}}(u(t^{**}))=0$. We assume that $t^{**}$ is the first time such that $I_{\mu ,\delta ^{*}}(u(t))=0$, then $I_{\mu ,\delta ^{*}}(u(t))<0$ for $0\le t<t^{**}$. By Lemma 2.6 (ii), we have $\Vert \nabla u\Vert ^{2}_{2}>r(\delta ^{*})$ for $0\le t<t^{**}$. Hence, $\Vert \nabla u(t^{**})\Vert ^{2}_{2}>r(\delta ^{*})$, which implies that $u(t^{**})\in {\mathcal {N}}_{\delta ^{*}}$ provided that $I_{\mu ,\delta ^{*}}(u_{t^{**}})=0$. By the definition of $m_{\mu }(\delta ^{*})$, we can also obtain $J_{\mu }(u(t^{**}))\ge m_{\mu }(\delta ^{*})$, a contradiction to (3.20). Consequently, the proof is complete.$\square$

Proof of Theorem 1.5

Suppose that there existence a global weak solution u(t), i.e. $T_{\max }=+\infty$, we define a auxiliary function

$$\begin{aligned} f(t)=\int ^{t}_{0}\int _{\Omega }\vert u(s)\vert ^{2}dxds. \end{aligned}$$

(3.21)

By (2.2) and (3.21), we have

$$\begin{aligned} f^{\prime }(t)= & {} \int _{\Omega }|u(t)|^{2}dx \nonumber \\= & {} \int _{\Omega }u_{0}^{2}dx +2\int ^{t}_{0}\left( -\int _{\Omega }|\nabla u|^{2}dx +\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\right) ds, \qquad \quad \end{aligned}$$

(3.22)

and

$$\begin{aligned} f^{\prime \prime }(t)=-2\left( \int _{\Omega }|\nabla u|^{2}dx -\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\right) =-2I_{\mu }(u). \end{aligned}$$

(3.23)

By (2.4) and (3.23), we have

$$\begin{aligned} f^{\prime \prime }(t)\ge & {} 2\left( 2^{*}_{\mu }-1\right) \int _{\Omega }|\nabla u|^{2}dx -42^{*}_{\mu }J_{\mu }(u_{0}) +42^{*}_{\mu }\int ^{t}_{0}\int _{\Omega }|u_{s}|^{2}dxds \nonumber \\\ge & {} 2\left( 2^{*}_{\mu }-1\right) \lambda _{1}f^{\prime }(t) -42^{*}_{\mu }J_{\mu }(u_{0}) +42^{*}_{\mu }\int ^{t}_{0}\int _{\Omega }|u_{s}|^{2}dxds, \end{aligned}$$

(3.24)

where $\lambda _{1}$ is the first eigenvalue of problem $\Delta \varphi +\lambda \varphi =0, \varphi |_{\partial \Omega }=0$.

Note that

$$\begin{aligned} \left( \int ^{t}_{0}\int _{\Omega }u_{s}udxds\right) ^{2}= & {} \left( \frac{1}{2}\int ^{t}_{0}\frac{d}{ds}\int _{\Omega }|u|^{2}dxds\right) ^{2}\\= & {} \frac{1}{4}\left( \left( \int _{\Omega }|u|^{2}dx\right) ^{2}-2\int _{\Omega }|u|^{2}dx\int _{\Omega }|u_{0}|^{2}dx +\left( \int _{\Omega }|u_{0}|^{2}dx\right) ^{2}\right) \\= & {} \frac{1}{4}\left( \left( f^{\prime }(t)\right) ^{2}-2f^{\prime }(t)\Vert u_{0}\Vert _{2}^{2} +\Vert u_{0}\Vert _{2}^{4}\right) \end{aligned}$$

then, we can get

$$\begin{aligned} \left( f^{\prime }(t)\right) ^{2}=4\left( \int ^{t}_{0}\int _{\Omega }u_{s}udxds\right) ^{2} +2f^{\prime }(t)\Vert u_{0}\Vert _{2}^{2} -\Vert u_{0}\Vert _{2}^{4}. \end{aligned}$$

(3.25)

Furthermore, by (3.24)–(3.25), and making use of the Schwartz inequality, we have

$$\begin{aligned}{} & {} f^{\prime \prime }(t)f(t)-2^{*}_{\mu }\left( f^{\prime }(t)\right) ^{2} \nonumber \\{} & {} \quad \ge 42^{*}_{\mu }\left( \int ^{t}_{0}\int _{\Omega }|u_{s}|^{2}dxds\int ^{t}_{0}\int _{\Omega }u(s)^{2}dxds -\left( \int ^{t}_{0}\int _{\Omega }u_{s}udxds\right) ^{2}\right) \nonumber \\{} & {} \qquad +2\left( 2^{*}_{\mu }-1\right) \lambda _{1}f^{\prime }(t)f(t) -22^{*}_{\mu }f^{\prime }(t)\Vert u_{0}\Vert _{2}^{2} -42^{*}_{\mu }J_{\mu }(u_{0})f(t) \nonumber \\{} & {} \quad \ge 2\left( 2^{*}_{\mu }-1\right) \lambda _{1}f^{\prime }(t)f(t) -22^{*}_{\mu }f^{\prime }(t)\Vert u_{0}\Vert _{2}^{2} -42^{*}_{\mu }J_{\mu }(u_{0})f(t). \end{aligned}$$

(3.26)

In the following we shall complete the proof by considering two separate cases.

(i):

If $J_{\mu }(u_{0})\le 0$, then

$$\begin{aligned} f^{\prime \prime }(t)f(t)-2^{*}_{\mu }\left( f^{\prime }(t)\right) ^{2} \ge 2\left( 2^{*}_{\mu }-1\right) \lambda _{1}f^{\prime }(t)f(t) -22^{*}_{\mu }f^{\prime }(t)\Vert u_{0}\Vert _{2}^{2}, \end{aligned}$$

(3.27)

for all $t>0$. Now, we claim that $I_{\mu }(u)<0$ for all $t>0$. Otherwise, there exist $t_{0}>0$ such that $I_{\mu }(u(t_{0}))=0$ and $I_{\mu }(u(t))<0$ for $0\le t<t_{0}$. Then, by Remark 1.3, we have $J_{\mu }(u(t_{0}))\ge m_{\mu }$, which contradicts (2.4). Hence, by (3.23), we can get $f^{\prime \prime }(t)>0$ for $t\ge 0$. And since $f^{\prime }(0)=\int _{\Omega }|u_{0}|^{2}dx\ge 0$, then there exists a $t_{0}\ge 0$ such that $f^{\prime }(t_{0})>0$. For $t\ge t_{0}$, we derive that

$$\begin{aligned} f(t)\ge f^{\prime }(t_{0})(t-t_{0})>f^{\prime }(0)(t-t_{0}). \end{aligned}$$

Therefore, for t large enough, we can get

$$\begin{aligned} \left( 2^{*}_{\mu }-1\right) \lambda _{1}f(t) >2^{*}_{\mu }\Vert u_{0}\Vert _{2}^{2}. \end{aligned}$$

Furthermore, by (3.27), we have

$$\begin{aligned} f^{\prime \prime }(t)f(t)-2^{*}_{\mu }\left( f^{\prime }(t)\right) ^{2} >0. \end{aligned}$$

(ii):

If $0<J_{\mu }(u_{0})<m_{\mu }$, then it follows from Proposition 3.4 that $u(t)\in V_{\delta }$ for $1<\delta <\delta _{2}$ and $t\ge 0$, where $\delta _{2}$ is the large root of $m_{\mu }(\delta )=J_{\mu }(u_{0})$. Hence, $I_{\mu ,\delta }(u)<0$ for $1<\delta <\delta _{2}$ and $t\ge 0$. Furthermore, by Lemma 2.6 (ii), we have $\Vert \nabla u\Vert ^{2}_{2}>r(\delta )$ for $1<\delta <\delta _{2}$ and $t\ge 0$. Hence, $I_{\mu ,\delta _{2}}(u)\le 0$ and $\Vert \nabla u\Vert ^{2}_{2}\ge r(\delta _{2})$ for $t\ge 0$. By (3.23), we can get

$$\begin{aligned} f^{\prime \prime }(t)=-2I_{\mu }(u)= & {} 2(\delta _{2}-1)\Vert \nabla u\Vert ^{2}_{2}-2I_{\mu ,\delta _{2}}(u)\\\ge & {} 2(\delta _{2}-1)r(\delta _{2}),\ t\ge 0. \end{aligned}$$

Furthermore, we have

$$\begin{aligned} f^{\prime }(t)\ge (\delta _{2}-1)r(\delta _{2})t+f^{\prime }(0) \ge (\delta _{2}-1)r(\delta _{2})t,\ t\ge 0, \end{aligned}$$

and

$$\begin{aligned} f(t)\ge \frac{1}{2}(\delta _{2}-1)r(\delta _{2})t^{2},\ t\ge 0. \end{aligned}$$

Therefore, for t large enough, we deduce that

$$\begin{aligned} (2^{*}_{\mu }-1)\lambda _{1}f(t)>22^{*}_{\mu }\Vert u_{0}\Vert _{2}^{2}\ \text {and}\ (2^{*}_{\mu }-1)\lambda _{1}f^{\prime }(t) >42^{*}_{\mu }J(u_{0}). \end{aligned}$$

Then, from (3.26) it follows that

$$\begin{aligned} f^{\prime \prime }(t)f(t)-2^{*}_{\mu }\left( f^{\prime }(t)\right) ^{2}\ge & {} 2\left( 2^{*}_{\mu }-1\right) \lambda _{1}f^{\prime }(t)f(t) -22^{*}_{\mu }f^{\prime }(t)\Vert u_{0}\Vert _{2}^{2}\\{} & {} -42^{*}_{\mu }J_{\mu }(u_{0})f(t)\\= & {} \left( (2^{*}_{\mu }-1)\lambda _{1}f(t) -22^{*}_{\mu }\Vert u_{0}\Vert _{2}^{2}\right) f^{\prime }(t)\\{} & {} +\left( (2^{*}_{\mu }-1)\lambda _{1}f(t) -42^{*}_{\mu }J(u_{0})\right) f(t)\\> & {} 0. \end{aligned}$$

Then, by Lemma 2.10, there exists a $T>0$ such that $\lim _{t\rightarrow T^{-}}f(t)=+\infty$, which contradicts $T_{\max }=+\infty$.

Proof of Theorem 1.5(Upper bound estimate of blow-up time). Next, we prove an upper bound for blow-up of $J_{\mu }(u_{0})<0$. Define $g(t)=\int _{\Omega }\vert u\vert ^{2}dx$, by (2.2), we have

$$\begin{aligned} g^{\prime }(t)=2\int _{\Omega }uu_{t}dx= & {} -2\int _{\Omega }|\nabla u|^{2}dx +2\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\nonumber \\\ge & {} -22^{*}_{\mu }\int _{\Omega }|\nabla u|^{2}dx +2\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\nonumber \\= & {} h(t), \end{aligned}$$

(3.28)

where

$$\begin{aligned} h(t):=-22^{*}_{\mu }\int _{\Omega }|\nabla u|^{2}dx +2\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy=-42^{*}_{\mu }J_{\mu }(u). \end{aligned}$$

By (2.3) and standard computation, we have

$$\begin{aligned} h^{\prime }(t)= & {} -42^{*}_{\mu }\int _{\Omega }\nabla u\nabla u_{t}dx +42^{*}_{\mu }\int _{\Omega }\int _{\Omega }\frac{|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}|u(x)|^{2^{*}_{\mu }-2}u(x)u_{t}dxdy\nonumber \\= & {} 42^{*}_{\mu }\int _{\Omega }\vert u_{t}\vert ^{2}dx>0, \end{aligned}$$

(3.29)

which implies that $h(t)\ge h(0)=-42^{*}_{\mu }J_{\mu }(u_{0})>0$ for all $t\ge 0$ provided that $J_{\mu }(u_{0})<0$.

Next, by (3.28) and Schwarz’s inequality, we obtain

$$\begin{aligned} g(t)h^{\prime }(t)= & {} 42^{*}_{\mu }\int _{\Omega }\vert u_{t}\vert ^{2}dx\int _{\Omega }\vert u\vert ^{2}dx\nonumber \\\ge & {} 42^{*}_{\mu }\left( \int _{\Omega }uu_{t}\right) ^{2} =2^{*}_{\mu }(g^{\prime }(t))^{2}\ge 2^{*}_{\mu } g^{\prime }(t)h(t). \end{aligned}$$

(3.30)

Integrating (3.30) from 0 to t and by (3.28), we have

$$\begin{aligned} \frac{g^{\prime }(t)}{(g(t))^{2^{*}_{\mu }}} \ge \frac{h(0)}{(g(0))^{2^{*}_{\mu }}} =\frac{-42^{*}_{\mu }J_{\mu }(u_{0})}{\Vert u_{0}\Vert ^{22^{*}_{\mu }}_{2}}. \end{aligned}$$

Integrating from 0 to t again, we can derive

$$\begin{aligned} \frac{1}{(g(t))^{2^{*}_{\mu }-1}}\le \frac{1}{(g(0))^{2^{*}_{\mu }-1}} -(2^{*}_{\mu }-1)\frac{-42^{*}_{\mu }J_{\mu }(u_{0})}{\Vert u_{0}\Vert ^{22^{*}_{\mu }}_{2}}t. \end{aligned}$$

Then, let t tends to T, one has

$$\begin{aligned} T<\frac{\Vert u_{0}\Vert ^{2}_{2}}{-42^{*}_{\mu }(2^{*}_{\mu }-1)J_{\mu }(u_{0})}. \end{aligned}$$

Consequently, the proof is complete.

Next, by (1.9) and the Poincaré inequality $\lambda _{1}\Vert u\Vert ^{2}_{2}\le \Vert \nabla u\Vert ^{2}_{2}$, we further have the following corollary.

Corollary 3.5

Under the conditions of Theorem 1.5, we also have

$$\begin{aligned} \lim _{t\rightarrow T^{-}}\Vert \nabla u(t)\Vert _{2}=+\infty . \end{aligned}$$

3.2 Decay Estimate of Global Solutions

In this section, we prove decay rate of the $H^{1}_{0}$ and $L^{2}$ norm of the global solutions for problem (P). Firstly, we give the following lemma.

Lemma 3.6

Let u(x, t) is the solution for problem (P) with $u_{0}\in H^{1}_{0}(\Omega )$ satisfies $J_{\mu }(u_{0})<m_{\mu }$ and $I_{\mu }(u_{0})>0$. Then

$$\begin{aligned} \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy \le (1-\kappa )\int _{\Omega }|\nabla u|^{2}dx, \end{aligned}$$

(3.31)

for $t\in [0,\infty )$, where $\kappa \in (0,1)$.

Proof

Since $u_{0}\in H^{1}_{0}(\Omega )$ satisfies $J_{\mu }(u_{0})<m_{\mu }$ and $I_{\mu }(u_{0})>0$, as in the proof of Theorem 1.4, we have $I_{\mu }(u(t))>0$ for all $t>0$. Furthermore, we can get

$$\begin{aligned} J_{\mu }(u)= & {} \frac{1}{2}\int _{\Omega }|\nabla u|^{2}dx -\frac{1}{22^{*}_{\mu }} \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\nonumber \\\ge & {} \frac{N-\mu +2}{2(2N-\mu )}\int _{\Omega }|\nabla u|^{2}dx. \end{aligned}$$

(3.32)

By (2.4), (3.32) and the Hardy–Littlewood–Sobolev inequality

$$\begin{aligned} \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy <S^{-\frac{2N-\mu }{N-2}}_{H,L}\left( \int _{\Omega }|\nabla u|^{2}dx\right) ^{\frac{2N-\mu }{N-2}}, \end{aligned}$$

we can derive

$$\begin{aligned} \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\le & {} S^{-\frac{2N-\mu }{N-2}}_{H,L}\left( \frac{2(2N-\mu )}{N-\mu +2}J_{\mu }(u)\right) ^{\frac{N-\mu +2}{N-2}} \int _{\Omega }|\nabla u|^{2}dx\\\le & {} S^{-\frac{2N-\mu }{N-2}}_{H,L}\left( \frac{2(2N-\mu )}{N-\mu +2}J_{\mu }(u_{0})\right) ^{\frac{N-\mu +2}{N-2}} \int _{\Omega }|\nabla u|^{2}dx \end{aligned}$$

Let $\kappa :=1-S^{-\frac{2N-\mu }{N-2}}_{H,L}\left( \frac{2(2N-\mu )}{N-\mu +2}J_{\mu }(u_{0})\right) ^{\frac{N-\mu +2}{N-2}}$, then we complete the proof of (3.31).

Next, since

$$\begin{aligned} J_{\mu }(u_{0})<m_{\mu }=\frac{N-\mu +2}{2(2N-\mu )}S^{\frac{2N-\mu }{N-\mu +2}}_{H,L}, \end{aligned}$$

we can derive that $\kappa \in (0,1)$. Consequently, the proof is complete. $\square$

Proof of Theorem 1.6

Under the condition in Theorem 1.4, let u be a global solution. By Lemma 3.6, we have

$$\begin{aligned} \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy \le (1-\kappa )\int _{\Omega }|\nabla u|^{2}dx, \end{aligned}$$

(3.33)

and so, we ca derive

$$\begin{aligned} I_{\mu }(u)\ge \kappa \int _{\Omega }|\nabla u|^{2}dx>0. \end{aligned}$$

(3.34)

Furthermore, by (1.2), (1.8) and (3.34), we have

$$\begin{aligned} J_{\mu }(u(t))= & {} {} \frac{1}{2}\int _{\Omega }|\nabla u(t)|^{2}dx -\frac{1}{22^{*}_{\mu }}\int _{\Omega }\int _{\Omega }\frac{|u(x,t)|^{2^{*}_{\mu }}|u(y,t)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy \nonumber \\ {}= & {} {} \frac{2^{*}_{\mu }-1}{22^{*}_{\mu }}\int _{\Omega }|\nabla u(t)|^{2}dx+\frac{1}{22^{*}_{\mu }}I_{\mu }(u(t))\nonumber \\ {}\ge & {} {} \frac{2^{*}_{\mu }-1}{22^{*}_{\mu }}\int _{\Omega }|\nabla u(t)|^{2}dx. \end{aligned}$$

(3.35)

By (3.34) and (3.35), we can also derive

$$\begin{aligned} J_{\mu }(u(t))\le \left( \frac{2^{*}_{\mu }-1}{22^{*}_{\mu }\kappa }+\frac{1}{22^{*}_{\mu }}\right) I_{\mu }(u(t)). \end{aligned}$$

(3.36)

Let $T>0$ be an arbitrary number but fixed, by (2.2) and Poincare’s inequality, we have

$$\begin{aligned} \int ^{T}_{t}I_{\mu }(u(s))ds= & {} -\frac{1}{2}\int _{\Omega }|u(T)|^{2}dx+\frac{1}{2}\int _{\Omega }|u(t)|^{2}dx \nonumber \\\le & {} \frac{1}{2}\int _{\Omega }|u(t)|^{2}dx\le \frac{1}{2\lambda _{1}}\int _{\Omega }|\nabla u(t)|^{2}dx, \end{aligned}$$

(3.37)

where $\lambda _{1}$ is the first eigenvalue of $-\Delta$ with homogeneous Dirichlet boundary condition.

It follows from (3.35) and (3.37) that

$$\begin{aligned} \int ^{T}_{t}I_{\mu }(u(s))ds\le \frac{2^{*}_{\mu }}{\lambda _{1}(2^{*}_{\mu }-1)}J_{\mu }(u(t)),\ \text {for}\ 0\le t\le T. \end{aligned}$$

(3.38)

Therefore, by (3.36) and (3.38), we can derive

$$\begin{aligned} \int ^{T}_{t}J_{\mu }(u(s))ds\le \left( \frac{2^{*}_{\mu }-1}{22^{*}_{\mu }\kappa }+\frac{1}{22^{*}_{\mu }}\right) \frac{2^{*}_{\mu }}{\lambda _{1}(2^{*}_{\mu }-1)}J_{\mu }(u(t)),\ \text{ for }\ 0\le t\le T. \end{aligned}$$

Since the arbitrariness of $T>0$, we can have

$$\begin{aligned} \int ^{\infty }_{t}J_{\mu }(u(s))ds\le {\bar{C}}J_{\mu }(u(t)), \end{aligned}$$

where ${\bar{C}}:=\left( \frac{2^{*}_{\mu }-1}{22^{*}_{\mu }\kappa }+\frac{1}{22^{*}_{\mu }}\right) \frac{2^{*}_{\mu }}{\lambda _{1}(2^{*}_{\mu }-1)}$.

Next,taking $T_{0}>0$ large enough such that ${\bar{C}}\le T_{0}$, then we have

$$\begin{aligned} \int ^{\infty }_{t}J_{\mu }(u(s))ds\le T_{0}J_{\mu }(u(t))\ \text {for}\ t\ge 0. \end{aligned}$$

(3.39)

Let

$$\begin{aligned} F(t)\equiv \int ^{\infty }_{t}J_{\mu }(u(s))ds. \end{aligned}$$

Then, $F^{\prime }(t)=-J_{\mu }(u(t))$. By (3.35), we have $J_{\mu }(u(t))>0$ for $t\ge 0$. Integrating (3.39) from $T_{0}$ to t, we have

$$\begin{aligned} F(t)\le F(T_{0})e^{1-\frac{t}{T_{0}}}, \end{aligned}$$

for all $t>T_{0}$. That is

$$\begin{aligned} \int ^{\infty }_{t}J_{\mu }(u(s))ds\le \int ^{\infty }_{T_{0}}J_{\mu }(u(s))dse^{1-\frac{t}{T_{0}}}, \ \text {}\ t>T_{0}. \end{aligned}$$

(3.40)

Next, by (1.3) and (3.39), for $t>T_{0}$, we have

$$\begin{aligned} \int ^{\infty }_{T_{0}}J_{\mu }(u(s))ds \le T_{0}J_{\mu }(u(T_{0})) \le T_{0}J_{\mu }(u_{0}). \end{aligned}$$

(3.41)

Therefore, by (3.40) and (3.41), we can derive

$$\begin{aligned} \int ^{\infty }_{t}J_{\mu }(u(s))ds\le T_{0}J_{\mu }(u_{0})e^{1-\frac{t}{T_{0}}}, \end{aligned}$$

(3.42)

for all $t>T_{0}$.

On the other hand, using (1.3) again, we obtain

$$\begin{aligned} \int ^{\infty }_{t}J_{\mu }(u(s))ds \ge \int ^{T_{0}+t}_{t}J_{\mu }(u(s))ds \ge T_{0}J_{\mu }(u(T_{0}+t)). \end{aligned}$$

(3.43)

It follows from (3.42) and (3.43) that

$$\begin{aligned} J_{\mu }(u(T_{0}+t))\le J_{\mu }(u_{0})e^{1-\frac{t}{T_{0}}}\ \text {for all}\ t>T_{0}. \end{aligned}$$

Furthermore, by (3.35), we can get

$$\begin{aligned} \int _{\Omega }|\nabla u(T_{0}+t)|^{2}dx\le \frac{22^{*}_{\mu }}{2^{*}_{\mu }-1}J_{\mu }(u(T_{0}+t)) \le \frac{22^{*}_{\mu }}{2^{*}_{\mu }-1}J_{\mu }(u_{0})e^{1-\frac{t}{T_{0}}}, \end{aligned}$$

which implies the decay of global solution $\Vert \nabla u(t)\Vert ^{2}_{2}\le Ce^{-\frac{t}{T_{0}}}$ for some $C>0$ and $t>T_{0}$ large enough. So, we complete the proof of (1.10).

Next, multiplying (2.1) by any $d(t)\in [0,\infty )$, we can get

$$\begin{aligned} (u_{t},d(t)v)+(\nabla u,\nabla (d(t)v))= & {} \left( \left( |x|^{-\mu }*|u|^{2^{*}_{\mu }}\right) |u|^{2^{*}_{\mu }-2}u,d(t)v\right) ,\ \forall v\in H^{1}_{0}(\Omega ),\\{} & {} \quad \forall d(t)\in C[0,\infty ), \end{aligned}$$

and

$$\begin{aligned} (u_{t},w)+(\nabla u,\nabla w)=\left( \left( |x|^{-\mu }*|u|^{2^{*}_{\mu }}\right) |u|^{2^{*}_{\mu }-2}u,w\right) ,\ \forall w\in L^{\infty }(0,\infty ;H^{1}_{0}(\Omega )). \nonumber \\ \end{aligned}$$

(3.44)

Letting $w=u$, by (3.44), one has

$$\begin{aligned} \frac{1}{2}\frac{d}{dt}\int _{\Omega }u^{2}dx+\int _{\Omega }|\nabla u|^{2}dx =\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy. \end{aligned}$$

(3.45)

It follows from Lemma 3.6 and (3.45) that

$$\begin{aligned} \frac{d}{dt}\int _{\Omega }u^{2}dx<-2\kappa \int _{\Omega }|\nabla u|^{2}dx \le -\frac{2\kappa }{\lambda _{1}}\int _{\Omega }|u|^{2}dx, \end{aligned}$$

(3.46)

where $\lambda _{1}$ is the first eigenvalue of $-\Delta$ with homogeneous Dirichlet boundary condition.

Integrating (3.46) on (0, t), we obtain

$$\begin{aligned} \Vert u(t)\Vert ^{2}_{2}\le \Vert u_{0}\Vert ^{2}_{2}e^{-\frac{2\kappa t}{\lambda _{1}}}. \end{aligned}$$

So, we complete the proof of (1.11).

3.3 Regularity of Global Solutions

In this section, we shall prove the regularity of global solutions with lower energy initial value by applying a nonlocal version of the Brezis-Kato estimate (see [3, 27]).

Proposition 3.7

If $H, K\in L^{\frac{2N}{N-\mu }}(\Omega )+L^{\frac{2N}{N-\mu +2}}(\Omega )$ and $u\in L^{\infty }(0,\infty ; H^{1}_{0}(\Omega ))$ with $u_{t}\in L^{2}(0,\infty ; L^{2}(\Omega ))$ be a global solution of

$$\begin{aligned} u_{t}-\Delta u=\left( \vert x\vert ^{-\mu }*Hu\right) K. \end{aligned}$$

(3.47)

Then, $u\in L^{p}(\Omega \times [t_{0},\infty ))$ for every $p\in [2,\frac{N}{N-\mu }\frac{2N}{N-2})$.

Proof

For $M>0$, we define the function $u_{M}$ by

$$\begin{aligned} u_{M}(x)=\left\{ \begin{array}{lll} -M,\ u(x)\le -M,\\ u(x),\ -M<u(x)<M,\\ -M,\ u(x)\ge M. \end{array} \right. \end{aligned}$$

And for any fixed $t_{0}>0$ and $T>0$, choosing $\eta \in C^{\infty }(0,T)$ with $0\le \eta \le 1$ in (0, T), $\eta =1$ in $[t_{0},T]$, $\eta =0$ in $[0,\frac{t_{0}}{2}]$ and $|\eta _{t}|<\frac{1}{t_{0}}$.

Taking $\varphi (x,t)=\vert u_{M}\vert ^{p-2}u_{M}\eta$ as a test function to (3.47), we can obtain

$$\begin{aligned} \int ^{T}_{0}\int _{\Omega }[u_{t}\varphi +\nabla u\nabla \varphi -\left( |x|^{-\mu }*Hu\right) K\varphi ]dxdt=0. \end{aligned}$$

(3.48)

By manipulation, we have

$$\begin{aligned} \int ^{T}_{0}\int _{\Omega }u_{t}\varphi dxdt= & {} \int ^{T}_{0}\int _{\Omega }u_{t}\vert u_{M}\vert ^{p-2}u_{M}\eta dxdt=\frac{1}{p}\int ^{T}_{0}\int _{\Omega }(\vert u_{M}\vert ^{p})_{t}\eta dxdt \nonumber \\= & {} \frac{1}{p}\int ^{T}_{0}\int _{\Omega }(\vert u_{M}\vert ^{p}\eta )_{t}dxdt -\frac{1}{p}\int ^{T}_{0}\int _{\Omega }\vert u_{M}\vert ^{p}\eta _{t}dxdt, \end{aligned}$$

(3.49)

and

$$\begin{aligned} \int ^{T}_{0}\int _{\Omega }\nabla u\nabla \varphi dxdt= & {} \int ^{T}_{0}\int _{\Omega }\nabla u\nabla (\vert u_{M}\vert ^{p-2}u_{M}\eta )dxdt \nonumber \\= & {} \frac{4(p-1)}{p^{2}}\int ^{T}_{0}\int _{\Omega }|\nabla \vert u_{M}\vert ^{\frac{p}{2}}|^{2}\eta dxdt. \end{aligned}$$

(3.50)

If $p<\frac{2N}{N-\mu }$, by [27, Lemma 3.2] with $\theta =\frac{2}{p}$, one can get

$$\begin{aligned}{} & {} \int ^{T}_{0}\int _{\Omega }\left( |x|^{-\mu }*H u\right) K\varphi dxdt \nonumber \\{} & {} \quad =\int ^{T}_{0}\int _{\Omega }\left( |x|^{-\mu }*H u\right) K\vert u_{M}\vert ^{p-2}u_{M}\eta dxdt\nonumber \\{} & {} \quad \le \int ^{T}_{0}\eta \int _{\Omega } (\vert x\vert ^{-\mu }*Hu_{M})K\vert u_{M}\vert ^{p-2}u_{M}dxdt\nonumber \\{} & {} \qquad +\int ^{T}_{0}\eta \int _{A_{M}} (\vert x\vert ^{-\mu }*Hu_{M})K\vert u_{M}\vert ^{p-2}u_{M}dxdt \nonumber \\{} & {} \quad \le \frac{2(p-1)}{p^{2}}\int ^{T}_{0}\eta \int _{\Omega }\vert \nabla \vert u_{M}\vert ^{\frac{p}{2}}\vert ^{2}+C\int ^{T}_{0}\int _{\Omega }\vert \vert u_{M}\vert ^{\frac{p}{2}}\vert ^{2}\eta dxdt\nonumber \\{} & {} \qquad +\sup _{t}\int _{A_{M}} (\vert x\vert ^{-\mu }*Hu_{M})K\vert u_{M}\vert ^{p-2}u_{M}dxdt \end{aligned}$$

(3.51)

for some $C>0$, where $A_{M}:=\left\{ x\in \Omega \,\ \vert u(x)\vert >M\right\}$.

By the Hardy–Littlewood–Sobolev inequality, one has

$$\begin{aligned} \lim _{M\rightarrow \infty }\sup _{t}\int _{A_{M}} (\vert x\vert ^{-\mu }*Hu_{M})K\vert u_{M}\vert ^{p-2}u_{M}dxdt =0. \end{aligned}$$

(3.52)

From (3.48)–(3.52), we have

$$\begin{aligned}{} & {} \frac{1}{p}\sup _{t}\int _{\Omega }\vert u_{M}\vert ^{p}\eta dxdt +\frac{2(p-1)}{p^{2}}\int ^{T}_{0}\int _{\Omega }|\nabla \vert u_{M}\vert ^{\frac{p}{2}}|^{2}\eta dxdt\nonumber \\{} & {} \quad \le \frac{1}{p}\int ^{T}_{0}\int _{\Omega }\vert u_{M}\vert ^{p}\eta _{t}dxdt +C\int ^{T}_{0}\int _{\Omega }\vert u_{M}\vert ^{p}\eta dxdt \end{aligned}$$

(3.53)

Next, by the Sobolev inequality, we have

$$\begin{aligned}{} & {} \left( \int _{0}^{T}\int _{\Omega }\vert u\vert ^{\frac{pN}{N-2}}\eta ^{\frac{N}{N-2}} dxdt\right) ^{\frac{N-2}{N}}\nonumber \\{} & {} \quad \le \frac{1}{S}\int _{0}^{T} \int _{\Omega }\vert \nabla \vert u\vert ^{\frac{p}{2}}\vert ^{2}\eta dx dt \le C\int ^{T}_{0}\int _{\Omega }\vert u\vert ^{p}\eta dxdt \end{aligned}$$

(3.54)

By iterating over p a finite number of times, we cover the range $p\in [2,\frac{N}{N-\mu }\frac{2N}{N-2})$. $\square$

Proof of Theorem 1.7

Let $H=K=\vert u\vert ^{2^{*}_{\mu }-2}u$. By Proposition 3.7, $u(x,t)\in L^{p}(\Omega \times [t_{0},\infty ))$ for every $p\in [2,\frac{N}{N-\mu }\frac{2N}{N-2})$. Then $\vert u\vert ^{2^{*}_{\mu }}\in L^{q}(\Omega \times [t_{0},\infty ))$ for every $q\in [\frac{2(N-2)}{2N-\mu },\frac{N}{N-\mu }\frac{2N}{2N-\mu })$. Since $\frac{2(N-2)}{2N-\mu }<\frac{N}{N-\mu }<\frac{N}{N-\mu }\frac{2N}{2N-\mu }$, we have $\vert x\vert ^{-\mu }*\vert u\vert ^{2^{*}_{\mu }}\in L^{\infty }(\Omega \times [t_{0},\infty ))$. By the classical bootstrap method, we have $u(x,t)\in W_{r}^{2,1}(\Omega \times [t_{0},\infty ))$ for every $r>1$. Applying the Schauder estimate in [17], we can derive $u(x,t)\in C^{(2,\alpha )(1,\alpha )}(\Omega \times [t_{0},\infty ))$. Therefore, u(x, t) is a classical solution for all $t\ge t_{0}>0$.

4 Critical Energy Initial Value

In this section, we consider the global existence and blow-up of solution with critical energy initial value, i.e. $J_{\mu }(u_{0})=m_{\mu }$ and the decay estimate of global solutions.

Proof of Theorem 1.9(i)

Since $J_{\mu }(u_{0})=m_{\mu }$, we can see that $\Vert u_{0}\Vert ^{2}_{2}\ne 0$. Choose a sequence $\{\beta _{k}\}$ such that $0<\beta _{k}<1$, $k=1,2,\cdots$ and $\beta _{k}\rightarrow 1$ as $k\rightarrow \infty$, and let $u_{0,k}(x)=\beta _{k}u_{0}(x)$. Consider the initial and boundary value problem as follows:

$$\begin{aligned} \left\{ \begin{array}{lll} u_{t}-\Delta u=\left( |x|^{-\mu }*|u|^{2^{*}_{\mu }}\right) |u|^{2^{*}_{\mu }-2}u,\ {} &{} x\in \Omega ,\ t>0\\ u(x,t)=0,\ {} &{} x\in \partial \Omega ,\ t>0\\ u(x,0)=u_{0,k}(x),\ {} &{} x\in \Omega \end{array} \right. \end{aligned}$$

(4.1)

Since $I_{\mu }(u_{0})>0\ \mathrm{by\ Lemma\ }$ 2.5, there exist a ${\bar{s}}:=s(u_{0})>1$ such that $I_{\mu }({\bar{s}}u_{0})=0$. Thus, since $\beta _{k}<1< {\bar{s}}$, we can deduce that $I_{\mu }(u_{0,k})=I_{\mu }(\beta _{k}u_{0})>0$ and $J_{\mu }(u_{0,k})=J_{\mu }(\beta _{k}u_{0})<J_{\mu }(u_{0})=m_{\mu }$. It follows from Theorem 1.4 that for each k, Eq. (4.1) admits a global weak solution $u_{k}(t)\in L^{\infty }(0,T;H^{1}_{0}(\Omega ))$ with $(u_{k})_{t}\in L^{2}(\Omega _{T})=L^{2}(0,T;L^{2}(\Omega ))$ and $u_{k}(t)\in W$ for $0\le t<\infty$ satisfying

$$\begin{aligned} ((u_{k})_{t},v)+(\nabla u_{k},\nabla v)=\left( \left( |x|^{-\mu }*|u_{k}|^{2^{*}_{\mu }}\right) |u_{k}|^{2^{*}_{\mu }-2}u_{k},v\right) ,\ \forall v\in H^{1}_{0}(\Omega ),\ t>0,\nonumber \\ \end{aligned}$$

(4.2)

and

$$\begin{aligned} \int ^{t}_{0}\int _{\Omega }|(u_{k})_{s}|^{2}dxds +J(u_{k}) =J(u_{0,k}) <m_{\mu }. \end{aligned}$$

(4.3)

From (4.3) and

$$\begin{aligned} J(u_{k})=\frac{2^{*}_{\mu }-1}{22^{*}_{\mu }}\int _{\Omega }|\nabla u_{k}|^{2}dx +\frac{1}{22^{*}_{\mu }}I(u_{k}), \end{aligned}$$

we can get

$$\begin{aligned} \int ^{t}_{0}\int _{\Omega }|(u_{k})_{s}|^{2}dxds +\frac{2^{*}_{\mu }-1}{22^{*}_{\mu }}\int _{\Omega }|\nabla u_{k}|^{2}dx \le J(u_{0,k}) <m_{\mu }. \end{aligned}$$

(4.4)

This implies that

$$\begin{aligned} \int _{\Omega }|\nabla u_{k}|^{2}dx<S^{\frac{2N-\mu }{N-\mu +2}}_{H,L},,\ 0\le t<\infty , \end{aligned}$$

(4.5)

$$\begin{aligned} \int ^{t}_{0}\Vert (u_{k})_{\tau }\Vert _{2}^{2}d\tau<\frac{N-\mu +2}{2(2N-\mu )}S^{\frac{2N-\mu }{N-\mu +2}}_{H,L},,\ 0\le t<\infty , \end{aligned}$$

(4.6)

and by the Hardy–Littlewood–Sobolev inequality, we have

$$\begin{aligned}{} & {} \int _{\Omega }\left( \left( \vert x\vert ^{-\mu }*|u_{k}|^{2^{*}_{\mu }}\right) |u_{k}|^{2^{*}_{\mu }-2}u_{k} \right) ^{\frac{2N}{N+2}}dx\nonumber \\{} & {} \quad \le \left( \int _{\Omega } \left( \vert x\vert ^{-\mu }*|u_{k}|^{2^{*}_{\mu }}\right) ^{\frac{2N}{N+2}\frac{N+2}{\mu }}dx\right) ^{\frac{\mu }{N+2}}\left( \int _{\Omega } (|u_{k}|^{2^{*}_{\mu }-2}u) ^{\frac{2N}{N+2}\frac{N+2}{N-\mu +2}}dx\right) ^{\frac{N-\mu +2}{N+2}}\nonumber \\{} & {} \quad \le \left( \int _{\Omega } |u_{k}|^{2^{*}_{\mu }\frac{2N}{2N-\mu }}dx\right) ^{\frac{\mu }{N+2}}\left( \int _{\Omega } |u_{k}|^{\frac{2N}{N-2}}dx\right) ^{\frac{N-\mu +2}{N+2}} \le C\left( \int _{\Omega }|\nabla u_{k}|^{2}dx\right) ^{\frac{2^{*}}{2}}. \end{aligned}$$

(4.7)

Therefore, there exist a u and a subsequence $\{u^{\nu }\}$ such that

$$\begin{aligned} u^{\nu }\rightarrow & {} u\ \text {in}\ L^{\infty }(0,\infty ;H_{0}^{1}(\Omega ))\ \text {weak star and a.e. in}\ Q=\Omega \times [0,\infty ),\\ u_{t}^{\nu }\rightarrow & {} u_{t}\ \text {in}\ L^{2}(0,\infty ;L^{2}(\Omega ))\ \text {weakly star}\\ \left( \vert x\vert ^{-\mu }*|u^{\nu }|^{2^{*}_{\mu }}\right) |u^{\nu }|^{2^{*}_{\mu }-2}u^{\nu }\rightarrow & {} \left( \vert x\vert ^{-\mu }*|u|^{2^{*}_{\mu }}\right) |u|^{2^{*}_{\mu }-2}u \ \text {in}\ L^{\infty }(0,\infty ;L^{\frac{2N}{N+2}}(\Omega ))\ \text {weak star}. \end{aligned}$$

In (4.2), we fixed s and letting $k=\nu =\infty$, we can get

$$\begin{aligned} (u_{t},\phi _{s})+(\nabla u_{t},\nabla \phi _{s}) =\left( \left( |x|^{-\mu }*|u|^{2^{*}_{\mu }}\right) |u|^{2^{*}_{\mu }-2}u,\phi _{s}\right) ,\ \forall s=1,2,\cdots , \end{aligned}$$

and

$$\begin{aligned} (u_{t},v)+(\nabla u_{t},\nabla v) =\left( \left( |x|^{-\mu }*|u|^{2^{*}_{\mu }}\right) |u|^{2^{*}_{\mu }-2}u,v\right) ,\ \forall v\in H^{1}_{0}(\Omega ),\ \forall t. \end{aligned}$$

Moreover, (4.2) gives $u(x,0)=u_{0}(x)$ in $H^{1}_{0}(\Omega )$. The reminder proof is similar to the case of $J_{\mu }(u_{0})<m_{\mu }$, here we omit it. Consequently, the proof is complete.

Proof of Theorem 1.9(ii)

Let u(t) be any weak solution of the problem (P) with $J_{\mu }(u_{0})=m_{\mu }$ and $I_{\mu }(u_{0})<0$, we shall prove $T_{\max }<\infty$, where $T_{\max }$ be the existence time of u(t). On the contrary, we suppose $T_{\max }=\infty$, and we define a auxiliary function

$$\begin{aligned} g(t)=\int ^{t}_{0}\int _{\Omega }u(s)^{2}dxds. \end{aligned}$$

By (2.2) and standard manipulation, we have

$$\begin{aligned} g^{\prime }(t)= & {} \int _{\Omega }|u(t)|^{2}dx \nonumber \\= & {} \int _{\Omega }u_{0}^{2}dx +2\int ^{t}_{0}\left( -\int _{\Omega }|\nabla u|^{2}dx +\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\right) ds, \qquad \quad \end{aligned}$$

(4.8)

and

$$\begin{aligned} g^{\prime \prime }(t)=-2\int _{\Omega }|\nabla u|^{2}dx +2\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy =-2I_{\mu }(u). \end{aligned}$$

(4.9)

By (2.4) and (4.9), we have

$$\begin{aligned} g^{\prime \prime }(t)\ge & {} 2\left( 2^{*}_{\mu }-1\right) \int _{\Omega }|\nabla u|^{2}dx -42^{*}_{\mu }J_{\mu }(u_{0}) +42^{*}_{\mu }\int ^{t}_{0}\int _{\Omega }|u_{s}|^{2}dxds \nonumber \\\ge & {} 2\left( 2^{*}_{\mu }-1\right) \lambda _{1}g^{\prime }(t) -42^{*}_{\mu }m_{\mu } +42^{*}_{\mu }\int ^{t}_{0}\int _{\Omega }|u_{s}|^{2}dxds. \end{aligned}$$

(4.10)

Note that

$$\begin{aligned} \left( \int ^{t}_{0}\int _{\Omega }u_{s}udxds\right) ^{2}= & {} \left( \frac{1}{2}\int ^{t}_{0}\frac{d}{ds}\int _{\Omega }|u|^{2}dxds\right) ^{2}\\= & {} \frac{1}{4}\left( \left( \int _{\Omega }|u|^{2}dx\right) ^{2}-2\int _{\Omega }|u|^{2}dx\int _{\Omega }|u_{0}|^{2}dx +\left( \int _{\Omega }|u_{0}|^{2}dx\right) ^{2}\right) \\= & {} \frac{1}{4}\left( \left( g^{\prime }(t)\right) ^{2}-2g^{\prime }(t)\Vert u_{0}\Vert _{2}^{2} +\Vert u_{0}\Vert _{2}^{4}\right) , \end{aligned}$$

then, we can get

$$\begin{aligned} \left( g^{\prime }(t)\right) ^{2}=4\left( \int ^{t}_{0}\int _{\Omega }u_{s}udxds\right) ^{2} +2g^{\prime }(t)\Vert u_{0}\Vert _{2}^{2} -\Vert u_{0}\Vert _{2}^{4}. \end{aligned}$$

(4.11)

Furthermore, by (4.10)–(4.11) and the Schwartz inequality, we have

$$\begin{aligned}{} & {} g^{\prime \prime }(t)g(t)-2^{*}_{\mu }\left( g^{\prime }(t)\right) ^{2} \nonumber \\{} & {} \quad \ge 42^{*}_{\mu }\left( \int ^{t}_{0}\int _{\Omega }|u_{t}|^{2}dxds\int ^{t}_{0}\int _{\Omega }u(s)^{2}dxds -\left( \int ^{t}_{0}\int _{\Omega }u_{s}udxds\right) ^{2}\right) \nonumber \\{} & {} \qquad +2\left( 2^{*}_{\mu }-1\right) \lambda _{1}g^{\prime }(t)g(t) -22^{*}_{\mu }g^{\prime }(t)\Vert u_{0}\Vert _{2}^{2} -42^{*}_{\mu }m_{\mu }g(t) \nonumber \\{} & {} \quad \ge 2\left( 2^{*}_{\mu }-1\right) \lambda _{1}g^{\prime }(t)g(t) -22^{*}_{\mu }g^{\prime }(t)\Vert u_{0}\Vert _{2}^{2} -42^{*}_{\mu }m_{\mu }g(t) \nonumber \\{} & {} \quad =\left( (2^{*}_{\mu }-1)\lambda _{1}g(t) -22^{*}_{\mu }\Vert u_{0}\Vert _{2}^{2}\right) g^{\prime }(t) +\left( (2^{*}_{\mu }-1)\lambda _{1}g^{\prime }(t) -42^{*}_{\mu }m_{\mu }\right) g(t). \qquad \quad \end{aligned}$$

(4.12)

Since $J_{\mu }(u_{0})=m_{\mu }$ and $I_{\mu }(u_{0})<0$, and by the continuity of $J_{\mu }$ and $I_{\mu }$ with respect to t, there exists a sufficiently small $t_{1}>0$ such that $J_{\mu }(u(t))>0$ and $I_{\mu }(u(t))<0$ for $0\le t\le t_{1}$. Then, $(u_{t},u)=-I_{\mu }(u)>0$, and $\Vert u_{t}\Vert ^{2}_{2}>0$ for $0\le t\le t_{1}$. Hence

$$\begin{aligned} J_{\mu }(u(t_{1}))\le m_{\mu }-\int _{0}^{t_{1}}\Vert u_{\tau }\Vert ^{2}_{2}d\tau ={\tilde{m}}_{\mu }<m_{\mu }. \end{aligned}$$

Thus, we choose $t=t_{1}$ as the initial time and by Proposition 3.4, we have $u(t)\in V_{\delta }$ for $\delta _{1}<\delta <\delta _{2}$ and $t_{1}\le t<\infty$, where $\delta _{1}$ and $\delta _{2}$ are two roots of $m_{\mu }(\delta )={\tilde{m}}_{\mu }$. Hence, $I_{\mu ,\delta }(u)<0$ and $\Vert \nabla u\Vert ^{2}_{2}>r(\delta )$ for $\delta _{1}<\delta <\delta _{2}$ and $t_{1}\le t<\infty$. Hence, $I_{\mu ,\delta _{2}}(u)<0$ and $\Vert \nabla u\Vert ^{2}_{2}>r(\delta _{2})$ for $t_{1}\le t<\infty$.

By (4.9), we can get

$$\begin{aligned} g^{\prime \prime }(t)= & {} -2\Vert \nabla u\Vert ^{2}_{2} +2\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\\= & {} 2(\delta _{2}-1)\Vert \nabla u\Vert ^{2}_{2}-2I_{\mu ,\delta _{2}}(u)\\\ge & {} 2(\delta _{2}-1)r(\delta _{2}),\ t_{1}\le t<\infty . \end{aligned}$$

Furthermore, we have

$$\begin{aligned} g^{\prime }(t)\ge 2(\delta _{2}-1)r(\delta _{2})(t-t_{1})+g^{\prime }(t_{1}) \ge 2(\delta _{2}-1)r(\delta _{2})(t-t_{1}),\ t_{1}\le t<\infty , \end{aligned}$$

and

$$\begin{aligned} g(t)\ge (\delta _{2}-1)r(\delta _{2})(t-t_{1})^{2}+g(t_{1}) >(\delta _{2}-1)r(\delta _{2})(t-t_{1})^{2},\ t_{1}\le t<\infty . \end{aligned}$$

Therefore, for t large enough, we can get that

$$\begin{aligned} (2^{*}_{\mu }-1)\lambda _{1}g(t)>22^{*}_{\mu }\Vert u_{0}\Vert _{2}^{2}\ \text {and}\ (2^{*}_{\mu }-1)\lambda _{1}g^{\prime }(t) >42^{*}_{\mu }m_{\mu }. \end{aligned}$$

Then, from (4.12) it follows that

$$\begin{aligned} g^{\prime \prime }(t)g(t)-2^{*}_{\mu }\left( g^{\prime }(t)\right) ^{2}>0. \end{aligned}$$

Then, by Lemma 2.10, there exists a $T>0$ such that $\lim _{t\rightarrow T^{-}}f(t)=+\infty$, which contradicts $T_{\max }=+\infty$.

5 High Energy Initial Value

In this section, we investigate the conditions to ensure the existence of global or finite time blow-up of solutions to problem (P) with high energy initial value, i.e. $J_{\mu }(u_{0})>m_{\mu }$. As mentioned in Introduction, we define

$$\begin{aligned} {\mathcal {N}}_{+}=\{u\in H^{1}_{0}(\Omega )\ |\ I_{\mu }(u)>0\}\ \text {and}\ {\mathcal {N}}_{-}=\{u\in H^{1}_{0}(\Omega )\ |\ I_{\mu }(u)<0\}, \end{aligned}$$

and the level set of $J_{\mu }$ as follows:

$$\begin{aligned} J_{\mu }^{d}:=\{u\in H^{1}_{0}(\Omega )\ |\ J_{\mu }(u)<d\}. \end{aligned}$$

Obviously, we have

$$\begin{aligned} {\mathcal {N}}_{d}:={\mathcal {N}}\cap J^{d}=\left\{ u\in {\mathcal {N}}\ |\ \Vert \nabla u\Vert ^{2}_{2}<\frac{2(2N-\mu )d}{N-\mu +2}\right\} \ne \emptyset ,\ \text {for}\ d>m_{\mu }. \end{aligned}$$

Furthermore, for all $d>m_{\mu }$, we set

$$\begin{aligned} \lambda _{d}=\inf \{\Vert u\Vert ^{2}_{2}\ |\ u\in {\mathcal {N}}_{d}\}\ \text{ and }\ \Lambda _{d}=\sup \{\Vert u\Vert ^{2}_{2}\ |\ u\in {\mathcal {N}}_{d}\}. \end{aligned}$$

It is clear that $\lambda _{d}$ is nonincreasing and $\Lambda _{d}$ is nondecreasing in d.

If $T_{\max }=\infty$, we denote by

$$\begin{aligned} \omega (u_{0}):= \bigcap _{t\ge 0}\overline{\lbrace u(s):\ s\ge t \rbrace } \end{aligned}$$

the $\omega$-limit set of $u_{0}\in H^{1}_{0}(\Omega )$. Finally, we introduce the following sets

$$\begin{aligned} {\mathcal {B}}&=\left\{ u_{0}\in H^{1}_{0}(\Omega )\ |\ \text {the solution}\ u=u(t)\ \text {of } ({ P}) \text { blows up in finite time} \right\} ,\\ {\mathcal {G}}&=\left\{ u_{0}\in H^{1}_{0}(\Omega )\ |\ \text {the solution}\ u=u(t)\ \text {of } ({ P}) \text { exist for all}\ t>0 \right\} ,\\ {\mathcal {G}}_{0}&=\left\{ u_{0}\in {\mathcal {G}}\ |\ u(t)\rightarrow 0\ \text {in}\ H^{1}_{0}(\Omega )\ \text {as}\ t\rightarrow \infty \right\} . \end{aligned}$$

Clearly, $H^{1}_{0}(\Omega )={\mathcal {G}}\cup {\mathcal {B}}$. Now, we give two lemmas, which play important roles in the proof of the main results.

Lemma 5.1

We have

• (i) 0 is away from both ${\mathcal {N}}$ and ${\mathcal {N}}_{-}$, i.e. $dist(0,{\mathcal {N}})>0$ and $dist(0,{\mathcal {N}}_{-})>0$;

• (ii) For any $d>0$, the set $J^{d}\cap {\mathcal {N}}_{+}$ is bounded in $H^{1}_{0}(\Omega )$.

Proof

(i):

For any $u\in {\mathcal {N}}$, we have

$$\begin{aligned} m_{\mu }\le J_{\mu }(u)= & {} \frac{1}{2}\int _{\Omega }|\nabla u|^{2}dx -\frac{1}{22^{*}_{\mu }} \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\\= & {} \frac{N-\mu +2}{2(2N-\mu )}\int _{\Omega }|\nabla u|^{2}dx, \end{aligned}$$

which implies that there exists a constants $c>0$ such that $dist(0,{\mathcal {N}})=\inf _{u\in {\mathcal {N}}}\Vert \nabla u\Vert _{2}>0$. For any $u\in {\mathcal {N}}_{- }$ , that is $I_{\mu }(u)<0$, we have $\Vert \nabla u\Vert _{2}\ne 0$. Then, it follows the Hardy–Littlewood–Sobolev inequality that

$$\begin{aligned} \Vert \nabla u\Vert ^{2}_{2} <\int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy \le S^{-\frac{2N-\mu }{N-2}}_{H,L}\left( \Vert \nabla u\Vert ^{2}_{2}\right) ^{\frac{2N-\mu }{N-2}}, \end{aligned}$$

which implies that $\Vert \nabla u\Vert ^{2}_{2}>S^{\frac{2N-\mu }{N-\mu +2}}_{H,L}$. Therefore, $dist(0,{\mathcal {N}}_{-})=\inf _{u\in {\mathcal {N}}_{-}}\Vert \nabla u\Vert _{2}>0$.

(ii):

For any $u\in J^{d}\cap {\mathcal {N}}_{+}$, that is $J_{\mu }(u)<d$ and $I_{\mu }(u)>0$. Then, it follows from this and the Hardy–Littlewood–Sobolev inequality that

$$\begin{aligned} d> J_{\mu }(u)= & {} \frac{1}{2}\int _{\Omega }|\nabla u|^{2}dx -\frac{1}{22^{*}_{\mu }} \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\\ {}= & {} {} \frac{N-\mu +2}{2(2N-\mu )}\int _{\Omega }|\nabla u|^{2}dx +\frac{1}{22^{*}_{\mu }}I_{\mu }(u)\\ {}> & {} {} \frac{N-\mu +2}{2(2N-\mu )}\int _{\Omega }|\nabla u|^{2}dx, \end{aligned}$$

which implies that

$$\begin{aligned} \Vert \nabla u\Vert ^{2}_{2}<\frac{2(2N-\mu )d}{N-\mu +2}. \end{aligned}$$

Consequently, the proof is complete.$\square$

Lemma 5.2

Let $u_{0}\in H^{1}_{0}(\Omega )$. Then,

$$\begin{aligned} \frac{d}{dt}\Vert u\Vert ^{2}_{2}=-2I_{\mu }(u), \ \text {for all}\ t\in (0,T_{\max }). \end{aligned}$$

(5.1)

Proof

Multiplying (P) by u(t) and integrating by parts immediately, we can complete the proof. $\square$

Now, the proof of Theorem 1.10-1.12 are to show as follows.

Proof of Theorem 1.10

(i):

Assume that $u_{0}\in {\mathcal {N}}_{+}$ with $\Vert u_{0}\Vert _{2}\le \lambda _{J(u_{0})}$. We claim that $u(t)\in {\mathcal {N}}_{+}$ for all $t\in [0,T_{\max })$. On the contrary, there is a $t_{0}>0$ such that $u(t)\in {\mathcal {N}}_{+}$ for $0\le t<t_{0}$ and $u(t_{0})\in {\mathcal {N}}$, then (1.3) and (5.1) imply that

$$\begin{aligned} \Vert u(t_{0})\Vert _{2}\le \Vert u_{0}\Vert _{2}\le \lambda _{J(u_{0})},\ J_{\mu }(u(t_{0}))\le J_{\mu }(u_{0}). \end{aligned}$$

This cintradicts the definition of $\lambda _{J(u_{0})}$ and proves the claim. By Lemma 5.1 (ii), we have that the orbit $\left\{ u(t)\right\}$ remains bounded in $H^{1}_{0}(\Omega )$ for $[0,T_{\max })$, so that $T_{\max }=+\infty$. Now, for any $w\in \omega (u_{0})$, by (1.3) and (5.1), we have

$$\begin{aligned} \Vert w\Vert _{2}<\lambda _{J_{\mu }(u_{0})}\ \text {and}\ J_{\mu }(w)\le J_{\mu }(u_{0}). \end{aligned}$$

By the definition of $\lambda _{J_{\mu }(u_{0})}$, we can get that $\omega (u_{0}) \cap {\mathcal {N}}=\emptyset$, hence $\omega (u_{0}) =\left\{ 0\right\}$. In other words, $u_{0}\in {\mathcal {G}}_{0}$.

(ii):

$u_{0}\in {\mathcal {N}}_{-}$ and $\Vert u_{0}\Vert _{2}\le \Lambda _{J(u_{0})}$. A similar argument as (i), one can get that $u(t)\in {\mathcal {N}}_{-}$ for all $t\in [0,T_{\max })$.

Next, by contradiction, if $T_{\max }=\infty$, then for every $w\in \omega (u_{0})$, it follows from (1.3) and (5.1) that

$$\begin{aligned} \Vert w\Vert _{2}>\Lambda _{J_{\mu }(u_{0})}\ \text {and}\ J_{\mu }(w)\le J_{\mu }(u_{0}). \end{aligned}$$

By the definition of $\Lambda _{J_{\mu }(u_{0})}$, we derive that $\omega (u_{0}) \cap {\mathcal {N}}=\emptyset$. However, since $dist(0,{\mathcal {N}}_{-})>0$ in Lemma 5.1 (i), we also have $0\notin \omega (u_{0})$. This gives $\omega (u_{0}) =\emptyset$, contrary to the assumption that u(t) is a global solution. Hence, $T_{\max }<\infty$ and the proof is complete.

Proof of Theorem 1.11

For

$$\begin{aligned} J_{\mu }(u)=\frac{1}{2}\int _{\Omega }|\nabla u|^{2}dx -\frac{1}{22^{*}_{\mu }} \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy. \end{aligned}$$

Since $r_{\Omega }=\sup _{x,y\in \Omega }\vert x-y\vert$, for any $u\in H^{1}_{0}(\Omega )$, there hold

$$\begin{aligned} \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\ge (r_{\Omega })^{-\mu }\Vert u\Vert ^{22^{*}_{\mu }}_{2^{*}_{\mu }}. \end{aligned}$$

(5.2)

By (1.12) and (5.2) and using the H$\ddot{o}$lder inequality, for $\mu <4$, we get

$$\begin{aligned} \int _{\Omega }\int _{\Omega }\frac{|u_{0}|^{2^{*}_{\mu }}|u_{0}|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\ge & {} (r_{\Omega })^{-\mu }\Vert u_{0}\Vert ^{22^{*}_{\mu }}_{2^{*}_{\mu }}>(r_{\Omega })^{-\mu }\vert \Omega \vert ^{-2^{*}_{\mu }+2}\Vert u_{0}\Vert ^{22^{*}_{\mu }}_{2}\\\ge & {} \frac{22^{*}_{\mu }}{2^{*}_{\mu }-1}J_{\mu }(u_{0}) \end{aligned}$$

Then, we readily infer that $\int _{\Omega }\int _{\Omega }\frac{|u_{0}|^{2^{*}_{\mu }}|u_{0}|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy>\Vert \nabla u_{0}\Vert ^{2}_{2}$, which implies that $u_{0}\in {\mathcal {N}}_{-}$.

Next, we shall show $u_{0}\in {\mathcal {B}}$. Since $u_{0}\in {\mathcal {N}}_{-}$, by Theorem 1.10, we only need to prove that $\Vert u_{0}\Vert _{2}\ge \Lambda _{J(u_{0})}$. For any $u\in {\mathcal {N}}_{J(u_{0})}$ i.e. $u\in {\mathcal {N}}$ and $J_{\mu }(u)<J_{\mu }(u_{0})$, by the H$\ddot{o}$lder inequality, we have

$$\begin{aligned} (r_{\Omega })^{-\mu }\vert \Omega \vert ^{-2^{*}_{\mu }+2}\Vert u\Vert ^{22^{*}_{\mu }}_{2}<(r_{\Omega })^{-\mu }\Vert u\Vert ^{22^{*}_{\mu }}_{2^{*}_{\mu }}\le \int _{\Omega }\int _{\Omega }\frac{|u(x)|^{2^{*}_{\mu }}|u(y)|^{2^{*}_{\mu }}}{|x-y|^{\mu }}dxdy\le \frac{22^{*}_{\mu }}{2^{*}_{\mu }-1}J(u_{0}). \end{aligned}$$

Therefore, taking the supremum over ${\mathcal {N}}_{J(u_{0})}$, we immediately get

$$\begin{aligned} \Lambda ^{22^{*}_{\mu }}_{J(u_{0})}\le \frac{22^{*}_{\mu }}{2^{*}_{\mu }-1}(r_{\Omega })^{\mu }\vert \Omega \vert ^{2^{*}_{\mu }-2}J_{\mu }(u_{0})\le \Vert u_{0}\Vert ^{22^{*}_{\mu }}_{2}. \end{aligned}$$

Therefore, $\Vert u_{0}\Vert _{2}\ge \Lambda _{J(u_{0})}$ and we complete the proof by Theorem 1.10.

Proof of Theorem 1.12

Let $M>0$ and $\Omega _{1},\Omega _{2}$ be two arbitrary disjoint open subdomain of $\Omega$. Furthermore, let $v\in H^{1}_{0}(\Omega _{1})\subset H^{1}_{0}(\Omega )$ be an arbitrary nonzero function. Then, we can choose $\alpha$ large enough such that $\Vert \alpha v\Vert ^{22^{*}_{\mu }}_{2}\ge \frac{22^{*}_{\mu }}{2^{*}_{\mu }-1}(r_{\Omega })^{\mu }\vert \Omega \vert ^{2^{*}_{\mu }-2}M$ and $J(\alpha v)\le 0$. Fix such $\alpha >0$ and pick a function $w\in H^{1}_{0}(\Omega )$ with $J(w)=M-J(\alpha v)$. Then, $u_{M}:=w+\alpha v$ satisfies $J(u_{M})=J(w)+J(\alpha v)=M$ and

$$\begin{aligned} \Vert u_{M}\Vert ^{22^{*}_{\mu }}_{2}\ge \Vert \alpha v\Vert ^{22^{*}_{\mu }}_{2}\ge \frac{22^{*}_{\mu }}{2^{*}_{\mu }-1}(r_{\Omega })^{\mu }\vert \Omega \vert ^{2^{*}_{\mu }-2}J(u_{M}), \end{aligned}$$

By Theorem 1.11, it is seen that $u_{M}\in {\mathcal {N}}_{-}\cap {\mathcal {B}}$. This complete the proof.

6 The Proof of Theorem 1.13 and Theorem 1.14

Proof of Theorem 1.13

Let us denote $u_{n}:=u(x,t_{n})$. Since $\left\{ u_{n}\right\}$ is uniformly bounded in $H^{1}_{0}(\Omega )$, then there exists a subsequence (here we still denote by $\left\{ u_{n}\right\}$ ) and a function $w\in H^{1}_{0}(\Omega )$ such that

$$\begin{aligned} u_{n}\rightharpoonup w\ {}{} & {} \text {in}\ H^{1}_{0}(\Omega )\\ u_{n}\rightarrow w\ {}{} & {} \text {in}\ L^{2}(\Omega )\\ u_{n}\rightarrow w\ {}{} & {} \text {a.e. in}\ \Omega . \end{aligned}$$

Let $U_{n}:=u(t_{n}+s)$ for $s\in (0,1)$. Clearly, $U_{n}$ is uniformly bounded in $H^{1}_{0}(\Omega )$, we show

$$\begin{aligned} U_{n}\rightarrow w\ \text {in}\ L^{2}(\Omega ). \end{aligned}$$

Indeed, for $s\in (0,1)$, by (2.4), we have

$$\begin{aligned} \int ^{\infty }_{0}\Vert u_{\tau }\Vert _{2}^{2}d\tau +J_{\mu }(u(t)) =J_{\mu }(u_{0})<\infty , \end{aligned}$$

which means $u_{t}\in L^{2}(\Omega )$. So

$$\begin{aligned} \int _{\Omega }\vert U_{n}-u_{n}\vert ^{2}dx=t\int ^{s+t_{n}}_{t_{n}}\int _{\Omega }\vert u_{\tau }\vert ^{2}dxd\tau \rightarrow 0, \end{aligned}$$

for $0\le s\le 1$ as $t_{n}\rightarrow \infty$, which implies that $\Vert u(s+t_{n})-u(t_{n})\Vert _{2}\rightarrow 0$ as $t_{n}\rightarrow \infty$ for $0\le s\le 1$. Therefore, we have

$$\begin{aligned} U_{n}\rightarrow w\ \text {in}\ L^{2}(\Omega ). \end{aligned}$$

and

$$\begin{aligned} U_{n}\rightarrow w\ \text {a.e. in}\ \Omega . \end{aligned}$$

Since $\left\{ U_{n}\right\}$ is uniformly bounded in $H^{1}_{0}(\Omega )$, by (4.7), we also have $\left( |x|^{-\mu }*|U_{n}|^{2^{*}_{\mu }}\right) |U_{n}|^{2^{*}_{\mu }-2}U_{n}$ is bounded in $L^{\frac{2N}{N+2}}$, and

$$\begin{aligned} \left( |x|^{-\mu }*|U_{n}|^{2^{*}_{\mu }}\right) |U_{n}|^{2^{*}_{\mu }-2}U_{n}\rightharpoonup \left( |x|^{-\mu }*|w|^{2^{*}_{\mu }}\right) |w|^{2^{*}_{\mu }-2}w\ \text {in}\ L^{\frac{2N}{N+2}}(\Omega )\ \text {weak star}.\nonumber \\ \end{aligned}$$

(6.1)

In order to show that w is an equilibrium, we pass to the limit (as $t_{n}\rightarrow \infty$) in the identity (2.1) with a suitably chosen test function. Let

$$\begin{aligned} \phi (x,t)= \left\{ \begin{array}{lll} \rho (t-t_{n})\Psi (x),\ {} &{} t>t_{n},\ x\in {\bar{\Omega }},\\ 0,\ {} &{} 0\le t\le t_{n},\ x\in {\bar{\Omega }}, \end{array} \right. \end{aligned}$$

where

$$\begin{aligned} \Psi \in H^{1}_{0}(\Omega ), \rho \in C^{2}_{0}(0,1), \rho \ge 0, \int _{0}^{1}\rho (s)ds=1. \end{aligned}$$

Take $\phi$ as test function in (2.1), we have

$$\begin{aligned} \int _{t_{n}}^{t_{n}+1}\int _{\Omega }\left[ u\rho ^{\prime }(t-t_{n})\Psi -\rho (t-t_{n})\nabla u\nabla \Psi +\left( |x|^{-\mu }*|u|^{2^{*}_{\mu }}\right) |u|^{2^{*}_{\mu }-2}u\rho (t-t_{n})\Psi \right] dxdt=0. \end{aligned}$$

Furthermore, by transforming about t, we get

$$\begin{aligned} \int _{0}^{1}\int _{\Omega }\left[ U_{n}\rho ^{\prime }\Psi -\rho \nabla U_{n}\nabla \Psi +\left( |x|^{-\mu }*|U_{n}|^{2^{*}_{\mu }}\right) |U_{n}|^{2^{*}_{\mu }-2}U_{n}\rho \Psi \right] dxdt=0. \end{aligned}$$

(6.2)

From the choice of $\rho$, we can derive

$$\begin{aligned} \int _{\Omega }\left[ \nabla U_{n}\nabla \Psi +\left( |x|^{-\mu }*|U_{n}|^{2^{*}_{\mu }}\right) |U_{n}|^{2^{*}_{\mu }-2}U_{n}\Psi \right] dx=o(1),\ \text {as}\ n\rightarrow \infty . \end{aligned}$$

Consequently, the assertion follows then from (6.1).

Proof of Theorem 1.14

Let $u=u(t,x)$ be a global solution of problem (P). Then, we have

$$\begin{aligned} \int ^{\infty }_{0}\int _{\Omega }u^{2}_{t}dxdt\le C<\infty . \end{aligned}$$

(6.3)

And hence, there exists a sequence $\left\{ t_{n}\right\}$ satisfying $t_{n}\rightarrow \infty$ as $n\rightarrow \infty$ such that

$$\begin{aligned} \int _{\Omega }\vert u_{t}(t_{n},x)\vert ^{2}dx\rightarrow 0,\ \text {as}\ n\rightarrow \infty . \end{aligned}$$

(6.4)

Indeed, on the contrary, if there exist $c>0$ such that $\int _{\Omega }\vert u_{t}(t_{n},x)\vert ^{2}dx>c$ as $n\rightarrow \infty$, then, we can derive a contradiction with (6.3).

Next, let $u_{n}:=u(t_{n},x)$. By Theorem 1.4 and Remark 1.3, we have $J_{\mu }(u(t))>0$ for $t>0$. Then, by (2.4), we have

$$\begin{aligned} 0<J_{\mu }(u(t))\le J_{\mu }(u_{0}). \end{aligned}$$

Therefore, we have for the sequence $\left\{ t_{n}\right\}$ hold

$$\begin{aligned} 0<J_{\mu }(u(t_{n}))\le J_{\mu }(u_{0}). \end{aligned}$$

(6.5)

Then, (6.4) and (6.5) implies that $u_{n}:=u(t_{n},x)$ is a PS sequence related to the stationary equation of problem (P). similar to the argument of [9], it is easy to prove that there exists a constant C such that

$$\begin{aligned} \int _{\Omega }\vert \nabla u_{n}\vert ^{2}dx\le C, \end{aligned}$$

and then there exists a subsequence (denote still by $\left\{ u_{n}\right\}$ ) and a function w such that

$$\begin{aligned} u_{n}\rightharpoonup w,\ {}{} & {} \text {in}\ H^{1}_{0}(\Omega ),\\ u_{n}\rightarrow w,\ {}{} & {} \text {in}\ L^{q}(\Omega ) (2\le q<2^{*}). \end{aligned}$$

Furthermore, $u_{n}\rightarrow w\not = 0$ in $H^{1}_{0} (\Omega )$, which means that w is a nontrivial stationary solution.