Image correlation spectroscopy of randomly distributed disks

Abstract

Image correlation spectroscopy (ICS) has been widely used to quantify spatiotemporal distributions of fluorescently labelled cell membrane proteins and receptors. When the membrane proteins are randomly distributed, ICS may be used to estimate protein densities, provided the proteins behave as point-like objects. At high protein area fraction, however, even randomly placed proteins cannot obey Poisson statistics, because of excluded area. The difficulty can arise if the protein effective area is quite large, or if proteins form large complexes or aggregate into clusters. In these cases, there is a need to determine the correct form of the intensity correlation function for hard disks in two dimensions, including the excluded area effects. We present an approximate but highly accurate algorithm for the computation of this correlation function. The correlation function was verified using test images of randomly distributed hard disks of uniform intensity convolved with the microscope point spread function. This algorithm can be readily modified to compute exact intensity correlation functions for any probe geometry, interaction potential, and fluorophore distribution; we show how to apply it to describe a random distribution of large proteins labeled with a single fluorophore.

Appendix: Hard ring image correlation spectroscopy

The normalized intensity autocorrelation function of a ring of inner radius R ₁ and outer radius R ₂ can be derived from geometrical considerations. For $2R_1 \leq \left( {R_{\,2} -R_1 } \right)$ the c.f. can be written as

$$\begin{array}{lll} &&{\kern-32pt}g_{\text{auto,ring}} \left( {r;R_1 ,R_{\,2} } \right)\nonumber\\ &&{\kern-24pt}=\frac{1}{\left( {R_{\,2}^{\,2} -R_{\,1}^{\,2} } \right)}\left\{ {{\begin{array}{*{20}l} {R_{\,2}^{\,2} -R_1^{\,2} ,\;r=0} \\[3pt] {R_{\,2}^{\,2} g_{\text{auto}} \left( {r;R_{\,2} } \right)-R_1^{\,2} g_{\text{auto}} \left( {r;R_1 } \right)-2R_1^{\,2} \left[ {1-g_{\text{auto}} \left( {r;R_1 } \right)} \right],}\\{\kern12pt}{0<r<2R_1 } \\[3pt] {R_{\,2}^{\,2} g_{\text{auto}} \left( {r;R_{\,2} } \right)-2R_1^{\,2} ,\;2R_1 \leq r\leq \left( {R_{\,2} -R_1 } \right)} \\[3pt] {R_{\,2}^{\,2} g_{\text{auto}} \left( {r;R_{\,2} } \right)-2R_1^{\,2} g_{\text{cross}} \left( r \right),\;\left( {R_2 -R_1 } \right)<r<\left( {R_{\,2} +R_1 } \right)} \\[3pt] {R_{\,2}^{\,2} g_{\text{auto}} \left( {r;R_{\,2} } \right),\;\left( {R_{\,2} +R_1 } \right)\leq r<2R_{\,2} } \\[3pt] {0,\;\text{otherwise}} \\ \end{array} }} \right.\nonumber\\ ~\end{array}$$

(A.1)

and for $\left( {R_{\,2} -R_1 } \right)\leq 2R_{\,2}$ one obtains

$$\begin{array}{lll} &&{\kern-20pt}g_{\text{auto,ring}} \left( {r;R_1 ,R_{\,2} } \right)\nonumber\\ &&{\kern-6pt}=\frac{1}{\left( {R_{\,2}^{\,2} -R_1^{\,2} } \right)}\left\{ {{\begin{array}{*{20}l} {R_{\,2}^{\,2} -R_1^{\,2} ,\;r=0} \\[3pt] {R_{\,2}^{\,2} g_{\text{auto}} \left( {r;R_{\,2} } \right)-R_1^{\,2} g_{\text{auto}} \left( {r;R_1 } \right)-2R_1^{\,2} \left[ {1-g_{\text{auto}} (r;R_1 )} \right],}\\[3pt] {\kern12pt}{0<r\leq \left( {R_{\,2} -R_1 } \right)} \\[3pt] {R_{\,2}^{\,2} g_{\text{auto}} \left( {r;R_{\,2} } \right)-R_1^{\,2} g_{\text{auto}} \left( {r;R_1 } \right)-2R_1^{\,2} \left[ {g_{\text{cross}} \left( r \right)-g_{\text{auto}} \left( {r;R_1 } \right)} \right]},\\[3pt] {\kern12pt}{\left( {R_{\,2} -R_1 } \right)<r<2R_1 } \\[3pt] {R_{\,2}^{\,2} g_{\text{auto}} \left( {r;R_2 } \right)-2R_1^{\,2} g_{\text{cross}} \left( r \right),\;2R_1 \leq r<\left( {R_{\,2} +R_1 } \right)} \\[3pt] {R_{\,2}^{\,2} g_{\text{auto}} \left( {r;R_{\,2} } \right),\;\left( {R_{\,2} +R_1 } \right)\leq r<2R_{\,2} } \\[3pt] {0,\;\text{otherwise}} \\ \end{array} }} \right.\nonumber\\ ~\end{array}$$

(A.2)

In Eqs. A.1 or A.2 g _auto is given in Eq. 5 and from geometry the normalized intensity cross-correlation function g _cross of two disks of radius R ₁ and R ₂ ($R_{1} \ne R_{2})$ is

$$\label{A.3} g_{\text{cross}} \left( r \right)=\left\{ {{\begin{array}{*{20}l} {1,\;0\leq r\leq \left( {R_{\,2} -R_1 } \right)} \\[3pt] {\dfrac{\theta R_{\,2}^{\,2}}{\pi R_1^{\,2} }+\dfrac{\phi }{\pi }-z\left( {r;R_1 ,R_{\,2} } \right),\;\left( {R_{\,2} -R_1 } \right)<r<\left( {R_{\,2} +R_1 } \right)} \\[3pt] {0,\;\text{otherwise}} \\ \end{array} }} \right.$$

(A.3)

with

$$\label{A.4} z\left( {r;R_1 ,R_{\,2} } \right)=\left\{ {{\begin{array}{*{20}l} {\dfrac{hr}{\pi R_1^{\,2} },\;\phi <90} \\[5pt] {\dfrac{R_{\,2}^{\,2} }{\pi R_1^{\,2} }\cos \left( \theta \right)\sin \left( \theta \right),\phi =90} \\[3pt] {\dfrac{R_{\,2}^{\,2} }{\pi R_1^{\,2} }\cos \left( \theta \right)\sin \left( \theta \right)-\dfrac{h\sqrt {R_1^{\,2} -h^2} }{\pi R_1^{\,2} },\;\text{otherwise}} \\ \end{array} }} \right.$$

(A.4)

where $h=R_{\,2} \sin \left( \theta \right)=R_1 \sin \left( \phi \right)$, $\theta =\cos^{-1}\left( {\frac{R_{\,2}^{\,2}+r^{\,2}-R_1^2}{2R_{\,2} r}} \right)$, and $\phi =\cos^{-1}\left( {\frac{R_1^{\,2}+r^{\,2}-R_{\,2}^{\,2}}{2R_1 r}} \right)$. Equations A.1 or A.2 can be substituted into Eq. 4 instead of the normalized intensity c.f. of a disk, Eq. 5, to obtain the rotationally averaged c.f. of randomly distributed hard rings with a uniform intensity profile, g _ring(r), where the area fraction is defined as $\eta =\rho \pi R_{\,2}^{\,2}$ in Eq. 8. To estimate the number of rings in a given observation volume one has to compute the appropriate scaling constant. Following Eq. 1 the scaling constant is given as

$$ C_{\text{ring}} =\left[ {\frac{w^{\,2}}{N\pi \left( {R_{\,2}^{\,2} -R_1^{\,2} } \right)}-1} \right]\frac{1}{g_{\text{ring}} \left( {0;R_1 ,R_{\,2} } \right)} $$

(A.5)

and the un-normalized rotationally averaged intensity c.f. for hard rings can be computed by evaluating

$$ g_{N,\text{ring}} \left( r \right)=\left[ {\frac{w^{\,2}}{N\pi \left( {R_{\,2}^{\,2} -R_1^{\,2} } \right)}-1} \right]\frac{g_{\text{ring}} \left( {r;R_1 ,R_{\,2} } \right)}{g_{\text{ring}} \left( {r;R_1 ,R_{\,2} } \right)}\text{.} $$

(A.6)

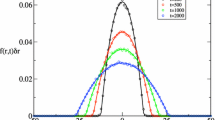
Equation A.6 was verified by simulating a test image (inset in Fig. 6a) of rings with inner radius R ₁ = 5 pixels and outer radius R ₂ = 10 pixels, w = 600 pixels, η = 0.30. These parameters were used to compute the theoretical intensity c.f. for hard rings. Figure 6a shows that the theoretical c.f. (solid line) fits the simulated data (open circles) quite well, verifying that the presented algorithm can also be applied to distributions of hard rings as expected. Following the presented algorithm one can obtain an expression for hard ring ICS by convolving Eqs. A.1 or A.2 with the autocorrelation of the Gaussian PSF:

$$\label{A.7} g_{\text{PSF}} \left( r \right)=C^\prime \int\limits_0^\infty {g_{\text{ring}} \left( {r^{\prime\prime} } \right)e^{-\frac{\left[ {r^2+\left( {r^{\prime\prime} } \right)^2} \right]}{4\sigma^2}}I_{\,0} \left( {\frac{r{r}^{\prime\prime}}{2\sigma^2}} \right){r}^{\prime\prime}d{r}^{\prime\prime}} \text{.}$$

(A.7)

Fig. 6

Panel a shows the rotationally averaged spatial correlation function of a simulated distribution (inset) of 350 homogeneous hard rings (open circles) compared to the theoretical intensity correlation function given by Eq. A.6 (solid line) for R ₁ = 5 pixels, R ₂ = 10 pixels, w = 600 pixels, and η = 0.30. The theoretical intensity correlation function fits the simulated data quite well, validating the developed theory. Bar = 36 pixels. b Depicts the best fit (solid line) to the intensity correlation function (open circles) of a simulated microscope image (inset). Simulation parameters are the same as in a with σ = 6 pixels. Hard ring image correlation spectroscopy (ICS) given in Eq. A.7 fits the intensity c.f. of the blurred image very well even though one cannot distinguish between disks or rings by eye. Hard ring ICS estimates the true number of rings N, the inner radius R ₁ as well as the outer radius R ₂ within 10%, holding σ constant. Bar = 6 σ

C ^′ is a scaling constant which can be estimated by fitting Eq. A.7 to the rotationally averaged intensity c.f. of a microscope image after deleting the g _PSF(0) datum. Figure 6b shows the best fit (solid line) to the intensity c.f. (open circles) of a simulated microscope image (inset). Hard ring ICS fits the intensity c.f. of the blurred image very well even though one cannot distinguish between disks or rings by eye. Hard ring ICS estimates the true number of rings within 5%. The estimate of the inner radius R ₁ is 0.78 σ and of the outer radius R ₂ is 1.66 σ. Both values are within 10%.

Note that the correlation function of two infinitely narrow rings can be calculated exactly and gives a simple form. The correlation function for two infinitely narrow rings, each of radius R, is obtained by writing each ring as a delta function and calculating the overlap integral. With one ring centered at the origin and the other at r, the correlation is

$$ g_{\text{auto},\delta-\text{ring}} \left( r \right)=\int\limits_0^\infty {\int\limits_0^{2\pi } {\frac{1}{4\pi^{2}\left( {r^\prime } \right)^{\,2}}\delta \left( {r^\prime -R} \right)\delta \left( {r^\prime -\left[ {r^2+R^{\,2}+2rR\cos \theta ^\prime } \right]^{1/2}} \right)r^\prime dr^\prime d\theta ^\prime } }, $$

(A.8)

where the prefactors are normalizations for the delta functions, and the law of cosines was used (θ ^′ is the angle between the point of interest in the plane and the line between ring centers). The integral over r ^′ is carried out by applying the first delta; to compute the integral over θ ^′, the second delta is treated as a function of θ ^′. Then

$$ \delta \left( {f\left( {\theta ^\prime } \right)} \right)=\sum\limits_{\theta _0^\prime } {\frac{\delta \left( {\theta -\theta_0^\prime } \right)}{\vert f^\prime \left( {\theta_0^\prime } \right)\vert }}, $$

(A.9)

where the summation is taken over the zeroes of f , where θ $^\prime = \theta_{0}^\prime$. When the rings overlap, there will be two zeroes of f , corresponding to the two points of overlap, and Eq. A.8 becomes

$$ g_{\text{auto},\delta -\text{ring}} \left( r \right)=\left\{ {\begin{array}{l} \dfrac{1}{2\pi^{2}R}\dfrac{1}{r\sin \theta_{0}^{\prime}},\;0<r<2R \\ 0,\;\text{otherwise}. \\ \end{array}} \right.$$

(A.10)

From geometry, $\sin (\theta_0^\prime )=[1-r^2/(4R^{\,2})]^{1/2}$, completing the derivation.