A phase-field study of the solidification process coupled with deformation

Abstract

Non-dendritic microstructures are generally obtained in metals after semi-solid deformation (deformation during solidification); however, dendritic growth is preferred without deformation. The fragmentation of dendrites is recognized as an essential contributing factor to non-dendritic microstructures. However, the underlying mechanism of fragmentation needs to be clarified in depth. It is infamously hard for researchers to carry out a direct observation of this process. Moreover, a comprehensive numerical survey of this process is not trivial. The present research reported a new method to model dendritic growth during semi-solid deformation. The motion and deformation of the solid coupled with liquid flow in the melt were treated as the two-phase flow because plastic materials could be formulated as non-Newtonian fluids. The vector-valued phase-field formulation and the self-constructed Navier–Stokes solver made it possible to simulate the growth, motion, deformation, fragmentation and agglomeration of two dendrites coupled with liquid flow in the melt. Computational results suggest that fragmentation can occur when the grain boundary is wet and penetrated by the melt, giving new supporting evidence to a previously proposed mechanism for the fragmentation of dendrites.

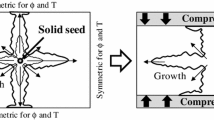
Graphical abstract

Appendices

Appendix 1 Solution sensitivity with respect to step and mesh sizes

The solution sensitivity with respect to different step and mesh sizes was investigated in this section. Initially, a seed was placed at the center of the domain with a size of 1.4 × 1.4 mm², which contained supersaturated Al-1 wt.% Cu alloy (Ω = 0.35). Solidification took place isothermally all the time, and ε₄ was set as 0.05. The mesh size near the interface (ϕ  = 0) was as 0.5 W₀ (1.4 × 2^–12 mm), and the step size was selected as 0.01 τ₀. The result at t = 0.54 s is shown in Fig. 10(a1)–(a3).

Figure 10(b1) compares the contours of ϕ  = 0 by only changing the step sizes (0.01 τ₀, 0.005 τ₀ and 0.0025 τ₀). Little difference could be found unless with an extremely close look (Fig. 10b2). This indicates a step size as small as 0.01 τ₀ guarantees a valid phase-field computation with this supersaturation.

Figure 10

A dendrite growing in a large domain (1.4 × 1.4 mm²; t = 0.54 s): (a1–a3) the phase field computed with the step size and mesh size (near the interface) selected as 0.01 τ₀ and 0.5 W₀, respectively; (b1-b2) a comparison of the contours ϕ  = 0 by only changing step sizes (black: 0.01 τ₀, red: 0.005 τ₀, green: 0.0025 τ₀). a2, a3 and b2 are the close-up images of the dashed box in a1, a2 and b1, respectively. The mesh near the interface is displayed in a3

Figure 10(a3) displays that the mesh size of 0.5 W₀ ensures the phase field varies smoothly from solid to liquid. Figure 11 demonstrates the result with the mesh size a level coarser (1.4 × 2^–11 mm = W₀) near the interface (other conditions kept unchanged), and the phase field did not have a smooth transition across the interface any more (Fig. 11c).

Consequently, in the current study, the mesh near the interface kept a size of 0.5 W₀. Considering the velocity computation (the step size Δt to solve Navier–Stokes equations should be limited by Δt ≤ h/|v| in every cell; h is the cell size [41]), the step size to solve phase-field equations should be adjusted dynamically (Sec. 2.4 in Ref. [41]) with an upper limit of 0.01 τ₀ (this limit is based on the test of alloy. For the pure metal, the limit should be selected as 0.05 τ₀, according to a similar test in Ref. [55]).

Figure 11

A dendrite growing in a large domain (1.4 × 1.4 mm²; t = 0.54 s). The mesh size on the interface was W₀. Other conditions kept the same as those of Fig. 10(a1–a3). b and c are the close-up images of the dashed box in a and b, respectively. The mesh near the interface is shown in c

Appendix 2 Numerical procedures to solve Navier–Stokes equations

The techniques to solve v and p from Navier–Stokes equations [Eqs. (8)–(9)] have already been discussed in Ref. [41]. Unlike Ref. [41], in the present study, v and p were solved separately rather than as a whole to enable a simpler preconditioner [77].

Linear system and preconditioner (without scaling)

v and p are approximated by finite element method:

$${\varvec{v}}{ = }\sum\limits_{j = 1}^{{N_{{\varvec{v}}} }} {Z_{j}^{v} {\varvec{\psi}}_{j}^{v} } {,}$$

(25)

$$p = \sum\limits_{j = 1}^{{N_{p} }} {Z_{j}^{p} \psi_{j}^{p} } ,$$

(26)

where v is discretized as a solution vector Z^v with N^v degrees of freedom (Z_j^v is the jth element in Z^v with the shape function ψ_j^v), and a similar definition is applicable for p. Equations (8)–(9) are discretized into a linear system [readers can refer to Eqs. (A.1), (A.5) and (A.10) in Ref. [41] for the space and time discretization]:

$$\left( {\begin{array}{*{20}c} {{\varvec{M}}^{vv} } & {{\varvec{M}}^{vp} } \\ {{\varvec{M}}^{pv} } & {{\varvec{M}}^{pp} } \\ \end{array} } \right)\left( {\begin{array}{*{20}c} {{\varvec{Z}}^{v} } \\ {{\varvec{Z}}^{p} } \\ \end{array} } \right) = \left( {\begin{array}{*{20}c} {{\varvec{R}}^{v} } \\ {{\varvec{R}}^{p} } \\ \end{array} } \right),$$

(27)

with

$$M_{ij}^{vv} = \left( {\rho \frac{{{\varvec{\psi}}_{j}^{v} }}{\Delta t},{\varvec{\psi}}_{i}^{v} } \right)_{S} + \left( {\mu {\varvec{D}}\left( {{\varvec{\psi}}_{j}^{v} } \right),\nabla {\varvec{\psi}}_{i}^{v} } \right)_{S} {,}$$

(28)

$$M_{ij}^{vp} { = }M_{ji}^{pv} = - \left( {\psi_{j}^{p} ,\nabla \cdot {\varvec{\psi}}_{i}^{v} } \right)_{S} {,}$$

(29)

$$\begin{aligned} R_{i}^{v} & { = }\left( {\rho \frac{{{\varvec{v}}^{\rm old} }}{\Delta t},{\varvec{\psi}}_{i}^{v} } \right)_{S} + \left( {\rho {\varvec{f}},{\varvec{\psi}}_{i}^{v} } \right)_{S} \\ & {\kern 1pt} \quad - \left( {\rho {\varvec{v}}^{\rm old} \cdot \left( {\nabla {\varvec{v}}^{{\text{old}}} } \right),{\varvec{\psi}}_{i}^{v} } \right)_{S} + \left\langle {\mu {\varvec{N}} \cdot \left( {\nabla {\varvec{v}}^{{\text{old}}} } \right)^{\rm{T}} ,{\varvec{\psi}}_{i}^{v} } \right\rangle_{{\Gamma_{2} }} , \\ \end{aligned}$$

(30)

where Δt is the step size. v^old is the velocity field that has been solved at the last step. (·, ·)_S and < ·, · > _Γ2 denote the integrations over the computational domain S and “do-nothing” boundary Γ₂ (p_m on Γ₂ is set as zero), respectively [Eqs. (A.2)-(A.3) in Ref. [41]]. If v has Dirichlet condition on the whole boundary, pressure is only defined up to a constant [41, 80, 94]. Here M^pp, R^p arise from the zero-pressure constraint at one point; therefore, M^ppZ^p = 0. Hence, Eq. (27) can be rewritten as

$${\varvec{M}}^{vv} {\varvec{Z}}^{v} \user2{ + M}^{vp} {\varvec{Z}}^{p} = {\varvec{R}}^{v} {,}$$

(31)

$${\varvec{M}}^{pv} {\varvec{Z}}^{v} = {\varvec{R}}^{p} {.}$$

(32)

Equation (31) is equivalent to

$${\varvec{M}}^{pv} {\varvec{Z}}^{v} \user2{ + M}^{pv} \left( {{\varvec{M}}^{vv} } \right)^{ - 1} {\varvec{M}}^{vp} {\varvec{Z}}^{p} = {\varvec{M}}^{pv} \left( {{\varvec{M}}^{vv} } \right)^{ - 1} {\varvec{R}}^{v} .$$

(33)

Equations (32) and (33) lead to

$$\left[ {{\varvec{M}}^{pv} \left( {{\varvec{M}}^{vv} } \right)^{ - 1} {\varvec{M}}^{vp} } \right]{\varvec{Z}}^{p} = {\varvec{M}}^{pv} \left( {{\varvec{M}}^{vv} } \right)^{ - 1} {\varvec{R}}^{v} - {\varvec{R}}^{p} {.}$$

(34)

Schur complement reads

$${\varvec{S}} = {\varvec{M}}^{pv} \left( {{\varvec{M}}^{vv} } \right)^{ - 1} {\varvec{M}}^{vp} {.}$$

(35)

S⁻¹ can be approximated by (S^*)⁻¹ as [41]

$${\varvec{S}}^{ - 1} \approx \left( {{\varvec{S}}^{*} } \right)^{ - 1} = \left( {{\varvec{L}}_{{\text{w}}}^{p} } \right)^{ - 1} + \left( {{\varvec{Q}}_{{\text{w}}}^{p} } \right)^{ - 1} ,$$

(36)

where

$$\left( {L_{{\text{w}}}^{p} } \right)_{ij} { = }\left( {\frac{\Delta t}{\rho }\nabla \psi_{j}^{p} ,\nabla \psi_{i}^{p} } \right)_{S} ,$$

(37)

$$\left( {Q_{{\text{w}}}^{p} } \right)_{ij} { = }\left( {\frac{1}{\mu }\psi_{j}^{p} ,\psi_{i}^{p} } \right)_{S} .$$

(38)

L_w^p should be assembled with the zero-pressure constraint at one point in the domain to ensure it is positive definite. Equation (34) is hard to solve in a straightforward way [77]; thus, it is preconditioned with Eq. (36):

$$\left[ {{\varvec{S}}\left( {{\varvec{S}}^{*} } \right)^{{{ - }1}} } \right]{\varvec{Y}}^{p} = {\varvec{M}}^{pv} \left( {{\varvec{M}}^{vv} } \right)^{ - 1} {\varvec{R}}^{v} - {\varvec{R}}^{p} {.}$$

(39)

After Y^p is solved with the CG solver from Eq. (39), Z^p can be recovered from Y^p:

$${\varvec{Z}}^{p} = \left( {{\varvec{S}}^{*} } \right)^{ - 1} {\varvec{Y}}^{p} ,$$

(40)

and then

$${\varvec{Z}}^{v} = \left( {{\varvec{M}}^{vv} } \right)^{ - 1} \left( {{\varvec{R}}^{v} - {\varvec{M}}^{vp} {\varvec{Z}}^{p} } \right).$$

(41)

Diagonal scaling

In the current study, the procedures described in “Linear system and preconditioner (without scaling)” of Appendix 2 cannot be directly applied. It is impractical to obtain the exact inverse of a matrix; thus, (M^vv)⁻¹, (L_w^p)⁻¹ and (Q_w^p)⁻¹ are represented by Cholesky decomposition. However, the variable viscosity in Eqs. (28) and (38) indicates that there are variations of orders of magnitude inside M^vv and Q_w^p. To guarantee effective decomposition, M^vv and Q_w^p are scaled with their diagonal elements, and the corresponding scaling vectors are defined as

$$d_{i}^{v} = \sqrt {M_{ii}^{vv} } {,}$$

(42)

$$d_{i}^{p} = \sqrt {\left( {Q_{{\text{w}}}^{p} } \right)_{ii} } ,$$

(43)

respectively, with a diagonal matrix

$${{\varvec{\Lambda}}}^{p} = {\text{diag}}\left( {d_{1}^{p} , \ldots ,d_{{N_{p} }}^{p} } \right).$$

(44)

The scaled linear system is

$$\left( {\begin{array}{*{20}c} {{\varvec{M}}_{{\text{I}}}^{vv} } & {{\varvec{M}}_{{\text{I}}}^{vp} } \\ {{\varvec{M}}_{{\text{I}}}^{pv} } & {{\varvec{M}}_{{\text{I}}}^{pp} } \\ \end{array} } \right)\left( {\begin{array}{*{20}c} {{\varvec{Z}}_{{\text{I}}}^{v} } \\ {{\varvec{Z}}_{{\text{I}}}^{p} } \\ \end{array} } \right) = \left( {\begin{array}{*{20}c} {{\varvec{R}}_{{\text{I}}}^{v} } \\ {{\varvec{R}}_{{\text{I}}}^{p} } \\ \end{array} } \right),$$

(45)

where

$$\left( {M_{{\text{I}}}^{vv} } \right)_{ij} = \frac{{M_{ij}^{vv} }}{{d_{i}^{v} d_{j}^{v} }},$$

(46)

$$\left( {M_{{\text{I}}}^{vp} } \right)_{ij} = \left( {M_{{\text{I}}}^{pv} } \right)_{ji} = \frac{{M_{ij}^{vp} }}{{d_{i}^{v} d_{j}^{p} }},$$

(47)

$$\left( {M_{{\text{I}}}^{pp} } \right)_{ij} = \frac{{M_{ij}^{pp} }}{{d_{i}^{p} d_{j}^{p} }},$$

(48)

$$\left( {R_{{\text{I}}}^{v} } \right)_{i} = \frac{{R_{i}^{v} }}{{d_{i}^{v} }},$$

(49)

$$\left( {R_{{\text{I}}}^{p} } \right)_{i} = \frac{{R_{i}^{p} }}{{d_{i}^{p} }}.$$

(50)

Subsequently, Eqs. (39)–(41) become

$$\left[ {{\varvec{S}}_{{\text{I}}} \left( {{\varvec{S}}_{{\text{I}}}^{*} } \right)^{{{ - }1}} } \right]{\varvec{Y}}_{{\text{I}}}^{p} = {\varvec{M}}_{{\text{I}}}^{pv} \left( {{\varvec{M}}_{{\text{I}}}^{vv} } \right)^{ - 1} {\varvec{R}}_{{\text{I}}}^{v} - {\varvec{R}}_{{\text{I}}}^{p} {,}$$

(51)

$${\varvec{Z}}_{{\text{I}}}^{p} = \left( {{\varvec{S}}_{{\text{I}}}^{*} } \right)^{ - 1} {\varvec{Y}}_{{\text{I}}}^{p} {,}$$

(52)

$${\varvec{Z}}_{{\text{I}}}^{v} = \left( {{\varvec{M}}_{{\text{I}}}^{vv} } \right)^{ - 1} \left( {{\varvec{R}}_{{\text{I}}}^{v} - {\varvec{M}}_{{\text{I}}}^{vp} {\varvec{Z}}_{{\text{I}}}^{p} } \right).$$

(53)

In Eq. (51),

$${\varvec{S}}_{{\text{I}}} = {\varvec{M}}_{{\text{I}}}^{pv} \left( {{\varvec{M}}_{{\text{I}}}^{vv} } \right)^{ - 1} {\varvec{M}}_{{\text{I}}}^{vp} {,}$$

(54)

$$\left( {{\varvec{S}}_{{\text{I}}}^{*} } \right)^{ - 1} = {{\varvec{\Lambda}}}^{p} \left( {{\varvec{L}}_{{\text{w}}}^{p} } \right)^{ - 1} {{\varvec{\Lambda}}}^{p} + \left( {{\varvec{Q}}_{{\text{w}}}^{p} } \right)_{{\text{I}}}^{ - 1} {,}$$

(55)

$$\left( {\left( {{\varvec{Q}}_{{\text{w}}}^{p} } \right)_{{\text{I}}} } \right)_{ij} { = }\frac{{\left( {Q_{{\text{w}}}^{p} } \right)_{ij} }}{{d_{i}^{p} d_{j}^{p} }}.$$

(56)

where (M_I^vv)⁻¹, (L_w^p)⁻¹ and (Q_w^p)_I⁻¹ are represented by Cholesky decomposition. Z^v and Z^p can be recovered by

$$Z_{i}^{v} = \frac{{\left( {Z_{{\text{I}}}^{v} } \right)_{i} }}{{d_{i}^{v} }},$$

(57)

$$Z_{i}^{p} = \frac{{\left( {Z_{{\text{I}}}^{p} } \right)_{i} }}{{d_{i}^{p} }}.$$

(58)

Appendix 3 Principal symbols

Matrices, vectors, tensors are in boldface, while scalars are in lightface.

ϕ:

Phase field

α:

Orientation field

α₄:

4α

c:

Solute concentration field

a₁, a₂:

Constants in phase-field model (Table 2)

a_s:

Anisotropy [Eq. (5)]

T:

Temperature

d₀:

Capillary length (Table 1)

W₀:

Spatial scale

τ₀:

Time scale

τ_ϕ:

Phase-field relaxation time (Table 1)

τ_α:

Orientation relaxation time

U_T:

Dimensionless temperature for pure metal (Table 1)

U_c:

Dimensionless concentration for alloy [Eq. (3)]

Y:

Thermal/solutal driving force (Table 1)

λ:

Coupling constant

ω:

Rotation rate

F = (F₁, F₂):

Vector-valued phase field [Eq. (7)]

W:

Diffuse interface thickness

P:

Mobility function [Eq. (6)]

v:

Velocity

p:

Pressure

σ_m:

Mean stress

p_m:

Mean pressure across the “do-nothing” boundary

t:

Time

c_∞:

Initial concentration in the melt

T_l–s:

Freezing range

ρ:

Density

I:

Unit diagonal tensor

f:

External force acting on a unit mass [Eq. (8)]

f_s:

Local solid fraction: f_s = (1 + ϕ)/2

$\dot{\boldsymbol\varepsilon }$:

Strain rate [Eq. (24)].

$\dot{\varepsilon }_{{{\text{eq}}}}$ , σ_eq:

Equivalent strain rate [Eq. (12)] and stress [Eq. (15)]

μ:

Dynamic viscosity

μ_l, μ_s, μ_s–l:

Dynamic viscosity in the liquid, solid [Eq. (14)], and within the s-l interface [Eq. (16)]

σ_y:

Yield strength

σ:

Stress tensor

s:

Deviatoric stress tensor

μ_max(s), μ_max(s-l) :

Cutoff viscosity in the solid and within the s-l interface [Eq. (17)]

ε₄:

Anisotropic strength

Ω:

Supersaturation

θ:

Dimensionless undercooling for the alloy

T_m:

Melting point

D:

Diffusivity (Table 1)

D_T:

Thermal diffusivity

D_cl:

Solute diffusivity in the liquid

ν:

Kinematic viscosity

k:

Solute partition coefficient

m :

Liquidus slope

μ_c, η, β, s:

Constants in the phase-field model, listed in Table 2

Γ:

Gibbs-Thomson coefficient

L:

Volumetric latent heat

c_p:

Volumetric specific heat

σ_sl, σ_gb:

Interfacial tensions on the s-l interface and grain boundary

ψ_j^v, ψ_j^p:

Shape functions for the jth degree of freedom in the finite element space of v and p [Eqs. (25)–(26)]

Z^v, Z^p:

Solution vectors of v and p [Eqs. (25)–(26)]

N^v:

The number of degrees of freedom in the finite element space of v

Z_I^v, Z_I^p:

Scaled Z^v, Z^p [Eqs. (52)–(53)]

d^v, d^p:

Scaling vectors [Eqs. (42)–(43)]

Λ^p:

Diagonal matrix constructed by d^p [Eq. (44)]

M^vv, M^vp, M^pv, M^pp:

Blocks in linear system Eq. (27) [Eqs. (28)–(29)]

M_I^vv, M_I^vp, M_I^pv, M_I^pp:

Scaled M^vv, M^vp, M^pv, M^pp [Eqs. (46)–(47)]

R^v, R^p:

Right hand sides in linear system Eq. (27)

R_I^v, R_I^p :

Scaled R^v, R^p [Eqs. (49)–(50)]

S:

Schur complement [Eq. (35)]

(S^*)⁻¹:

Approximation to S^-1 [Eq. (36)]

L_w^p, Q_w^p:

Matrices used to evaluate S* [Eqs. (37)–(38)]

(Q_w^p)_I:

Scaled Q_w^p [Eq. (56)]

Y^p:

Solution vector in linear system Eq. (39)

Y_I^p:

Solution vector in scaled linear system Eq. (51)

S_I:

Scaled Schur complement [Eq. (54)]

(S_I^*)^-1:

Approximation to (S_I)^-1 [Eq. (55)]

Δt:

Step size for solving the Navier–Stokes equations

S:

Computational domain

Γ₂:

“Do-nothing” boundary

N:

Outward unit normal to the boundary

(·, ·)_S, <·, ·>_Γ2:

Integration over the domain and “do-nothing” boundary

D(·):

Operator defined by Eq. (11)

::

Double dot product between two second-order tensors [Eq. (13)]

(x₁, x₂), (X₁, X₂):

Axes of the local and global frame (Fig. 1 in Ref. [55])