Introduction

Utilizing wastestreams for microalgal cultivation is known to significantly reduce the overall production cost and ultimately increases the sustainability of microalgae cultivation (Markou et al. 2019). Wastestreams may however be contaminated with undesired substances, compromising the microalgal quality and use ability (Shih and Yan 2016). To avoid such problems, extracting of specific components from wastestreams can be a solution. In this context, element phosphorus (P) is particularly relevant, as it is an essential component of culture media and fertilizers used in the agricultural industry. Due to the new regulations of (EU) 2019/1009 (Huygens et al. 2019), raw materials such as struvite recoverable from the treatment of wastewater could potentially be used as safe material. Struvite is a crystal form generated by precipitation of magnesium-ammonium-phosphorus (MAP) with the formula NH4MgPO4·6H2O. In this context, struvite is an interesting component, as it is already included as a commercial fertilizer in the Registration, Evaluation, Authorisation and Restriction of Chemicals (REACH) EC No. 1907/2006 and in the 2023-amendment to the EU eco-regulation/inclusion. Europe is one of the biggest struvite producers, currently producing up to 1250 tonnes of the 1350 tonnes struvite annually in a quality suitable for direct use as a fertilizer or as a secondary raw material in fertilizers industry (Muys et al. 2021). Urban wastewater, industrial wastewater, manure, and livestock slurries are exploited as input aqueous wastes for struvite production (Huygens et al. 2019).

Despite its well-established agricultural applications, some possible obstacles must be overcome when using struvite for microalgae cultivation. With a solubility constant of 10–13.26 (Shih and Yan 2016), struvite has a very low solubility of 160 mg L−1 (Bhuiyan et al. 2007). Because PO4 availability through in a growth medium depends on its solubility, this could lead to a limitation in microalgal growth. In addition to PO4, magnesium (Mg) and ammonium [\({\mathrm{NH}}_{4}^{+}\)] are also a component of struvite, which are released through struvite dissolution. Ammonium ion carries a positive charge and forms an equilibrium with ammonia [NH3] in the aqueous solutions (Ullmann's encyclopedia of industrial chemistry 2003). This equilibrium depends on the pH of solution, with a pKa [\({\mathrm{NH}}_{4}^{+}\)/ NH3] of 9.25 (Markou et al. 2016). The bicarbonate/carbonate buffer with a pH of 9.4, used for cultivation of Arthrospira platensis (Aiba and Ogawa 1977), causes a shift from \({\mathrm{NH}}_{4}^{+}\) to NH3. Contrary to \({\mathrm{NH}}_{4}^{+}\) uptake regulated by the cells, NH3 can passively diffuse through the cell membrane and accumulate in the cells at high concentrations Click or tap here to enter text., resulting in negative impact on cell growth and the functions of the photosynthetic apparatus (Boussiba 1991; Markou et al. 2016).

To date, few studies have tested the possibility of struvite utilization to replace the mineral phosphorous components in the A. platensis growth medium. For example, struvite from biogas anaerobic digestate origin resulted in the same biomass yield and biochemical composition of A. platensis when compared to the control medium (Markou et al. 2019). In addition, Chlorella vulgaris showed higher growth rates in struvite-based media (as a replacement for K2HPO4, KH2PO4, MgSO4·7H2O and NaNO3) compared to growth rates obtained in Bold’s Basal Medium (BBM) (Moed et al. 2015). Thus, using struvite as a P-replacement component also affects the supplementation of magnesium and ammonia concentrations in the growth medium. Therefore, the present study aimed to investigate the effect of different struvite sources and pre-treatment on the growth performance and C-phycocyanin (C-PC) production in A. platensis. Arthrospira platensis, is a photoautotrophic blue-green microalga (cyanobacterium), which was chosen in the present study because it is characterized by high adaptability and predominance in native habitat conditions, has many pharmaceutical applications (Shao et al. 2019), and contains relatively high content of C-phycocyanin (Usharani et al. 2012). The biliprotein C-PC is of importance due to its application in food supplements, food colourants, pharmaceuticals and cosmetic products (Sánchez et al. 2003; Soni et al. 2017). Any negative effects of culture media on C-PC content can potentially outweigh positive cost or sustainability effects. As bovine urine is considered a highly sustainable P-source (Papa et al. 2017), recovery was done in the present study through controlled precipitation of MAP in the form of struvite by adding magnesium oxide [MgO] as shown in Eq. 1 (Huang et al. 2014; Stolzenburg et al. 2015; Ariyanto et al. 2017). Bovine urine was used as the removal of phosphate from agricultural wastestreams establishes an easy way for farmers to combat overfertilization and to open opportunities to use struvite as an alternative fertilizer or as a product to sell. In this study, pure commercially available struvite was compared with the self-produced one. Additionally, the effect of microwave pre-treated struvite was evaluated. With struvite as a recovered pure substance, new possibilities open for the utilization of nutrients from agricultural waste streams (Papa et al. 2017).

$${Mg}^{2+}+{NH}_{4}^{+}+{H}_{n}{PO}_{4}^{3-n}+{6H}_{2}O\to {MgNH}_{4}{PO}_{4}\cdot {6H}_{2}O+{nH}^{+}$$
(1)

Materials and methods

Microalgal strain and growth conditions

Arthrospira platensis (UTEX 2340) was obtained from the culture collection of algae at the University of Texas. For upscaling from stock cultures, two 1-L Schott Duran Erlenmeyer flasks were prepared. Arthrospira platensis was cultivated and incubated in the microalgae cultivation container at Alfred Wegener Institute (AWI), Bremerhaven, where four different culture media were used. For inoculum preparation and as a control group, the standard SAG-spirul culture medium was used, with the recipe recommended by the culture collection of algae at the Georg-August-University of Göttingen (Experimental Phycology and Culture Collection of Algae at the University of Goettingen 2008). The modified culture media were based on the SAG spirul medium recipe, with mainly changes made to the phosphorus source, exchanging dipotassium phosphate with struvite.

Struvite source and pre-treatment

Two different sources of struvite were used in the present study. As a first struvite source, synthesized struvite was obtained from Merck (Product-Nr.: 529,354). The second struvite source was that recovered from bovine urine. For this purpose, urine was collected over the course of a week at the Friedrich-Löffler-Institut in Braunschweig as part of food experiments on cow feed. In this way, a total of approximately 141.2 L of bovine urine was set aside for struvite extraction, divided into six canisters. Struvite was obtained by adding MgO based on the existing stoichiometric ratios of molar weights of the three components forming struvite, i.e. NH4, MgO and PO43-. Afterwards, the suspensions were stirred for approximately 30 min with a stirring attachment on an accumulator drill, then the suspensions were rested for 30 min allowing the sedimentation of struvite. The supernatant was rinsed out of the containers and the concentrated struvite was finally filtered and dried at 60 °C for 20 h. As a final step, organic residues were removed by incinerating the sediments at 500 °C in a muffle furnace for five hours.

Culture media

The growth media were prepared in two solutions, which were separately autoclaved, to be subsequently combined. SAG(-) culture media is based on the spirulina medium (SAG-spirul) recipe. For the modified media, dipotassium phosphate was replaced with struvite as a new phosphate source. In the case of CS(+) culture media, the self-produced struvite-substrate was used. Based on the chemical composition (IBEN GmbH), 10.9 g of struvite substrate was added to act as a sufficient phosphate source for 4 L of culture media. Regarding S(-) and S(+) culture media, 2.8 g of commercially available struvite was used. These calculations were based on the molar mass of the chemical components. 0.5 g of dipotassium phosphate, as specified in the SAG-spirul recipe for 1 L of culture medium, can be divided into 0.273 g of phosphate and 0.112 g of potassium. 0.271 g of phosphate can be provided by 0.7 g of struvite in its hydrated form, which results in 2.8 g of struvite hexahydrate for 4 L of culture media. As the struvite provides not only phosphate, but also magnesium, 0.2 g L−1 of magnesium sulphate was removed from the medium recipe.

To complement the missing 0.112 g potassium and 0.078 g sulphate per litre of culture medium, 0.25 g potassium sulphate were added to the already required 1.0 g L−1 of potassium sulphate in S(-), S(+) and CS(+) culture media, resulting in 5.0 g of potassium sulphate to be added in total, instead of 4.0 g. The chemical components were weighed with an analytical balance (δm: ± 0.1 mg) (Sartorius, Germany) according to the values presented in Table 1 and added to the respective quantities of deionized water in 2 L Kimble Kimax glass bottles (DWK Life Sciences, USA).

Table 1 A) Chemical components of the culture media with their respective modifications. SAG(-) is based on the spirulina medium recipe (SAG-spirul), with an identical chemical composition. For CS(+), bovine urine struvite substrate was used as an alternative phosphate source, including microwave treatment in 0.8 M sodium hydroxide solution. S(-) refers to commercial struvite as an alternative to dipotassium sulphate, whereas S(+)includes microwave digestion of commercial struvite. In CS(+), S(-) and S(+), magnesium sulphate was removed, and potassium sulphate was added to substitute the missing potassium. All culture media were prepared in two solutions and were autoclaved separately to be combined before the inoculation. B) Chemical components of the micronutrient solution
Full size table

Pre-treatment of struvite-based growth media

Based on the results of struvite analysis, microwave digestion was used in the preparation of CS(+) and S(+) culture media. As to have a sufficient amount of pre-treated solution for 4 L of culture media, 200 ml of solution were used. For CS(+) pre-treatment, 15.53 g of bovine urine struvite was added to 200 ml of 0.8 M NaOH solution. For S(+) pre-treatment, 4 g of commercial struvite were added to 200 ml of 0.8 M NaOH solution as well. Then the solutions were heated in a microwave (Severin Elektrogeräte GmbH, Germany) at 460 W for 8 min. The solutions were then filtered with a medium fast qualitative folded filter paper (Macherey–Nagel, Germany) before adding 140 mL of each solution to their respective culture media solution I. The culture media were separately autoclaved and stored until use.

Upscaling process of A. platensis

For upscaling from the stock cultures (Fig. 1), two 2 L Kimble Kimax glass bottles were prepared. Further, 600 mL of SAG(-) medium was inoculated with 200 mL of stock culture (Fig. 1). Over a period of one week, the inoculum incubated at a temperature of 22 ± 1 °C with a 12:12 (light/dark) cycle, provided by a daylight fluorescent tube.

Fig. 1
figure 1

Exemplary process describing the upscaling from starter cultures to final inoculation of the cultures used in the current experiments

Full size image

Experimental setup

Cultivation of A. platensis was carried out in 1 L Schott Duran and 1 L Kimble Kimax Erlenmeyer flasks. The Erlenmeyer flasks were sterilized via autoclaving. Prior to inoculation, a photometric measurement of the inoculum using optical density was conducted to calculate the optimal volume to be inoculated. After inoculation, the flasks were sealed with sterile gauze balls, kept in an oval shape through a cotton mesh, and incubated at 29 ± 2 °C, 35 cm from a LED-light source (SKY [65000 K; PPE: 1.6 μmol J−1]; LEDaquaristik, Hövelhof, Germany) with a 14:10 (light/dark) cycle. After one week of incubation, the cultures were moved closer to 25 cm. The cultures were cultivated on different shelves at different light intensities [values in PPFD]. In the upper shelf with 41 μmol photons m−2 s−1 and in the lower shelf with 66 μmol photons m−2 s−1 for a distance of 35 cm. After the cultures were moved closer to 25 cm, average light intensities were 55 μmol photons m−2 s−1 in the upper shelf and approximately 93 μmol photons m−2 s−1 in the lower one. For further analysis, 15 mL of each culture were taken under sterile conditions. Samples were taken twice a week, in total over 42-day period, directly analysed for optical density, and subsequently stored at -80 °C till further measurements.

Cultivation of A. platensis in new culture media

For different culture media, three flasks were inoculated each, for a total of 12 inoculated flasks. For each flask, 500 mL of the respective culture medium was inoculated with 100 mL of the previously upscaled A. platensis (UTEX 2340). Flasks 1–6 were stored on the upper shelf, while 7–12 on the lower shelf. Further analysis with a regression fit and the Gompertz fit was conducted to determine the specific generation time and the maximum generation time of the 12 cultures.

Measurements and analysis

Photometric measurements

For the photometric measurements of optical density, 1 mL was taken from the 15 mL culture samples and transferred to a 1.5 mL semi-micro cuvette (Brand, Germany). Measurements of the absorbance spectrum between 450 and 750 nm were carried out using S50 UV/Vis Biochrom Libra spectrophotometer. The absorbance values of wavelengths at 480 nm, representing the absorbance of beta-carotene (Barsanti 2014), and 700 nm, representing the absorbance of chlorophyll-a in the red region (Koehne and Trissl 1998; Barsanti 2014), were then recorded. For each sample, their respective typical culture medium was used as a blank. In the event that the absorbance value exceeded 1.0, the measured sample was diluted appropriately, then measurements were corrected for the dilution factor.

C-PC concentration was measured using ET2020 handheld photometer (Econix, University of Texas, USA) via the absorbance and transmittance values at the C-PC absorption maximum (λmax = 620 nm) (Glazer et al. 1973; Boussiba and Richmond 1979). For this purpose, 2 mL of the 15 mL culture samples were taken and transferred to the 2 mL cuvette. The absorbance and transmittance values were then recorded as described in the previous paragraph.

Dry weight and biomass productivity

A correlation formula between optical density and dry weight was generated. Briefly, 50 mL of A. platensis (UTEX 2340) stock was taken to prepare a dilution series (Supplementary data, Table S1).

The measured dry weight [DW] was plotted against the absorption at 480 nm [OD480] (Fig. S1, Supplementary data). With a linear fit, the correlation between the two parameters was evaluated and confirmed with a Pearson´s R of 0.993.

Biomass productivity [BP (g DW L−1 day−1)] was calculated from values of dry weight [DW (g L−1)] at the early stage of exponential growth phase [tE (day)] and at the late stage of the exponential growth phase [tL (dY)] using the following formula (Abomohra et al. 2013; El-Sheekh et al. 2013).

$${BP}_{{t}_{L}-{t}_{E}}=\left({DW}_{{t}_{L}}-{DW}_{{t}_{E}}\right)*{\left({t}_{L}-{t}_{E}\right)}^{-1}$$

The exponential growth phase was defined by a period from the early stage [tE = 6 day] to the late stage of exponential growth [tL = 35 day].

C-phycocyanin content and productivity

With Beer´s law, C-PC concentration was calculated from the retrieved absorbance values at a wavelength of 620 nm. Beer´s law is defined by the following formula with the parameters of absorbance [A], molar absorptivity [ε (L mol−1 cm−1)], the path length of the sample [b (cm)], and the concentration [c (mol L−1)] (Dean and Lange 2001; Worsfold 2005).

$$A=\varepsilon bc$$

To calculate the concentration in mg ml−1, the molar absorptivity [ε] must be converted into [E1%], which represents the value of absorbance measured in 1% (= 1 g per100 mL) solution. The relationship of [E1% (L g−1 cm−1)] to molar absorptivity [ε (L mol−1 cm−1)] and the molecular weight [MW] can be stated as follows (Fasman 1989),

$$10\varepsilon ={E}^{1\%}*MW$$

This correlation, applied to Beer´s law, can be used to calculate the concentration [c (mg mL−1)] of the component in a solution using the following formula,

$$c=\frac{A}{{E}^{1\%}}10$$

where \({E}_{620}^{1\%}\) values of C-PC are stated at 60 and 72 L g−1 cm−1 (Fasman 1989) or in a range from 60 to 77 L g−1 cm−1 (Boussiba and Richmond 1979; Furuki et al. 2003). These values are dependent on several factors, like pH and the chemical environment of the sample (Worsfold). This range of \({E}_{620}^{1\%}\) values made it necessary to find the right \({E}_{620}^{1\%}\) value for samples taken during the experiment. Thus, a second approach was necessary.

Using Bennett and Bogorad’s formula, C-PC concentration [\({c}_{CPC}\) (mg mL−1)] of A. platensis samples could be determined through measurement of absorbance at 620 nm [OD620], and 652 nm [OD652] and constant factors of –0.474 and 5.34 (Bennett and Bogorad 1973; Safari et al. 2019).

$${c}_{CPC}=\frac{OD_{620}-0.474*OD_{652}}{5.34}$$

By comparing the results of both approaches, the optimal value of \({E}_{620}^{1\%}\) was determined. As the concentration of several pigments found in algae was shown to be proportional to absorption with absorbance values below 0.8, Beer´s law was only applied on sufficiently diluted samples (Richards and Thompson 1952). In Table S2 (Supplementary data), the results of two methods used for calculation of C-PC concentration [\({c}_{CPC}\) (mg mL−1)] in A. platensis stock are presented. Using Bennett and Bogorad´s formula, a mean \({c}_{CPC}\) of 0.45 ± 0.003 mg mL−1 was obtained (Bennett and Bogorad 1973). Using Beer´s law and a value of 64 L g−1 cm−1 for \({E}_{620}^{1\%}\), a mean \({c}_{CPC}\) of 0.45 ± 0.006 mg mL−1 was obtained (Worsfold et al. 2005). When comparing the results of both methods, a value of 64 L g−1 cm−1 for \({E}_{620}^{1\%}\) was found to sufficiently represent the in vivo \({c}_{CPC}\) in the analysed samples (Table S3, Supplementary data). This value lies within the range of literature values for \({E}_{620}^{1\%}\) between 60 and 77 L g−1 cm−1 (Boussiba and Richmond 1979; Fasman 1989; Furuki et al. 2003).

C-PC productivity [CPC-P (mg L−1 day−1)] was calculated from the values of C-PC concentration [c (mg L−1)] at an early stage of the exponential growth phase [tE (day)] and at a late stage of the exponential growth phase [tL (day)], with the following formula,

$${CPC-P}_{{t}_{E}-{t}_{L}}=\left({c}_{{t}_{L}}-{c}_{{t}_{E}}\right)*{\left({t}_{L}-{t}_{E}\right)}^{-1}$$

To get a more accurate estimate of the C-PC productivity per DW, the values of DW had to be adjusted. With the subtraction of the intercept value [2.175 (± 0.017) g L−1], displayed in Fig. S1, an adjusted DW was determined. With this new value for DW, the adjusted C-PC content and C-PC productivity per DW were calculated.

Statistical analysis

Regression fit

The specific growth rate [μ] was calculated by linear regression, considering a period from day 4 to day 20. To describe the exponential growth of the cultures, the raw data was transformed into the logarithm of the relative population size against time (Zwietering et al. 1990; Allen and Waclaw 2019). According to this, the logarithm of the respective absorbance value of the density measurement, divided by the absorbance value of the density measurement of day 0, was plotted against time in days.

$$t\left[d\right]=\mathrm{ln}\left(\frac{{OD}_{n}}{{OD}_{0}}\right)$$

From these values, the formula of a regression line was calculated, and a growth rate [μ] was obtained from it. This is possible since the specific growth rate [μ] is equal to the slope coefficient.

$$\ln\left[\frac{{OD}_n}{{OD}_0}\right]=\mu\;t\lbrack d\rbrack+c$$

Using the specific growth rate [μ], mean doublings per day [κ] were calculated (Lakshmanan et al. 2013). Based on [\(\mu\)], the mean generation time [t2] was calculated as well (Vonshak 1997; Barsanti 2014). The respective standard error of the values calculated in this way was determined according to Gaussian error propagation.

Determination of doublings per day [κ]:

$$\kappa =\frac{\mu }{\mathrm{ln}\left(2\right)}$$

Error propagation on [κ]:

$${\sigma }_{[\kappa ]}=\frac{{\sigma }_{\mu }}{\mathrm{ln}\left(2\right)}$$

Calculation of the doubling time [t2]:

$${t}_{2}=\frac{\mathrm{ln}\left(2\right)}{\mu }$$

Error propagation on [t2]:

$$\sigma_{\lbrack t_2\rbrack}=ln\left(2\right)\;\sigma_{\lbrack\mu\rbrack}\sqrt{\left(\frac1{\left(\mu^4\right)}\right)}$$

Gompertz fit

To determine the maximum specific growth rate [μmax] over a period from day 4 to day 42, a sigmoidal fit was applied to the data. Similarly to the regression model, the raw data was first transformed via calculating the logarithm of the respective absorbance value of the density measurement, divided by the absorbance value of the density measurement of day 0. Then the results were plotted against time in days.

$$t\left[day\right]=\mathrm{ln}\left(\frac{{OD}_{n}}{{OD}_{0}}\right)$$

Now the Gompertz-Fit was used to model the growth curve of each culture using the following formula,

$$y=a\;e^{\left({-e}^{\left(-k\bullet\left(x-xc\right)\right)}\right)}$$

To transform the coefficients into biological parameters, the formula used for the Gompertz fit was transformed into the following formula, called the Zwietering modification (Zwietering et al. 1990; Tjørve and Tjørve 2017),

$$y\left(t\right)=A\;e^{\left({-e}^{\left(\frac{e\mu_{max}}A\left(\lambda-t\right)+1\right)}\right)}$$

The following transformations for the individual parameters were derived from this. The asymptote [a] can be equated to the carrying capacity [A],

$$a=A$$

From the coefficient [xc] and the growth coefficient [k], the lag time [λ] can be determined by transformation,

$$\lambda = \frac{\left(\left(k xc\right)-1\right)}{k}$$

Error propagation on [λ] according to Gaussian error propagation was calculated per the following formula,

$${\sigma }_{[\lambda ]}=\sqrt{\left(\frac{\left({{\sigma }_{[xc]}}^{2}\right)+\left({{\sigma }_{[k]}}^{2}\right)}{\left({k}^{4}\right)}\right)}$$

From the asymptote [a] and the growth coefficient [k], the maximum specific growth rate [μmax] can be calculated,

$${\mu }_{max}= \frac{\left(a k\right)}{e(1)}$$

Error propagation on [μmax] was calculated as follows,

$${\sigma }_{\left[{\mu }_{max}\right]}=\frac{\left(\sqrt{\left({a}^{2}\right)\left({{\sigma }_{k}}^{2}\right)+\left({k}^{2}\right)\left({{\sigma }_{a}}^{2}\right)}\right)}{{e}^{(1)}}$$

Using the maximum specific growth rate [μmax], maximum mean doublings per day [κmax] and the maximum generation time [t2max] were calculated. This was done using the same formulas as for calculating the mean generation time [t2]. The respective standard error of the values was determined according to Gaussian error propagation.

Pearson’s correlation, ANOVA and post-hoc testing

To analyse linear correlations between two datasets, Pearson´s correlation test was used (Benesty et al. 2009; Schober et al. 2018). With Pearson’s correlation test, a dimensionless coefficient of the covariance was determined, varying in a range from -1 to + 1. For interpretation of the coefficient, it was assumed that a value of < 0.1 is an indicator of negligible, and one of > 0.9 for strong linear dependency in the case of positive correlation, with negative values for negative correlation (Schober et al. 2018).

For analysis of variance, one-way ANOVA testing was used to determine possible significant differences in between groups of samples. For the analysis with ANOVA, the significance level was set to P < 0.05. In case of significances, a post-hoc test was performed for multiple comparisons (Abdi and Williams 2010).

As a pairwise comparison test, the Tukey test was used, which is based on the Studentized Range sampling distribution developed by Gosset (Abdi and Williams 2010). With this distribution a coefficient, qobserved is calculated, which is compared with a so-called critical value. This value depends on the chosen α-level for the P-value, in this case P < 0.05, but also on the degrees of freedom, and the number of means being tested. The advantage of this method of calculating significance is that the type I error is equal to the selected alpha level Abdi and Williams 2010; Salkind 2010).

In addition, a pairwise t-test was utilized for the comparison of two datasets of equal or similar sample-size. The test was conducted first assuming an equal variance and then with the Welch correction without assuming an equal variance (Sakai 2016). At a P-value lower than 0.05, the compared means of the selected datasets are significantly different (Posten 1984).

Results

Growth of A. platensis

The results of growth, beta-carotene, and chlorophyll-a showed insignificant differences in A. platensis (UTEX 2340) between the four studied culture media [SAG(-), CS(+), S(-), and S(+)] with a P-values above 0.05 (Fig. 2).

Fig. 2
figure 2

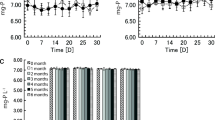
Growth curve of A. platensis cultures in 1 L Erlenmeyer Flasks using different culture media over a 42-days period. Each plotted point shows the mean of six measurements of the absorbance at 480 nm [A] and 700 nm [B]. For each culture medium, three flasks were measured twice for each time point. To visualize the growth, a logistic fit was used to connect the plotted points. n = 72 per treatment, F- and P-value for dataset of A: F = 0.295; P = 0.829, F- and P-value for dataset of B: F = 0.381 P = 0.766

Full size image

Growth analysis with regression fit

The results of the linear regression showed insignificant effect of struvite-based media on the growth of A. platensis cultivated in different growth media [P480 = 0.498; P700 = 0.373; P620 = 0.435 (Table 2)]. The specific generation times between SAG (-) to CS (+), S (-) and S (+) for A. platensis decreased by 6.3% [SAG (-)/CS (+)], 8.0% [SAG (-)/S (-)] and 12.2% [SAG (-)/S ( +)] for measurements of absorption from carotenoids [480 nm] (Table 2 [A]). For measurements of chlorophyll [700 nm] it decreased by 8.6% [SAG (-)/CS (+)], 7.4% [SAG (-)/S (-)] and 12.8% [SAG (-)/S (+)] (Table 2 [B]). Between the untreated [S (-)] and treated [S (+)] struvite medium the specific generation time decreased by 4.5% [S (-)/S (+)] for measurements of carotenoids [480 nm] and by 5.8% [S (-)/S (+)] for measurements of chlorophyll [700 nm]. For the measurements of absorption at 620 nm, the specific generation times are 3.85% [SAG (-)/CS (+)], 1.9% [SAG (-)/S (-)] and 10.1% [SAG (-)/S (+)] lower (Table 2 [C]). Between the untreated [S (-)] and treated [S (+)] struvite culture growth medium the specific generation time decreased by 8.4% [S (-)/S (+)].

Table 2 Results of linear regression from absorbance measurements at 480 nm [A], 700 nm [B] and 620 nm [C] taken between day 4 and 20 of the cultivation period and further calculated parameters describing growth of A. platensis in four different culture media [SAG(-), CS(+), S(-), S(+)]. As the slope is equal to the specific growth rate [μ], it was used to calculate doublings per day [k], and the generation time [T2]. Also shown is the standard error (δ [ ±]) for each value. As well as F and P-values calculated via ANOVA testing to find statistically significant differences between the specific generation times [T2] of different used culture media for each respective measurement of absorbance [480 nm, 700 nm, 620 nm]
Full size table

Growth analysis with Gompertz fit

The transformed data from day 4 to 42 was modelled with the Gompertz fit with great accuracy, visible in sufficient adj. R-square values, with values not falling below 0.976 (Table S4, Supplementary data). This data were then plotted in Figs. 3, 4, and 5. Results showed that the maximum production of beta-carotene and maximum production of chlorophyll-a were not significantly different (Table S5, supplementary data).

Fig. 3
figure 3

Gompertz fit to determine the maximum growth rate [μmax], lag-time [λ] and growth capacity [A] for cultures grown in four culture media [SAG(-), CS(+), S(-), S(+)]. Each data point shows the mean of the values derived from the six absorbance measurements at 480 nm [A] and 700 nm [B] for each time point over a period from day 4 to day 42. For the fit, the logarithm of each respective absorbance value of the conducted density measurements was divided by the absorbance value of the density measurement at day 0, which was then plotted against time in days. n = 66 per treatment, F- and P-values for dataset of A: F = 0.5862; P = 0.62455, F- and P-values for dataset of B: F = 0.56926 P = 0.63572

Full size image
Fig. 4
figure 4

Comparison of maximum generation time [T2max] of A. platensis cultivated in different culture media [SAG(-), CS(+), S(-), S(+)].The maximum generation time was calculated by generating a Gompertz fit formula with absorbance measurements at 480 nm,700 nm [A] and 620 nm [B] taken between days 4 and 42 of the cultivation period. The measurements of absorbance at 480 nm indicate the growth of A. platensis regarding the production of beta-carotene, and at 700 nm the production of chlorophyll-a. The measurements of absorption at 620 nm indicate the growth of A. platensis according to the C-phycocyanin production of the microalgae. Each boxplot represents the maximum generation times of three cultures per growth medium. The whiskers show the respective standard deviation with a coefficient of 1.5. n = 3 per boxplot, F- and P-value for dataset of A480: F = 2.88608; P = 0.10252, F- and P-value for dataset of A700: F = 2.86753; P = 0.1038, F- and P-value for dataset of B: F = 1.20107 P = 0.36968

Full size image
Fig. 5
figure 5

Plot of the maximum generation time [T2max] against the time it was reached. The values for each measurement of absorption of pigments at their respective wavelengths are represented by colour (beta-carotene [480 nm], red; Chlorophyll-a [700 nm], green; C-phycocyanin [620 nm], blue). Each growth medium is plotted separately to allow comparisons when the maximum generation time of each pigment is reached

Full size image

The maximum generation times between SAG(-) to CS(+), S(-) and S(+) for A. platensis were lower by 3.1% [SAG(-)/CS(+)], 12.7% [SAG(-)/S(-)] and 17.7% [SAG(-)/S(+)] than that in SAG(-) control for measurements of absorption from carotenoids [480 nm] (Table 3 [A]). For measurements of absorption from chlorophyll [700 nm], it was 4.7% [SAG(-)/CS(+)], 11.4% [SAG(-)/S(-)] and 17.6% [SAG(-)/S(+)] lower (Table 3 [B]). Between the untreated [S(-)] and treated [S(+)] struvite culture growth medium, the maximum generation time decreased by 5.8% [S(-)/S(+)] for measurements of absorption from carotenoids [480 nm] and by 7.0% [S(-)/S(+)] for measurements of absorption from chlorophyll [700 nm]. For the measurements of absorption at 620 nm, the specific generation times were 5.1% [SAG(-)/CS(+)], 7.1% [SAG(-)/S(-)] and 14.6% [SAG(-)/S(+)] lower (Table 3 [C]). Between the untreated [S(-)] and treated [S(+)] struvite culture growth media, the specific generation time decreased by 8.0% [S(-)/S(+)]. With further analysis via ANOVA testing, no significant difference in the growth was detected for each wavelength between the different growth media (P-values at 480 nm = 0.103; at 700 nm = 0.104; and at 620 nm = 0.370).

Table 3 Results of Gompertz fit on absorbance measurements at 480 nm [A], 700 nm [B] and 620 nm [C] taken between days 4 and 42 of the cultivation period and further calculated parameters describing growth of A. platensis in four different culture media [SAG(-), CS(+), S(-), S(+)]. The lag-time [λ] and growth capacity [A] could be determined from transformation of coefficients of the Gompertz fit into biological parameters. Using the maximum growth rate [μmax], derived from the growth coefficient of the Gompertz fit [k], maximum doublings per day [kmax], maximum generation time [T2max], and the time when T2max was reached were calculated. The standard error (δ [ ±]) for each value is also presented
Full size table

A significant difference between the production of carotenoids and chlorophyll and the production of C-PC in SAG(-) and S(-) culture media was shown through ANOVA and Tukey tests, compared to no significant difference in CS(+) and S(+) culture media (Tables S5 and S6, Supplementary data). This difference is seen in the value of T2maxwas reached (Table 3), where T2max of C-PC absorption was reached in 38.4 h earlier than that of carotenoids and chlorophyll in SAG(-) and 33.6 h and 28.8 h earlier in S(+) culture media. In contrast, T2max of absorption at the three observed wavelengths occurred at the same time in CS(+) and S(+) culture media, with only minor differences observable.

Nutrients removal

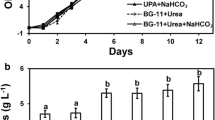
As shown in Fig. 6, A. platensis cultivation showed the largest PO4 decrease in S(+) culture medium, with a significant reduction rate of 3.91 mg L−1 day−1 when compared to S(-) and CS(+) (P-value S(+)/S(-) = 0.007, and S(+)/CS(+) = 0.015). The reduction of PO4 in the other culture media showed insignificant differences, although PO4 decrease in SAG(-) was on average slightly higher than in the CS(+) and S(-) culture media, resulting in insignificant difference between S(+) and SAG(-). On the other hand, NH4 concentration increased significantly in S(+) and S(-)media at day 0, with 23.3 mg L−1 in S(+) and 12.2 mg L−1 (P-value S(-) to SAG(-) ≈ 0, P-value S(+) to SAG(-) ≈ 0). After day 42 no elevated NH4 concentration was recorded. The maximum PO4 reductions of 54.7% and 52.9% were recorded in S(-) and S(+), respectively (Table 4). However, the highest daily removal rate of 3.91 mg L−1 was recorded in S(+).

Fig. 6
figure 6

Analysis results of phosphate concentrations (PO4 [mg L−1]; [A] and [B]) and ammonium (NH4 [mg L−1]; [C] and [D])) in the growth media and final samples, respectively. For each growth medium [SAG(-), CS(+), S(-), and S(+)], the difference between day 0 and day 42 is shown. The whiskers show the respective standard deviation with a coefficient of 1.5. n = 6–12 per boxplot, F- and P-value for dataset A: F = 742.57; P = 4.83E-31, F- and P-value for dataset B: F = 391,86; P = 9.61E-32, F- and P-value for dataset C: F = 261.97; P = 1.48E-23, F- and P-value for dataset D: F = 3.155; P = 0.36968

Full size image
Table 4 Average of the reduction of phosphate (PO4 [mg L−1]) during the growth period of 42 days for each growth medium and the decrease of PO4 compared to the total amount of PO4 in solution
Full size table

Biomass and C-PC productivities

Biomass productivity of A. platensis cultivated in different struvite-based media showed higher values compared to that of SAG(-), i.e. 4.2% [SAG(-)/CS(+)], 7.2% [SAG(-)/S(-)] and 10.5% [SAG(-)/S(+)] higher (Fig. 7A and Table 5. The differences are comparable to the values calculated through the Gompertz fit, as a higher BP is an indicator for higher multiplication rate and a lower value of T2max. However, no significant differences between the values of BP for each respective growth medium were found. Due to increase of BP and C-PC content, CPC-P also increased in the struvite-based media compared to that of SAG(-), by 19.0% [SAG(-)/CS(+)], 12.3% [SAG(-)/S(-)] and 26.5% [SAG(-)/S(+)] (Fig. 7B and Table 5). When applying a two-sample t-test to each compared dataset, significant differences in CPC-P between SAG(-) and CS(+) [EV: Prob >|t|= 0.03; WC: Prob >|t|= 0.04] and between SAG(-) and S(+) [EV: Prob >|t|= 0.02; WC: Prob >|t|= 0.02] were found. The differences shown in Fig. 7[B] differ from the pattern visible when comparing BP or T2max.

Fig. 7
figure 7

Boxplots of biomass productivity [BP (g L−1d−1)] [A] and C-phycocyanin productivity [CPC-P (mg. L−1 day.−1)] [B] of A. platensis grown in different culture media [SAG(-), CS(+), S(-), and S(+)]. The whiskers show the respective standard deviation with a coefficient of 1.5. The data was calculated from the interval of early exponential phase [day 6] to late exponential phase [day 35] during the cultivation period. Each data point represents the mean values of BP and CPC-P. The whiskers show the respective standard error of the means. n = 6 per boxplot F- and P-value for dataset of A: F = 0.759; P = 0.53, F- and P-value for dataset of B (SAG(-)/CS(+)): F = 6.303; P = 0.0309, (SAG(-)/S(-)): F = 0.957; P = 0.351, (SAG(-)/S(+)): F = 7.021; P = 0.024

Full size image
Table 5 The means of BP [g L−1d−1] and CPC-P [mg L−1d−1] of the cultures grown in different culture media [SAG(-), CS(+), S(-), and S(+)] with respective standard error and standard deviation are shown. The data were calculated from the interval of early exponential phase [day 6] to late exponential phase [day 35] during the cultivation period. The increase in BP and CPC-P [%] compared to the control [SAG(-)] is also shown
Full size table

C-PC production per DW (Table 6) continues the pattern of C-PC productivity (Table 5). With the adjustment of DW a C-PC content of 14.5% in SAG(-), 15.8% in CS(+), 13.9% in S(-) and 15.0% in S(+) culture media was calculated. Compared to SAG(-) the C-PC content in DW increased by 9% in CS(+) and by 3.4% in S(+) culture media but decreased by 4.1% in S(-) culture media.

Table 6 The means of C-PC productivity per DW [mg g−1] of A. platensis grown in different culture media [SAG(-), CS(+), S(-), and S(+)] with respective standard error and standard deviation at day 35
Full size table

Discussion

CPC-P in CS(+) and S(+) was significantly higher compared to that of the untreated growth media. In addition, C-PC content (expressed per biomass) was significantly higher compared to that of the untreated growth media. Despite this positive effect of struvite, values of C-PC production are still below those reported in literature. For instance, Eriksen (2008) evaluated CPC-P in different strains of A. platensis and reported values ranging from 3.0 to 24 mg g−1 L−1 in open pond systems, while up to 92 mg g−1 L−1 in closed bioreactors. Ming et al. (2019) summarised values of C-PC content in a range from 121.0—167.9 mg g−1 under red light [PPFD: 100 μmol photons m−2 s−1] and up to 353.0 mg g−1 under blue light [PPFD: 75 μmol photons m−2 s−1]. Overall, lower values of C-PC production in the present study are related to lower growth rates in the present study compared to growth rates reported in the cited studies, providing a chance to further enhance the CPC-P under struvite application by optimization of growth conditions, which requires further research.

The positive effect of struvite on C-PC yield cannot be a result of nitrogen sparing for C-PC production since growth rates did not differ between struvite-based media and SAG(-) in the present study. In general, nitrogen is essential nutrient for A. platensis growth as it is a component of nucleic acids, amino acids and pigments like Chl a and C-PC (Hsieh-Lo et al. 2019). Similar to carotenoids, Chl a is bound in the protein complex of PS I and PS II, and functions as an integral part in the photosystem for light harvest and energy transfer (Vonshak 1997). This makes Chl a essential for enhanced microalgal growth. C-PC however is bound as a light harvesting pigment in the phycobilisome, which function as an antenna system for light harvest with absorption at wavelengths where Chl a absorption does not reach, to expand the efficiency of light harvest (Vonshak 1997; Barsanti 2014). If the high growth rates are attributed to increased light availability, biliproteins bound in the phycobilisome lose their importance and function as a nitrogen source for Chl a production (Hsieh-Lo et al. 2019). Under C-PC depletion because of nitrogen starvation, it is suggested that C-PC, like other biliproteins, functions as nitrogen reserve, and is additionally reduced to free nitrogen for Chl a production (Chen et al. 2010; Hsieh-Lo et al. 2019).

Enhanced C-PC yields in struvite media could be explained by the presence of traces of NH3 residues which are not fully removed by microwave treatment. However, high level of NH3 in the culture media inhibits the growth of A. platensis and, therefore, results in lower CPC-P. To combat such inhibition, struvite was treated via thermal decomposition in order to evaporate NH3. However, increase in C-PC production significantly in the pre-treated CS(+) and S(+) can be explained by the presence of NH3 residues in the medium in a concentration where its toxic effect on PS II is negligible, functioning as an additional nitrogen source and allowing the storage of nitrogen in biliproteins as C-PC.

It was shown that NH3 is the preferred nitrogen source for microalgae, like A. platensis, due to the low cellular energy cost needed for NH3 assimilation (Boussiba 1991; Markou et al. 2019). Additionally, repression of nitrate assimilation was observed, in which continuous presence of NH3 inhibits nitrate uptake through inhibiting glutamine synthetase via inhibitor methionine sulfoximine (MSX) (Boussiba 1991). With inhibition of glutamine synthetase, the main route of nitrate assimilation is blocked, saving energy for the cell, as nitrate has to be intracellularly reduced to ammonia before further use (Boussiba 1991; Markou et al. 2016). This finding concludes that low concentrations of NH3 can have a positive impact on growth and BP, which was previously reported by Markou et al. (2019). Subsequently, a positive impact on CPC-P at low concentrations of NH3 as an energy efficient and quick nitrogen source for A. platensis could have been a reason for the increased CPC-P in the pre-treated culture media under struvite application.

PO4 availability did not seem to be a limiting factor of A. platensis growth in S(-). Struvite in S(-) contained the same amount of PO4 as that present in the form of K2HPO4 in SAG(-). With a solubility constant of 10–13.26 (Shih and Yan 2016), struvite has a very low solubility of 160 mg L−1 (Bhuiyan et al. 2007). This stands out especially if compared to K2HPO4, which is freely soluble in water (Merck & Co 2013) with a solubility of 1600 g L−1 (ChemicalBook 2022). Additionally, struvite solubility decreases with increasing the pH, with a minimum solubility pH of 10.7 (Bhuiyan et al. 2007). As the used culture media for A. platensis utilizes bicarbonate/carbonate buffer with alkaline pH of 9.4 (Aiba and Ogawa 1977), struvite solubility might be negatively impacted. However, the effect of high salinity and ionic strength in A. platensis culture media seemed to have facilitated struvite solubility and PO4 availability, where struvite was almost completely dissolved after 90 min (Markou et al. 2019). When applied to A. platensis culture media, like SAG(-) and S(-), availability of PO4 from struvite should be high enough to allow optimal growth of A. platensis. A difference in initial PO4 concentration is noticeable, as it is reduced by 27.3% in S(-) compared to the initial PO4 concentration in SAG(-). This could be an indicator that the effects of pH and the general low solubility partially outweigh the effects of high salinity and ionic strength for the struvite concentration found in S(-) [0.7 g L−1]. Although a lower PO4 concentration was observed, similar values of PO4 removal compared to SAG(-) showed that no limitations in PO4 availability occurred in S(-). It can be concluded that limited PO4 availability in S(-) cannot be the reason behind reduced growth of A. platensis.

By struvite dissolution, not only PO4 is released into the growth media, but \({\mathrm{NH}}_{4}^{+}\) as well. Bicarbonate/carbonate buffer then causes a shift from \({\mathrm{NH}}_{4}^{+}\) to NH3 (Ullmann's encyclopedia of industrial chemistry 2003; Markou et al. 2016). As NH3 passively diffuses through cell membrane and accumulates in the cells (Boussiba 1991; Markou et al. 2016), it negatively affects cell growth and functions of the photosynthetic apparatus (Markou et al. 2016). Boussiba (1991) reported 20% reduction of light dependent O2 evolution in A. platensis at NH3 concentration of 2.5 mmol. Markou et al. (2016) reported that increased NH3 concentration mainly inhibits PSII activity in A. platensis, with a negative effect on the complete electron transport chain. With inhibition of PSII primarily linked to uncoupling of the Mn cluster of the oxygen-evolving complex [OEC] through NH3, resulting in significant reduction in O2 evolution (Boussiba 1991; Markou et al. 2016). At NH3 concentration of 50 mg L−1, a reduction in O2 evolution to a level of ca. 50% of the control was reported as well (Markou et al. 2016). Chlorophyll fluorescence transients were measured as well, which showed significant fluorescence reduction at NH3 concentration of 50 mg L−1 (Markou et al. 2016). As chlorophyll fluorescence correlates with biomass productivity and is used for stress detection in algae culturing (Suggett et al. 2011), Markou et al. (2016) indicated a lower growth of A. platensis at NH3 concentrations of just 12.5 mg L−1. A gradual inhibition of growth due to NH3 increase during A. platensis cultivation was also reported (Markou et al. 2014). Through dissolution of struvite in S(-), an average \({\mathrm{NH}}_{4}^{+}\) concentration of 23.3 mg L−1 was recorded. Assuming most of \({\mathrm{NH}}_{4}^{+}\) is found as NH3 in S(-), it would explain the reduced growth in this medium. To prevent the inhibition through NH3, a pre-treatment was applied to struvite prior to application to the culture medium in order to remove \({\mathrm{NH}}_{4}^{+}\) in S(+). This pre-treatment is based on thermal decomposition of struvite by microwave radiation which results in evaporation of \({\mathrm{NH}}_{4}^{+}\) (Chen et al. 2020). Therefore, the concentration of \({\mathrm{NH}}_{4}^{+}\) was significantly lower (12.2 mg L−1) after pre-treatment, with a P-value of 3.62E-6 between S(-) and S(+).

A lower increase in maximum growth rate between SAG(-) and CS(+) (increase in μmax[480] = 3.5%; increase in μmax[700] = 5.0%) was measured as well. The slight improvement was also visible in BP, with an increase of 4.2% when compared to the BP of SAG(-). In that context, Celekli et al. (2009) reported a peak of biomass productivity in media with a pH of 10.0 at a PO4 concentration of 0.5 g L−1. With a similar PO4 concentration in CS(+) [cPO4: 499.1 (± 2,8) mg L−1], this could have increased BP and growth rates of A. platensis in CS(+). However, this effect was shown to be dependent on pH, as no peak in growth could be seen at a pH of 9.5 or 10.5 for cPO4: 0.5 g L−1 (Celekli et al. 2009). Generally, variations in PO4 concentration up to 0.5 g L−1 do not affect the algal biomass productivity, as long no limitations of PO4 availability occur, which can be confirmed in previous studies (Celekli et al. 2009; Markou et al. 2012). The high concentration of PO4 found in CS(+) was presumably the result of the assumption, that struvite would be found in its hexahydrate form. As the process of hydration might not have been complete, the percentage of PO4 per gram of struvite substrate was higher than anticipated. Similar to S(+), the effect of NH3 growth inhibition was not visible in CS(+), as measured \({\mathrm{NH}}_{4}^{+}\) concentration of 1.1 mg L−1 confirms the positive impact of the pre-treatment. Through the removal of \({\mathrm{NH}}_{4}^{+}\), struvite can be seen as a viable and sustainable option as a main phosphate source for commercial A. platensis cultivation.

Conclusion

The present study showed that no statistically significant negative effect of struvite was found on the growth rates of A. platensis. Interestingly, C-PC content and productivity using in the media using microwave-pretreated struvite [CS(+) and S(+)] were significantly higher compared to that of the untreated struvite. It is attributed to the removal of ammonia after microwave treatment, which has negative impacts at high concentrations. However, it is hypothesized that traces of NH3, remaining after the pre-treatment of struvite, could have a positive impact on the growth and C-PC yields as an energy efficient and quick nitrogen source for A. platensis.