Symmetric Derivation of Singlet Correlations in a Quaternionic 3-sphere Model

Abstract

Using the powerful language of geometric algebra, we present an observationally symmetric derivation of the strong correlations predicted by the entangled singlet state in a deterministic and locally causal model, usually also referred to as a local-realistic model, in which the physical space is assumed to be a quaternionic 3-sphere, or $S^3$, available as the spatial part of a solution of Einstein’s field equations of general relativity, and compare it in quantitative detail with Bell’s local-realistic model for the singlet correlations set within a flat Euclidean space ${\mathrm {I\!R}^3}$. Since the quantitatively detailed expressions of relative-angle-dependent probabilities of observing measurement outcomes for Bell’s local model do not seem to have been fully articulated before, our novel analysis exploiting the non-commutative properties of quaternions, in addition to allowing the comparison with the quaternionic 3-sphere model, may also provide useful comparisons for other less compelling local-realistic models, such as those relying on retrocausality or superdeterminism. Apart from the conservation of zero spin angular momentum, the key attribute underlying the strong singlet correlations within $S^3$ in comparison with Bell’s local model turns out to be the spinorial sign changes intrinsic to quaternions that constitute the 3-sphere. In addition, we also discuss anew a macroscopic experiment that can, in principle, test our 3-sphere hypothesis.

Appendices

Appendix A: Proofs of the Equalities (35) and (47)

In this appendix we prove the equalities (35) and (47), which amounts to proving that the “product of limits equal to limits of product” rule holds for quaternions and bivectors. To that end, we begin with the left-hand side of (35):

$$\begin{aligned} \bigg [\lim _{{\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}}}\left\{ -\,{\textbf{D}}({\textbf{a}})\,{\textbf{L}}({\textbf{s}}_1)\right\} \bigg ]\left[ \lim _{{\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}}}\left\{ +\,{\textbf{L}}({\textbf{s}}_2)\,{\textbf{D}}({\textbf{b}})\right\} \right]&=\bigg [\lim _{{\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}}}\left\{ -I_3{\textbf{a}}\,I_3{\textbf{s}}_1\right\} \bigg ]\left[ \lim _{{\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}}}\left\{ +I_3{\textbf{s}}_2\,I_3{\textbf{b}}\right\} \right] \end{aligned}$$

(A1)

$$\begin{aligned}&=\bigg [\lim _{{\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}}}\left\{ -(I_3)^2\,{\textbf{a}}\,{\textbf{s}}_1\right\} \bigg ]\left[ \lim _{{\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}}}\left\{ +(I_3)^2\,{\textbf{s}}_2\,{\textbf{b}}\right\} \right] \end{aligned}$$

(A2)

$$\begin{aligned}&=\bigg [\lim _{{\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}}}\left\{ +\,{\textbf{a}}\,{\textbf{s}}_1\right\} \bigg ]\left[ \lim _{{\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}}}\left\{ -\,{\textbf{s}}_2\,{\textbf{b}}\right\} \right] \end{aligned}$$

(A3)

$$\begin{aligned}&=\,\left[ \mu _1{\textbf{a}}\,{\textbf{a}}\right] \left[ -\mu _2{\textbf{b}}\,{\textbf{b}}\right] \end{aligned}$$

(A4)

$$\begin{aligned}&=-\mu _1\mu _2\,, \end{aligned}$$

(A5)

where we have used the fact that all vectors involved in the model are unit vectors and the fact that the pseudoscalar $I_3$ commutes with all other elements of $\textrm{Cl}_{3,0}$ and squares to $-1$. Similarly, the right-hand side of (35) simplifies to

$$\begin{aligned} \lim _{\begin{array}{c} {\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}} \\ {\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}} \end{array}}\left[ \left\{ -\,{\textbf{D}}({\textbf{a}})\,{\textbf{L}}({\textbf{s}}_1)\right\} \left\{ +\,{\textbf{L}}({\textbf{s}}_2)\,{\textbf{D}}({\textbf{b}})\right\} \right]&=\lim _{\begin{array}{c} {\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}} \\ {\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}} \end{array}}\left[ \left\{ -I_3{\textbf{a}}\,I_3{\textbf{s}}_1\right\} \left\{ +I_3{\textbf{s}}_2\,I_3{\textbf{b}})\right\} \right] \end{aligned}$$

(A6)

$$\begin{aligned}&=\lim _{\begin{array}{c} {\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}} \\ {\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}} \end{array}}\left[ \left\{ -(I_3)^2\,{\textbf{a}}\,{\textbf{s}}_1\right\} \left\{ +(I_3)^2\,{\textbf{s}}_2\,{\textbf{b}})\right\} \right] \end{aligned}$$

(A7)

$$\begin{aligned}&=\lim _{\begin{array}{c} {\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}} \\ {\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}} \end{array}}\left[ \left\{ +\,{\textbf{a}}\,{\textbf{s}}_1\right\} \left\{ -\,{\textbf{s}}_2{\textbf{b}})\right\} \right] \end{aligned}$$

(A8)

$$\begin{aligned}&=\lim _{\begin{array}{c} {\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}} \\ {\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}} \end{array}}\left[ -\,{\textbf{a}}\,{\textbf{s}}_1\,{\textbf{s}}_2{\textbf{b}}\right] \end{aligned}$$

(A9)

$$\begin{aligned}&=\left[ -\mu _1{\textbf{a}}\,{\textbf{a}}\,\mu _2{\textbf{b}}\,{\textbf{b}}\right] \end{aligned}$$

(A10)

$$\begin{aligned}&=-\mu _1\mu _2. \end{aligned}$$

(A11)

Since the right-hand sides of (A5) and (A11) are equal, “the product of limits equal to limits of product” rule holds.

Analogously, we can also prove the equality (47) by simplifying its left-hand side and right-hand side, as follows:

$$\begin{aligned} \left[ \lim _{{\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}}}\left\{ +\,{\textbf{L}}({\textbf{s}}_2)\,{\textbf{D}}({\textbf{b}})\right\} \right] \left[ \lim _{{\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}}}\left\{ -\,{\textbf{D}}({\textbf{a}})\,{\textbf{L}}({\textbf{s}}_1)\right\} \right]&=\bigg [\lim _{{\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}}}\left\{ +I_3{\textbf{s}}_2\,I_3{\textbf{b}}\right\} \bigg ]\left[ \lim _{{\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}}}\left\{ -I_3{\textbf{a}}\,I_3{\textbf{s}}_1\right\} \right] \end{aligned}$$

(A12)

$$\begin{aligned}&=\bigg [\lim _{{\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}}}\left\{ +(I_3)^2\,{\textbf{s}}_2\,{\textbf{b}}\right\} \bigg ]\left[ \lim _{{\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}}}\left\{ -(I_3)^2\,{\textbf{a}}\,{\textbf{s}}_1\right\} \right] \end{aligned}$$

(A13)

$$\begin{aligned}&=\bigg [\lim _{{\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}}}\left\{ -\,{\textbf{s}}_2\,{\textbf{b}}\right\} \bigg ]\left[ \lim _{{\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}}}\left\{ +\,{\textbf{a}}\,{\textbf{s}}_1\right\} \right] \end{aligned}$$

(A14)

$$\begin{aligned}&=\,\left[ -\mu _2{\textbf{b}}\,{\textbf{b}}\right] \left[ +\mu _1{\textbf{a}}\,{\textbf{a}}\right] \end{aligned}$$

(A15)

$$\begin{aligned}&=-\mu _1\mu _2 \end{aligned}$$

(A16)

and

$$\begin{aligned} \lim _{\begin{array}{c} {\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}} \\ {\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}} \end{array}}\left[ \left\{ -{\textbf{D}}({\textbf{b}})\,{\textbf{D}}({\textbf{a}})\right\} \left\{ \,{\textbf{L}}({\textbf{s}}_1)\,{\textbf{L}}({\textbf{s}}_2)\right\} \right]&=\lim _{\begin{array}{c} {\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}} \\ {\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}} \end{array}}\left[ \left\{ -I_3{\textbf{b}}\,I_3{\textbf{a}}\right\} \left\{ I_3{\textbf{s}}_1\,I_3{\textbf{s}}_2)\right\} \right] \end{aligned}$$

(A17)

$$\begin{aligned}&=\lim _{\begin{array}{c} {\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}} \\ {\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}} \end{array}}\left[ \left\{ -(I_3)^2\,{\textbf{b}}\,{\textbf{a}}\right\} \left\{ (I_3)^2\,{\textbf{s}}_1\,{\textbf{s}}_2)\right\} \right] \end{aligned}$$

(A18)

$$\begin{aligned}&=\lim _{\begin{array}{c} {\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}} \\ {\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}} \end{array}}\left[ \left\{ +\,{\textbf{b}}\,{\textbf{a}}\right\} \left\{ -\,{\textbf{s}}_1\,{\textbf{s}}_2)\right\} \right] \end{aligned}$$

(A19)

$$\begin{aligned}&=\lim _{\begin{array}{c} {\textbf{s}}_2\,\rightarrow \,\mu _2{\textbf{b}} \\ {\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}} \end{array}}\left[ -\,{\textbf{b}}\,{\textbf{a}}\,{\textbf{s}}_1\,{\textbf{s}}_2\right] \end{aligned}$$

(A20)

$$\begin{aligned}&=\left[ -\mu _1\mu _2\,{\textbf{b}}\,{\textbf{a}}\,{\textbf{a}}\,{\textbf{b}}\right] \end{aligned}$$

(A21)

$$\begin{aligned}&=\left[ -\mu _1\mu _2\,{\textbf{b}}\,{\textbf{b}}\right] \end{aligned}$$

(A22)

$$\begin{aligned}&=-\mu _1\mu _2. \end{aligned}$$

(A23)

Since the right-hand sides of (A16) and (A23) are equal, “the product of limits equal to limits of product” rule holds.

Appendix B: Questions and Answers

In no particular order, in this appendix I answer some questions concerning the $S^3$ model for the singlet correlations presented in this paper.

Question 1: While several good plausibility arguments are presented in the Introduction of the paper, they do not prove that physical space should be modeled as $S^3$ rather than $\mathrm {I\!R}^3$. How, then, is that assumption justified in the absence of proof?

Answer 1: Apart from the plausibility arguments presented in the Introduction, there are several good reasons that justify the assumption of $S^3$. While this assumption is usually not made in the context of Bell’s theorem, the fact that quaternions play a fundamental role in understanding the algebra, geometry, and topology of physical space is well-known since the works of Hamilton and Clifford in the 19th century and those of Pauli and Dirac in the 20th century. Dirac’s belt trick and Feynman’s plate trick are often used pedagogically to illustrate this fact. It has contributed to the formulation of Hypothesis 1 stated in the paper, which is a formal statement of my view that the best terrestrial evidence for $S^3$ as the geometry of physical space is the observed strong correlations in the Bell-test experiments.

But perhaps the strongest justification for the assumption of $S^3$ as physical space stems from the fact that the traditional interpretation of Bell’s theorem is recovered from the $S^3$ model within the flat geometry of ${{\mathrm {I\!R}^3}}$ in which the characteristically non-trivial algebra, geometry, and topology of ${S^3}$ are absent. Consequently, the absolute upper bound of 2 on the Bell-CHSH combination (1) of expectation values is respected within $\mathrm {I\!R}^3$, as I have demonstrated, for example, in [9] and [14]. In fact, it is not difficult to demonstrate that the results presented in the current paper also reproduce the traditional interpretation of Bell’s theorem in the flat geometry of ${{\mathrm {I\!R}}^3}$, which is usually taken for granted in the context of Bell’s theorem. There are several different ways of appreciating this fact, as explained in Section X of [9], each providing a different insight into how the standard interpretation of Bell’s theorem is recovered in ${{\mathrm {I\!R}}^3}$.

As demonstrated in Section VI of [9], one way to appreciate it is by analyzing $S^3\rightarrow \mathrm {I\!R}^3$ limit in the event-by-event numerical simulations of the strong singlet correlations. A second way to appreciate it is by setting the parallelizing torsion ${{\mathscr {T}}}$ in $S^3$ to zero, as demonstrated in Section IX of [9], which reduces $S^3$ to $\mathrm {I\!R}^3$ as well as the absolute bound of $2\sqrt{2}$ on the Bell-CHSH sum (1) of expectation values to 2. A third way to appreciate it is by recognizing that the eigenvalue of the sum of quantum mechanical operators involved in Bell-CHSH inequalities and Bell-test experiments necessarily includes a purely geometrical contribution stemming from the $S^3$ geometry of physical space, and when that contribution is set to zero the absolute bound of $2\sqrt{2}$ on the Bell-CHSH sum (1) reduces to 2, as shown in [14].

But perhaps the best way to appreciate the recovery of the traditional interpretation of Bell’s theorem within $\mathrm {I\!R}^3$ from the $S^3$ model is geometrical, by recognizing that the strong singlet correlations $-\cos (\eta _{{\textbf{a}}{\textbf{b}}})$ are a direct consequence of Möbius-like twists in the Hopf bundle of $S^3$, as explained in Section 5.

Question 2: Does the 3-sphere hypothesis proposed in the paper mean that an observationally flat universe (which is the view preferred by the presently dominant $\Lambda$-CDM model of cosmology) would essentially disqualify the proposed $S^3$ model?

Answer 2: No. The hypothesis proposed in the Introduction of the paper stands on its own. It has strong support from the cosmological observations, and from the fact that the 3-sphere is a permitted spatial part of one of the well-known solutions of Einstein’s field equations of general relativity, but it is not dependent on this evidence from the cosmological observations.

Question 3: The topology of the quaternionic 3-sphere is a global property of $S^3$. Does not that make the $S^3$ model a non-local realistic model rather than a local-realistic one as claimed?

Answer 3: The topology of $S^3$ is indeed a global property. But that does not make the $S^3$ model non-local realistic, at least for two fundamental reasons. To begin with, $S^3$, when viewed as physical space as in the model, is a spatial part of a well-known solution of Einstein’s field equations of general relativity, which is a locally causal theory. Moreover, within the context of Bell’s theorem, local causality is very specifically defined in terms of measurement results, such as the functions ${\mathscr {A}}({\textbf{a}},{\textbf{s}}^i_1)=\pm 1$ and ${\mathscr {B}}({\textbf{b}},{\textbf{s}}^i_2)=\pm 1$ defined in (17) and (23). If these functions are local, then the model is said to be local. And it is easy to verify that the functions are indeed local in the sense espoused by Einstein and later mathematically formulated by Bell [4]: Apart from the hidden variable $\textbf{s}^i_1$, the result ${{\mathscr {A}}=\pm 1}$ depends only on the measurement direction $\textbf{a}$, chosen freely by Alice, regardless of Bob’s actions. And, analogously, apart from the hidden variable $\textbf{s}^i_2$, the result ${{\mathscr {B}}=\pm 1}$ depends only on the measurement direction $\textbf{b}$, chosen freely by Bob, regardless of Alice’s actions. In particular, the function ${{\mathscr {A}}(\textbf{a},\,\textbf{s}^i_1)}$ does not depend on $\textbf{b}$ or ${\mathscr {B}}$ and the function ${{\mathscr {B}}(\textbf{b},\,\textbf{s}^i_2)}$ does not depend on $\textbf{a}$ or ${\mathscr {A}}$. Moreover, the hidden variables $\textbf{s}^i_1$ and $\textbf{s}^i_2$ do not depend on $\textbf{a}$, $\textbf{b}$, ${\mathscr {A}}$, or ${\mathscr {B}}$. Therefore, the model presented in the paper is manifestly local-realistic.

Question 4: Is it possible that $S^3$ geometry is relevant only for the space of entangled particles rather than physical space?

Answer 4: That would be a different hypothesis, not the one considered in the paper. It would amount to assuming that $S^3$ is an internal space, as in gauge theories. That hypothesis would not allow us to overcome Bell’s theorem while respecting both the conditions of local realism and the observational constraints set by the Bell-test experiments. In other words, under that hypothesis, it would not be possible to derive the strong correlations (63), together with vanishing averages (32) and (33) for the separate results observed by Alice and Bob, while maintaining the strict locality condition explained above. This is because Bell’s theorem remains valid within the flat geometry of $\mathrm {I\!R}^3$, as explained above and in Section X of Ref. [9].

Question 5: What is meant by the statement “during the free evolution, the spins do not change in either their senses or directions”? If the spins do not change in their senses or directions, then in what sense is there “evolution" of the spins?

Answer 5: Within the context of EPR-Bohm experiments, “free evolution” means the motion of the emerging spins free from interactions before they reach the detectors. Consequently, between the source and the detectors, the senses and directions of the spins are preserved. However, they are still propagating from the source to the detectors during this time. Soon after the constituent fermions emerge from the source as shown in Fig. 1, they cease to interact with each other appreciably but continue their journey toward the detectors. Moreover, since the spin system is assumed to be isolated, there is no external interaction between the spins and any other physical system until they interact with the two detectors. Also, their combined spin angular momentum would remain equal to zero, thanks to the law of the conservation of angular momentum. Therefore, the two spins do not change in their senses or directions while evolving between the source and the detectors. In other words, the EPR-Bohm correlations are purely kinematical effects. This is well understood since the first analysis of the singlet correlations using fermionic spins was carried out by Bohm in Section 22.16 of his 1951 book on quantum theory [23].

Question 6: The Stern-Gerlach device has a non-uniform electromagnetic field that aligns incoming charged particles to its orientation. In the $S^3$ model, the physics of this interaction and the nature of the particle itself are ignored, with the process of alignment being modeled with limit functions. But while it may be natural to mathematically represent spins of fermionic particles with bivectors, there is a vast difference between a Stern-Gerlach detector and a particle spin, and yet in the $S^3$ model both are represented with bivectors. Is that justified?

Answer 6: Yes, that is perfectly justified and natural. The details of a specific experimental device are not relevant in the $S^3$ model, just as they are not relevant in Bell’s local model set within $\mathrm {I\!R}^3$. In Bell’s local model, what is represented by a vector, such as “$\textbf{a}$” appearing in the argument of the functions ${\mathscr {A}}(\textbf{a},\textbf{s}_1^i)$, is an orientation of a detector, in three-dimensional physical space, mathematically modeled as $\mathrm {I\!R}^3$. The detectors such as the Stern-Gerlach apparatus themselves are, of course, complicated physical objects, whereas a vector is a geometrical object that models only the orientation of a detector in physical space. That is fine when physical space is a non-compact flat space such as $\mathrm {I\!R}^3$. The compact space $S^3$, however, has very different properties. I have defined it as a set of quaternions:

$$\begin{aligned} S^3:=\left\{ \,\textbf{q}(\beta ,\,{\textbf{r}})=\varrho _r\exp \left[ {\textbf{J}}({\textbf{r}})\,\frac{\beta }{2}\right] \Bigg |\;\left| \left| \,\textbf{q}\left( \beta ,\,{\textbf{r}}\right) \,\right| \right| =\varrho _r\right\} . \end{aligned}$$

(B1)

As it is constituted by quaternions, an orientation of a detector within $S^3$ is best represented by a pure quaternion or bivector such as $\textbf{D}(\textbf{a}):=I_3\textbf{a}$. Recall that a bivector is also a geometrical object, much like a vector within $\mathrm {I\!R}^3$. It is an abstraction of an oriented plane segment with only three properties: magnitude, direction, and sense of rotation (see Fig. 7). A bivector $\textbf{D}(\textbf{a}):=I_3\textbf{a}$ within $S^3$ is thus a counterpart of a vector “$\textbf{a}$” within $\mathrm {I\!R}^3$. As such, it provides the most adequate representation of an orientation of a detector in $S^3$. A vector cannot serve this purpose within $S^3$.

Question 7: What is the motivation for using mathematical limits to model the physical processes of detecting spin values?

Fig. 7

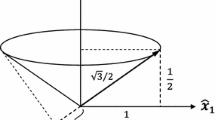
A bivector, which represents a continuous binary rotation about some axis, is understood as an abstraction of a directed plane segment, with only a magnitude and a sense of rotation—i.e., clockwise (${-}$) or counterclockwise (${+}$). Neither the depicted oval shape of its plane, nor its axis of rotation such as $\textbf{a}$, is an intrinsic part of the bivector ${I_3\textbf{a}}$. After [9]

Answer 7: Recall that the measurement function ${{\mathscr {A}}(\textbf{a},\,\textbf{s}^i_1)}$ for Alice is defined in Bell’s local model using the sign function:

$$\begin{aligned} {\mathscr {A}}(\textbf{a},\,\textbf{s}^i_1)=\text {sign}(\textbf{a}\cdot \textbf{s}^i_1)=\pm 1. \end{aligned}$$

(B2)

As noted before, the details of specific experimental devices, or the physical workings of the measurement instruments, are not relevant in formulating Bell’s local model, set within $\mathrm {I\!R}^3$. What is modeled in (B2) is the experimental fact that measurement instruments like any Stern-Gerlach device detect a spin by aligning its axis of rotation $\textbf{s}^i_1$ with the vector $\textbf{a}$ that specifies the orientation of the device in space. This produces an observable spot projected onto a screen, indicating that the spin is “up” or “down” along the direction $\textbf{a}$, which is then mathematically represented by a number $+1$ or $-1$. Any other details of the physical workings of the device are not relevant to Bell’s definition (B2) of the measurement results. But this definition is for the detection processes taking place within $\mathrm {I\!R}^3$. Its use of sign function is not valid for the detection processes taking place within $S^3$. Since the counterparts of the vectors $\textbf{a}\in \mathrm {I\!R}^3$ and $\textbf{s}_1\in \mathrm {I\!R}^3$ within $S^3$ are the bivectors ${\textbf{D}}({\textbf{a}})$ and ${\textbf{L}}({\textbf{s}}_1)$ representing rotations about the axes $\textbf{a}$ and $\textbf{s}_1$ (see Fig. 7), what is required is a limit of the product quaternion ${\textbf{D}}({\textbf{a}})\,{\textbf{L}}({\textbf{s}}_1)$ representing the physical interaction between ${\textbf{D}}({\textbf{a}})$ and ${\textbf{L}}({\textbf{s}}_1)$ to produce the discrete results $\pm 1$ observed by Alice. Fortunately, definition (B2) can be amended to accommodate this for the detection processes taking place within $S^3$, so that the correct definition of the measurement function ${\mathscr {A}}(\textbf{a},\,\textbf{s}^i_1)$ is

$$\begin{aligned} S^3\ni {\mathscr {A}}({\textbf{a}},{\textbf{s}}^i_1)\,&=\lim _{{\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}}}\left\{ -\,{\textbf{D}}({\textbf{a}})\,{\textbf{L}}({\textbf{s}}_1)\right\} \end{aligned}$$

(B3)

$$\begin{aligned}&=\lim _{{\textbf{s}}_1\,\rightarrow \,\mu _1{\textbf{a}}}\left\{ -(I_3{\textbf{a}})(I_3{\textbf{s}}_1)\right\} \end{aligned}$$

(B4)

$$\begin{aligned}&=\mu _1\,, \end{aligned}$$

(B5)

where the quaternion $\left\{ -\,{\textbf{D}}({\textbf{a}})\,{\textbf{L}}({\textbf{s}}_1)\right\}$ is an element of set $S^3$,

$$\begin{aligned} \mu _1 :=\text {sign}(\textbf{a}\cdot \textbf{s}^i_1)=\pm 1, \end{aligned}$$

(B6)

and the substitution rule for limits (and the fact that the unit bivector $I_3{\textbf{a}}$ squares to $-1$) is used to derive the equality (B5). Thus, definition (B3) reproduces Bell’s definition (B2) in $\mathrm {I\!R}^3$, with the use of limit function accomplishing the experimentally required alignment of the vector $\textbf{s}^i_1$ with the vector $\textbf{a}$. In other words, the use of limits to model the physical processes within $S^3$ of spin detections seen in Stern-Gerlach devices is not only required by the geometrical properties of $S^3$, but also natural. It is also worth noting that the substitution rule for limits used above, which essentially amounts to writing

$$\begin{aligned} \lim _{{\textbf{s}}_1\,\rightarrow \,{\textbf{a}}}\,I_3\,{\textbf{s}}_1\,=\,I_3\,{\textbf{a}} \end{aligned}$$

(B7)

because ${\textbf{L}}({\textbf{s}}_1):=I_3\,{\textbf{s}}_1$ and ${\textbf{L}}({\textbf{a}}):=I_3\,{\textbf{a}}$, follows at once from the rules of Geometric Algebra. Moreover, it is easy to verify that the substitution rule for limits itself can be derived rigorously for any functions of vector quantities using the $\epsilon$–$\delta$ definition of limits from basic calculus [24].

Question 8: Why are the directions of the constituent spins anti-aligned in Bell’s model by setting $\textbf{s}_1=-\textbf{s}_2$, whereas in the $S^3$ model they are aligned to each other? There might be some subtlety in this regard, because if one of the observers is rotated by 180 degrees in order to face oncoming particles, then that will flip the horizontal directions of their coordinate axes.

Answer 8: A fundamental difference between Bell’s model set within $\mathrm {I\!R}^3$ and the quaternionic 3-sphere model set within $S^3$ is that, while in Bell’s model the spin angular momenta are incorrectly represented by ordinary vectors such as $\textbf{s}_1$ and $\textbf{s}_2$ and the conservation of initial zero spin is stipulated as $\textbf{s}_1+\textbf{s}_2=0$ or as $\textbf{s}_1=-\textbf{s}_2$, in the $S^3$ model the spin angular momenta are correctly represented by the bivectors $-{\textbf{L}}({\textbf{s}}_1)$ and ${\textbf{L}}({\textbf{s}}_2)$ because they are rotating in opposite senses. As a result, the conservation of net zero spin is stipulated in (13) as

$$\begin{aligned} -\,\textbf{L}(\textbf{s}_1)\,+\,\textbf{L}(\textbf{s}_2)=0 \;\Longleftrightarrow \;\textbf{L}(\textbf{s}_1)=\textbf{L}(\textbf{s}_2) \Longleftrightarrow \;\textbf{s}_1=\,\textbf{s}_2\,\equiv \,\textbf{s}. \end{aligned}$$

(B8)

because $-{\textbf{L}}({\textbf{s}}_1)=-I_3\textbf{s}_1$ and ${\textbf{L}}({\textbf{s}}_2)=I_3\textbf{s}_2$, which is equivalent to the following conservation condition stated in equation (14):

$$\begin{aligned} \textbf{L}(\textbf{s}_1)\,\textbf{L}(\textbf{s}_2)=\,I_3\textbf{s}\,I_3\textbf{s}=(I_3)^2\,\textbf{s}\,\textbf{s}=-1. \end{aligned}$$

(B9)

Once these mathematical relations are strictly followed, no verbal description with regard to what will happen in a given specific scenario such as “what if one of the observers is rotated by 180 degrees to face the oncoming particles,” etc. is necessary.

Question 9: Bell’s general argument and other impossibility arguments do not assume that space has the structure of $\mathrm {I\!R}^3$.

Answer 9: Although widely believed, this assertion is quite incorrect. Bell’s theorem and other impossibility arguments do implicitly assume that physical space has the structure of $\mathrm {I\!R}^3$. It is unfortunate that neither Bell nor other proponents of his theorem state this assumption explicitly. The only exception is a brief remark by Bell, quoted in the Introduction of the paper, which reads: “The space time structure has been taken as given here. How then about gravitation?” By ignoring the qualitative features of spacetime, Bell and his followers have neglected the geometrical and topological properties of physical space in their theorems, such as the non-commutativity of quaternions that constitute the 3-sphere. It is evident from the model considered in the paper that when these properties are taken into account, the observed strong singlet correlations can be easily derived.

Question 10: Bell’s general argument and other impossibility arguments do not rely on the particular local model discussed in Section 3 of Bell’s 1964 paper [4] and in Peres’s book [16].

Answer 10: This is not quite correct. The mathematical core of Bell’s theorem, while not dependent on the specific local model discussed in Section 3 of his paper, is nevertheless based on the correlations predicted by the singlet state. As such, the local model in Section 3 of his paper is of considerable interest. It is very much a part of the impossibility claim of the theorem.