Solutions of Noncommutative Two-Dimensional Position–Dependent Mass Dirac Equation in the Presence of Rashba Spin-Orbit Interaction by Using the Nikiforov–Uvarov Method

Abstract

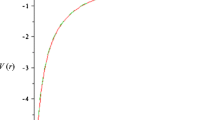
We study the combined effects of both position-dependent mass (PDM) and Rashba spin-orbit interaction (RSOI) on the Dirac equation of a spin 1/2 particle moving in a plane and in the presence of a magnetic field within noncommutative (NC) phase-space. The eigenvalues of the two-dimensional system are calculated and the corresponding eigenstates are obtained. The model is solved using NU method then some numerical results are given and used to extensively investigate the behavior of the system under the various influences of linear potential (involved in PDM), magnetic field and RSOI in both commutative and NC frameworks.

Appendix: Nikiforov-Uvarov Method

The Nikiforov Uvarov (NU) method is an exact approach to obtain the energy eigenvalues and corresponding wave functions of a vast variety of quantum systems, as done in Refs. [38, 59, 60], etc.

Here we give a brief description of the conventional NU method [38]. This powerful mathematical method was proposed to solve large classes of second-order linear ordinary differential equation of hyper-geometric type or a general Schrödinger-type equation with an appropriate coordinate transformation $s=s(r$) as

$$\begin{aligned} \left\{ \frac{\partial ^{2}}{\partial s^{2}}+\frac{a_{1}-a_{2}s}{s\left( 1-a_{3}s\right) }\frac{\partial }{\partial s}+\frac{-\lambda _{1}s^{2}+\lambda _{2}s-\lambda _{3}}{s^{2}\left( 1-a_{3}s\right) ^{2}}\right\} \chi \left( s\right) =0. \end{aligned}$$

(A1)

To obtain the eigenvalues and corresponding wave functions, we introduce the following relations

$$\begin{aligned} \begin{array}{ccc} a_{4}=\frac{1}{2}\left( 1-a_{1}\right) , &{} a_{5}=\frac{1}{2}\left( a_{2}-2a_{3}\right) , &{} a_{6}=a_{5}^{2}+\lambda _{1},\\ a_{7}=2a_{4}a_{5}-\lambda _{2}, &{} a_{8}=a_{4}^{2}+\lambda _{3}, &{} a_{9}=a_{3}a_{7}+a_{3}^{2}a_{8}+a_{6},\\ a_{10}=a_{1}+2a_{4}+2\sqrt{a_{8}},\; &{} a_{11}=a_{2}-2a_{5}+2\left( \sqrt{a_{9}}+a_{3}\sqrt{a_{8}}\right) , &{} a_{12}=a_{4}+\sqrt{a_{8}},\\ &{} a_{13}=a_{5}-\left( \sqrt{a_{9}}+a_{3}\sqrt{a_{8}}\right) . \end{array} \end{aligned}$$

(A2)

Then, the energy equation reads

$$\begin{aligned} a_{2}N-\left( 2N+1\right) a_{5}+\left( 2N+1\right) \left( \sqrt{a_{9}}+a_{3}\sqrt{a_{8}}\right) +N\left( N-1\right) a_{3}+a_{7}+2a_{3}a_{8}+2\sqrt{a_{8}a_{9}}=0. \end{aligned}$$

(A3)

Furthermore, the final wave function in NU method will be as follows

$$\begin{aligned} \psi \left( s\right) =s^{a_{12}}\left( 1-a_{3}s\right) ^{-a_{12}-\frac{a_{13}}{a_{3}}}P_{N}^{\left( a_{10}-1,\frac{a_{11}}{a_{3}}-a_{10}-1\right) }\left( 1-2a_{3}s\right) . \end{aligned}$$

(A4)

If $a_{3}=0$, (A4) will be reduced to

$$\begin{aligned} \psi \left( s\right) =s^{a_{12}}e^{a_{13}s}L_{N}^{a_{10}-1}\left( a_{11}s\right) . \end{aligned}$$

(A5)

Here $L_{N}$, $P_{N}^{\left( A,B\right) }$ are Laguerre functions and Jacobi polynomials respectively, which are given as follows [59]

$$\begin{aligned} P_{N}^{\left( A,B\right) }\left( s\right) =\frac{\Gamma \left( A+n+1\right) }{n!\Gamma \left( A+B+n+1\right) }\sum _{m=0}^{n}\left( \begin{array}{c} n\\ m \end{array}\right) \frac{\Gamma \left( A+n+m+1\right) }{n!\Gamma \left( A+m+1\right) }\left( \frac{s-1}{2}\right) ^{m}, \end{aligned}$$

(A6)

and [60]

$$\begin{aligned} L_{N}=\frac{e^{s}}{N!}\frac{d^{N}}{ds^{N}}\left( e^{-s}s^{N}\right) =\frac{1}{N!}\left( \frac{d}{ds}-1\right) ^{N}s^{N}, \end{aligned}$$

(A7)

which defined by the Rodrigues formula.