Non-Markovian Effect in Optomechanical System

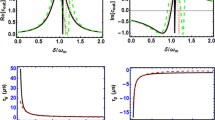
Abstract

Most studies on optomechanical systems have been performed under the Markovian approximation. In this paper, we extend the study from the Markovian to the non-Markovian regime. According to the Markovian optomechanically induced transparency (OMIT) theory in Weis et al. (Science 330, 1520, 2010), we propose the non-Markovian counterpart. We find that the non-Markovianity might give rise to negative absorption, i.e., the probe field gains from the environment. By calculating the mean position of the mechanical resonator (MR), we illustrate the effect of non-Markovianity on the dynamics of the moving mirror.

Appendices

Appendix A

From the Hamiltonian (4), we can obtain the Heisenberg equation of the bath operator $\hat {b}$

$$ \dot{\hat{b}}(\omega)=k(\omega)\hat{a}e^{-i(\omega_{0}-\omega)t}, $$

(A1)

and we solve (A1) to obtain

$$ \hat{b}(\omega)=\hat{b}_{0}(\omega)+k(\omega){{\int}_{0}^{t}}\hat{a}(t^{\prime})e^{-i(\omega_{0}-\omega)t^{\prime}}dt^{\prime}, $$

(A2)

where $\hat {b}_{0}(\omega )$ is the value of $\hat {b}(\omega )$ at t = 0. The Heisenberg equation of the system operator $\hat {a}$ is

$$\begin{array}{@{}rcl@{}} \dot{\hat{a}}&=&-i\left[-\frac{g}{\hbar}\hat{a}\hat{q}+i\varepsilon_{l}e^{-i(\omega_{l}-\omega_{0}) t}+i\varepsilon_{p}e^{-i(\omega_{p}-\omega_{0}) t}\right]\\ &&-{\int}_{-\infty}^{\infty} k^{*}(\omega)\hat{b}(\omega)e^{i(\omega_{0}-\omega)t}d\omega, \end{array} $$

(A3)

We substitute (A2) into (A3) to obtain

$$\begin{array}{@{}rcl@{}} \dot{\hat{a}}&=&-i\left[-\frac{g}{\hbar}\hat{a}\hat{q}+i\varepsilon_{l}e^{-i(\omega_{l}-\omega_{0}) t}+i\varepsilon_{p}e^{-i(\omega_{p}-\omega_{0}) t}\right]\\ &&-{\int}_{-\infty}^{\infty}d\omega k^{*}(\omega)e^{i(\omega_{0}-\omega)t}\\ &&\times\left[\hat{b}_{0}(\omega)+k(\omega){{\int}_{0}^{t}}\hat{a}(t^{\prime})e^{-i(\omega_{0}-\omega)t^{\prime}}dt^{\prime}\right]. \end{array} $$

(A4)

The final quantum langevin equation for cavity mode a can be written as

$$\begin{array}{@{}rcl@{}} \dot{\hat{a}}&=&-i\left[-\frac{g}{\hbar}\hat{a}\hat{q}+i\varepsilon_{l} e^{-i(\omega_{l}-\omega_{0}) t} +i\varepsilon_{p}e^{-i(\omega_{p}-\omega_{0}) t}\right]\\ &&-\left[\hat{b}_{in}(t)+{{\int}_{0}^{t}}f(t-t^{\prime})\hat{a}(t^{\prime})dt^{\prime}\right], \end{array} $$

(A5)

where the input field is defined as the Fourier transformation of $k^{*}(\omega )\hat {b}_{0}(\omega )$

$$\begin{array}{@{}rcl@{}} \hat{b}_{in}(t)={\int}_{-\infty}^{\infty}k^{*}(\omega)\hat{b}_{0}(\omega)e^{i(\omega_{0}-\omega)t}d\omega. \end{array} $$

(A6)

The kernel f(t − t^′) is given by the correlation function, which takes the form

$$\begin{array}{@{}rcl@{}} f(t-t^{\prime})={\int}_{-\infty}^{\infty} |k(\omega)|^{2}e^{i(\omega_{0}-\omega)t}d\omega. \end{array} $$

(A7)

Suppose the cavity field couple to an environment with Lorentzian spectral density

$$\begin{array}{@{}rcl@{}} J(\omega)=|k(\omega)|^{2}=\frac{\gamma}{2\pi}\frac{\lambda^{2}}{(\omega_{0}-\omega)^{2}+\lambda^{2}}, \end{array} $$

(A8)

where γ is the damping rate and λ is the spectral width of coupling. In the wide-band limit (i.e., λ →∞), the spectral density approximately takes $J(\omega )\rightarrow \frac {\gamma }{2\pi }$, equivalently, $k(\omega )=k(\omega )^{*}\rightarrow \sqrt {\frac {\gamma }{2\pi }}$. This describes the case in the Markovian limit, and the non-Markovianity quantum Langevin equation (A5) reduce to a Markovian quantum Langevin equation as

$$\begin{array}{@{}rcl@{}} \dot{\hat{a}}&=&-i\left[-\frac{g}{\hbar}\hat{a}\hat{q}+i\varepsilon_{l}e^{-i(\omega_{l}-\omega_{0}) t} +i\varepsilon_{p}e^{-i(\omega_{p}-\omega_{0}) t}\right]\\ &&-\left[\hat{b}_{in,M}(t)+\frac{1}{2}\gamma \hat{a}\right], \end{array} $$

(A9)

where the Markovian input field is defined as

$$\begin{array}{@{}rcl@{}} \hat{b}_{in,m}(t)=\sqrt{\frac{\gamma}{2\pi}} {\int}_{-\infty}^{\infty}\hat{b}_{0}(\omega)e^{i(\omega_{0}-\omega)t}d\omega. \end{array} $$

(A10)

Appendix B

Now we rewrite the last equations of (16) as

$$\begin{array}{@{}rcl@{}} \tilde{a}_{s}=\frac{-\varepsilon_{l}(i{\Delta}+\lambda)}{{\Delta}\tilde{{\Delta}}-i\lambda \tilde{{\Delta}}-\frac{1}{2}\gamma\lambda}, \end{array} $$

(B1)

where $\tilde {{\Delta }}={\Delta }-\frac {g}{\hbar }q_{s}$. $|\tilde {a}_{s}|^{2}$ can be calculated as

$$\begin{array}{@{}rcl@{}} |\tilde{a}_{s}|^{2}=\frac{\lambda^{2}{\varepsilon_{l}^{2}}+{\Delta}^{2}{\varepsilon_{l}^{2}}}{({\Delta}\tilde{{\Delta}}-\frac{1}{2}\gamma\lambda)^{2}+\lambda^{2}\tilde{{\Delta}}^{2}}, \end{array} $$

(B2)

Substituting (B2) into the second equation of (16)

$$\begin{array}{@{}rcl@{}} q_{s}=\frac{g}{m{\omega_{m}^{2}}}\frac{\lambda^{2}{\varepsilon_{l}^{2}}+{\Delta}^{2}{\varepsilon_{l}^{2}}}{({\Delta}\tilde{{\Delta}}-\frac{1}{2}\gamma\lambda)^{2}+\lambda^{2}\tilde{{\Delta}}^{2}}, \end{array} $$

(B3)

Substituting q _s into $\tilde {{\Delta }}={\Delta }-\frac {g}{\hbar }q_{s}$ and considering $\tilde {{\Delta }}=\omega _{m}$, we get

$$\begin{array}{@{}rcl@{}} {\Delta}-\frac{{\varepsilon_{l}^{2}} (\lambda^{2} +{\Delta}^{2})}{({\Delta} \omega_{m} - \frac{1}{2} \gamma \lambda)^{2}+\lambda^{2}{\omega_{m}^{2}}}\frac{g^{2}}{\hbar m{\omega_{m}^{2}}}= \omega_{m}. \end{array} $$

(B4)