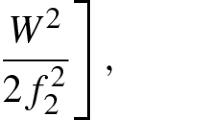
Abstract
The nature of the scalar field responsible for the cosmological inflation, the “inflaton”, is found to be rooted in the most fundamental concept of the Weyl’s differential geometry: the parallel displacement of vectors in curved space-time. The Euler-Lagrange theory based on a scalar-tensor Weyl-Dirac Lagrangian leads straightforwardly to the Einstein equation admitting as a source the characteristic energy-momentum tensor of the inflaton field. Within the dynamics of the inflation, e.g. in the slow roll transition from a “false” toward a “true vacuum”, the inflaton’s geometry implies a temperature driven symmetry change between a highly symmetrical “Weylan” to a low symmetry “Riemannian” scenario. Since the dynamics of the Weyl curvature scalar, constructed over differentials of the inflaton field, has been found to account for the quantum phenomenology at the microscopic scale, the present work suggests interesting connections between the “micro” and the “macro” aspects of our Universe.
Similar content being viewed by others
Notes
Some useful mathematical results. \(W(L)=+1, W(g_{\rho \sigma })=+2, W(g^{\rho \sigma })=-2, W(\sqrt {-g})=+4, W(m\equiv \text {mass})=-1, W(R)=-2\). Here g ρ σ is the metric tensor, g = det(g ρ σ ), and R = R g + R W is the sum of the Riemann and the Weyl curvature scalars. The Riemann covariant divergence of ϕ ρ is \(\phi ^{\rho }_{\mid \rho }=\partial _{\rho }+ {\Gamma }^{\rho }_{\sigma \rho }\phi ^{\sigma }\), where \({\Gamma }^{\eta }_{\sigma \rho }\) are the Christoffel symbols, while the co-covariant derivative of the scalar quantity X is: D ρ (X) = [∂ ρ X − W(X)ϕ ρ X] so that W(D ρ X) = W(X). A useful result is g ρ σ∣σ = g ρ σ;σ = 0. Our signature of g ρ σ is (−, +, +, +). The Laplace-Beltrami operator acting on any function X is defined as : \(\nabla _{B} X\equiv \frac {1}{\sqrt {-g}}\partial _{\rho }(\sqrt {-g}g^{\rho \sigma }\partial _{\sigma }X)\). \(\nabla _{B} \phi \equiv \phi ^{\rho }_{\mid \rho }\). The Weyl curvature scalar in D = 4 is \(R_{W}=6[\phi _{\rho }\phi ^{\rho }-\phi ^{\rho }_{\mid \rho }]\). The sign of the Ricci tensor R ρ σ and of the curvature scalar R = g ρσ R ρ σ is determined by the choice of the lower index of the Riemann-Christoffel curvature tensor \(R^{i}_{klm}\) to be saturated by the upper index. In modern GR Texts (e.g. by: P.A.M. Dirac, E.A.Lord, D.F.Lawden) the tensor \(R_{kl} = R^{i}_{kli}\) is adopted while R k m = −R k l is adopted in other Texts, e.g. by Weyl [8]. In the present work the first option is chosen and this leads to values of R g and of R W opposite to the ones often adopted in the context of the Weyl geometry: (P.A.M. Dirac, General Theory of Relativity (Princeton, 1993); D.F. Lawden, An introduction to Tensor calculus, Relativity and Cosmology (Wiley 1982)).
The correct application of co-covariance was overlooked by Weyl himself after discovering his erroneous interpretation of the electromagnetic field as expressed by the quantity ϕ ρ σ .
The present field conception of the mass is generally adopted by the modern elementary particle physics within the context of the spontaneous symmetry breaking and of the Higgs mechanism. Indeed, the “mass field” considered here is proportional to a corresponding “Higgs field.”
See note 1.
A complete, exact solution of the Euler-Lagrange method applied to the lagrangian L implies the only addition of the term: \((-\frac {1}{2}g_{\rho \sigma }\frac {V(\phi , T)}{\partial \phi })\) within the square brackets at the r.h.s. of Eq. 4.
See note 1.
The exponential solution for the inflaton potential V (K ϕ) proposed in this Text (at pages 139 and 149) for the “quintessence” field coincides with our solution, Eq. 5, which is imposed by the strict co-covariance of the Lagrangian L.
References
Starobinsky, A.A.: Phys. Lett. B91, 99–102 (1980)
Guth, A.H.: Phys. Rev. D 23, 347 (1981)
Linde, A.D.: Phys. Lett. B 108, 389 (1982)
Albrecht, A., Steinhardt, P.J.: Phys. Rev. Lett. 48, 1220 (1982)
Dodelson, S.: Modern cosmology. Academic Press, New York (2003)
Weinberg, S.: Cosmology. Oxford University Press, London (2008)
Weinberg, S.: Gravitation and Cosmology. Wiley, New York (1972)
Weyl, H.: Space, Time, Matter, 4th edn. Dover Publications, Inc., New York (1952)
Lord, E.A.: Tensors, Relativity and Cosmology. Tata McGraw-Hill, New Delhi (1979)
Jammer, M.: Concepts of Mass. Princeton University Press, Princeton (2000)
Quigg, C.: Gauge theories of the strong, weak and electromagnetic interactions. Menlo Park, Benjamin (1983)
Dirac, P.A.M.: Proc. R. Soc. London, Ser. A 333, 403 (1973)
Ehlers, J., Pirani, F.A., Schild, A.: In: O’Raifeartaigh, L. (ed.) General relativity (papers in honour of J. L. Synge), p 63. Clarendon Press, Oxford (1972)
Ehlers, J., Schild, A.: Commun. Math. Phys. 32, 119 (1973)
Trautman, A.: Gen. Relativ. Gravit. 44, 1581 (2012)
De Laurentis, M., Fatibene, L., Francaviglia, M.: Frontiers of fundamental physics and physics education research, pp 127–140. Springer, Berlin (2014)
Scholz, E.: ArXiv:1111.3220 [math.HO] (2011)
Scholz, E.: ArXiv:1206.1559 [gr-qc] (2012)
Fatibene, L., Garruto, S., Polistina, M.: Int. J. Geom. Meth. Mod. Phys. 12, 1550044 (2015)
Salam, A., Strathdee, J.: Ann. Phys. 141, 316 (1982)
Penrose, R., Hawking, S.W.: The nature of space and time. Princeton University Press, Princeton (1996)
Montani, G., Battisti, M., Benini, R., Imponente, G.: Primordial cosmology. World Scientific, Singapore (2011)
Hochberg, D., Plunien, G.: Phys. Rev. D 43, 3358 (1991)
’t Hooft, G.: Int. J. Mod. Phys. D 24, 15430002 (2015)
Amendola, L., Tsujikawa, S.: Dark energy. Cambridge University Press, New York
Santamato, E.: Phys. Rev. D 29, 216 (1984)
Santamatom, E., De Martini, F.: Found. Phys. Lett. 43, 631 (2013)
De Martini, F., Santamato, E.: Int. J. Theor. Phys. doi:10.1007/s10773-013-1651-y (2013)
De Martini, F., Santamato, E.: Physica Scripta T163, 014015 (2014)
De Martini, F., Santamato, E.: Int. J. Quantum Inf. 12, Issue 7 (2014)
De Martini, F., Santamato, E.: J. Adv. Phys. 4, N.3,272 (2015)
Santamato, E., De Martini, F.: Found. Phys. 45, 858 (2015)
De Martini, F., Santamato, E.: ArXiv:1604.06253v1 [quant-ph] (2016)
De Martini, F., Santamato, E.: ArXiv:1609.01149 [gr-qc] (2016)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
De Martini, F., Santamato, E. The Geometrical Nature of the Cosmological Inflation in the Framework of the Weyl-Dirac Conformal Gravity Theory. Int J Theor Phys 56, 4105–4111 (2017). https://doi.org/10.1007/s10773-017-3465-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10773-017-3465-9