1 Introduction

Hydrocarbons represent a fluid class that is among the most relevant ones in process and energy technology. Liquid hydrocarbons can be found as bulk chemicals and working fluids in various technical applications, such as their synthesis in the Fischer–Tropsch process [1] or the use as phase change media for energy storage [2, 3], fuels in combustion processes [4,5,6], and lubricating oils in machineries [7, 8]. For the modeling of heat transfer in such processes, one key property is the thermal conductivity of the involved fluids.

In general, there exists a relatively large experimental database for the thermal conductivity of liquid hydrocarbons in the literature. This holds especially true for linear n-alkanes with a carbon number between 5 and 16 as well as for the cyclic aliphatic and aromatic C6-based compounds cyclohexane and benzene including related derivatives. For these substances, corresponding reference correlations for the thermal conductivity as a function of temperature and pressure were developed by considering reliable experimental data sources and are recommended in the NIST reference database REFPROP [9]. These correlations are associated with typical uncertainties in the liquid state between (2 and 4) %. A considerably smaller experimental database is given for longer-chained and/or branched hydrocarbons such as the C30-based 2, 6, 10, 15, 19, 23-hexamethyltetracosane, commonly known as squalane. The latter compound represents also a potential high-viscosity reference fluid [10], but experimental information on its thermal conductivity is lacking to the best of the authors’ knowledge.

The aim of the present study is the accurate experimental determination of the thermal conductivity of four liquid hydrocarbons at ambient pressure as a function of temperature. In addition to validating and extending the existing database for the thermal conductivity of selected hydrocarbons, this study contributes to a recently started collaborative research project addressing the flow in oil-lubricated rotary displacement compressors, where the oil is in contact with the refrigerant. The efficiency of such compressor types depends strongly on the unavoidable gap flow between the rotating contour and the static housing that is influenced by the thermophysical properties of the oil–refrigerant mixtures [11]. Therefore, one main objective of this project is to improve the modeling of the thermophysical properties of such highly asymmetric mixtures based on the data of their pure components. This should be achieved by gradually increasing the asymmetry of binary model mixtures. In combination with the so-called natural refrigerants propane and carbon dioxide which are alternatives to classical refrigerants such as hydrofluorocarbons, the compounds n-decane (n-C10H22), n-hexadecane (n-C16H34), and squalane (C30H62) serve as model representatives with increasing molecular size on the oil side. For these hydrocarbons, the thermal conductivity data presented here serve as a reference for the corresponding mixture data to be measured later on. Cyclohexane (C6H12) was additionally studied here as a model hydrocarbon with a cyclic structure, which is relevant as, e.g., feedstock for the synthesis of plastic materials [12, 13] or as medium for the production of metallic oxide nanoparticles with dimensions of only a few nanometers [14]. In the present work, absolute measurements on the thermal conductivity of the oil-based compounds were performed by using a steady-state guarded parallel-plate instrument, GPPI [15] in the temperature range from (278 to 353) K at ambient pressure.

In the following, first the sample preparation and a concise description of the GPPI are given. Thereafter, the results of the thermal conductivity measurements of the investigated hydrocarbons are presented and discussed by comparison with existing reference correlations. In the case of squalane, the measurement results are correlated as function of temperature and used to test simple estimation schemes reported in the literature.

2 Experimental Section

2.1 Materials and Sample Preparation

Table 1 summarizes the relevant information about the used hydrocarbon chemicals studied in this work. It lists the molar mass M as well as the source and the specified purity of the samples. The certificates of analysis are given in the Supplementary Information. All samples were used as provided by the manufacturer. The water content of the samples with an expanded (coverage factor k = 2) uncertainty of 10 % was determined via coulometric Karl-Fischer titration (Metrohm 899) before and after the thermal conductivity measurements. In all cases, the average values of the water mass fractions listed in Table 1 are smaller than 10−4 which correspond to values smaller than 100 parts per million (ppm).

Table 1 Specification of the investigated hydrocarbons
Full size table

2.2 Guarded Parallel-Plate Instrument (GPPI): Thermal Conductivity

For the absolute measurement of the thermal conductivity λ of the four hydrocarbons at ambient pressure as a function of temperature T, a steady-state guarded parallel-plate instrument (GPPI) was used. Details of this instrument [15] and its application to the investigation of different fluids [16,17,18] can be found in the references provided. In this section, only the main features of the instrument and the experimental procedure relevant to the present study are described.

The working principle of the GPPI is to represent the ideal one-dimensional form of Fourier’s law of heat conduction for a planar sample as accurately as possible in the experiment. Here, the sample is placed between two parallel circular copper plates at a defined layer thickness. By maintaining a temperature difference ΔT between the two outer surfaces of the sample, a heat flux is transferred from the upper hot plate through the sample to the cold bottom plate as a result of the electrical power dissipated in the upper plate of known heat transfer area. To determine λ in an accurate way, advective heat transfer through the sample, parasitic heat leakages to the surrounding, and radiative heat transfer between the two plate surfaces have to be minimized or considered. Contributions from advection and heat leakages are suppressed to a minimum with the help of the design of the GPPI, cf. Refs. [15, 19].

Radiative heat transfer is reduced by the use of polished plate surfaces covered by a thin chrome layer with an emission coefficient of about 0.04. Since organic compounds are weakly absorbing fluids, the radiation contribution λr needs to be considered in order to accurately determine λ. For this, λr is subtracted from the thermal conductivity value λeff (= λ + λr) measured effectively in the experiment. Following the procedure from our previous works [16,17,18,19,20], λr was estimated by the approach proposed by Braun et al. [21] that is detailed in Refs. [19, 22]. As input parameters, the refractive index and the transmission spectra of the samples in the infrared wavelength range are required. Infrared refractive-index data n for the studied liquids are not available in the literature and are not easy to measure. As an approximation, the refractive index at the sodium vapor line (nD = 589.3 nm) and at the mean temperatures of all λ measurements were used, as collected from Refs. [18, 23]. The transmissions D of the four liquids were measured at ambient conditions with a Fourier transform infrared spectrometer (JASCO FT/IR-4600) for wave numbers ν ranging from (700 to 4000) cm−1 at an assumed layer thickness of 1 µm and were employed consistently for all temperatures studied in the thermal conductivity experiments. The raw data for the transmission spectra are provided in the Supplementary Information. For the investigated systems, the contribution of λr to the measured λeff values was found to be between (0.80 and 2.2) %.

In all thermal conductivity measurements, the sample layer thickness remained constant at (2.06 ± 0.04) mm (k = 2). Details to the procedure for sample filling can be found in Ref. [15]. To exchange the samples between two measurement series, approximately 250 ml of ethanol were circulated through the sample layer. Thereafter, remaining contaminations from the sample layer were removed by increasing T of the GPPI to 343 K and applying a vacuum for at least 0.5 h. Following this drying procedure, the sample layer was flushed with 50 ml of the sample to be investigated prior to the filling. Initially, n-decane was investigated, followed by cyclohexane, n-hexadecane, and squalane. For three ΔT = (2.0, 2.5, and 3.0) K, the thermal conductivity was measured from the lowest to the highest temperature at different mean T, corresponding to the averages between the surface temperatures of the hot and cold plates. While the lowest T investigated was set to 278.15 K for n-decane and squalane, somewhat larger values of 283.15 K for cyclohexane and 298.15 K for n-hexadecane were selected considering the melting points. The upper T investigated was adjusted to be 333.15 K for cyclohexane and 353.15 K for the other three liquids. For all liquids, repetition measurements conducted at (293.15 or 298.15) K after reaching the largest T were found to match the initial λ results clearly within the single experimental uncertainties. At the end, an independent repetition measurement with freshly filled n-decane was conducted to verify if there were changes in the sample thickness, formation of deposits on the plate surfaces, and/or cross-contaminations from previous fillings. The results for λ obtained from the repetition at (293.15, 313.15, and 333.15) K at ΔT = (2.0, 2.5, 3.0) K show excellent agreement with the data from the first measurement set with relative deviations within 0.3 %.

3 Results and Discussion

In this section, the thermal conductivity results for pure cyclohexane, n-decane, n-hexadecane, and squalane at ambient pressure are presented and discussed first. This includes a comparison of the results from this work with existing reference correlations for cyclohexane, n-decane, and n-hexadecane. Thereafter, the measured thermal conductivities of squalane are correlated as a function of T between (278 and 353) K and are compared against selected estimation models.

3.1 Thermal Conductivity of Investigated Hydrocarbons

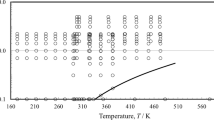
The individual results for the thermal conductivity of pure cyclohexane, squalane, n-decane and n-hexadecane obtained at ΔT = (2.0, 2.5, and 3.0) K are summarized in the Supplementary Information. Since the individual results of same uncertainties agree very well with each other and clearly within their measurement uncertainties for each liquid and state point, an unweighted averaging of the thermal conductivities was performed. These final measurement results for the thermal conductivity with a total expanded (k = 2) measurement uncertainty of 2 % are listed in Table 2 and are illustrated in Fig. 1 as a function of temperature. For the average temperatures T, an expanded (k = 2) uncertainty of 11 mK can be stated based on the procedure discussed in Ref. [15]. In Fig. 1, the reference correlations λref of cyclohexane [24], n-decane [25], n-hexadecane [26] with stated estimated uncertainties of (2.5, 3.0, and 4.0) %, respectively, are also included for comparison.

Table 2 Measurement results for the thermal conductivity λ of cyclohexane, n-decane, n-hexadecane, and squalane at atmospheric pressure as a function of the mean temperature obtained by GPPI for s = 2.06 mm using three different ΔT
Full size table
Fig. 1
figure 1

Thermal conductivity λ of cyclohexane (), n-decane (), n-hexadecane (), and squalane (■) at atmospheric pressure as a function of temperature obtained by GPPI and listed in Table 2. The error bars represent the expanded (k = 2) uncertainties of the measurement results of 2 % which are exemplarily shown for each measurement series. The solid lines indicate the reference correlations for λ of cyclohexane () [24], n-decane () [25], and n-hexadecane () [26]. The dashed line (– –) serves as a guide for the eye for representing the thermal conductivities of squalane

Full size image

Figure 1 shows that the thermal conductivities of the four investigated hydrocarbons cover relatively low values in a total range between about (110 and 145) mW·m−1·K−1 and decrease linearly with increasing T. Moreover, λ increases with increasing carbon number, with the exception of the branched alkane squalane. The latter compound shows thermal conductivities between those of n-decane and n-hexadecane. It has been reported in literature [27, 28] that the thermal conductivity of branched alkanes is lower than that of their linear isomers, indicating the reduced capability of branched compounds to transport thermal energy. It is worth to mention that the experimental λ results for squalane exhibit the weakest temperature-dependent dependency, while the other three linear and cyclic hydrocarbons show steeper and very similar trends.

For cyclohexane, n-decane, n-hexadecane, a very good agreement between the present measurement results and the reference correlations [24,25,26] is observed, cf. Figure 1. As visualized in more detail in Fig. 2, the relative percentage deviations of all measured data from λref are in a range between (− 0.9 and + 1.3) %, i.e., clearly within the experimental uncertainties. In addition, the temperature-dependent trend of the reference correlations is reproduced by the measurement results. A further comparison with available experimental data for the thermal conductivity of the three relevant substances is not in the scope of the present work since all the considered reference correlations have been developed based on critically selected, reliable experimental data sets. Overall, the agreement of the present measurement results with reference data once again demonstrates the reliability of the used GPPI for the absolute determination of the thermal conductivity of fluids.

Fig. 2
figure 2

Relative deviations of the thermal conductivities of cyclohexane (), n-decane (), and n-hexadecane () at atmospheric pressure measured in this work from the corresponding reference correlations [24,25,26] as a function of temperature. The dashed lines represent the expanded (k = 2) uncertainties of λref given by 2.5 % for cyclohexane (), 3.0 % for n-decane () and 4.0 % for n-hexadecane ()

Full size image

3.2 Thermal Conductivity of Squalane

For squalane, the first experimental λ data reported in literature so far are listed in Table 2. Figure 3 depicts the experimental data for λ at ambient pressure as a function of T, together with a simple correlation for the thermal conductivity of squalane. This correlation represents a fit of the measurement results in the form of a polynomial of second-order fit with respect to temperature T in Kelvin between (278 and 353) K according to

$$\lambda_{cor} = \lambda_{0} + \lambda_{1} T + \lambda_{2} T^{2} .$$
(1)
Fig. 3
figure 3

Thermal conductivity λ of squalane at atmospheric pressure measured in this work (■) and correlated via Eq. 1 (—) in comparison with calculated values obtained from the Latini model [29, 30] (– –) and the Sastri model [31] (– ▪ –) as a function of temperature. The error bars represent the expanded (k = 2) uncertainties of the measurement results of 2 % which are exemplarily shown

Full size image

In the fitting, the experimental data were considered with equal statistical weight. Table 3 summarizes the fit coefficients of Eq. 1 and their statistical uncertainties (k = 2). All measured thermal conductivities deviate from λcor within ± 0.28 %, with an  average absolute relative deviation (AARD) of 0.12 %.

Table 3 Coefficients of Eq. 1 and their statistical expanded (k = 2) uncertainties for the correlation of the thermal conductivity of squalane at atmospheric pressure obtained by fit of the present measurement results
Full size table

To check whether common estimation schemes for the thermal conductivity of liquids can represent the experimental behavior, we applied the original version of the Latini model [29, 30] and the Sastri model [31], as described in Ref. [32]. In the latter reference, it is stated that these two empirical models can estimate the thermal conductivity of organic liquids reasonably well with typical deviations from experimental data below 15 %. As input for both models, the required information on the normal boiling temperature at 0.1 MPa, Tb = 695.2 K [33], and the critical temperature Tc = 795.9 K [34] were taken from measurement results for squalane reported in the literature. Furthermore, the molar mass M provided in Table 1 is included in the Latini model.

As can be seen from Fig. 3, the Latini model [29, 30] overestimates the experimental λ values of squalane by around +6.9 % at 278.15 K and +2.8 % at 353.15 K, while the Sastri model [31] gives underestimations by −5.4 % at 278.15 K and −7.1 % at 353.15 K. In both cases, the T-dependency of the thermal conductivity is somewhat too large in comparison to the experimental behavior. The values for λ calculated from both models are also very sensitive to minor variations in Tb and Tc. This demonstrates that accurate experimental data are indispensable for testing and further developing prediction or, better to say, estimation models for the thermal conductivity of liquids, in particular for relatively long-chained hydrocarbons such as squalane.

4 Conclusions

The present work provides measurement results for the thermal conductivity of liquid cyclohexane, n-decane, n-hexadecane, and squalane at atmospheric pressure as a function of temperature up to 353 K with an expanded (k = 2) uncertainty of 2 %. The comparison of the measured thermal conductivities of cyclohexane, n-decane, and n-hexadecane with existing reference correlations revealed very good agreement with a maximum deviation of 1.3 %. This validates the performance of the used steady-state guarded parallel-plate instrument for the study of liquids with relatively low thermal conductivity and weak absorption of radiation, where thermal radiation effects need to be considered. In the case of squalane between (278 and 353) K, a new experimental database for the thermal conductivity was established and tested against common estimation schemes that fail in predicting the behavior quantitatively. Thus, the results from this work can serve as a reference not only for testing and improving corresponding correlation schemes, but also for future investigations on the thermal conductivity of binary mixtures with so-called natural refrigerants such as carbon dioxide and propane.

5 Supplementary Information

Raw data related to the thermal conductivity measurements and infrared spectra are available in two Microsoft Excel files. The file with the name “1_Raw_data_thermal_conductivity_hydrocarbons.xlsx” contains the raw data for the thermal conductivity measurement of pure cyclohexane, n-decane, n-hexadecane, and squalane, while the raw data for the infrared spectra are given in the file “2_Raw_data_infrared_spectrum_hydrocarbons.xlsx”.