Optical $n(p,\ T_{90})$ Measurement Suite 1: He, Ar, and N$_2$

Abstract

An $n(p,\ T_{90})$ measurement suite is reported for the gases helium, argon, and nitrogen. The methodology is optical refractive-index gas metrology, operating at laser wavelength $633\ {\text {nm}}$ and covering the temperature range $(293< T < 433)\ {\text {K}}$ and pressures $p < 0.5\ {\text {MPa}}$. The measurement suite produces several things of thermophysical interest. First, the helium dataset deduces the effective compressibility of the apparatus with a relative standard uncertainty of $1.3 \times 10^{-4}$. Next, the argon dataset determines $T - T_{90}$ with a relative standard uncertainty of about $3\ \upmu {\text {K}}{\cdot }{\text {K}}^{-1}$. (The implementation is relative primary thermometry; $T - T_{90}$ is the difference between thermodynamic temperature and ITS-90.) Finally, the nitrogen dataset estimates the temperature dependence of polarizability within $3.5\ \%$ relative standard uncertainty. As a by-product of the nitrogen and argon measurements, values of the second density virial coefficient $B_{\rho }(T)$ are derived with uncertainties smaller than those of previous experiments. More broadly, the work enables conversion of a measured refractivity at known temperature to optical pressure within $3.5\ \upmu {\text {Pa}}{\cdot }{\text {Pa}}^{-1}$ across the stated range, albeit traceable to the diameter of a piston-gage.

Appendices

Appendix 1: Derivation of Eq. 1

Resonance occurs in a two-mirror Fabry–Perot cavity when

$$\begin{aligned} 2 \pi m = \frac{4 \pi L}{\lambda } + 2 \phi + \Phi . \end{aligned}$$

(10)

Here, m is the integer mode number, L is the separation between the front facets of the mirrors, $\lambda = \frac{c}{n \nu }$ is resonant wavelength, with c the speed of light in vacuum, $\nu$ the optical frequency, and n the refractive index of the medium between the mirrors. The treatment above includes phase-shifts caused by mirror reflection $\phi$ and Gouy phase $\Phi$. It is the dependence of both these phase-shifts on refractive index that leads to small departures from Refs. [6, 11] mentioned in Sect. 2, as now explained.

The reflection phase-shift $\phi = \phi _0 + 2 \pi \tau (\nu - \nu _{\text {c}})$ can be modeled having a linear dependence on frequency near the center frequency of the mirror $\nu _{\text {c}}$. Here, $\phi _0$ is the phase-shift at the center frequency of the mirrors, and equals $\pi$ when the high-index layer is outermost, and faces the incident laser. As one operates away from $\nu _{\text {c}}$, the $\phi (\nu )$ dependence is described by the mirror group delay $\tau = \frac{1}{2 \pi }\frac{\text {d} \phi }{\text {d} \nu }$. The group delay $\tau$ is customarily specified with vacuum as the incident medium. However, $\tau$ is medium dependent [68], and $\tau _{\text {gas}} = n \tau$ when the high-index layer is outermost.

For a Gaussian beam propagating in the z direction, the Gouy phase-shift is $\Phi (z) = \arctan ( z / z_r)$ [69]. In Gaussian optics the Rayleigh length is defined $z_r = \pi n w_0^2 / \lambda$ by the beam waist $w_0$ at the focal point, and a related quantity is the beam-front radius of curvature $r(z) = z_r^2/z + z$. When an input laser is mode-matched to a plano-concave FP cavity of length L, the cavity mode has a radius of curvature $r = z_r^2 / L + L$, which leads to the customary vacuum result $z_r = \sqrt{(r - L)L}$ in classic texts [69]. However, in operation a FP cavity refractometer adjusts frequency to maintain constant wavelength, and by definition $z_{r,\text {gas}} = n z_r$. So, when mode-matched in vacuum $\Phi = \arctan \left[ \sqrt{L /(r-L)} \right]$ and in gas $\Phi _{\text {gas}} = \arctan \left[ \frac{1}{n} \sqrt{L/(r-L)} \right]$. Taylor expansion of $\Phi _{\text {gas}}$ about the nominal geometry $\sqrt{L / (r-L)}$ shows $\Phi _{\text {gas}} = \Phi - (n - 1)\frac{z_r}{r}$.

Returning now to Eq. 10, the resonance frequency at vacuum can be written

$$\begin{aligned} \nu _{\text {vac}} = \frac{c}{2L + 2 \tau c}\left( m - \frac{\phi _0 - 2 \pi \tau \nu _{\text {c}}}{\pi } + \frac{\Phi }{2 \pi } \right) . \end{aligned}$$

(11)

From the $\phi (n)$ and $\Phi (n)$ explanations above, the resonance frequency in gas (ignoring compression of L) becomes

$$\begin{aligned} \nu _{\text {gas}} = \frac{c}{2nL + 2 n \tau c}\left[ m + \Delta m - \frac{\phi _0 - 2 \pi n \tau \nu _{\text {c}}}{\pi } + \frac{\Phi - (n - 1)\frac{z_r}{r}}{2 \pi } \right] , \end{aligned}$$

(12)

with $\Delta m$ being the integer change in mode number. Subtracting Eq. 12 from Eq. 11 and solving for refractivity yields

$$\begin{aligned} n - 1 = \frac{(\nu _{\text {vac}} - \nu _{\text {gas}}) (1+\epsilon _{\tau }) + \Delta m \dfrac{c}{2L}}{\nu _{\text {gas}} + \epsilon _{\tau } (\nu _{\text {gas}} - \nu _{\text {c}}) + \dfrac{c}{2 \pi L}\dfrac{z_r}{r} }, \end{aligned}$$

(13)

with the parameter $\epsilon _{\tau } = \frac{\tau c}{L}$ for two mirrors. The approximation $\nu _{\text {gas}} \approx m \frac{c}{2\,L}$ introduces the parameter $\epsilon _{\text {d}} = \frac{z_r}{r}\frac{1}{\pi m}$ to Eq. 1. Finally, Eq. 1 is produced from Eq. 13 by adding the distortion term $n \kappa \Delta p \equiv n \frac{\Delta L}{L}$ to the right-hand side of Eq. 13 and solving for $n - 1$.

Appendix 2: Details on the Helium Analysis

The constant density, fractional change in frequency was extrapolated to time-zero using a representative function

$$\begin{aligned} \left( \frac{\Delta f}{\nu } \right) _{\rho } (t) = \frac{1}{\nu } \left[ f_0 + \beta \left( t^{\frac{1}{2}} - \frac{\pi }{16} \beta t \right) \right] . \end{aligned}$$

(14)

This function is physically-motivated by the total amount of substance entering a solid absorbing rod, at short times, when exposed to constant surface concentration, with the initial condition of a radially-distributed internal concentration [70]. Using Eq. 14, the effect of helium diffusion on cavity length is described by a single parameter $\beta$. The parameter is plotted in Fig. 8(a) for all pressures and temperatures in the helium $n(p,\ T_{90})$ dataset. The parameter was free for all pressures and temperatures, and any specific $\beta (p,\ T)$ exhibited deviation of about $2\ \%$ on the mean value. Deviation on the mean value $\beta$ is expected; for example, because internal concentration changes, or because of correlation in the fit parameters. The purpose of the fit is to individually best describe (in the least squares sense) helium diffusion in each $(\frac{\Delta f}{\nu })_{\rho }$ data series. In this context, deviation on the mean value of $\beta$ is not an uncertainty. Next follows discussion about $u(\beta )$.

Uncertainty in $\beta$ is the largest part of $u(\kappa )$, and has three contributions. The first component is statistical error in the regression of Eq. 14, identifying $f_0$ and $\beta$. Relative statistical error in the fit parameter $\beta$ is shown in Fig. 8(b). Combined, statistical uncertainty in $f_0$ and $\beta$ contributes less than $2 \times 10^{-6} {\cdot } \kappa$. For any $(p,\ T_{90})$, the statistical uncertainty in $f_0$ and $\beta$ is considerably smaller than the standard deviation errorbars in Fig. 2(b). The combined statistical and systematic uncertainty in $\kappa (T)$ is now explained.

Fig. 8

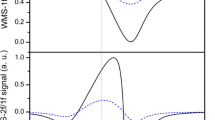
(a) Fit coefficient $\beta$ for the helium diffusion in Eq. 14, and (b) its statistical error as a function of pressure and temperature. (c) Change in constant-density fractional frequency and temperature immediately after a charge of $0.3\ \text {MPa}$ helium. (d) Temporal evolution of difference between calculated $\frac{p}{T}\frac{3 A_{\text {R}}}{2 R}$ and measured $\frac{\Delta f}{\nu }$ refractivities. Deviation from constant value is attributed to systematic error in Eq. 14 describing the effect of diffusion on cavity length. The shaded area spans standard deviation on five repeat measurements

The second and largest component contributing to $u(\kappa )$ is error in the model of Eq. 14. This error shows as transient effects in the numerator of Eq. 2, the difference between calculated $n_{\text {calc}} \approx 1 + \frac{p}{T}\frac{3 A_{\text {R}}}{2 R}$ and measured $n_{\text {meas}} \approx 1 + \frac{\Delta f}{\nu }$ refractive indexes. The model error is visualized in Fig. 8(c) and (d), for representative helium data at $p = 0.3\ \text {MPa}$ and $T_{90} = 373\ \text {K}$. Figure 8(c) shows the fractional change in resonant frequency and thermometer reading immediately after the helium charge. To be clear: $\beta$ is signed positive, and diffusion causes cavity length to increase and the resonant frequency in gas to decrease; the increasing frequency of Fig. 8(c) is because the refractometer framework has $(\nu _{\text {vac}} - \nu _{\text {gas}}) \rightarrow \Delta f$. Figure 8(d) shows how the difference $n_{\text {calc}} - n_{\text {meas}}$ changes over time. Model error is the deviation of $n_{\text {calc}} - n_{\text {meas}}$ from constant value for times after settled. Model error may arise because Eq. 14 describes a rod; however, it is likely that thermal disturbance and gradients at $t < 0.2\ \text {h}$ have some influence, as well as gradual build-up of gas impurities. These transient effects and model error explain the large errorbars in Fig. 2(b), where it is clear deviation becomes larger at higher temperatures.

Finally, a third contributor to $u(\kappa )$ is irreproducibility in $n_{\text {meas}}$. Standard deviation for the five repeat $n_{\text {meas}}$ measurements shows as the shaded band in Fig. 8(d). The contribution of irreproducibility in $n_{\text {meas}}$ is about $3 \times 10^{-5} {\cdot } \kappa$.

To summarize combined $u(\kappa )$ by reference to Fig. 8(d): irreproducibility in $n_{\text {meas}}$ plus statistical error $f_0$ and $\beta$ is covered by the shaded area, and model error is covered by taking half the range of excursion for $(0.3< t < 1.1)\ \text {h}$.

[An aside: the wire suspension of Fig. 1 provides frictionless support of the cavity, and is an essential attribute of reproducible compressibility. Achieving the $1\ {\text {mm}}$ clearance between metal surfaces and a swinging block of glass requires care. An initial characterization found semi-reasonable results for $\kappa (T)$, but some peculiarities in the $T - T_{90}$. It was found that one end of the cavity was resting on the bottom of the chamber. The effect was investigated more deliberately. The cavity was taken off the suspension wires, and mounted on a pair of PTFE shims placed at the Airy points. The cavity rested on the bottom of the chamber, and the glass-to-aluminum contact was mediated by the two PTFE-to-PTFE mounting points. A full characterization in helium suggested $\kappa _{303}$ increased $0.5\ \%$ and $k_1$ increased $7\ \%$. The argon dataset was unreliable, exhibiting clear $\pm 2\ \upmu {\text {Pa}}{\cdot }{\text {Pa}}^{-1}$ quartic nonlinearity in the regression of Eq. 5, and temperature-cycle hysteresis of $11\ \upmu \text {K}{\cdot }\text {K}^{-1}$ in the estimate of $T - T_{90}$.]

Appendix 3: About Outgassing

Gases of purity $99.9999\ \%$ were employed for all measurements. The wait time between gas filling and the $(\Delta f,\ p,\ T_{90})$ sample acquisition allowed build-up of impurity in the initially pure gas volume. One way to observe the build-up of impurity is to study the resonant frequency of the cavity when filled with gas. The analysis is best done via the constant density effective difference frequency $(\frac{\Delta f}{\nu })_{\rho }$, which corrects fractional changes in resonant frequency to a condition of constant pressure and temperature. (Effectively, the correction is for changing temperature because the piston-gage generates nearly constant pressure by regulating volume.) At constant density, a pure volume would have constant refractivity, and $(\frac{\Delta f}{\nu })_{\rho }$ should be constant over time. Deviation from constant $(\frac{\Delta f}{\nu })_{\rho }$ means that refractivity is changing, most likely because of impurity build-up through outgassing. (The positive pressures of this work preclude leaks as a mechanism for impurity. Total gas volume was about $2\ \text {L}$, with $0.7\ \text {L}$ in the chamber.)

Estimates of outgassing rates in the argon and nitrogen datasets are shown in Fig. 9. The ordinate is rate of change with respect to time on the constant density effective difference frequency $(\frac{\Delta f}{\nu })_{\rho }$, with correction for temporal drift in vacuum cavity length $\frac{\text {d} L}{L}$. The $\frac{\text {d} L}{L}$ is plotted as a dashed line; Fig. 2(d) shows that the $\frac{\text {d} L}{L}(T)$ trend was highly reproducible between the argon and nitrogen isotherms. Several qualitative comments can be made about Fig. 9:

• Outgassing is a minor problem in some cases. As a representative example: at $0.25\ \text {MPa}$ and $373\ \text {K}$, nitrogen refractivity is $n - 1 \approx 5.4 \times 10^{-4}$, outgassing from Fig. 9 is $6 \times 10^{-10}\ \text {h}^{-1}$, and the settling-time of the apparatus allows acquisition of a $(\Delta f,\ p,\ T_{90})$ sample within $1\ \text {h}$. So, without correction, outgassing would contribute $1.1 \times 10^{-6} {\cdot } (n - 1)$ error on the measurement of nitrogen refractivity.

• The major constituent of outgassing would be water vapor, which has a molar refractivity of $3.8\ {\text {cm}}^{3}{\cdot }{\text {mol}}^{-1}$, relatively smaller compared to nitrogen $4.4\ {\text {cm}}^{3}{\cdot }{\text {mol}}^{-1}$ than compared to argon $4.2\ {\text {cm}}^{3}{\cdot }{\text {mol}}^{-1}$. For all isotherms, Fig. 9 shows outgassing in nitrogen decreasing the refractivity of the initially pure gas volume. By contrast, argon isotherms $T_{90} < 393\ \text {K}$ show no observable outgassing. It appears that the impurity mixture outgassing in the sealed chamber is (fortuitously) very close to the molar refractivity of argon.

• For the $T_{90} = 433\ \text {K}$ isotherm (and to less extent the $T_{90} = 413\ \text {K}$ isotherm), impurities of molar refractivity larger than argon begin to build-up. The likely cause is polymeric hydrocarbon release [71], either from the elastomer o-rings or the wire insulation to the cSPRT.

Fig. 9

Estimate of impurity build-up over time in the argon and nitrogen datasets. Each marker is the average over 5 repeats at the 19 generated pressures, and the barely visible errorbars show standard deviation

Based on Fig. 9 and trends in $(\frac{\Delta f}{\nu })_{\rho }$, linear extrapolations to time-zero were applied to the argon and nitrogen datasets. For argon, corrections were applied to the two isotherms $T_{90} > 413\ \text {K}$. For nitrogen, corrections were applied to all isotherms. The linear extrapolation reduces error caused by outgassing and impurity to less than $0.2 \times 10^{-6} {\cdot } (n - 1)$.

Finally, as explained in Appendix 2, the $(\frac{\Delta f}{\nu })_{\rho }$ for helium is not constant because permeation changes the cavity length. Based on observations for argon and nitrogen in Fig. 9, it is certain that the $(\frac{\Delta f}{\nu })_{\rho }$ of helium has a small additional component caused by outgassing, which could cause error up to $10^{-5} {\cdot } (n - 1)$. However, the helium data is also temporally corrected by a diffusion-motivated fit to $(\frac{\Delta f}{\nu })_{\rho }$. The diffusion correction simultaneously cancels most the effect of outgassing, but the correction is imperfect and residual (model) errors can be larger than $3 \times 10^{-6} {\cdot } (n - 1)$. More details in Appendix 2. Furthermore, helium diffusion presents a possible problem to the measurement sequence, because the cavity has absorbed so much gas that it takes a long time to return to its natural drift rate. Figure 10 shows nitrogen was measured after helium, which was not ideal. The cavity had to be left at $160\ ^{\circ }\text {C}$ for 15 days for “all” the helium to be released. The reported measurement sequence could allow residual helium released from the glass to slowly contaminate the nitrogen (reduce its refractivity), which might explain some behavior in Fig. 9. This is not believed to be the case, because throughout extensive characterization of this system nitrogen has consistently been observed to have a weakly decreasing refractivity as a function of time. Furthermore, the drift rates on cavity length in Fig. 2(d) suggest that possible residual helium release would have been very small (i.e., because the vacuum drift rate during the nitrogen isotherms was similar to the vacuum drift rate during the argon isotherms).

Appendix 4: Crosschecks on Resistance Thermometry

Between the gas datasets, the resistance of the cSPRT used to infer gas temperature ($R_{5053}$) was repeatedly checked at the ITS-90 fixed-points of water and gallium. Two water triple-point cells were employed, one of which was capable of internal distillation. The water and gallium fixed-points were maintained in the same lab in which the RIGT took place. Therefore, repeated checks on the cSPRT resistance were accomplished with the same resistance bridge and cabling, and minimal disruption. Furthermore, a second cSPRT ($R_{4686}$) was repeatedly checked: this second cSPRT was not used in the RIGT, and stayed at room-temperature (when not cycled on the fixed-points). The combination of repeated checks of two cSPRTs in multiple fixed-points using the same (undisturbed) bridge allows reasonable assessment between drifts in the cSPRTs, the fixed-points, and the bridge.

Fig. 10

Relative change in resistance of the two cSPRTs $R_{5053}$ and $R_{4626}$ at the ITS-90 fixed-points of water (TPW) and gallium (GaMP)

The synopsis of the cSPRT crosschecks are shown in Fig. 10, and the plot is annotated with the activity ongoing between the resistance checks. The synopsis is shown as relative change from the initial resistance in either water or gallium. Before the initialization of $R_{5053}$, there was some 260 days refining procedure and system testing, in which system temperature was cycled between $293\ \text {K}$ and $433\ \text {K}$ multiple times. These data support the statement in Table 3 that reproducibility and stability for the cSPRT and resistance bridge was better than $0.2\ \upmu \text {K}{\cdot }\text {K}^{-1}$.