More necessary and less sufficient: an age-period-cohort approach to overeducation from a comparative perspective

Abstract

In many countries, the skilled labor market has lagged educational expansion. As a result of increased competition, younger cohorts of the highly educated face decreasing returns to education or overeducation. Surprisingly, decreasing occupational outcomes do not coincide empirically with the economic returns among those with tertiary education. Regarding the process of changes in economic returns to education based on cohort transformations, we expect that the expansion of tertiary education affects specific cohorts, which find themselves facing more labor market competition. As a result, the economic returns to education should decrease among younger cohorts even when the overall returns to education remain stable over time. To study this process, we model economic returns with a new age-period-cohort-trended lag (APCTLAG) method, which allows us to compare the gap in economic returns between tertiary and less than tertiary education over cohorts. Using the Luxembourg Income Study (LIS), we analyze trends over three decades in 12 countries. Our results confirm that educational returns for tertiary education have declined over time, even though the gap between the educated and the less educated has remained similar in most of the countries. For younger cohorts, tertiary education has become more necessary to survive in the competitive labor market, but the actual economic returns have decreased—making tertiary education less sufficient than before.

Introduction

This paper provides new evidence on the variation of returns to education cohorts and countries in light of educational expansion and changes in the labor market. Economic returns to higher education have an important role in determining the individuals’ chances later in life. Moreover, changes in the returns to education might affect social inequality, especially if they are associated with changes in inequality of educational opportunities. The massive expansion of higher education at the second half of the twentieth century and especially since the 1990s led to concerns about a possible decline in returns to education due to the expansion, and if the labor market demand for workers with higher education would be sufficient to meet the supply of such workers (Mills 1953; Smith 1986). This is known in the literature as the prediction of “overeducation” (Freeman 1976; Sicherman 1991) or “educational inflation” (Collins 1979). Overeducation occurs when attainment of higher levels of education increases, due to educational expansion, at a faster pace than the demand for educated workers in the labor market. Consequently, younger, better-educated cohorts face more competition in the labor market than earlier cohorts, forcing them to settle for less prestigious jobs with lower wages.

The fear of declines in the returns to education has gained much political and academic attention (Ioakimidis 2018; Mateos-Romero and del Mar Salinas-Jiménez 2018; Knight et al. 2017). However, the empirical results are somewhat contradicting: educational expansion coincided in many countries with a decrease in occupational outcomes of tertiary education holders, whereas economic returns increased at the same time (Bernardi and Ballarino 2016). Furthermore, the gap in economic returns between those with tertiary and non-tertiary education has widened (Bernardi and Ballarino 2016). This may be driven by increasing difficulties of people with postsecondary education to attain the same level of occupational status as previous cohorts, although they still earn more than people with a lower level of education. In their recent book, Bernardi and Ballarino (2016) report that in eight countries, the occupational level of the highly educated relative to those with lower levels of education has decreased over time, while economic returns decreased only in three countries.

In this paper, we focus on the cohort transformations in the economic returns to education. To date, cohort and period trends have only rarely been separated in studies on returns to education. Economic returns tend to be sensitive to age and period effects, as bonuses complementing earnings vary according to an employee’s experience as well as the economic performance of a company (or, more generally, with the economic cycle). However, not accounting for period effects may result in the overestimation of economic returns to education. For instance, in times of overall economic prosperity, economic returns to education are expected to increase, even if the occupational returns are low. Therefore, structural and temporal economic changes can affect the measurement of economic returns to education.

We also distinguish between absolute and relative economic returns to education (Rotman et al. 2016). Relative returns refer to the income gap between those with tertiary and non-tertiary educational attainment, whereas absolute returns indicate returns to higher education of current cohorts relative to the returns in previous times or of older cohorts. Both types of returns do not necessarily covary over time. For instance, absolute returns to education might decrease over time and cohorts, and relative returns might increase due to the crowding out effect of those with lower levels of education (Ben-David 2009). This situation illustrates a grim scenario, in which tertiary education becomes crucial for preventing downward mobility (i.e., it becomes more necessary), but is unable to provide better chances for upward mobility (less sufficient).

The paper is divided into three parts. First, we review previous findings regarding educational expansion and returns to education. Next, we estimate the educational expansion and the changes across cohorts in the returns to tertiary education for a number of countries. To do so, we use recent developments of age-period-cohort models that are able to account for cohorts trends in the dependent variable (APCTLAG). We then estimate, using linear regression, the effect of educational expansion and skill-biased technological change (SBTC) on the returns to education, for a smaller sample of 10 countries. Finally, we discuss the results and claim that, overall, the educational expansion decreased the economic returns to education, even though the trend is not identical in all countries.

Returns to education

Returns to education are usually regarded as the individual economic advantages based on a person’s level of education (Card 1999; Tomlinson 2017). These returns may diverge across educational levels due to labor market mechanisms that assign high-paying jobs to people with higher education and lower-paying jobs to those with less education. Some scholars have argued that this process is due to specific skills that are acquired in the process of education (Mincer 1958)—students learn important skills that might help them later in their work and are compensated for their newly acquired skills (Becker 1962; García-Aracil and Van der Velden 2008). Others claim that education is merely a sorting mechanism that students employ to demonstrate their skills (Spence 1973; Assaad et al. 2017) or other attributes that employers are willing to pay for (Bruin 1999).

Hence, returns to education are strongly affected by the supply and demand in the labor market (Machin 2009). According to a simple model of supply and demand, with an increase in the demand for highly skilled workers, returns for education are expected to increase. Similarly, when there is a rise in the supply of highly skilled workers (i.e., more people attaining higher education), returns for education are expected, ceteris paribus, to decline. In the last few decades, a rise in the supply of highly skilled workers was a very common situation, usually referred to in the literature as “educational expansion” (Brown 2001). However, its consequences turned out to be more complicated than the theoretical model had implied.

Educational expansion

The expansion of tertiary education is one of the major societal changes of the twentieth century (Calderon 2012; Sarrico 2017), for which several non-exhaustive explanations have been suggested. According to Schofer and Meyer (2005), the rate of enrollment in higher education (as the share of relevant cohort) has globally increased by more than 200% over the past century. The major part of the expansion occurred in the second half of the century and has continued until today. While post-secondary education was once a privilege reserved only for the elite, it is common among young cohorts today, even in less-developed countries (Hannum and Buchmann 2005; Sarrico 2017).

Economic theory suggests that the expansion of tertiary education is a result of an increase in the demand for skilled labor (Keep and Mayhew 1996; Béduwé and Planas 2003). During the last few decades, the labor market has become increasingly dependent on skilled workers. New industries and occupations emerged based on the development of information technology, and thus the need for professional occupations has increased (Berman and Machin 2000; Goldin and Katz 2009; Kristal 2013). This process is known as skill-biased technological change (SBTC). At the same time, the professionalization of management has augmented the demand for educated managers (Shenhav 1995). According to this explanatory framework, educational expansion thus is, in part, a result of labor market demand in new and developing occupations (Konstantinovskiy 2017). However, even after the demand for these occupations decreased, the supply of highly skilled workers persisted to increase (Beaudry et al. 2014, 2016), due to adaptive expectations (Giannini 2003).

In contrast, sociological theories have traditionally put a higher weight on non-economic factors, and three distinct strands of literature focus on public policy, political negotiation and institutional change as the forces behind educational expansion. One strand of literature claims that the expansion of tertiary education was driven in most countries by direct public policy rather than by the free market. With a few exceptions, governments especially in Europe were able to accelerate the development of tertiary education institutions through increasing the number of available higher education opportunities and encouraging student enrollment. Similarly, tuition fees also decreased in many Western countries, despite important exceptions such as the UK.

In some cases, the public policy related to educational expansion focused not only on the educational supply, but also on the degree structure. In most European countries, the Bologna process (Witte 2006) has made (directly and indirectly) changes easier and created new types of tertiary education degrees, sometimes transforming former post-secondary education diplomas into academic degrees, or creating new academic intuitions, usually with less prestige than the former ones. In Germany, for example, post-secondary (Berufsakademien) were able to grant bachelor’s degrees (Witte 2006). In Finland, the process has led to the introduction of the bachelor’s degree, whereas before only the master’s degree existed. As a result, bachelor’s students who were formerly considered dropouts are now considered tertiary-educated (Välimaa et al. 2007). In other countries, such as the Netherlands, the UK and France, the process has enabled less prestigious institutions to gain more prestige and to attract more students (Witte 2006).

A second strand of literature suggests that this expansion was a result of a political struggle between groups (such as classes, strata and other social groups) who wanted to ensure better mobility chances for their children (Collins 1971). Such groups fought to expand educational opportunities, regardless of market conditions and needs. A third aspect of the literature, also suggesting that educational expansion is not a result of labor market demand, focuses on institutional change. Schofer and Meyer (2005) explained the expansion of tertiary education as a result of four global institutional changes: (1) democratization, which increased demands for tertiary education as a civil right; (2) the scientization of society that magnified the importance of the role of schooling and science in society; (3) the rise of national development logics, which perceived educational expansion as a means of progression; and (4) the rise of the global diffusion of pro-educational cultural models. All of these explanations undermine the role of the market in the expansion of education. Thus, in the subordination of labor market rationales, educational expansion may result in a mismatch between highly skilled labor supply and demand, which we refer to here as “overeducation” (Collins 1979; Sicherman 1991). In contrast, some scholars only refer to overeducation as a mismatch between the level of education needed for a specific job and the actual level of education utilized in the job (Di Stasio et al. 2016). However, this definition implies that the skills required for each job are orthogonal to the market demands.

Expansion and changes in returns to education

Collins (1979) claimed that the expansion of tertiary education would lead to “credential inflation.” He argues that tertiary education would become the modal education, with insufficient high-skill jobs added to the labor market to meet the resulting demand. Competition over jobs would increase, leading to higher requirements for each job. Collins predicted that, in the future, even a technical position would require a doctorate degree. Collins’s argument also implies that educational expansion will lead to decreasing returns to tertiary education.

Halaby (1994) noted that overeducation could manifest itself in various forms. First, the result of competition could lead to fewer economic returns to skilled jobs, such as reduced wages for professional occupations. In this scenario, the relative economic returns, as the ratio of the wages of the tertiary-educated to the less-than-tertiary-educated, may actually increase, namely in cases where lower-educated workers are crowded out of their jobs by their better-educated counterparts.

Second, it is also possible that, similar to Collins’ (1979) prediction, the occupational returns to education decrease: under the condition that there are fewer possibilities for tertiary education holders to acquire a (prestigious) job that fits their skills. In this scenario, absolute economic returns to education, as well as the gap between those with low and high educational levels, should remain stable or even decrease, in cases where the highly skilled cannot compete for low-skill jobs (Sakamoto and Powers 1995).

Therefore, it is necessary to distinguish between absolute and relative returns. Since a decrease in the occupational standing of the tertiary-educated may, in the long run, lead to a crowding out of those with lower levels of education, the latter might find themselves pushed out of the labor force or into precarious jobs due to competition with the former group. Consequently, this will lead to an increasing gap between the two groups, and for maintaining the economic status quo, education will become “more necessary.” Further, returns to tertiary education holders should diminish over cohorts, with returns to non-tertiary-educated individuals diminishing even more rapidly, resulting in education to be “less sufficient.” In that case, tertiary education will become more necessary but less sufficient to succeed in the labor market than before. Accordingly, regarding returns to education, we hypothesize:

• H₁: Tertiary education becomes more necessary: Educational expansion increases returns to higher educational levels relative to those with less education.

• H₂: Tertiary education becomes less sufficient: Educational expansion decreases returns to higher educational levels relative to those of older cohorts.

The distinct results obtained for the two types of returns stems from common problems in measuring overeducation. First, cohort trends in returns to education have often been ignored. Most studies analyze the differences between two or more time points in the association between education and income or occupational score. However, while educational attainment remains fairly stable across time, occupational attainment does not. In other words, educational expansion concerns mostly younger cohorts, namely those at the age of graduation from their last educational level. Second, temporary changes in the labor market might affect returns to education for a short period, without really influencing them in the long run. Therefore, ignoring cohort trends in measuring returns to education might lead to biased results.

SBTC and changes in returns to education

As noted, empirical studies on overeducation have not yielded conclusive results to date. In many countries, there is strong evidence supporting overeducation, regarding occupational returns, but much less in terms of economic returns. That is, while tertiary-educated persons tend to be more often employed in occupations that do not match their credentials, their economic returns from education do not decrease. Recent studies (Bernardi and Ballarino 2016) have found that occupational returns to post-secondary education holders decreased in Spain, Italy, Sweden, Israel, the Netherlands, Russia, France and Japan. However, economic returns to education only decreased in Sweden, France and Norway. In contrast, economic returns to education actually increased in Hungary, the Netherlands, Russia and Israel. These trends seem to be due to an increase in the demand for highly skilled workers (SBTC), which occurred in parallel to the education expansion.

Crivellaro (2016) used an IV strategy to study the effect of supply and demand for higher education on the returns to education in 12 European countries. He finds that the increase in the demand (which is usually related to SBTC) had only a non-significant positive effect on the returns for education, once the educational expansion was controlled for. Hence, according to his findings, the effect of SBTC is mediated by the educational expansion.

Davia et al. (2016) found similar results in an international comparison of 25 European countries. They discovered that, while educational expansion is associated with occupational overeducation, the ratio of supply to demand for highly skilled labor has an important role in increasing overeducation. Countries that experience SBTC (which increased the demand for highly skilled labor) face lower levels of overeducation. Unfortunately, Davia and colleagues, as well as Crivellaro, did not directly measure overeducation in terms of economic returns.

In sum, regarding the effect of educational expansion, we hypothesize:

• H₃: Barring SBTC, educational expansion will decrease returns to education over cohorts.

• H₄: In cases when substantial SBTC occurs, educational expansion will increase returns to education over cohorts.

Data, variables and methods

Data

Our analysis is based on data of the Luxembourg Income Study (LIS), which to our knowledge is the best available cross-nationally comparable data source providing harmonized demographic and economic information across a time span of more than three decades (Gornick 2014). The data contain detailed information on the respondents’ household income as well as other important demographic variables such as age, education and gender. We construct 5-year cohorts based on the year of birth from 1940 to 1980 and follow them over the period from 1980 until 2010. The data are structured in a pseudo panel design, similar to the one suggested by Deaton (1985), Deaton and Paxson (1994) and Verbeek and Vella (2005). In the pseudo panel cohort design, which is also referred to as a Lexis table (Carstensen 2007), it is possible to follow different cohorts over their life course, even if the individual respondents are drawn from different samples.

To increase our standard of harmonization, we limit our comparison to the 12 industrialized countries for which all the data regarding education and income were available for all the waves. Because of a lack of consistent, detailed occupational information and thus the inability to construct the SBTC measure, we exclude Norway and Italy from the last part of the analysis. Consequently, the overall sample consisted of 100 country-cohort data points in 10 countries.

Variables

Returns to education

We use two different measures of the economic returns to education. The first is the household disposable income (PPP adjusted) equivalised per standard adult (DPI).¹ To obtain our second measure, we transformed the DPI into logitranks (Chauvel 2016; Rotman et al. 2016; Tam 2013) (Eq. 1) for each cohort (c) in each time period (p) to create income-based ranks that are indifferent to both cohort and period. In other words, educational returns are calculated at different stages of one’s life, but individuals of one cohort are only compared to those of other cohorts in the same age group. This standardization strategy enabled us to more accurately compare the economic returns over time and across countries. Because of the limited representativeness of survey data in the tails of the distribution, we trimmed the data to the range between −4 (pertains to approximately the bottom 2% of the income distribution) and 4 (the top 2%). A logitrank of zero refers to the median income.

$$ logit{\left({r}_i\right)}_{c,p}=\ln \left(\frac{r_i}{1-{r}_i}\right)c,p $$

(1)

r_i ∈ ]0, 1[ - rank order of income quantiles

The returns to education are not bound to individual labor income alone. We adopt here a wider view, taking into account that labor market decisions are made jointly in the household, and that there are tradeoffs between household members that individual earnings are not able to reflect. In addition, inequalities in educational returns may be magnified in households. Studies of assortative mating repeatedly found homogamy in terms of education (Mare 1991; Schwartz and Mare 2017). In other words, having tertiary education increases the likelihood of marriage to a spouse with tertiary education. At least in recent decades, this seems to have important economic consequences: As the male breadwinner model declines (Blossfeld and Buchholz 2009), marital educational homogamy is assumed to increase the household income of the tertiary education owners and thus widen inequality in educational returns between the low- and highly educated. Empirical studies, however, found little evidence of the effect of marital homogamy on overall income inequality (Breen and Salazar 2009, 2011; Breen and Andersen 2012). The reason put forward is that the increased employment gap between individuals with tertiary and non-tertiary education accounted for most of the inequality rather than the gap based on marriage composition (Breen and Salazar 2009). Nevertheless, the household income gap between tertiary- and non-tertiary-educated persons is higher than the gap in individual income from labor, implying that the returns to education are also evident in household income (Yang 2004).

Moreover, the tendency to exchange tertiary education with other forms of capital in the marriage market (i.e. tertiary-educated as documented by Schwartz et al. 2016) suggests that individual income in fact underestimates the actual economic returns to education. People use their tertiary education to marry partners with less education, but with higher social origin. Hence, the gains from tertiary education are arguably broader than mere individual earnings suggest.

One must note, however, that the household approach might overestimate the disadvantage of singles in the labor market. This is especially true for women, who are generally more educated than men but earn less income (Kim and Sakamoto 2015; Bar-Haim et al. 2018). In this study, we control for gender in order to minimize this bias.² Moreover, using a composite measure makes it more difficult to disentangle the causes. Yet, our aim here is less to focus on the precise underlying process rather but rather to estimate the total amount of inequality between the groups.

Education

We dichotomized the highest level of education achieved, as calculated by the LIS, into tertiary vs. non-tertiary. Tertiary education pertains to ISCED level 5 or 6. Non-tertiary education pertains to ISCED levels from 0 to 4.

Skill-biased technological change (SBTC)

In order to measure this variable at the cohort level, we first identified high-skill occupations based on the 1-digit ISCO88 classification provided by LIS. Following the results of Goos et al. (2014), we considered all professional (category 2) and technical (category 3) occupations as high-skill occupations. The proportion of people in high-skill occupations serves as an indicator for SBTC.³

Method

We develop two new features of age-period-cohort (APC) models in order to observe cohort level changes in the returns to education, and in the factors that are driving the changes. We start by shortly reviewing traditional APC models: The purpose of APC models is to explain a dependent variable, y, observed in a series of cross-sectional surveys assembled in a Lexis table (Age x Period) through its decomposition in effects of age a (α_a), period p (π_p) and cohort membership c (γ_c), as can be seen in Eq. 2.

$$ {y}^{apc}=\mu +{\alpha}_a+{\pi}_p+{\gamma}_c(APC) $$

(2)

In an APC model, the dependent variable is affected by the age of the respondent, the period in which he or she lives and the generation, or birth cohort to which he or she belongs to. However, since cohort is a linear combination of age and period c = p − a, the basic model suffers from an identification problem, which has been extensively discussed and reviewed (Mason and Wolfinger 2001).

In the recent decade, several solutions have been suggested to solve the problem of the identification, mainly by imposing constraints on the APC model (Smith 2008; Chauvel and Schroeder 2015). To avoid arbitrary choices on constraints, it is important to clearly specify their meaning. One solution is to build an age-period-cohort detrended (APCD) model where age, period and cohort vectors are constrained to be sum zero and linear trend zero, so that we estimate and test the difference to zero of the deviation from the trends (“bumps”) to be attributed to age period or cohort effect (Chauvel 2011, Kuang et al. 2008, Freedman 2017). Equation 3 presents the formalization of the model.

$$ \Big\{{\displaystyle \begin{array}{c}{y}^{apc}={\alpha}_a+{\pi}_p+{\gamma}_c+{\alpha}_0 rescale(a)+{\gamma}_0 rescale(c)+{\beta}_0+{\varepsilon}_i\\ {}\Big\{\begin{array}{c}\sum {\alpha}_a=\sum {\pi}_p=\sum {\gamma}_c=0\\ {} Slop{e}_a\left({\alpha}_a\right)= Slop{e}_p\left({\pi}_p\right)= Slop{e}_c\left({\gamma}_c\right)=0\\ {}\min (c)<c<\max (c)\end{array}\end{array}} $$

(3)

β₀ denotes the constant, α_a is the age effect vector, π_p is the period effect vector, and γ_c is the cohort effect vector. The constraints set the sum and the slope of each of these vectors to zero. The linear trends in age and cohort (and also period) are absorbed by Rescale(a) and Rescale(c) that are transformations from the initial values of a and c into a range between −1 and + 1. Lastly, the oldest and youngest cohorts (which only appear once in the Lexis table) need to be omitted from the analysis.

The APCD model detects deviations from the linear trends of age, period and cohort: it is able to identify specific cohorts defined by higher or lower suicide rates, for instance (Chauvel et al. 2016), but it cannot identify the actual linear trends.

A way to solve the indetermination on the linear trends in APC is to have a substantial definition of one of these trends. Since we are most interested in identifying cohort trends, we constrain the age linear trend to equate to the average within-cohort age effect across the cohorts in the observation window. Further, we impose a slope zero on period but no longer need a constraint on the cohort vector to make the model identifiable. In this age-period-cohort-trended lag (APCTLAG) model (Chauvel et al. 2017), the cohort vector will absorb the general linear trend of social change and make relative changes in economic returns to education visible. The model is formalized in Eq. 4:

$$ \Big\{{\displaystyle \begin{array}{c}{y}^{apc}={\alpha}_a+{\pi}_p+{\gamma}_c++{\varepsilon}_i\\ {}\Big\{\begin{array}{c}\sum {\alpha}_a=\sum {\pi}_p=0\\ {} Slope\left({\pi}_p\right)=0\\ {} Slope\left({\alpha}_a\right)=\frac{\sum \left({y}_{\mathrm{a}+1,\mathrm{p}+1,\mathrm{c}}-{y}_{\mathrm{a},\mathrm{p},\mathrm{c}}\right)}{\left(\mathrm{p}-1\right)\left(\mathrm{a}-1\right)}\ \\ {}\min (c)<c<\max (c)\end{array}\end{array}} $$

(4)

The constraints mean that the sum of the age and period vectors is zero and the period linear trend is zero. In the APCTLAG, compared to the APCD, the cohort coefficients will include the constant and the linear trend, on top of the non-linear deviation patterns.

The constraint on age linear trend requires that we have at least three consecutive cohorts, as reflected in Eq. 4. However, for the first and the last cohorts, we have an incomplete set of age groups. Since the estimation is based on linear prediction of the average age effect, the results might be biased due to extrapolation of the age effect for these cohorts. In the appendix, we provide two robustness checks: estimation for a narrower age span and simulation with limited data.

The APCTLAG model (Chauvel et al. 2017) implemented on the log equivalised disposable income (ln(DPI)) and the logitranks of it (logitranks(DPI)) for the sample restricted to tertiary education holders only estimates the cohort variations of absolute returns to tertiary education. The estimation of the relative cohort returns of tertiary diploma holders relative to the non-tertiary-educated population, we first calculate the differences in the average ln(DPI) and logitranks(DPI) between tertiary and non-tertiary education holders on the Lexis table. We then estimate the cohort effect using the APCTLAG for the differences as the dependent variable.

The last part of the analysis is a linear regression in which the cases are country-cohort data points to predict the returns to education, aiming at assessing the extent to which educational expansion, SBTC, and their interaction impact returns to education. Our assumption underlying this is that there is an interaction between expansion and SBTC, i.e. the expansion of tertiary education affects its returns depending on the extent to which the market is able to absorb the newly educated, i.e. the extent to which there is SBTC. Both expansion and SBTC enter the equation as independent variables in a linear model. The model is described in Eq. 5:

$$ Y={\beta}_0+{\beta}_1 Expansion+{\beta}_2 SBTC+{\beta}_3 Expansion\ast SBTC $$

(5)

where Y are the returns to education at the cohort by country level, based on the cohort coefficients obtained from the previous models. The same method of acquiring the data points was used for expansion and SBTC, which are the independent variables in the model.

Results

Educational expansion

Figure 1 presents changes in the proportion of tertiary education holders over cohorts. In all countries, tertiary education massively expanded, from 10% to about 50%, across the cohorts born between 1935 and 1980. An exception to this general trend, however, is Italy, which experienced only minor expansion during this time. In a number of countries—most notably France, Luxembourg, Finland, Israel, the United Kingdom and the Netherlands—the expansion was not linear, with a substantial shift in the proportion of cohorts with tertiary education born in 1970–1975. This is in line with previous studies highlighting a massive educational expansion that occurred during the 1990s, when the aforementioned cohorts entered the higher educational system (Marginson 2016; Liu et al. 2016) and can be attributed to some extent to the above-mentioned changes in the higher educational systems, at least for the UK, the Netherlands, France and Finland.

Fig. 1

Cohort change in the proportion of tertiary education holders. Source: LIS. Note: The Y-axis represent the percentage of tertiary education within a birth cohort

In the United States and Denmark, the educational expansion began earlier, with cohorts born in 1960–1965 being its first beneficiaries. In Italy, a similar pattern can be observed, but, as noted, its expansion was considerably smaller than in all other countries. None of the other countries experienced a shift in the trend of educational expansion—the increase in tertiary education remained relatively stable over time.

The gap between tertiary and non-tertiary education

Figure 2 depicts the APC-GO results, i.e. the returns to tertiary education relative to those with less education in terms of equivalized disposable household income. Except for Spain, which has experienced a massive decline in returns to education, our results do not support the hypothesis of an overall “overeducation” process: The overall trend in returns to tertiary education can be described as stable or slightly increasing. However, there is also no indication of a major upgrade due to SBTC in the returns to education.

Fig. 2

The gap between persons with tertiary and non-tertiary education across birth cohorts in terms of household disposable income per standard adult (DPI). Source: LIS. Note: The Y-axis represents the average gap in natural log DPI between persons with tertiary education and those with less than tertiary education in each birth cohort

As a sensitivity analysis, Fig. 3 depicts the same analysis, showing the gap within each cohort relative to the income distribution in each period, but now using standardized logitranks of income instead of the PPP adjustment. Reflecting the hierarchical position, the ranks provide a different strategy of dealing with the challenge of comparing economic returns over time periods. Both measures yield similar results, confirming the robustness of the finding that the gap between the individuals with tertiary and non-tertiary education changed very little in most of the countries examined here.

Fig. 3

The gap between tertiary- and non-tertiary-educated persons across birth cohorts in terms of logitranks of DPI. Source: LIS. Note: The Y-axis represents the average gap in logitranks of DPI between persons with tertiary education and those with less than tertiary education in each birth cohort

Returns to tertiary education

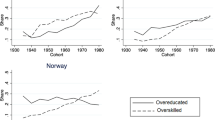
Figure 4 presents the cohort change in the logitranks of DPI for tertiary education holders. In Germany, Denmark, the Netherlands and Italy, absolute returns to education did not decline across cohorts. In these countries, the returns have remained similar or—as in Italy and Germany—even increased, albeit not significantly.

Fig. 4

Returns to tertiary education (logitrank DPI). Source: LIS. Note: The Y-axis represents the average logitranks of DPI for tertiary-educated by cohort

In the other countries, however, returns to education have decreased significantly—people with a post-secondary education who were born earlier had higher incomes than their later counterparts. Indeed, for these countries, education is less sufficient for maintaining the status quo regarding labor market outcomes.

Educational expansion and returns to education

In order to test whether returns to education changed as a result of educational expansion and SBTC, we employed linear regression analyses with country by cohort as units of analysis. As SBTC is not available for Italy and Norway, and due to the instability of Israel and Italy in the robustness check (Appendix), we restrict the analysis to nine countries in this part. Figure 5 plots the levels of SBTC against educational expansion in each country-cohort context. Not surprisingly, both educational expansion and SBTC increased between the cohorts of 1930–1940 and 1970–1980. However, for some countries (e.g. the UK in the 1980s), the educational expansion increased more rapidly than the SBTC while in other countries, such as Germany and Luxembourg, the SBTC increased more rapidly than the educational expansion. In countries experiencing stronger SBTC than expansion, there is a higher demand than supply for skilled workers, which thus should lead to increased returns to education, while in those countries experiencing stronger educational expansion than SBTC, we would expect the contrary.

Fig. 5

Educational expansion (supply) and SBTC (demand) among different cohorts in 10 countries. Note: The Y-axis represents the percentages of people with skilled and technical occupations within each cohort. The X-axis represent the percentages of people with tertiary education within each cohort

The results are presented in Table 1. Model 1 describes the effects of expansion and SBTC on the gap in returns between tertiary and non-tertiary education holders or on relative returns to education. There is a significant—yet rather small—negative effect of educational expansion. Educational expansion thus slightly decreases the gap between tertiary and non-tertiary education holders while controlling for SBTC. The effect of SBTC is not significant.

Table 1 OLS estimates of economic returns to education (logitrank DPI)

In Model 2, we added an interaction term between educational expansion and SBTC. Again, the effect of expansion is negative and significant while the effect of SBTC remains insignificant. In line with our hypotheses, the interaction effect is positive and significant, implying that when there is a substantial skill-biased technological change, educational expansion can increase the gap between tertiary and non-tertiary education holders.

The results presented in Model 3 and Model 4 reflect absolute returns to tertiary education, regardless of non-tertiary education. Here, the effect of expansion is also negative and significant, and the interaction term is again positive and significant. As can be inferred from the adjusted R² of the models, the explanatory power of the model is above 30%. In sum, independent of the measure of educational returns, first, educational expansion is associated with decreasing returns to tertiary education. However, second, the effect of educational expansion is reversed in the presence of SBTC, i.e. when the labor market provides new job opportunities. Figures 6 presents the predicted values of Model 2 and Model 4 in Table 1 for plausible values of SBTC and educational expansion. We predict the returns for the highest, lowest and average levels of SBTC and educational expansion in our sample. As can be seen from Fig. 6, which depicts the returns of tertiary education relative to less than tertiary education, the negative effect of educational expansion is significant only when SBTC does not occur. With SBTC present, the negative effect of expansion diminishes to insignificance.

Fig. 6

Predicted economic returns to education by expansion and SBTC

Figure 6 presents the predicted returns to education in comparison to older cohorts. Here, there is also a negative effect of expansion at an average level of SBTC. Only with a high level of SBTC does the effect of expansion become insignificant. This confirms H₂: In the presence of SBTC, the negative effect of educational expansion diminishes.

Discussion

Since the late 1960s, scholars have warned of overeducation as a result of massive educational expansion. However, while there is strong evidence of declining returns to tertiary education in relation to occupational standing (Di Stasio et al. 2016; Sarkar 2016), results that actually confirm the phenomenon of “overeducation” in terms of economic returns to tertiary education are scarce (for an exception see Psacharopoulos 1989). On the contrary, most studies found an increase in economic returns after the educational expansion (Montenegro and Patrinos 2014).

As educational attainment is an intrinsic cohort phenomenon, a cohort design is the most appropriate tool to analyze educational returns in our eyes. Whereas many previous studies only used a two-time-point design, we employ a new approach to study economic returns to education with an advanced age-period-cohort method. This gives us a wider perspective and enables us to observe the changes for a much longer period.

Observing the changes in relative returns to education across cohorts born between 1940 and 1980, our findings confirm the “more necessary and less sufficient” hypothesis: In most of the countries studied, economic returns to education increased for individuals with higher education compared to those with lower education. Furthermore, people with a tertiary educational level born in younger cohorts perform much better than their less-educated counterparts, in comparison with older cohorts. In other words, for younger cohorts, education is crucial even more so than before.

However, absolute returns to education decreased steadily for most of the researched countries. In those countries, post-secondary education holders from younger cohorts are doing much worse than their older counterparts. Consequently, for younger generations, tertiary education is not sufficient to maintain the same standard of living as older generations.

Investigating the cohort effects of returns to education in each country on educational expansion, we found that educational expansion is associated with decreasing returns to tertiary education. We could also show that the effect of educational expansion is reversed in the presence of skill-biased technological change. In other words, when the labor market is able to create jobs for the newly educated, the income of tertiary education holders will not decline. It is thus indeed the particular combination of expanding labor markets together with increasing educational opportunities provide the most positive context for economic returns.

It should be noted, however, the findings presented here are an accurate description of the association between educational expansion and returns to education only for the set of countries and cohorts under investigation. Because of data limitations, we were not able to compare a larger set of countries for the entire time span, limiting us to a single-level analysis instead of a multilevel model that would have allowed us to differentiate between country and cohort effects. Moreover, due to the complexity of a cohort-based analysis, we could not control for other structural factors considered important for the analysis of educational returns, such as income inequality and growth.

Nevertheless, our study advances the current debate in a number of ways. Our results shed light on reasons for the inconsistency in results between returns to education, in terms of occupational standing, and economic returns to education presented in previous studies (Bernardi and Ballarino 2016). Economic returns to education are not actually increasing because a tertiary-level education pays better, but because of the fact that a lower education, in particularly completed secondary education, pays worse than before. Therefore, the loss of occupational standing due to overeducation is less visible in terms of income. The decline of returns across cohorts is likely due to the lower occupational positions in younger cohorts.

In conclusion, the educational expansion of the late twentieth century generally was a mixed blessing regarding the labor market and economic returns of affected cohorts. Tertiary education holders from younger cohorts struggle to find suitable labor market positions, and therefore find themselves competing with less educated individuals for jobs for which they are overqualified. As a result, those with a higher educational level experience a decrease in income in comparison with their counterparts from older cohorts. At the same time, increased competition for low-skill jobs is crowding out applicants with lower educational levels, thus further increasing the gap between tertiary and non-tertiary education holders. In many countries, having a higher educational level is more valuable in terms of payout than a lower educational level, but its value has decreased compared to payouts in the past. With education being “more necessary and less sufficient,” the results seem to imply, similar to Collins’s (1979) prediction, a stronger competition within the elite of the higher education holders on a small fraction of lucrative jobs, while the lower-educated are competing for getting a job, no matter which one. This scenario is direful for the sustainability of the higher educational system as well as for the entire society.

Appendix: Robustness checks

The results from the first and the last cohort are based on prediction (extrapolation) from the average effect of age on income differences between the types of education. As such, they are unbiased only if the assumption that the effect of age, net of period effect, is similar across cohorts. To test the robustness of the results, we provide two additional analyses. The first one is identical to the analysis presented in paper, but restricting the age span to 35–45 years old. The results are presented in Fig. 7, jointly with a the results taken from the main analysis (Fig. 3). The results are very similar to one another.

Fig. 7

The gap between tertiary- and non-tertiary-educated persons across birth cohorts: age 25–55 years (dotted line) and age 35–45 years (solid line). Source: LIS. Note: The Y-axis represents the average gap in logitranks of DPI between persons with tertiary education and those with less than tertiary education in each birth cohort

In the second robustness check, we restricted our analysis excluding the first and the last cohorts. In other words, now we extrapolate the results for those cohorts for whom we have complete results. Comparing the complete with the restricted results, we show that the differences with the extrapolated results (Fig. 8) are minor with two exceptions—Israel and Italy. Yet, only in Israel were the differences significant. In Israel, the linear trend appears to be less steep than in the full data model. This might be due to the late labor market entry of younger cohorts (born after 1950), for whom a 3 years military service in Israel is mandatory.

Fig. 8

The gap between tertiary- and non-tertiary-educated across birth cohorts: complete data (dotted line) and without oldest and youngest cohorts (solid line). Source: LIS. Note: The Y-axis represents the average gap in logitranks of DPI between persons with tertiary education and those with less than tertiary education in each birth cohort