Constructing wormhole solutions of attractive geometries in the framework of f(R) gravity

Abstract

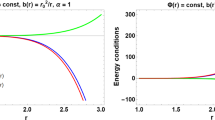
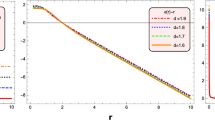
In this work, we show that any viable f(R) gravity model with constant scalar curvature could be implemented to construct wormholes that are supported by ordinary matter. In particular, the constructed wormholes give rise to attractive geometries at least in specific regions, if the ratio between the Lagrangian density function f(R) and its derivative $F=\frac{df(R)}{dR}$ satisfies certain constraints. In this context, we derive static, spherically symmetric and traversable wormhole solutions supported by anisotropic matter field where both the weak and the strong energy conditions could be satisfied. The obtained solutions are physically realistic as they respect the asymptotic flatness condition. The case of traceless energy-momentum tensor is further investigated where it is shown that if the Ricci scalar is constant, then the only admitted f(R) gravity model is the one involving a square Lagrangian, i.e $f(R)=cR^2$. For this model we derived the constraints that allow the corresponding wormhole to satisfy the energy conditions.

Appendices

Appendices

Consider the following curvature invariants:

$$\begin{aligned} R&=g^{ij}R_{ij}~~~~~~~~\\ R_1&=\frac{1}{4}S_a^bS_b^a~~~~~\\ R_2&=-\frac{1}{8}S_a^bS_b^aS_b^c \end{aligned}$$

where R is the Ricci scalar, $R_1$ and $R_2$ are respectively the first and the second Ricci invariants and $S_{ij}$ is the trace free Ricci tensor given by $S_{ij}=R_{ij}-\frac{R}{4}g_{ij}$. The value of the above invariants for the general static wormhole metric (1) are given below

$$\begin{aligned} R&=2\left( 1-\frac{b}{r}\right) (\Psi ''+\Psi '^2)+\left( \frac{4}{r}-\frac{3b}{r^2}-\frac{b'}{r}\right) \Psi '-\frac{2b'}{r^2}\\ R_1&=-\frac{1}{16r^6}(r^2(b'^2(r^2\psi '^2+2)-4rb'\psi '(r^2\psi ''+r^2\psi '^2-2)\\&\quad +4r^2(r^2\psi ''^2+r^2\psi '^4+2\psi '^2(r^2\psi ''+1)))\\&\quad +2rb(b'(-2r^3\psi '^3+\psi '(6r-2r^3\psi '')+r^2\psi '^2+2)\\&\quad +2r(2r^3\psi '^4+2\psi '^2(2r^3\psi ''+r)+2r\psi ''(r^2\psi ''-1)\\&\quad -r^2\psi '^3+\psi '(2-r^2\psi ''))) -b^2(4r^4\psi ''^2+4r^4\psi '^4 \\&\quad -4r^3\psi '^3-8r^2\psi ''-4r\psi '(r^2\psi ''-3)+\psi '^2(8r^4\psi ''+r^2)+6)). \\ R_2&=\frac{3}{64r^9}(b(2r\psi '+1)-r(b+2r\psi '))^2(r^2(b'\psi '\\&\quad -2r(\psi ''+\psi '^2))+b((2r^2\psi ''+2r^2\psi '^2-r\psi '-2)) \end{aligned}$$

Ricci invariants of the first solution (20)

Recall that $b(r)=r_0$ and $\psi (r)=\psi _0$ so the invariants are given by

$$\begin{aligned} R&=0\\ R_1&=0\\ R_2&=\frac{3r_0^3}{P32r^9}(1-r)^2 \end{aligned}$$

Ricci invariants of the second solution (27)

In this case, $b(r)=\frac{r_0^2}{2r_0+1}(2+\frac{1}{r})$ and $\psi (r)=\ln {\frac{r+1}{r}}$ so the invariants are given by

$$\begin{aligned} R&=0\\ R_1&=-\frac{1}{16r^6}\left( r^2\left( \frac{r_0^4}{(2r_0+1)^2r^4}\left( \frac{1}{(r+1)^2}+2\right) -\frac{4r_0^2}{(2r_0+1)(r^3+r^2)}\left( \frac{2r}{(r+1)^2}-2\right) \right. \right. \\&\left. \quad + 4r^2\left( \frac{2r+1}{(r+1)^2}+\frac{1}{r^2(r+1)^2}+\frac{2}{r^2(r+1)^2}\left( \frac{2r+1}{(r+1)^2}+1\right) \right) \right) \\&\quad + 2rc_1\left( 2+\frac{1}{r}\right) \left( \frac{-r_0^2}{(2r_0+1)r^2}\left( \frac{2}{(r+1)^3}-\frac{1}{r(r+1)}\left( 6r-\frac{2r^2+r}{(r+1)^2}\right) +\frac{1}{(r+1)^2}+2\right) \right. \\&\left. \left. \quad + \frac{1}{r(r+1)^3}-\frac{1}{r(r+1)}\left( 2-\frac{2r+1}{(r+1)^2}\right) \right) \right) -4c_1^2\left( 2+\frac{1}{r}\right) ^2\frac{(2r+1)^3+1}{(r+1)^4}\\&\quad + \frac{4}{(r+1)^3}-\frac{8(2r+1)}{(r+1)^2}+\frac{4(2r+1)}{(r+1)^3}-\frac{12}{r+1}+\frac{8(2r+1)}{(r+1)^4}+\frac{6}{r^2(r+1)^2}\\ R_2&=\frac{-3}{64r^9}\left( \left( 2c_1+\frac{c_1}{r}\right) \left( 1-\frac{2}{r+1}\right) -2c_1r-c_1+\frac{2r}{r+1}\right) ^2\left( \frac{r_0^2}{r(2r_0+1)(r+1)}-\frac{4r}{r+1}\right) \\&\quad + \left( 2c_1+\frac{c_1}{r}\right) \left( \frac{5}{r+1}-2\right) \end{aligned}$$

Ricci invariants of the third solution (37)

In this case, $b(r)=\frac{r+10r^2+39r^3+71r^4+51.5r^5+(r_0^{-2}+9r_0^{-1}+32r_0+28.5)r^5e^{2/r-2/r_0}}{(1+2r)^5}$ and $\psi (r)=-\frac{1}{r}$ so the invariants are given by

$$\begin{aligned} R&=0\\ R_1&=-\frac{1}{16r^6}\\&\quad \left( (1+2r^2)\left( \frac{r+10r^2+39r^3+71r^4+51.5r^5+(r_0^{-2}+9r_0^{-1}+32r_0+28.5)r^5e^{2/r-2/r_0}}{(1+2r)^5}\right) '^2~~~\right. \\&\qquad +(8-\frac{4}{r}+8r)\left( \frac{r+10r^2+39r^3+71r^4+51.5r^5+(r_0^{-2}+9r_0^{-1}+32r_0+28.5)r^5e^{2/r-2/r_0}}{(1+2r)^5}\right) '\\&\left. \qquad +16+\frac{4}{r^2}+8\left( 1-\frac{2}{r}\right) \right) \\&\qquad +2r\left( \frac{r+10r^2+39r^3+71r^4+51.5r^5+(r_0^{-2}+9r_0^{-1}+32r_0+28.5)r^5e^{2/r-2/r_0}}{(1+2r)^5}\right) \\&\qquad \left( \left( \frac{r+10r^2+39r^3+71r^4+51.5r^5+(r_0^{-2}+9r_0^{-1}+32r_0+28.5)r^5e^{2/r-2/r_0}}{(1+2r)^5}\right) '\right. \\&\quad \left( \frac{6}{r}+\frac{5}{r^2}-\frac{2}{r^3}+2\right) \left. \left. +2r\left( \frac{2}{r^5}-\frac{8}{r^4}+\frac{10}{r^3}+\frac{4}{r^2}\right) -\frac{1}{r^4}+\frac{2}{r^2}+\frac{2}{r^3}\right) \right) \\&\qquad -\left( \frac{r+10r^2+39r^3+71r^4+51.5r^5+(r_0^{-2}+9r_0^{-1}+32r_0+28.5)r^5e^{2/r-2/r_0}}{(1+2r)^5}\right) ^2\left( \frac{28}{r}+\frac{25}{r^2}-\frac{20}{r^3}+10\right) \\ R_2&=-\frac{3}{64r^9}\left( \left( \frac{2}{r}-r+1\right) \left( \frac{r+10r^2+39r^3+71r^4+51.5r^5+(r_0^{-2}+9r_0^{-1}+32r_0+28.5)r^5e^{2/r-2/r_0}}{(1+2r)^5}\right) -2\right) ^2\\&\qquad \times \left( \left( \frac{r+10r^2+39r^3+71r^4+51.5r^5+(r_0^{-2}+9r_0^{-1}+32r_0+28.5)r^5e^{2/r-2/r_0}}{(1+2r)^5}\right) '+4-\frac{2}{r}\right. \\&\left. \qquad + \left( \frac{2}{r^2}-\frac{5}{r}-2\right) \left( \frac{r+10r^2+39r^3+71r^4+51.5r^5+(r_0^{-2}+9r_0^{-1}+32r_0+28.5)r^5e^{2/r-2/r_0}}{(1+2r)^5}\right) \right) \end{aligned}$$