Study on early inflationary phase using a new form of non-canonical scalar field model

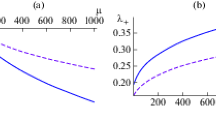
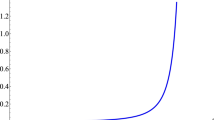
Abstract

We study the inflationary phase of the early universe as modeled by a non-canonical scalar field. The homogeneous scalar field equation is derived from a Lagrangian density containing a new form of non-canonical kinetic term and a general potential function. The Lie symmetry is studied and a one parameter Lie point symmetry for the homogeneous scalar field equation is found. We use Lie symmetry generator to construct the exact analytical group invariant closed-form solution of the homogeneous scalar field equation without applying any slow-roll approximation from invariant curve condition. The solution thus obtained is seen to be consistent with the Friedmann equations subject to constraint conditions on the potential parameter $\lambda$. In this scenario, we obtain the values for various inflationary parameters and make useful checks on the observational constraints on the parameters from Planck data by imposing a set of bounds on the parameter $\lambda$ of the potential. The results for scalar spectral index ($n_S$) and tensor-to-scalar ratio (r) presented in the $(n_S,\,r)$ plane in the background of Planck2015 and Planck2018 data are in good agreement with cosmological observations. We find $r\sim 10^{-3}$, the targeted value of r that will be detected by the future CMB observation such as LiteBIRD. Interestingly, most significant primordial non-Gaussianity is also achieved. For theoretical completeness of our non-canonical model, we obtain the allowed parameter space in which the ghosts and Laplacian instabilities are absent. We apply the formulas for slow-roll parameter to explain exit from the inflationary phase using the general potential. We also treat the non-canonical scalar field model equation by the dynamical system theory to provide useful checks on the stability of the critical points and show that the group invariant non-canonical inflationary solution is stable attractor in the phase space.

Appendix A: Lie point symmetry and Group invariant solution

The so-called Lie symmetry formulation has its origin in the classic work of Sophus Lie, who pioneered this modern approach for studying and finding special solutions of systems of differential equations [35]. In the nineteenth century Norwegian mathematician Sophus Lie began to investigate the continuous groups of transformations leaving differential equations invariant. The original Lie’s aim was that of setting a general theory for the integration of ordinary differential equations similar to that developed by E. Galois for algebraic equations [59]. In Lie group approach, consider the infinitesimal transformations for independent variables

$$\begin{aligned} {x^{i'}} = {x^i} + \delta {x^i},\,\,\,\,\,\delta {x^i} = \epsilon {\xi ^i}(x,f) \end{aligned}$$

(A-1)

and for dependent variable

$$\begin{aligned} f' = f + \delta f,\,\,\,\,\,\,\delta f = \epsilon \eta (x,f) \end{aligned}$$

(A-2)

for a field variable $f=f(x^i)$ with $\epsilon$, an arbitrary small quantity. Here the functions $\xi (x,f)$ and $\eta (x,f)$ are not infinitesimals. These are the coefficient functions of a finite differential operator which, when coupled with infinitesimal parameter $\epsilon$, gives the generator of the infinitesimal transformation. For an arbitrary analytic function $g=g(x^i,f)$, it is straightforward to show that

$$\begin{aligned} \delta g = \epsilon Xg \end{aligned}$$

(A-3)

with

$$\begin{aligned} X = \xi ^i \frac{\partial }{{\partial {x^i}}} + \eta \frac{\partial }{{\partial f}}, \end{aligned}$$

(A-4)

the generator of the infinitesimal transformations in (A-1) and (A-2). A similar consideration when applied to $h=h(x^i,f,f_i)$ with $f_i=\frac{\partial f}{\partial x^i}$ gives

$$\begin{aligned} \delta h = \epsilon {X^{(1)}}h \end{aligned}$$

(A-5)

with

$$\begin{aligned} {X ^{(1)}} = X + \left( {{\eta _i} - \xi _i^j{f_j}} \right) \frac{\partial }{{\partial {f_i}}}. \end{aligned}$$

(A-6)

Understandably, $X^{(1)}$ stands for the first-order prolongation of X to the extended space of the variables $(x^i,f,f_i,f_{ii})$. Similarly we can find

$$\begin{aligned} {X ^{(2)}} = {\xi ^i}\frac{\partial }{{\partial {x^i}}} + \eta \frac{\partial }{{\partial f}} + {\eta ^{(1)}}\frac{\partial }{{\partial {f_i}}} + {\eta ^{(2)}}\frac{\partial }{{\partial {f_{ii}}}}, \end{aligned}$$

(A-7)

the second-order prolongation of the vector field X to the extended space of the variables $(x^i,f,f_i,f_{ii})$ with

$$\begin{aligned} {\eta ^{(k)}}\left( {x,f,{f_i},\,\, \ldots {f^{(k)}}} \right) = \frac{{D{\eta ^{(k - 1)}}}}{{Dx}} - {f^{(k)}}\frac{{D\xi }}{{Dx}},\,\,\,\,k = 1,2,3 \ldots \end{aligned}$$

(A-8)

Here ${\eta ^{(0)}} = \eta ({x^i},f),\,\,{f^{(k)}} = \frac{{{d^k}f}}{{d{x^k}}}$ and $\frac{D}{Dx}$ is the total derivative (Lie Derivatives) defined as [35]

$$\begin{aligned} \frac{D}{{Dx}} = \frac{\partial }{{\partial x}} + {f_i}\frac{\partial }{{\partial f}} + {f_{ii}}\frac{\partial }{{\partial {f_i}}} + \ldots . \end{aligned}$$

(A-9)

Similarly, we can find higher-order prolongation. Here number superscripted in (A-7) denotes the order of prolongation of the generator X. For Lie symmetry analysis for the partial differential equations we have to consider more than one independent variables and at least one dependent variable and detailed of this study with example are given in many monographies and textbooks [59] and references therein.

Throughout this paper we will deal mainly with Lie groups of transformations admitted by ordinary differential equations with infinitesimals depending on the one independent and one dependent variable only. For ordinary differential equation the method introduced by Lie (1891) considers the invariance of the form of the differential equation itself under an infinitesimal transformation of the independent variable $(x^i=t)$ and the dependent variable $(f(x^i)=x(t))$ given by

$$\begin{aligned} \delta t = \epsilon \xi (t,x)\,\,\,\,\,{\text {and}}\,\,\,\,\,\delta x = \epsilon \eta (t,x) \end{aligned}$$

(A-10)

generated by Lie point symmetry generator. Here generator (A-4) takes the form

$$\begin{aligned} X = \xi (t,x)\frac{\partial }{{\partial t}} + \eta (t,x)\frac{\partial }{{\partial x}}. \end{aligned}$$

(A-11)

A second-order ordinary differential equation of the form ${\mathcal {M}}\left( {t,x,\dot{x},\ddot{x}} \right) =0$ possesses Lie point symmetry generator of the form given by (A-11) provided

$$\begin{aligned} {X^{(2)}}{\mathcal {M}}\left( {t,x,\dot{x},\ddot{x}} \right) = 0. \end{aligned}$$

(A-12)

A dot means differentiation with respect to t. Here in the extended space of variables $\left( {t,x,\dot{x},\ddot{x}} \right)$, $X^{(2)}$ in (A-7) the second-order prolongation of the generator X (A-11), takes the form

$$\begin{aligned} {X^{(2)}} = X + \left[ {\dot{\eta }(t,x) - \dot{\xi }(t,x)\dot{x}} \right] \frac{\partial }{{\partial \dot{x}}} + {\eta ^{(2)}}\frac{\partial }{{\partial \ddot{x}}}. \end{aligned}$$

(A-13)

In writing (A-13) we have used

$$\begin{aligned} {\eta ^{(2)}} = \left[ {\ddot{\eta }(t,x) - 2\dot{\xi }(t,x)\ddot{x} - \ddot{\xi }(t,x)\dot{x}} \right] . \end{aligned}$$

(A-14)

Eq. (A-12) provides a natural basis to carry out Lie symmetry analysis for finding the symmetry for second-order ordinary differential equations, whereas invariant curve condition in (A-15) can be used to find the group invariant solutions generated by the symmetry generators in (A-11). To find the group invariant solution generated by a particular generator X, we follow the approach. Any curve C, is an invariant curve if and only if the tangent to C at each point (t, x) is parallel to the tangent vector $(\xi (t,x),\eta (t,x))$. This condition can be expressed mathematically by introducing the characteristic $Q=\eta (t,x)-\dot{x}\xi (t,x)$ [60]. Every curve C on the (t, x) plane that is invariant under the group generated by X satisfies

$$\begin{aligned} Q\equiv \eta (t,x)-\dot{x}\xi (t,x)=0 \end{aligned}$$

(A-15)

on C. From this invariant curve condition we can find out the solution for a differential equation from symmetry. Recently, there has been increasing interest in studying the symmetries of differential equations modelling physical systems. It has been applied, in recent years, to several equations of motion for dynamical systems.