Cosmological evolution of the gravitational entropy of the large-scale structure

Abstract

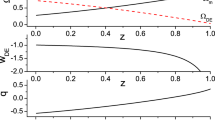
We consider the entropy associated with the large-scale structure of the Universe in the linear regime, where the Universe can be described by a perturbed Friedmann–Lemaître spacetime. In particular, we compare two different definitions proposed in the literature for the entropy using a spatial averaging prescription. For one definition, the entropy of the large-scale structure for a given comoving volume always grows with time, both for a CDM and a $\Lambda$CDM model. In particular, while it diverges for a CDM model, it saturates to a constant value in the presence of a cosmological constant. The use of a light-cone averaging prescription in the context of the evaluation of the entropy is also discussed.

Appendices

Appendix 1: Dynamics of the background spacetime

We assume that the background spacetime is well-described by a spatially Euclidean Friedmann–Lemaître universe with the late time dynamics dictated only by pressureless matter and cosmological constant with density parameters

$$\begin{aligned} \Omega _\mathrm{m0} = \frac{8\pi G\rho _\mathrm{m0}}{3H_0},\qquad \Omega _{\Lambda 0} = \frac{\Lambda }{3H_0} \end{aligned}$$

(38)

that satisfy $\Omega _\mathrm{m0}+ \Omega _{\Lambda 0}=1$. The Friedmann equation then takes the usual form

$$\begin{aligned} \frac{H^2(z)}{H_0^2} = \Omega _\mathrm{m0}(1+z)^3 + \Omega _{\Lambda 0}\,, \end{aligned}$$

(39)

and, using the proper time t, its solution is given by

$$\begin{aligned} a(t) \propto \sinh ^{2/3}\left( \frac{3}{2}\sqrt{\Omega _{\Lambda 0}} H_0t \right) . \end{aligned}$$

(40)

The normalization to the Hubble constant today, $H_0$, implies that

$$\begin{aligned} \sinh \left( \frac{3}{2}\sqrt{\Omega _{\Lambda 0}} H_0t_0 \right) = \frac{\Omega _{\Lambda 0}^{1/2}}{(1-\Omega _{\Lambda 0})^{1/2}}\equiv \kappa _0^{3/2} \end{aligned}$$

(41)

so that the redshift is given by

$$\begin{aligned} 1+z =\frac{\kappa _0}{\sinh ^{2/3}\left( \frac{3}{2}\sqrt{\Omega _{\Lambda 0}} H_0t \right) }. \end{aligned}$$

(42)

Appendix 2: Linear perturbation theory

The evolution of the degrees of freedom of the metric (13) can be found in many textbooks, e.g. Ref. [1].

It can first be shown that there is only six gauge invariant degrees of freedom usually defined as

$$\begin{aligned} \Psi\equiv & {} \psi +\frac{1}{2} \Delta E+\frac{\mathcal {H}}{2}(\beta +E'), \end{aligned}$$

(43)

$$\begin{aligned} \Phi\equiv & {} \alpha -\frac{\mathcal {H}}{2}(\beta +E')-\frac{1}{2}(\beta +E')', \end{aligned}$$

(44)

$$\begin{aligned} \bar{\Phi }^i\equiv & {} \frac{1}{2}\bar{\chi }^{i'}+\frac{1}{2}\bar{B}^{i},\,\,\,\,\,\,\, \bar{h}^{i j} , \end{aligned}$$

(45)

where the prime denotes the derivative with respect to conformal time and ${\mathcal {H}}=a'/a$.

Considering a matter sector described by a perfect fluid with stress-energy tensor

$$\begin{aligned} T_{\mu \nu }=(\rho +P)u_\mu u_\nu +P g_{\mu \nu }, \end{aligned}$$

(46)

where the density and pressure can be split as $\rho (\eta , {\varvec{x}})=\rho ^{(0)}(\eta )+\rho ^{(1)} (\eta , {\varvec{x}})$ and $P(\eta , {\varvec{x}})=P^{(0)}(\eta )+P^{(1)} (\eta , {\varvec{x}})$, and the velocity of the comoving observers is decomposed as $u^\mu =\bar{u}^\mu +\delta u^\mu$ with $u_\mu u^\mu =-1$. It follows that

$$\begin{aligned} u^\mu =a^{-1}(1-\alpha , v^i),\,\,\,u_\mu =a (-1-\alpha , v_i-1/2 B_i)\, \end{aligned}$$

(47)

and we decompose $v_i$ into scalar and a vector component according to

$$\begin{aligned} v_i=\partial _i v+\bar{v}_i\,. \end{aligned}$$

(48)

The scalar shear, given in Eq. (10), is by construction a second order quantity so that

$$\begin{aligned} (\sigma ^2)^{(0)}= (\sigma ^2)^{(1)}=0. \end{aligned}$$

(49)

Thus, at the lowest order and for a general metric, we have

$$\begin{aligned} (\sigma ^2)^{(2)}= & {} \frac{1}{2 a^2 {S'}^{2}}\left[ \delta S_{,i j} \delta S^{,i j}-\frac{1}{3}(\nabla ^2 \delta S)^2 \right] \nonumber \\&+\frac{1}{8 a^2}\left[ B_{i, j} B^{i, j}-\frac{1}{3}(\partial ^i B_i)^2 \right] \nonumber \\&-\frac{1}{2 a^2 S'}\left[ \delta S_{,i j} B^{i, j}-\frac{1}{3}(\nabla ^2 \delta S) (\partial ^i B_i) \right] \nonumber \\&-\frac{1}{a^2 {S'}} \delta S_{,i j} {\tilde{h}}^{',i j}+\frac{1}{2 a^2} B_{i, j} {\tilde{h}}^{',i j} \nonumber \\&+\frac{1}{2 a^2} {\tilde{h}}_{',i j} {\tilde{h}}^{',i j}\,, \end{aligned}$$

(50)

where $\tilde{h}_{,i j}=\frac{1}{2}D_{ij} E+\partial _{(i} \bar{\chi }_{j)}+\frac{1}{4}\bar{h}_{i j}$, we use the notation $X_{,i}\equiv \partial _i X$ for any field X, and $\delta S$ is the first order perturbation of the scalar $S(\mathbf{x}, t)$ defining the space-time foliation.

It is clear that first order perturbation theory is sufficient to obtain the general expression for the shear up to second order, since second order perturbations will contribute only to third or fourth order to $\sigma ^2$.