Generating anisotropic collapse and expansion solutions of Einstein’s equations

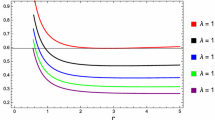
Abstract

Analytic gravitational collapse and expansion solutions with anisotropic pressure are generated. Metric functions are found by requiring zero heat flow scalar. It emerges that a single function generates the anisotropic solutions. Each generating function contains an arbitrary function of time which can be chosen to fit various astrophysical time profiles. Two examples are provided: a bounded collapse metric and an expanding cosmological solution.

Appendix: Energy-momentum and physical components

Metric

$$\begin{aligned} g_{\mu \nu }^{\text {aniso}}dx^{\mu }dx^{\nu }=A^{2}dt^{2}-B^{2}dr^{2}-R^{2}d\Omega ^{2} \end{aligned}$$

is Petrov type D. The two principal null vectors, normal to ($\vartheta ,\varphi$) two-surfaces, are

$$\begin{aligned} l^{\mu }\partial _{\mu }&= A^{-1}\partial _{t}+B^{-1}\partial _{r}\end{aligned}$$

(46)

$$\begin{aligned} n^{\mu }\partial _{\mu }&= A^{-1}\partial _{t}-B^{-1}\partial _{r}. \end{aligned}$$

(47)

The energy-momentum tensor is given by ($G=c=1$)

$$\begin{aligned} T^{\mu \nu }=w\hat{u}^{\mu }\hat{u}^{\nu }+p_{r}\hat{r}^{\mu }\hat{r}^{\nu }+p_{\perp }(\hat{\vartheta }^{\mu }\hat{\vartheta }^{\nu }+\hat{\varphi }^{\mu }\hat{\varphi }^{\nu })+q^{\mu }\hat{u}^{\nu }+\hat{u}^{\mu }q^{\nu } \end{aligned}$$

(48)

where $p_{r}$ is the radial pressure, $p_{\perp }$ is the tangential pressure, $w$ is the mass-energy density, and $q^{\mu }$ is the radial heat flow vector orthogonal to $\hat{u}^{\mu }$. We use notation of Taub [21] for $w$. Taub’s purpose was to distinguish mass-energy density from proper mass-density $\rho$, with $w=\rho (1+\epsilon )$. This allows the first law of thermodynamics to be written in its usual form $Tds=d\epsilon +pd(1/\rho )$ with specific entropy $s$ and specific internal energy $\epsilon$. The kinematics of the fluid are described by

$$\begin{aligned} \hat{u}_{\mu ;\nu }&= a_{\mu }\hat{u}_{\nu }+\sigma _{\mu \nu }-(\Theta /3)(\hat{r}_{\mu }\hat{r}_{\nu }+\hat{\vartheta }_{\mu }\hat{\vartheta }_{\nu }+\hat{\varphi }_{\mu }\hat{\varphi }_{\nu }),\end{aligned}$$

(49a)

$$\begin{aligned} a_{\mu }dx^{\mu }&= -(A^{\prime }/A)dr,\end{aligned}$$

(49b)

$$\begin{aligned} \Theta&= A^{-1}(\dot{B}/B+2\dot{R}/R),\end{aligned}$$

(49c)

$$\begin{aligned} \sigma _{\mu \nu }&= \sigma [-2\hat{r}_{\mu }\hat{r}_{\nu }+\hat{\vartheta }_{\mu }\hat{\vartheta }_{\nu }+\hat{\varphi }_{\mu }\hat{\varphi }_{\nu }],\,\,\sigma =A^{-1}(\dot{R}/R-\dot{B}/B) \end{aligned}$$

(49d)

where primes denote $\partial /\partial r$, and overdots denote $\partial /\partial t$. The rate-of shear $\sigma _{\mu \nu }$ is trace-free, and $\sigma _{\mu \nu }\sigma ^{\mu \nu }=6\sigma ^{2}$. The heat flow vector ($q^{\mu }\hat{u}_{\mu }=0$) is given by

$$\begin{aligned} 4\pi q^{\mu }\partial _{\mu }&= Q\partial _{r}\end{aligned}$$

(50)

$$\begin{aligned} Q&= (AB)^{-1}\left[ \frac{A^{\prime }}{A}\frac{\dot{R}}{R}+\frac{\dot{B}}{B}\frac{R^{\prime }}{R}-\frac{\dot{R}^{\prime }}{R}\right] \end{aligned}$$

(51)

The sectional curvature mass is

$$\begin{aligned} 2m=R^{3}R_{\alpha \beta \mu \nu }\hat{\vartheta }^{\alpha }\hat{\varphi }^{\beta }\hat{\vartheta }^{\mu }\hat{\varphi }^{\nu }=R[1+\dot{R}^{2}/A^{2}-(R^{\prime })^{2}/B^{2}]. \end{aligned}$$

(52)

The mass-energy density and pressures are given, respectively, by

$$\begin{aligned} 8\pi w&= \frac{1}{A^{2}}\left[ \left( \frac{\dot{R}}{R}\right) ^{2}+2\frac{\dot{R}}{R}\frac{\dot{B}}{B}\right] -\frac{1}{B^{2}}\left[ 2\frac{R^{\prime \prime }}{R}+\left( \frac{R^{\prime }}{R}\right) ^{2}-2\frac{B^{\prime }}{B}\frac{R^{\prime }}{R}\right] +\frac{1}{R^{2}} \qquad \end{aligned}$$

(53)

$$\begin{aligned} 8\pi p_{r}&= \frac{1}{B^{2}}\left[ \left( \frac{R^{\prime }}{R}\right) ^{2}+2\frac{R^{\prime }}{R}\frac{A^{\prime }}{A}\right] -\frac{1}{A^{2}}\left[ 2\frac{\ddot{R}}{R}+\left( \frac{\dot{R}}{R}\right) ^{2}-2\frac{\dot{R}}{R}\frac{\dot{A}}{A}\right] -\frac{1}{R^{2}} \end{aligned}$$

(54)

$$\begin{aligned} 8\pi p_{\perp }&= -\frac{1}{A^{2}}\left[ \frac{\ddot{R}}{R}+\frac{\ddot{B}}{B}+\frac{\dot{R}}{R}\left( \frac{\dot{B}}{B}-\frac{\dot{A}}{A}\right) +\frac{\dot{A}}{A}\frac{\dot{B}}{B}\right] +\frac{1}{B^{2}}\left[ \frac{R^{\prime \prime }}{R}+\frac{A^{\prime \prime }}{A}\right. \nonumber \\&\left. +\frac{R^{\prime }}{R}\left( \frac{A^{\prime }}{A}-\frac{B^{\prime }}{B}\right) -\frac{A^{\prime }}{A}\frac{B^{\prime }}{B}\right] \end{aligned}$$

(55)

1.1 Trapped surfaces

The topological two-spheres ($\vartheta ,\varphi$) nested in an $R=const$ surface at time $t$ have outgoing null geodesic normal $l^{\mu }$ and incoming null geodesic normal $n^{\mu }$. The two principal null vectors (46) and (47) provide trapping scalars

$$\begin{aligned} \kappa _{1}=l^{\mu }\partial _{\mu }(\ln R),\quad \kappa _{2}=n^{\mu } \partial _{\mu }(\ln R). \end{aligned}$$

When scalars [23] $\kappa _{1}$ and $\kappa _{2}$ have the same sign, a trapped surface [24] will exist:

$$\begin{aligned} \kappa _{1}&= A^{-1}(\dot{R}/R)+B^{-1}(R^{\prime }/R)\end{aligned}$$

(56)

$$\begin{aligned} \kappa _{2}&= A^{-1}(\dot{R}/R)-B^{-1}(R^{\prime }/R). \end{aligned}$$

(57)