Abstract
Torsion-sensitive intersection homology was introduced to unify several versions of Poincaré duality for stratified spaces into a single theorem. This unified duality theorem holds with ground coefficients in an arbitrary PID and with no local cohomology conditions on the underlying space. In this paper we consider for torsion-sensitive intersection homology analogues of another important property of classical intersection homology: topological invariance. In other words, we consider to what extent the defining sheaf complexes of the theory are independent (up to quasi-isomorphism) of choice of stratification. In addition to providing torsion sensitive versions of the existing invariance theorems for classical intersection homology, our techniques provide some new results even in the classical setting.
Similar content being viewed by others
Data availability
Data sharing not applicable to this article as no datasets were generated or analyzed during the current study.
Notes
Even though \(\mathcal {E}\) is a complex of sheaves, we do not write \(\mathcal {E}^*\) in order to emphasize the role of \(\mathcal {E}\) as coefficients.
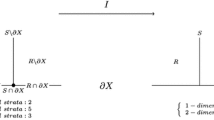
This is no longer true if the local system is only defined on a dense open set whose complement has codimension 1. For example let \(X=S^1\) with stratification \(S^1\supset \{pt\}\). Suppose E is the constant sheaf with stalk \(\mathbb {Z}\) on \(S^1-\{pt\}\). Then there are two non-isomorphic extensions of E to \(S^1\), namely the constant sheaf with stalk \(\mathbb {Z}\) and the twisted sheaf with stalks \(\mathbb {Z}\) such that a generator of \(\pi _1(S^1)\) acts by multiplication by \(-1\).
We see in this argument why \(\vec {p}_1(S)\ge -1\) is required, as well as our torsion assumptions; see also Sect. 4.2, below.
We see in this argument why \(\vec {p}_1(S)\le \text {codim}(S)-1\) is required, as well as our torsion-free assumptions; see also Sect. 4.2, below.
These \(\tilde{S}\) are called source strata in [5]. Note that the precise choice of \(\tilde{S}\) does not matter in the conditions that follow as the assumption that \(\vec {p} \) can be pushed forward assures us that any two choices would give the same perversity conditions.
In fact, since \(H^1(\mathcal {E}_x)\) is torsion, we can treat it as a module over \(R/\text {Ann}(H^1(\mathcal {E}_x))\). If \(H^1(\mathcal {E}_x)\ne 0\) then Ann\((H^1(\mathcal {E}_x))\ne 0\), and this is an Artinian ring since R is a PID. If \(H^1(\mathcal {E}_x)=0\), it is clearly Artinian.
We remark that if \(\vec {p}_2(k)=\emptyset \) for all k, which corresponds to \(\mathscr {S}^*\) satisfying the original Goresky–MacPherson axioms, then \(\vec {q}_2(k)=P(R)\) for all k. In this case \(q^{-1}(n-j+1,\mathfrak p)=q^{-1}(n-j,0)\) by Remark 5.3, which is again consistent with the expectation from the classical case.
A version of such a construction for fairly general sheaf complexes can be found in Habegger–Saper [16, Section 3].
References
Beĭlinson, A.A., Bernstein, J., Deligne, P.: Faisceaux pervers. In: Analysis and Topology on Singular Spaces, I (Luminy, 1981), Astérisque, vol. 100, Soc. Math. France, Paris, pp. 5–171 (1982)
Borel, A., et al.: Intersection cohomology. In: Progress in Mathematics, vol. 50, Birkhäuser Boston, Inc., Boston, MA (1984). Notes on the seminar held at the University of Bern, Bern (1983), Swiss Seminars
Bredon, G.E.: Sheaf theory. In: Graduate Texts in Mathematics, vol. 170, 2nd edn. Springer, New York (1997)
Cappell, S.E., Shaneson, J.L.: Singular spaces, characteristic classes, and intersection homology. Ann. Math. (2) 134(2), 325–374 (1991)
Chataur, D., Saralegi-Aranguren, M., Tanré, D.: Intersection homology: general perversities and topological invariance. Ill. J. Math. 63(1), 127–163 (2019)
Friedman, G.: Superperverse intersection cohomology: stratification (in)dependence. Math. Z. 252(1), 49–70 (2006)
Friedman, G.: Singular chain intersection homology for traditional and super-perversities. Trans. Am. Math. Soc. 359(5), 1977–2019 (2007)
Friedman, G.: Intersection homology with general perversities. Geom. Dedic. 148, 103–135 (2010)
Friedman, G.: An introduction to intersection homology with general perversity functions. In: Topology of Stratified Spaces, Mathematical Sciences Research Institute Publications, vol. 58, pp. 177–222. Cambridge University Press, Cambridge (2011)
Friedman, G.: Generalizations of intersection homology and perverse sheaves with duality over the integers. Mich. Math. J. 68(4), 675–726 (2019)
Friedman, G.: Singular intersection homology. In: New Mathematical Monographs, vol. 33. Cambridge University Press (2020)
Friedman, G.: Two short proofs of the topological invariance of intersection homology. J. Singul. 25, 144–149 (2022)
Goresky, M., MacPherson, R.: Intersection homology theory. Topology 19(2), 135–162 (1980)
Goresky, M., MacPherson, R.: Intersection homology. II. Invent. Math. 72(1), 77–129 (1983)
Goresky, M., Siegel, P.: Linking pairings on singular spaces. Comment. Math. Helv. 58(1), 96–110 (1983)
Habegger, N., Saper, L.: Intersection cohomology of cs-spaces and Zeeman’s filtration. Invent. Math. 105(2), 247–272 (1991)
Kashiwara, M., Schapira, P.: Sheaves on manifolds. In: Grundlehren der Mathematischen Wissenschaften [Fundamental Principles of Mathematical Sciences], vol. 292. Springer, Berlin (1994)
King, H.C.: Topological invariance of intersection homology without sheaves. Topol. Appl. 20(2), 149–160 (1985)
Ribenboim, P.: Rings and modules. In: Interscience Tracts in Pure and Applied Mathematics, No. 24, Interscience Publishers John Wiley & Sons Inc., New York (1969)
Saralegi-Aranguren, M.: de Rham intersection cohomology for general perversities. Ill. J. Math. 49(3), 737–758 (2005)
Schürmann, J.: Topology of singular spaces and constructible sheaves. In: Instytut Matematyczny Polskiej Akademii Nauk. Monografie Matematyczne (New Series) [Mathematics Institute of the Polish Academy of Sciences. Mathematical Monographs (New Series)], vol. 63, Birkhäuser Verlag, Basel (2003)
Siebenmann, L.C.: Deformation of homeomorphisms on stratified sets. I. Comment. Math. Helv. 47, 123–136 (1972)
Siebenmann, L.C.: Deformation of homeomorphisms on stratified sets. II. ibid 47, 137–163 (1972)
Valette, G.: A Lefschetz duality for intersection homology. Geom. Dedic. 169, 283–299 (2014)
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
This work was partially supported by a Grant from the Simons Foundation (#839707 to Greg Friedman).
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Friedman, G. Topological invariance of torsion-sensitive intersection homology. Geom Dedicata 217, 105 (2023). https://doi.org/10.1007/s10711-023-00843-6
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s10711-023-00843-6