Temperature dependence of mode I fracture behaviour of a textured magnesium alloy

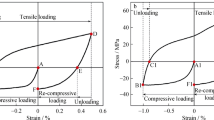
Abstract

Mode I, static fracture experiments and uniaxial tension/compression tests are conducted at three temperatures in the range from 25 to $100\,^\circ$C using fatigue pre-cracked four-point bend and micro-tensile/compression specimens machined from a rolled AZ31 Mg alloy plate. Digital image correlation technique along with in-situ optical imaging is employed to analyse the specimen surface deformation. It is found that the fracture mechanism which is operative near the tip changes from quasi-brittle cracking caused by tensile twins to ductile void growth and coalescence as temperature is raised above $65\,^\circ$C. This corroborates with reduction in tensile twin development near the crack tip with enhancement in temperature. On the other hand, at higher temperature, more profuse twinning and pronounced texture changes are perceived in the far-edge of the ligament, where compressive normal stress prevails. Simplified analyses are performed to show that the evolution rate of tensile twin volume fraction with energy release rate J near the tip will diminish strongly, while micro-void growth rate will enhance between 25 and $100\,^\circ$C, thereby triggering the observed brittle-ductile transition. The fracture toughness rises dramatically above $65\,^\circ$C and is also accompanied by significant notch blunting. This is rationalized from the transition in fracture mechanism and large plastic dissipation in the ligament far-edge due to twinning.

Appendices

Appendix A: Approximate analysis of evolution of TT volume fraction near the crack tip

In this Appendix, an approximate analysis of TT volume fraction near the tip is conducted based on structure of elastic–plastic crack tip fields. To this end, the uniaxial tension stress–strain curves along RD given in Fig. 3 are first represented in power law form as:

$$\begin{aligned} \frac{\epsilon }{\epsilon _{0t}} = \left( \frac{\sigma }{\sigma _{0t}}\right) ^n , \end{aligned}$$

(A.1)

where $\sigma _{0t}$ is the initial yield stress, $\epsilon _{0t} = \sigma _{0t}/E$, the initial yield strain and n is a hardening exponent. A value of $n = 9$ gives a reasonable approximation to these stress–strain curves. Within the framework of a small strain, deformation plasticity theory, the near-tip stress distribution can be described by a HRR-type field (Hutchinson 1968; Rice and Rosengren 1968; Pan and Shih 1986; Saeedvafa and Rice 1989; Symington et al. 1988), as:

$$\begin{aligned} \frac{\sigma _{ij}}{\sigma _{0t}} = \left( \frac{J^{nt}}{\sigma _{0t} \epsilon _{0t}I_nr}\right) ^{1/(n+1)}\tilde{\sigma }_{ij}(\theta ,n,p_{\alpha }) \,\, . \end{aligned}$$

(A.2)

Here (r, $\theta$) are crack tip polar coordinates, $J^{nt}$ is the J integral evaluated on a vanishing contour near the crack tip¹ (Rice 1968), $\tilde{\sigma }_{ij}$ are non-dimensional functions of $\theta$, n, and suitably defined plastic anisotropy parameters $p_{\alpha }$ (see, for example, Pan and Shih (1986) for orthotropic materials). Also, $I_n$ is a constant dependent on n. It must be emphasized here that no assumptions about isotropy need to be made in applying the above universal HRR-structure for the near tip fields in power law hardening solids. Indeed, such asymptotic solutions for orthotropic plastic solids and ductile single crystals with Taylor power law hardening have been derived by Pan and Shih (1986) and Saeedvafa and Rice (1989), respectively. While the effect of crack blunting on the near-tip fields is currently ignored, it will be considered subsequently.

The following phenomenological evolution law is assumed for twin volume fraction $f_{tt}$ pertaining to the polycrystalline alloy:

$$\begin{aligned} f_{tt} = A\sum _{m=1}^{N_g}\left( \frac{\tau _{RSS}^{(m)}}{\tau _0}\right) ^{n} f_g^{(m)},\quad \text {for} f_{tt} \le 1. \end{aligned}$$

(A.3)

In the above equation, $N_g$ is the number of grains considered in the underlying micro-structure at a material point, $\tau _{RSS}^{(m)}$ is the maximum resolved shear stress amongst the six TT variants (Kaushik 2013) and $f_g^{(m)}$, the volume fraction of the $m^{th}$ grain. Also, a small value of 0.01 is assumed for the constant A, while the reference shear stress $\tau _0$ is taken as 38 MPa (Vaishakh et al. 2020; Wang et al. 2020) and to be independent of temperature. It must be noted that Eq. (A.3) essentially represents a weighted average of twin evolution in individual grains that follow a power law behavior. Also, it must be mentioned here that a twin hardening model of the power-law type is just a phenomenological idealization (akin to saturation hardening), which is chosen here to be consistent with the assumed form for the uniaxial stress versus strain curve (Eq. (A.1)) that would enable applying the HRR-structure of the near-tip fields.

On using Eq. (A.2), $\tau _{RSS}^{(m)}$ near the tip can be written as,

$$\begin{aligned} \tau _{RSS}^{(m)} = \sigma _{0t}\left( \frac{J^{nt}}{\sigma _{0t} \epsilon _{0t}I_nr}\right) ^{1/(n+1)}\tilde{\tau }_{RSS}^{(m)} (\theta ,n,p_{\alpha }), \end{aligned}$$

(A.4)

where,

$$\begin{aligned} \tilde{\tau }_{RSS}^{(m)} = S_{ij}^{(m)} \tilde{\sigma }_{ij}(\theta ,n,p_{\alpha }). \end{aligned}$$

(A.5)

Here $S_{ij}^{(m)}$ are the Schmid tensor components pertaining to the TT variant in the $m^{th}$ grain which gives the highest value of resolved shear stress. On substituting Eq. (A.4) in Eq. (A.3), $f_{tt}$ near the tip can be written as :

$$\begin{aligned} f_{tt}&= A\left( \frac{\sigma _{0t}}{\tau _0}\right) ^n \left( \frac{EJ^{nt}}{\sigma _{0t}^2I_nr}\right) ^{n/(n+1)} \sum _{m=1}^{N_g}f_g^{(m)}\left( \tilde{\tau }_{RSS}^{(m)}\right) ^{n},\nonumber \\&\qquad \text {for} f_{tt} \le 1. \end{aligned}$$

(A.6)

The above evolution equation for $f_{tt}$ ahead of the tip can be expressed as:

$$\begin{aligned} f_{tt}|_{\theta =0}&= B\left( \frac{\sigma _{0t}}{\tau _0}\right) ^n \left( \frac{E}{\sigma _{0t}}\right) ^{n/(n+1)}\left( \frac{J^{nt}}{\sigma _{0t}r}\right) ^{n/(n+1)},\nonumber \\&\qquad \text {for} \,\,\, f_{tt} \le 1 , \end{aligned}$$

(A.7)

where,

$$\begin{aligned} B = \frac{A}{I_n^{n/(n+1)}}\sum _{m=1}^{N_g}f_g^{(m)} \left( \tilde{\tau }_{RSS}^{(m)}(\theta = 0)\right) ^{n}. \end{aligned}$$

(A.8)

To simplify the computation, only four grain orientations are considered. These orientations and corresponding $S_{ij}$ values are given in the Supplementary material. In the first and third orientations, the (0001) axis is taken parallel to $X_3$, whereas it is tilted by $15^\circ$ with respect to $X_3$ towards $X_2$ in the second and fourth orientations. In view of the near basal texture of this alloy, $f_g^{(1)} = f_g^{(3)} = 0.3$ and $f_g^{(2)} = f_g^{(4)} = 0.2$ is assumed. Further, since an analytical solution for the crack tip fields of the form given by Eq. (A.2) is not available for this material, $\tilde{\sigma }_{ij}$ and $I_n$ pertaining to $J_2$ deformation theory of plasticity are employed as a first level approximation. These values corresponding to plane strain condition and $n=10$ along with $\theta =0^\circ$ (ahead of the tip) are: $\tilde{\sigma }_{11} = 1.73$, $\tilde{\sigma }_{22} = 2.5$, $\tilde{\sigma }_{33} = 2.115$ and $I_n = 4.54$ (Symington et al. 1988). The above assumption can be partly justified by referring to the recent CPFE study of plane strain notch tip fields under small scale yielding (SSY) by Vaishakh and Narasimhan (2022) which has shown that for the present TD-RD orientation, prismatic slip is preponderant all around the crack tip followed by basal slip. The near-tip stress and plastic strain distributions as well as plastic zone shape and size are similar to those obtained from isotropic von Mises model (maximum difference being about $20\%$). The variations of $f_{tt}$ with respect to $J^{nt}$ based on Eq. (A.7) at $r = 0.1$ mm ahead of the tip are plotted in Fig. 16.

An estimate of $f_{tt}$ ahead of the crack tip following substantial blunting can also be obtained by employing the Rice and Johnson (1970) plane strain, logarithmic spiral slip line field (again based on the Von Mises theory). The stresses given by this solution are:

$$\begin{aligned}&\sigma _{11} = \frac{2\sigma _t}{\sqrt{3}}ln\left( 1+\frac{X_1}{\rho }\right) \nonumber \\&\sigma _{22} = \frac{2\sigma _t}{\sqrt{3}}\left( 1+ln\left( 1+\frac{X_1}{\rho }\right) \right) \nonumber \\&\sigma _{33} = \frac{\sigma _t}{\sqrt{3}}\left( 1+2ln\left( 1+\frac{X_1}{\rho }\right) \right) . \end{aligned}$$

(A.9)

In order to account for strain hardening, the value of $\sigma _t$ in the above equation is taken to be the average of the initial yield strength and UTS under RD tension, which is 189 MPa and 162.5 MPa at RT and $100\,^\circ$C, respectively, for the Mg alloy (see Sect. 3.1). Also, $\rho$ is the current radius of the blunted crack tip which is taken to be $\delta _t/2$, where $\delta _t \sim J^{nt}/(2\sigma _t)$ is the crack tip opening displacement (Shih 1981).

On again evaluating $\tau _{RSS}$, pertaining to the four chosen grain orientations using the above stress field (see Supplementary material) and substituting in Eq. (A.3), estimates of average TT area fraction just ahead of the blunted crack tip are obtained as 0.17 and 0.044 pertaining to RT and $100\,^\circ$C, respectively.

The above approximate analysis of $f_{tt}$ ahead of the tip is based on plane strain crack tip fields which are expected to apply on the specimen mid-plane. By contrast, the present results show that the TT area fraction on the surface is higher by a factor of 2 to 3 at RT and 1.6 at $100\,^\circ$C in comparison to the respective values on the mid-plane (see Table 1). This trend is counter to that expected on the basis that drop in stress triaxiality should inhibit twinning (Selvarajou et al. 2017). In order to understand this intriguing behaviour, it should first be noted that the stress state just ahead of the blunted crack tip would be given by $\sigma _{11}=0 , \sigma _{22}=\sigma _{t}$ and $\sigma _{33}=0$ on the specimen surface. For this stress state, resolved shear stress $\tau _{RSS}$ pertaining to TTs for grain orientations such as the first and third selected above, in which c-axis is close to ND, would be negative (see Supplementary material), inhibiting nucleation of TTs (due to their polar nature (Christian and Mahajan 1995)). Also, the $\tau _{RSS}$ for basal slip will be zero in these grains.

However, it was shown by Vaishakh et al. (2019) that under uniaxial tension, pronounced basal slip can occur in grains where c-axis is tilted towards the loading direction (i.e., RD or $X_2$-axis). For example, if the above tilt is $15^\circ$ (such as grain orientations 2 and 4), the maximum resolved shear stress $\tau _{RSS}$ for basal slip is around 0.22 to $0.25\sigma _{t}$, while that for TT is still negative or a small positive value (see Supplementary material). Indeed, the ratio of $\tau _{RSS}^{max}$ to CRSS for basal slip in this case would be 3 to 3.5 times that of prismatic slip (Vaishakh et al. 2019). The strong basal slip in such grains can promote pile-up of basal dislocations at their boundaries. The CPFE calculations of Vaishakh et al. (2019) show that due to this pile-up, intense stress concentration in the adjacent grains at the intervening boundaries is created with local stress $\sigma _{22}\sim 2\sigma _{t}$ and $\sigma _{11}\sim 0.75\sigma _{t}$. By contrast, the soft basal slip mode drastically reduces the local stress within the grains with the c-axis tilt resulting in $\sigma _{22}\sim 0$ and $\sigma _{11}\sim -0.75\sigma _{t}$ (compressive). These values would enable maintaining a macroscopically uniaxial stress state along $X_2$-direction. The above compressive $\sigma _{11}$ in turn, gives rise to a $\tau _{RSS}$ for tensile twinning of about 0.281 to $0.375\sigma _{t}$ (see Supplementary material). On substituting these $\tau _{RSS}$ values in Eq. (A.3), values of $f_{tt}$ just ahead of the blunted tip are estimated as 0.59 and 0.15, at RT and $100\,^\circ$C, respectively.

Appendix B: Approximate analysis of evolution of void volume fraction near the crack tip

Fig. 19

Schematic showing an initially spherical void of radius $R_0$ ahead of the tip that has grown due to the blunting crack tip fields

A simplified analysis is performed in this Appendix by combining the Rice and Johnson (1970) blunting crack tip fields (Eq. A.9) along with the Rice and Tracey (1969) equations for growth rate of a spherical void of initial radius $R_0$ located at a distance r ahead of the tip in the undeformed configuration (see Fig. 19). Again, since such analytical equations have not been developed for the present anisotropic alloy, above theories which pertain to isotropic plastic solid obeying the Von Mises yield condition are employed to gain an understanding of changing the temperature from RT to $100\,^\circ$C on near-tip void growth with respect to J.

As mentioned earlier, the above assumption can be partly justified on the basis of the recent plane strain, SSY, CPFE simulations of Vaishakh and Narasimhan (2022) for the TD-RD notch orientation. Further, numerical simulations of cylindrical void growth ahead of a notch tip in Mg single crystals wherein c-axis is parallel to the notch front (with prismatic slip being dominant and tensile twinning being negligible), has demonstrated that the behaviour is similar to isotropic solids (Prasad et al. 2016, 2017). In particular, the voids are observed to become moderately oblate and crack extension occurs by void-by-void growth mechanism as predicted by Tvergaard and Hutchinson (2002) for an isotropic plastic solid obeying the von Mises yield condition. Further support for this assumption is provided by the mode I fracture experiments at RT using notched compact tension specimens of an AZ31 Mg alloy conducted by Prasad et al. (2015) wherein dimple fracture was observed. They noted that the behavior pertaining to TD-RD and RD-TD notch orientations are exactly identical resulting in the same $J_c$ value. Therefore, it is reasonable to employ the Rice and Tracey (1969) equations to describe near-tip void growth for the present crack orientation wherein prismatic slip is dominant and tensile twinning is mild (note from Table 1 that TT area fraction near the tip in the specimen mid-plane at crack initiation is only about 0.06).

From Eq. (A.9), the hydrostatic stress experienced by the void can be written as:

$$\begin{aligned} \sigma _H = \frac{\sigma _t}{\sqrt{3}} \left( 1+2ln\left( 1+\frac{4\sigma _{t}r}{J^{nt}}\right) \right) . \end{aligned}$$

(B.1)

Thomason (1990) had approximately integrated the Rice and Tracey (1969) void growth rate equations assuming $\sigma _H$ to remain constant. From his results, under plane strain conditions, the mean radius of the void can be expressed as a function of the equivalent plastic strain $\overline{\epsilon }$ as:

$$\begin{aligned} \overline{R} = R_0\,exp\left( D\overline{\epsilon }\right) . \end{aligned}$$

(B.2)

In the above equation, the parameter D is dependent on the hydrostatic stress by

$$\begin{aligned} D = 0.558\,sinh\left( \frac{3\sigma _H}{2\sigma _t}\right) , \end{aligned}$$

(B.3)

for the non-hardening case.

In order to apply Eq. (B.2) in the present context, the equivalent plastic strain $\overline{\epsilon }$ as a function of normalized distance $(r/\delta _t)$ ahead of the tip in the undeformed configuration given by Rice and Johnson (1970) is employed. Noting again that $\delta _t\simeq J^{nt}/(2\sigma _t)$, the above variation can be well represented by the following empirical relation:

$$\begin{aligned} \overline{\epsilon } \simeq 3\,exp(-4.7\hat{r}), \end{aligned}$$

(B.4)

where,

$$\begin{aligned} \hat{r} = \frac{r}{(J^{nt}/\sigma _t)}. \end{aligned}$$

As before, the value of $\sigma _t$ is taken to be the mean of initial yield strength and UTS under RD tension. Further, a value of r = 0.325 mm is chosen which would correspond to $\hat{r} \sim 0.5$ for $100\,^\circ$C and 0.6 for RT at $J^{nt} = 100$ N/mm. From Eq. (B.4), and using plastic incompressibility along with plane strain conditions, the location of the void with respect to the tip in the deformed configuration at the above stage is deduced as 0.15mm.

The average value of $\sigma _H$ over the range of $\hat{r}$ from 1 (edge of the blunting zone) to 0.5 for $100\,^\circ$C and to 0.6 for RT is obtained from Eq. (B.1) as $2.18\sigma _t$ and $2.24\sigma _t$, respectively. The corresponding values of D determined from Eq. (B.3) are 7.3 and 8, respectively. Finally, substituting Eqs. (B.4) into (B.2) and using the above value of D, the mean radius of the void is given as a function of $J^{nt}$ by:

$$\begin{aligned} \overline{R} \simeq R_0 exp\left( C_1exp \left( -\frac{4.7r\sigma _t}{J^{nt}}\right) \right) , \end{aligned}$$

(B.5)

where, $C_1 = 3D = 21.9$ for $100\,^\circ$C and 24 for RT. Assuming a distribution of such initially spherical voids located ahead of the tip whose spacing is small compared to r, the current void volume fraction can be written in terms of the initial value, $f_{0v}$, and $J^{nt}$ as:

$$\begin{aligned} f_v \simeq f_{0v}exp\left( C_2 exp\left( -\frac{4.7r\sigma _t}{J^{nt}}\right) \right) , \end{aligned}$$

(B.6)

where, $C_2 = 66$ for $100\,^\circ$C and 72 for RT. The variations of $f_v/f_{0v}$ at $r = 0.325$ mm ahead of the tip with respect to $J^{nt}$ are plotted in Fig. 17.