Skip to main content
SpringerLink
Log in

Simulating Nelsonian Quantum Field Theory

  • Published:
Foundations of Physics Aims and scope Submit manuscript

Abstract

We describe the picture of physical processes suggested by Edward Nelson’s stochastic mechanics when generalized to quantum field theory regularized on a lattice, after an introductory review of his theory applied to the hydrogen atom. By performing numerical simulations of the relevant stochastic processes, we observe that Nelson’s theory provides a means of generating typical field configurations for any given quantum state. In particular, an intuitive picture is given of the field “beable”—to use a phrase of John Stewart Bell—corresponding to the Fock vacuum, and an explanation is suggested for how particle-like features can be exhibited by excited states. We then argue that the picture looks qualitatively similar when generalized to interacting scalar field theory. Lastly, we compare the Nelsonian framework to various other proposed ontologies for QFT, and remark upon their relative merits in light of the effective field theory paradigm. Links to animations of the corresponding beables are provided throughout.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Data availability statement

No datasets were generated or analysed during the current study.

Notes

  1. Bell [1] suggested the particular term “beable” for such things (rather than, e.g., “being” or “beer”) to emphasize “the essentially tentative nature of any physical theory,” a sentiment we want to reiterate in this work. “In fact, ‘beable’ is short for ‘maybe-able’.”

  2. A few caveats: In [4], this problem is avoided by remarking that such eigenstate distributions, in realistic scenarios, arise from complex interactions, during which the wave function is not so simple. Second, once spin is included, the electron is no longer motionless in such states [5, 6].

  3. Not everybody regards this as unappealing; see [7] for various perspectives on the status of the wave function. See also [8, 9].

  4. Whether Nelson succeeded in deriving QM in its entirety has been argued in the negative by Wallstrom [14], but there is some disagreement [15]. Proposals to “complete” the derivation, assuming the validity of Wallstrom’s criticism, have also been made in recent years [16, 17].

  5. See [18, 29], and [30,31,32] for similar field-based proposals.

  6. What seems to have stuck around for longer was the related enterprise of Stochastic Quantization (SQ)—but this too has mostly fallen out of fashion. An important difference between SQ and Nelson should be stressed: the “time” of SQ is a fictitious “simulation time,” whereas the time for Nelson is a real, physical time. See [40] for a recent perspective on Nelson-Yasue QFT; and see [41] for an introduction to SQ.

  7. Although the derivative \(\dot{{\varvec{x}}} = \textrm{d}{\varvec{x}}/\textrm{d}t\) here is ill-defined, rigorous definitions exist via Wiener processes [41, 46], which are here swept aside to ease the presentation.

  8. Here \((Df)({\varvec{x}},t):= \lim _{\epsilon \rightarrow 0^+} \epsilon ^{-1}\textrm{E}[f({\varvec{x}}_{t+\epsilon },t+\epsilon )-f({\varvec{x}}_t,t)|{\varvec{x}}_t={\varvec{x}}]\). One can define a “backward” stochastic process associated with the forward process, i.e., a rule relating the current positions to prior positions in a probabilistic fashion, and an associated backward derivative \(D_*\); see [13, 19, 47].

  9. Yasue [48] has shown that Nelson’s Second Law follows from a stochastic variational principle, together with the demand of positive semidefinite mean kinetic energy.

  10. See [54] for an animation of this motion.

  11. Or, from an average along a single trajectory, since this state has a time-independent density. In this case one must properly deal with autocorrelations in the time series in order to obtain correct stochastic error estimates; see, e.g., [55]

  12. Hence there is no “sign problem,” as it is known in the lattice community [57], for Schrödinger picture expectation values. However, whether Nelson’s theory can be used to study problems whose wave functions are not known beforehand is an open question, and there is a debate about the ability to compute Heisenberg picture expectation values, like \(\langle \widehat{x}(t) \widehat{x}(0) \rangle \), in Nelson’s theory. See [58, 59] for the debate.

  13. We refer the reader to [29, 60, 61] as standard references on the Schrödinger picture.

  14. Strictly speaking, these are just ordinary functions of many variables \(\phi _{\varvec{x}}\), but we use the term “functional” by convention due to the similarity with continuum formulations of QFT [60].

  15. One may either compute the norms abstractly using the operator algebra, or by evaluating the functional integral directly, for which standard Gaussian integration formulas apply.

  16. The diffusion coefficient \(\nu \) in Nelsonian QFT is therefore independent of any particle mass, or the Klein-Gordon mass which appears in the dispersion relation, unlike in the nonrelativistic theory where \(\nu = \hbar /2m\).

  17. The square of \(\omega _{{\varvec{x}},{\varvec{y}}}\) as a matrix is (minus) the lattice Laplacian operator, plus a mass term.

  18. These states are similar to the Newton-Wigner states considered, for example, in [62], except for the particular momentum-dependence of coefficients. The states I use here are built from “positive-frequency” components of the field operator, \((\phi ^+_{\varvec{x}})^\dag \).

  19. Note that the normalization of \(\Psi _1\) is \((D_{0,0})^{-1/2}\), implying the overlaps \(\langle \Psi _1({\varvec{x}}_1)|\Psi _1({\varvec{x}}_2)\rangle = D_{0,0}^{-1} D_{{\varvec{x}}_1,{\varvec{x}}_2}\). Since \(D_{0,0}\) is UV-sensitive, while \(D_{{\varvec{x}},{\varvec{y}}}\) remains finite in the continuum limit, the overlap of normalized states is suppressed by the cutoff scale for non-coincident points.

  20. It’s nevertheless true that \(\langle \phi _{{\varvec{x}}} \rangle = 0\) in the state \(\Psi _1\), since the ground state is symmetric under \(\phi \rightarrow -\phi \). But whereas the configuration \(\phi _{\varvec{x}}= 0\) is typical in the ground state, it is not typical in \(\Psi _1\).

  21. See [63] for an animation of the field beable in this state.

  22. For example, regularizing the canonical commutators by defining \([\widehat{\phi }({\varvec{x}}), \widehat{\pi }({\varvec{y}})] = if_\Lambda ({\varvec{x}}-{\varvec{y}})\) (with sufficiently quickly decaying \(f_\Lambda \)) rather than \(i\delta ({\varvec{x}}-{\varvec{y}})\) yields a vacuum \(\Psi _0(\phi ) \propto \exp [-\frac{1}{2} \int \textrm{d}^d p \; \omega ({\varvec{p}}) \phi ({\varvec{p}}) \phi (-{\varvec{p}})/ f_\Lambda ({\varvec{p}})]\), which in turn yields a likeliest field configuration with \(\varphi ({\varvec{x}}_0)^2 \propto \int \textrm{d}^d p f_\Lambda ({\varvec{p}})/\omega ({\varvec{p}})\), and is finite if \(f_\Lambda ({\varvec{p}})\) decays more quickly than \(1/{\varvec{p}}^{d-1}\).

  23. See [64] for an animation of this field.

  24. This observation is not limited to just Bohm or Nelson’s theories: it applies to any interpretation which takes wave functionals and field beables seriously.

  25. See [65] for an example of a Bohmian field beable for a 2-particle time-dependent state.

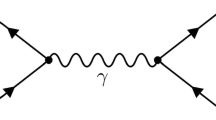
  26. The coefficients have a structure analogous to the standard Feynman diagrams of QFT. The quartic term in \(R^{(1)}\) corresponds to the tree-level diagram for the 4-point function, while the quadratic term in \(R^{(1)}\) is the 1-loop correction to the 2-point function. The sextic term in \(R^{(2)}\) gives a tree-level 6-point function; the quartic term gives the 1-loop contribution to the 4-point function (both 1PI and non-1PI diagrams); the quadratic term gives the 2-loop contribution to the 2-point function. The superficial degree of divergence of the loops is the same as the corresponding standard Feynman diagrams (see [66] for a standard account). In this way we see how diagrams similar to those of standard QFT “contribute” to the vacuum wave functional.

  27. Equations of striking similarity to these appear in the context of functional RG, where \(\ln \Psi _0\) is replaced by an RG fixed point action, and \(\mathcal P_n\) is replaced by a scaling operator associated with that fixed point; see, e.g., [68]. A connection between RG and stochastic processes has been explored in [69, 70].

  28. There are also terms proportional to \(\phi _{\pm {\varvec{p}}}\) with undetermined coefficients, reflecting the degeneracy of the free states \(| 1,\pm {\varvec{p}} \rangle \). We have set these coefficients to zero.

  29. The extension to (pure) lattice gauge theories should be possible, without too much difficulty, by utilizing methods from the stochastic quantization of such theories [41, 53].

  30. See also [74] for a Lorentz covariant proposal.

  31. This is perhaps borrowed from the association of the divergences in classical electromagnetism with a point-like model of electrons. In QFT, the association is more difficult to make, since the ontology of QFT is not as clear to begin with.

References

  1. Bell, J.S.:“Beables for Quantum Field Theory,” in Speakable and Unspeakable in Quantum Mechanics, ch. 19, pp. 173–180, Cambridge University Press, (1987)

  2. Bohm, D.: A Suggested Interpretation of the Quantum Theory in Terms of “Hidden" Variables. I. Phys. Rev. 85, 166–179 (1952)

    Article  ADS  MathSciNet  Google Scholar 

  3. Bohm, D.: A Suggested Interpretation of the Quantum Theory in Terms of “Hidden" Variables. II. Phys. Rev. 85, 180–193 (1952)

    Article  ADS  MathSciNet  Google Scholar 

  4. Dürr, D., Goldstein, S., Zanghí, N.: Quantum equilibrium and the origin of absolute uncertainty. Journal of Statistical Physics 67(5), 843–907 (1992)

    Article  ADS  MathSciNet  Google Scholar 

  5. Colijn, C., Vrscay, E.: Spin-dependent Bohm trajectories for hydrogen eigenstates. Physics Letters A 300, 334–340 (2002)

    Article  ADS  MathSciNet  Google Scholar 

  6. Krekels, S., Maes, C., Meerts, K., Struyve, W.: “Zig-zag dynamics in a Stern-Gerlach spin measurement,” arXiv:2311.13406

  7. Ney, A., Albert, D. Z.: The Wave Function: Essays in the Metaphysics of Quantum Mechanics. Oxford University Press, (2013)

  8. Norsen, T., Marian, D., Oriols, X.: Can the wave function in configuration space be replaced by single-particle wave functions in physical space? Synthese 192(10), 3125–3151 (2015)

    Article  MathSciNet  Google Scholar 

  9. Hubert, M., Romano, D.: The wave-function as a multi-field. European Journal for Philosophy of Science 8(3), 521–537 (2018)

    Article  MathSciNet  Google Scholar 

  10. Fényes, I.: Eine wahrscheinlichkeitstheoretische Begründung und Interpretation der Quantenmechanik. Zeitschrift fur Physik 132, 81–106 (1952)

    Article  ADS  MathSciNet  Google Scholar 

  11. Weizel, W.: Ableitung der quantentheorie aus einem klassischen, kausal determinierten modell. Zeitschrift für Physik 134(3), 264–285 (1953)

    Article  ADS  MathSciNet  Google Scholar 

  12. Bohm, D., Vigier, J.P.: Model of the causal interpretation of quantum theory in terms of a fluid with irregular fluctuations. Phys. Rev. 96, 208–216 (1954)

    Article  ADS  MathSciNet  Google Scholar 

  13. Nelson, E.: Derivation of the Schrödinger Equation from Newtonian Mechanics. Phys. Rev. 150, 1079–1085 (1966)

    Article  ADS  Google Scholar 

  14. Wallstrom, T.C.: On the derivation of the Schrödinger equation from stochastic mechanics. Foundations of Physics Letters 2(2), 113–126 (1989)

    Article  ADS  MathSciNet  Google Scholar 

  15. Kuipers, F.: Stochastic Mechanics: The Unification of Quantum Mechanics with Brownian Motion. Springer Nature Switzerland, (2023)

  16. Derakhshani, M.: “A Suggested Answer To Wallstrom’s Criticism: Zitterbewegung Stochastic Mechanics I,” arXiv:1510.06391, 2019

  17. Derakhshani, M.: “A Suggested Answer To Wallstrom’s Criticism: Zitterbewegung Stochastic Mechanics II,” arXiv:1607.08838, (2019)

  18. Bohm, D., Hiley, B.J.: The Undivided Universe: An Ontological Interpretation of Quantum Theory. Routledge, New York (1993)

    Google Scholar 

  19. Guerra, F.: Structural Aspects of Stochastic Mechanics and Stochastic Field Theory. Phys. Rept. 77, 263–312 (1981)

    Article  ADS  MathSciNet  Google Scholar 

  20. Seiler, E., “Stochastic quantization and gauge fixing in gauge theories,” in Stochastic Methods and Computer Techniques in Quantum Dynamics (H. Mitter and L. Pittner, eds.), pp. 259–308, Springer Vienna, (1984)

  21. Goldstein, S.: Stochastic mechanics and quantum theory. Journal of Statistical Physics 47(5), 645–667 (1987)

    Article  ADS  MathSciNet  Google Scholar 

  22. Bacciagaluppi, G.:“A Conceptual Introduction to Nelson’s Mechanics,” R. Buccheri, M. Saniga and A. Elitzur (eds), Endophysics, Time, Quantum and the Subjective, pp. 367–388

  23. Baker, D.J.: Against field interpretations of quantum field theory. British Journal for the Philosophy of Science 60(3), 585–609 (2009)

    Article  MathSciNet  Google Scholar 

  24. Fraser, D.: The fate of ‘particles’ in quantum field theories with interactions. Studies in History and Philosophy of Science Part B: Studies in History and Philosophy of Modern Physics 39(4), 841–859 (2008)

    Article  ADS  MathSciNet  Google Scholar 

  25. Wallace, D.: In defence of naiveté: The conceptual status of lagrangian quantum field theory. Synthese 151(1), 33–80 (2006)

    Article  MathSciNet  Google Scholar 

  26. Williams, P.: Scientific realism made effective. The British Journal for the Philosophy of Science 70(1), 209–237 (2019)

    Article  MathSciNet  Google Scholar 

  27. Egg, M., Lam, V., Oldofredi, A.: Particles, cutoffs and inequivalent representations. Foundations of Physics 47(3), 453–466 (2017)

    Article  ADS  MathSciNet  Google Scholar 

  28. Sebens, C.T.: The fundamentality of fields. Synthese 200(5), 380 (2022)

    Article  MathSciNet  Google Scholar 

  29. Holland, P.R.: The Quantum Theory of Motion: An Account of the de Broglie-Bohm Causal Interpretation of Quantum Mechanics. Cambridge University Press, (1993)

  30. Valentini, A.: (1996)“Pilot Wave Theory of Fields, Gravitation, and Cosmology,” in Bohmian Mechanics and Quantum Theory: An Appraisal (J. T. Cushing, A. Fine, and S. Goldstein, eds.), 184, 45–66, Kluwer Academic,

  31. Dewdney, C., Horton, G.:“de Broglie, Bohm and the Boson,” in Bohmian Mechanics and Quantum Theory: An Appraisal (J. T. Cushing, A. Fine, and S. Goldstein, eds.), vol. 184, pp. 169–189, Kluwer Academic, (1996)

  32. Struyve, W., Westman, H.: A minimalist pilot-wave model for quantum electrodynamics. Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences 463(2088), 3115–3129 (2007)

    Article  ADS  MathSciNet  Google Scholar 

  33. Bell, J.S., Aspect, A.: Speakable and Unspeakable in Quantum Mechanics: Collected Papers on Quantum Philosophy. Cambridge University Press, 2nd ed., (2004)

  34. Dürr, D., Goldstein, S., Tumulka, R., Zanghi, N.: Bell-type quantum field theories. J. Phys. A 38, R1 (2005)

    Article  ADS  MathSciNet  Google Scholar 

  35. Colin, S., Struyve, W.: A Dirac sea pilot-wave model for quantum field theory. J. Phys. A 40, 7309–7342 (2007)

    Article  ADS  MathSciNet  Google Scholar 

  36. Nikolić, H.: QFT as pilot-wave theory of particle creation and destruction. Int. J. Mod. Phys. A 25, 1477–1505 (2010)

    Article  ADS  MathSciNet  Google Scholar 

  37. Deckert, D., Esfeld, M., Oldofredi, A.: A Persistent Particle Ontology for Quantum Field Theory in Terms of the Dirac Sea. British Journal for the Philosophy of Science 70(3), 747–770 (2019)

    Article  MathSciNet  Google Scholar 

  38. Oldofredi, A., Öttinger, H.C.: The dissipative approach to quantum field theory: Conceptual foundations and ontological implications. European Journal for Philosophy of Science 11(1), 1–36 (2020)

    MathSciNet  Google Scholar 

  39. Guerra, F., Ruggiero, P.: New interpretation of the euclidean-markov field in the framework of physical minkowski space-time. Phys. Rev. Lett. 31, 1022–1025 (1973)

    Article  ADS  Google Scholar 

  40. Koide, T., Kodama, T.: “Stochastic variational method as quantization scheme: Field quantization of the complex Klein-Gordon equation,” PTEP, 2015(9), 093A03, (2015)

  41. Damgaard, P.H., Hüffel, H.: Stochastic quantization. Physics Reports 152(5), 227–398 (1987)

    Article  ADS  MathSciNet  Google Scholar 

  42. Wilson, K.G., Kogut, J.B.: The renormalization group and the epsilon expansion. Phys. Rept. 12, 75–200 (1974)

    Article  ADS  Google Scholar 

  43. Lepage, G.P.: “What is Renormalization?,” in Theoretical Advanced Study Institute in Elementary Particle Physics, (1989)

  44. Srednicki, M.: Quantum field theory. Cambridge University Press, (2007)

  45. Cao, T.Y., Schweber, S.S.: The conceptual foundations and the philosophical aspects of renormalization theory. Synthese 97(1), 33–108 (1993)

    Article  MathSciNet  Google Scholar 

  46. Pavliotis, G.: Stochastic Processes and Applications. Springer, (2014)

  47. Bohm, D., Hiley, B.: Non-locality and locality in the stochastic interpretation of quantum mechanics. Physics Reports 172(3), 93–122 (1989)

    Article  ADS  MathSciNet  Google Scholar 

  48. Yasue, K.: Stochastic calculus of variations. Journal of Functional Analysis 41(3), 327–340 (1981)

    Article  MathSciNet  Google Scholar 

  49. McClendon, M., Rabitz, H.: Numerical simulations in stochastic mechanics. Phys. Rev. A 37, 3479–3492 (1988)

    Article  ADS  MathSciNet  Google Scholar 

  50. Nitta, H., Kudo, T.: Time of arrival of electrons in the double-slit experiment. Phys. Rev. A 77, 014102 (2008)

    Article  ADS  Google Scholar 

  51. Hardel, V., Hervieux, P.A., Manfredi, G.:“Relaxation to quantum equilibrium and the Born rule in Nelson’s stochastic dynamics,” arXiv:2305.04084, (2023)

  52. Drummond, I., Duane, S., Horgan, R.: The stochastic method for numerical simulations: Higher order corrections. Nuclear Physics B 220(1), 119–136 (1983)

    Article  ADS  MathSciNet  Google Scholar 

  53. Batrouni, G.G., Katz, G.R., Kronfeld, A.S., Lepage, G.P., Svetitsky, B., Wilson, K.G.: Langevin simulations of lattice field theories. Phys. Rev. D 32, 2736–2747 (1985)

    Article  ADS  Google Scholar 

  54. Quantum Beables, “‘Hidden variables’ - Electron Motion in Hydrogen - Ground state,” YouTube, 5 July 2023. URL: https://www.youtube.com/shorts/7Xkwx5tYHII

  55. Montvay, I., Münster, G.: Quantum fields on a lattice. Cambridge University Press, Cambridge Monographs on Mathematical Physics (1997)

    Google Scholar 

  56. Nelson, E.: Quantum Fluctuations. Princeton University Press, (1985)

  57. Alexandru, A., Basar, G., Bedaque, P.F., Warrington, N.C.: Complex paths around the sign problem. Rev. Mod. Phys. 94(1), 015006 (2022)

    Article  ADS  MathSciNet  Google Scholar 

  58. Blanchard, P., Golin, S., Serva, M.: Repeated measurements in stochastic mechanics. Phys. Rev. D 34, 3732–3738 (1986)

    Article  ADS  MathSciNet  Google Scholar 

  59. Derakhshani, M., Bacciagaluppi, G.: “On Multi-Time Correlations in Stochastic Mechanics,” arXiv:2208.14189, (2022)

  60. Hatfield, B.: Quantum field theory of point particles and strings. Addison-Wesley. Frontiers in Physics, Volume 75, (1992)

  61. Jackiw, R.W. :“Schrödinger picture analysis of boson and fermion quantum field theories,” tech. rep., MIT. Cent. Theor. Phys., Cambridge, MA, 1987

  62. Myrvold, W.C.: What is a wavefunction? Synthese 192(10), 3247–3274 (2015)

    Article  MathSciNet  Google Scholar 

  63. Quantum Beables, “‘Hidden Variables’ - Two-Particle States in Quantum Field Theory,” YouTube, 6 July 2023. URL: https://youtube.com/shorts/WSUrnf6Exw4

  64. Quantum Beables, “’Hidden Variables’ : de Broglie-Bohm Field Beable in Quantum Field Theory (1-Particle State),” YouTube, 17 August 2023. URL: https://www.youtube.com/shorts/d4nayygNVyg

  65. Quantum Beables, “’Hidden Variables’ : de Broglie-Bohm Field Beable in Quantum Field Theory (2-Particle State),” YouTube, 26 November 2023. URL: https://www.youtube.com/shorts/lGO-YhFSFTI

  66. Amit, D.J., Martín-Mayor, V.: Field Theory, The Renormalization Group, and Critical Phenomena, 3rd edn. World Scientific, Singapore (2005)

    Book  Google Scholar 

  67. Coester, F., Haag, R.: Representation of states in a field theory with canonical variables. Phys. Rev. 117, 1137–1145 (1960)

    Article  ADS  MathSciNet  Google Scholar 

  68. Rosten, O.J.: Fundamentals of the Exact Renormalization Group. Phys. Rept. 511, 177–272 (2012)

    Article  ADS  MathSciNet  Google Scholar 

  69. Carosso, A., Hasenfratz, A., Neil, E.T.: “Stochastic Renormalization Group and Gradient Flow in Scalar Field Theory,” in 37th International Symposium on Lattice Field Theory, (2019)

  70. Carosso, A.: Stochastic Renormalization Group and Gradient Flow. JHEP 01, 172 (2020)

    Article  ADS  MathSciNet  Google Scholar 

  71. Symanzik, K.: Schrödinger Representation and Casimir Effect in Renormalizable Quantum Field Theory. Nucl. Phys. B 190, 1–44 (1981)

    Article  ADS  MathSciNet  Google Scholar 

  72. Cushing, J.T.: Quantum Mechanics: Historical Contingency and the Copenhagen Hegemony. The University of Chicago Press, (1994)

  73. Struyve, W.: Pilot-wave theory and quantum fields. Rept. Prog. Phys. 73, 106001 (2010)

    Article  ADS  MathSciNet  Google Scholar 

  74. Derakhshani, M., Kiessling, M. K. H. , Tahvildar-Zadeh, A.S.: “Covariant Guiding Laws for Fields,” arXiv:2110.09683, (2021)

  75. S. Goldstein, W. Struyve, and R. Tumulka, “The Bohmian Approach to the Problems of Cosmological Quantum Fluctuations,” arXiv:1508.01017

  76. P. N. Kaloyerou, “An Ontological Interpretation of Boson Fields,” in Bohmian Mechanics and Quantum Theory: An Appraisal (J. T. Cushing, A. Fine, and S. Goldstein, eds.), vol. 184, pp. 155–167, Kluwer Academic, 1996

  77. Vink, J.C.: Particle Trajectories for Quantum Field Theory. Found. Phys. 48(2), 209–236 (2018)

    Article  ADS  MathSciNet  Google Scholar 

  78. D. Bohm, N. R. Hanson, M. B. Hesse, N. Kemmer, A. B. Pippard, M. Pryce, D. Edge, and S. Toulmin, Quanta and Reality. American Reasearch Council, 1962

  79. Laws of Nature Discussion Series on Quantum Theory and Relativity, YouTube Channel. URL: https://www.youtube.com/@lawsofnature3448

Download references

Acknowledgements

I would like to thank Maaneli Derakhshani, Ward Struyve, and Charles Sebens for discussions and feedback. This work has also benefited from comments received after presenting the material at the 2023 European Conference on Foundations of Physics in Bristol, UK, as well as the online Laws of Nature Series [79]. Lastly, I thank the reviewer for their valuable comments and suggestions for improving the manuscript. This work was supported in part by U.S. DOE Grant No. DE-FG02-95ER40907.

Author information

Authors and Affiliations

  1. Department of Physics, George Washington University, Washington, DC, USA

    Andrea Carosso

Authors
  1. Andrea Carosso
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

A.C. is the sole author of the manuscript.

Corresponding author

Correspondence to Andrea Carosso.

Ethics declarations

Conflict of interest

The authors declare no competing interests.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Carosso, A. Simulating Nelsonian Quantum Field Theory. Found Phys 54, 30 (2024). https://doi.org/10.1007/s10701-024-00766-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10701-024-00766-6

Search

Navigation