Abstract
In this paper we show that \(\psi\)-ontic models, as defined by Harrigan and Spekkens (HS), cannot reproduce quantum theory. Instead of focusing on probability, we use information theoretic considerations to show that all pure states of \(\psi\)-ontic models must be orthogonal to each other, in clear violation of quantum mechanics. Given that (i) Pusey, Barrett and Rudolph (PBR) previously showed that \(\psi\)-epistemic models, as defined by HS, also contradict quantum mechanics, and (ii) the HS categorization is exhausted by these two types of models, we conclude that the HS categorization itself is problematic as it leaves no space for models that can reproduce quantum theory.

Similar content being viewed by others
Notes
In principle, PBR leaves open the possibility of \(\psi\)-epistemic models that violate statistical independence. Given that statistical independence is linked to entropy additivity, we will see that that loophole can be closed as well.
Note that, while many functions called “entropy” exist, the Shannon entropy is the only indicator of variability that is continuous, monotonic and linear in probability [30]. It is also the one that provides the correct link to the thermodynamic entropy.
Full calculations with all steps are included in Appendix.
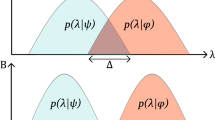
The notation of HS uses \(\lambda\) as both the value and measure for integration, as often done in single variable integrals.
The logarithm is assumed to be in base 2.
Note that \(H_S\) is the “discrete” Shannon entropy that acts on a countable set of coefficients that sum to one.
Note that the theorem applies to all ψ-ontic models, including the ψ-supplemented ones.
The degree of degeneracy one has to require is also extreme. For a qubit, in the simplest case, we would have a \(\psi\)-ontic state for each direction on the Bloch sphere, meaning the space of ontic states is the sphere \(S^2\). The space of all possible epistemic states is the set of all possible distributions integrable to one. This is an infinite dimensional function space. However, the space of mixed states for a quibit is the interior of the Bloch sphere, which is a bounded three-dimensional manifold. The equivalence classes of epistemic states, then, must be infinite dimensional.
As for instance de Broglie-Bohm theory, Everett’s relative state formulation, relational quantum mechanics or the many-worlds perspective etc..
References
Harrigan, N., Spekkens, R.: Einstein, incompleteness, and the epistemic view of quantum states. Found. Phys. 40, 125 (2010)
Leifer, M., Maroney, O.: Maximally epistemic interpretations of the quantum state and contextuality. Phys. Rev. Lett. 110, 120401 (2013)
Leifer, M., Pusey, M.F.: Is a time symmetric interpretation of quantum theory possible without retrocausality? Proc. R. Soc. A 473, 20160607 (2017)
Branciard, C.: How \(\psi \)-epistemic models fail at explaining the indistinguishability of quantum states. Phys. Rev. Lett. 113, 020409 (2014)
Hermens, R.: How real are quantum states in \(\psi \)-ontic models? Found. Phys. (2021). https://doi.org/10.1007/s10701-021-00448-7
Wood, C., Spekkens, R.: The lesson of causal discovery algorithms for quantum correlations: causal explanations of Bell-inequality violations require fine-tuning. New J. Phys. 17, 033002 (2015)
Ringbauer, M., Duffus, B., Branciard, C., Cavalcanti, E., White, A., Fedrizzi, A.: Measurement of the reality of the wave function. Nat. Phys. 11, 249 (2015)
Mazurek, M., Pusey, M.F., Kuniwal, R., Resch, K., Spekkens, R.: An experimental test of noncontextuality without unphysical idealizations. Nat. Commun. 7, 11780 (2016)
Bartlett, S., Rudolph, T., Spekkens, R.: Reconstruction of Gaussian quantum mechanics from Liouville mechanics with an epistemic restriction. Phys. Rev. A 86, 012103 (2012)
Oldofredi, A., Lopez, C.: On the classification between \(\psi \)-ontic and \(\psi \)-epistemic ontological models. Found. Phys. 50, 1315 (2020)
Hance, J., Rarity, J., Ladyman, J.: Could wavefunctions simultaneously represent knowledge and reality? Quant. Stud. Math. Found. 9, 333 (2022)
Pusey, M.F., Barrett, J., Rudolph, T.: On the reality of the quantum state. Nat. Phys. 6, 475 (2012)
Leifer, M.: Is the quantum state real? An extended review of \(\psi\)-ontology theorems. Quanta 3, 67 (2014)
Leifer, M.: \(\psi \)-epistemic models are exponentially bad at explaining the distinguishability of quantum states. Phys. Rev. Lett. 112, 160404 (2014)
Lewis, P., Jennings, D., Barrett, J., Rudolph, T.: Distinct quantum states can be compatible with a single state of reality. Phys. Rev. Lett. 109, 150404 (2012)
Colbeck, R., Renner, R.: Is a system’s wave function in one-to-one correspondence with its elements of reality? Phys. Rev. Lett. 108, 150402 (2012)
Colbeck, R., Renner, R.: A system’s wave function is uniquely determined by its underlying physical state. New J. Phys. 19, 013016 (2017)
Hardy, L.: Are quantum states real? Int. J. Mod. Phys. B (2013). https://doi.org/10.1142/S0217979213450124
Barrett, J., Cavalcanti, E., Lal, R., Maroney, O.: No \(\psi \)-epistemic model can fully explain the indistinguishability of quantum states. Phys. Rev. Lett. 112, 250403 (2014)
Patra, M., Pironio, S., Massar, S.: No-Go theorems for \(\psi \)-epistemic models based on a continuity assumption. Phys. Rev. Lett. 111, 090402 (2013)
Mansfield, S.: Reality of the quantum state: towards a stronger \(\psi \)-ontology theorem. Phys. Rev. A 94, 042124 (2016)
Schlosshauer, M., Fine, A.: Implications of the Pusey–Barrett–Rudolph quantum no-go theorem. Phys. Rev. Lett. 108, 260404 (2012)
Schlosshauer, M., Fine, A.: Is the Pusey–Barrett–Rudolph theorem compatible with quantum nonseparability? arXiv Preprint (2013). arXiv:1306.5805v1
Schlosshauer, M., Fine, A.: No-go theorem for the composition of quantum system. Phys. Rev. Lett. 112, 070407 (2014)
Aaronson, S., Bouland, A., Chua, L., Lowther, G.: \(\psi \)-epistemic theories: the role of symmetry. Phys. Rev. A 88, 032111 (2013)
Ben-Menahem, Y.: The PBR theorem: whose side is it on? Stud. Hist. Philos. Sci. Part B Stud. Hist. Philos. Mod. Phys. 57, 80 (2017)
Rizzi, A.: Does the PBR theorem rule out a statistical understanding of QM? Found. Phys. 48, 1770 (2018)
Oldofredi, A., Calosi, C.: Relational quantum mechanics and the PBR theorem: a peaceful coexistence. Found. Phys. (2021). https://doi.org/10.1007/s10701-021-00485-2
DeBrota, J., Stacey, B.: FAQBism. arXiv Preprint (2019). arXiv:1810.13401
Carcassi, G., Aidala, C., Barbour, J.: Variability as a better characterization of Shannon entropy. Eur. J. Phys. 42, 045102 (2021)
Ash, R.: Information Theory, new edn. Dover, New York (2010)
Nielsen, M., Chuang, I.: Quantum Computation and Quantum Information. Cambridge University Press, Cambridge (2010)
Werner, R.F.: Comments on ‘What bell did’. J. Phys. A Math. Theor. 47, 424011 (2014)
Pitowsky, I.: Quantum Probability-Quantum Logic. Springer, Berlin (1989)
Halvorson, H.: To be a realist about quantum theory. In: Lombardi, O., Fortin, S., Lopez, C., Holik, F. (eds.) Quantum Worlds: Perspectives on the Ontology of Quantum Mechanics. Cambridge University Press, Cambridge (2019)
Bengtsson, I., Zyczkowski, K.: Geometry of Quantum States: An Introduction to Quantum Entanglement. Cambridge University Press, Cambridge (2017)
Kirkpatrick, K.: arXiv Preprint (2001). https://arxiv.org/pdf/quant-ph/0109146.pdf
D’Espagnat, B.: arXiv Preprint (2001). https://arxiv.org/pdf/quant-ph/0111081.pdf
Acknowledgements
Andrea Oldofredi is grateful to the Fundação para a Ciência e a Tecnologia (FCT) for financial support (Grant No. 2020.02858.CEECIND). This work is in connection to Assumptions of Physics, a larger project that aims to identify a handful of physical principles from which the basic laws can be rigorously derived (https://assumptionsofphysics.org).
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix: Calculation
Appendix: Calculation
The figure and the derivations in the Appendix are courtesy of the Assumptions of Physics project.
Entropy of mixed non-overlapping distributions. We want to show that, given two non-overlapping probability distributions \(\rho _1\) and \(\rho _2,\) the entropy of \(\rho = \frac{1}{2} \rho _1 + \frac{1}{2} \rho _2\) is given by
Let \(U_1, U_2 \subset \Lambda\) be the respective supports of the distributions, which are non-overlapping i.e. \(U_1 \cap U_2 = \emptyset\). We then have
Entropy of quantum mixed states. We want to show that, given two states \(\psi\) and \(\phi\), the entropy of the mixed state \(\rho = \frac{1}{2}|\psi \rangle \langle \psi | + \frac{1}{2}|\phi \rangle \langle \phi |\) is

Note that \(\psi\) and \(\phi\) will identify a two-dimensional subspace which can be thought, without loss of generality, as a qubit and therefore can be represented by a Bloch sphere. The picture represents the intersection of the Bloch sphere with the plane identified by \(\psi\) and \(\phi\). As \(\rho\) is an equal mixture of the two states, it will be represented by the midpoint between the two. Taking the line that goes through \(\rho\) and the center of the sphere, we can see that \(\rho\) can also be seen as the mixture of the states \(+\) and − which, since they represent equal and opposite directions, form a basis. To diagonalize \(\rho\), then, means to express it in terms of + and −.
If \(\theta _{\psi \phi }\) is the angle between \(\psi\) and \(\phi\), we have
The angle is divided by two because the angle on the Bloch sphere (i.e. in physical space) is double the angle in the Hilbert space. For example, for \(z^+\) and \(z^-\) the angle on the Bloch sphere would be \(\pi\) and the inner product is zero (i.e. opposite directions in physical space correspond to orthogonal states).
Now we express \(\psi\) and \(\phi\) in terms of \(+\) and −, remembering that they form a basis. Given that \(\rho\) is at the midpoint, the figure is vertically symmetric. The angle between \(\psi\) and +, then, is half of \(\theta _{\psi \phi }\). The inner product between \(\psi\) and + is
Keeping in mind that we are composing vectors in the Hilbert space (and not in the geometry of the physical space) we have
The density matrices corresponding to the pure states are
We can now calculate the mixture
As \(\rho\) is in a diagonal form, the entropy is given by (14).
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Carcassi, G., Oldofredi, A. & Aidala, C.A. On the Reality of the Quantum State Once Again: A No-Go Theorem for \(\psi\)-Ontic Models. Found Phys 54, 14 (2024). https://doi.org/10.1007/s10701-023-00748-0
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s10701-023-00748-0