Skip to main content
SpringerLink
Log in

A Time-Symmetric Soliton Dynamics à la de Broglie

  • Published:
Foundations of Physics Aims and scope Submit manuscript

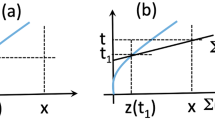
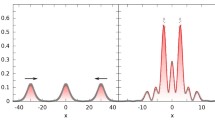
Abstract

In this work we develop a time-symmetric soliton theory for quantum particles inspired from works by de Broglie and Bohm. We consider explicitly a non-linear Klein–Gordon theory leading to monopolar oscillating solitons. We show that the theory is able to reproduce the main results of the pilot-wave interpretation for non interacting particles in a external electromagnetic field. In this regime, using the time symmetry of the theory, we are also able to explain quantum entanglement between several solitons and we reproduce the famous pilot-wave nonlocality associated with the de Broglie-Bohm theory.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Data Availability

No data associated in the manuscript.

Notes

  1. We use the Minkowski metric \(\eta _{\mu \nu }\) with signature \(+,-,-,-\) and the convention \(\hbar =1\), \(c=1\).

  2. In [22] we obtained the expression for \({\mathcal {M}}_{u}(x)\) (Eq. 66) without expliciting the first order correction \(O(\xi )\). The details of the calculations show that we have \({\mathcal {M}}_{u}(x)\simeq {\mathcal {M}}_{\Psi} (z(\tau ))+{\varvec{\xi }}\cdot {\varvec{\nabla }}{\mathcal {M}}_{\Psi} (z(\tau ))+O(\xi ^{2})\). A careful analysis shows that we have \(\partial _{\mu} {\mathcal {M}}_{u}(z(\tau ))=\partial _{\mu} {\mathcal {M}}_{\Psi} (z(\tau ))\).

  3. We have the fluid conservation: \(v_{u}\partial \ln {(f^{2}{\mathcal {M}}_{u}\delta ^{3}\sigma _{0})}:=\frac{d}{d\tau }\ln {(f^{2}{\mathcal {M}}_{u}\delta ^{3}\sigma _{0})}=0\).

  4. Ehrenfest theorem has been also applied by Bialynicki-Birula and Mycielski [5] in the context of classical Gausson dynamics driven by external electromagnetic forces. The theorem has also been used by Durt and coworkers [10, 33] in the particular context of the nonrelativistic Schrödinger–Newton equations. Our own independent results are based on the relativistic NLKG an is valid for a large range of equations.

  5. This invariance allows us to circumvent the conclusions of the Hobart–Derrick theorem [17, 31, 34] which usually precludes the existence of static and stable solitons in 3D space. In “Appendix 2” we give an elementary proof of this result.

  6. We stress that de Broglie rejected the non singular wave \(u(t,r)=e^{-i\omega _{0} t}\frac{\sin {(\omega _{0} r)}}{r}\) solution of the homogeneous wave equation. This alternative solution was later rediscovered by many authors including Mackinnon [36] and Barut [3]. At the experimental level such non dispersive de Broglie waves have been recently created [32] using optical methods inspired from diffraction-free beams works [23]. This is also related to the work of Fink concerning time reversal mirrors in acoustics and optics [2, 29] that plays with causality. This could perhaps play a role in order to develop a physical wave analog of our time symmetric soliton.

  7. We have \(G^{(0)}_{sym,\omega }(R)=\frac{1}{2}[G^{(0)}_{ret,\omega }(R)+G^{(0)}_{adv,\omega }(R)]\) and \(G^{(0)}_{ret/adv,\omega }(R)=\frac{e^{\pm i\omega R}}{4\pi R}\) are the retarded and advanced Green functions respectively.

  8. We have also \(K^{(0)}_{sym}(x,x')=\int _{-\infty }^{+\infty }G^{(0)}_{sym,\omega }(R)e^{-i\omega (t-t')}\frac{d\omega }{2\pi }\) with \(G^{(0)}_{sym,\omega }(R)\) given by Eq. 34.

  9. We mention that F. Fer in 1957 developed a method for analyzing the motion of singularities in the context of the DSP [27, 28]. Moreover, his approach using only retarded Green’s functions missed the time-symmetry needed to recover the wave particle duality considered here.

  10. More precisely we have \(\sigma _{\mp} =r\left( 1+\frac{\xi \ddot{z}}{2} \mp \frac{r\xi z^{(3)}}{6}-\frac{r^{2}(\ddot{z})^{2}}{24}+\frac{3(\xi \ddot{z})^{2}}{8}\right) +O(r^{4})\) with \(z^{(3)}:=\frac{d^{3} z(\tau )}{d\tau ^{3}}\).

  11. A proof is obtained by using the Fourier transform \(K(x,x')=\int \frac{d^{4}k}{(2\pi )^{4}}e^{ik(x-x')}G_{k}\). Equation 49 reads thus \(G_{k}=G_{k}^{(0)}+G_{k}^{(0)}(e^{2}A^{2}-2eAk)G_{k}\), i.e., \(G_{k}=\frac{G_{k}^{(0)}}{1-(e^{2}A^{2}-2eAk)G_{k}^{(0)}}\). Using \(G_{k}^{(0)}=-1/k^{2}\) we deduce \(G_{k}=-1/(k-eA)^{2}\) and after using the inverse Fourier transform we obtain \(K_{sym}(x,x')=K^{(0)}_{sym}(x,x')e^{-ieA(z)(x-x')}\).

  12. To prove this rather general statement a qualitative argument could go like this: Considering the LKG equation \(D^{2}\Psi =-\omega _{0}^{2}\Psi \) in a electrostatic potential \(V({\textbf{x}})\) the first Born order scattering amplitude for an incident plane wave \(\Psi _{0}({\textbf{x}})=e^{ik\textbf{n}_{0}\cdot {\textbf{x}}}\) (with \(k^{2}=\omega ^{2}-\omega _{0}^{2}\)) reads \(\Psi _{s}\simeq -2\omega \int d^{3}{\textbf{x}}''G_\omega ^{(0)}({\textbf{x}},{\textbf{x}}'')eV({\textbf{x}}'')\Psi _{0}({\textbf{x}}'')\simeq -2\omega \frac{e^{ikr}}{4\pi r} e\hat{V}_{\textbf{q}}\) where we neglected the quadratic term \(e^{2}V^{2}\), \(G_\omega ^{(0)}({\textbf{x}},{\textbf{x}}'')=\frac{e^{ikR|{\textbf{x}}-{\textbf{x}}''|}}{4\pi |{\textbf{x}}-{\textbf{x}}''|}\) [computed here for a retarded wave], and where \(\hat{V}_{\textbf{q}}\) is the Fourier transform of the potential at the wave wavevector \(\textbf{q}=k(\textbf{n}_{s}-\textbf{n}_{0})\). In a Coulomb field for example we have \(\Psi _{s}\simeq -\frac{2\omega \alpha }{\textbf{q}^{2}}\frac{e^{ikr}}{ r}\). The same calculation done for a plane wave solution of the linearized equation for u \(D^{2}u=0\) leads to the same expression with \(\omega ^{2}\) replacing \(k^{2}\). Therefore the scattered field \(u_{s}\) is smaller than \(\Psi _{s}\) by a coefficient \(\frac{u_{s}}{\psi _{s}}\simeq k^{2}/\omega ^{2}=v^{2}\) where v is the particle velocity. In general \(v^{2}\ll 1\) and \(u_{s}\) is negligible.

  13. We have \(D_{i}=\partial _{i}+eA(x_{i})\), \(\partial _{i}:=\frac{\partial }{\partial x_{i}}\) and the polar form \(\Psi _{N}(x_{1},\ldots ,x_{i},\ldots ,x_{N})=a_{N}(x_{1},\ldots ,x_{i},\ldots ,x_{N})e^{iS_{N}(x_{1},\ldots ,x_{i},\ldots ,x_{N})}\).

  14. This is also true in the recent important experimental/theoretical work done with hydrodynamical analogs by Bush, Vervoort and coworkers [38, 40, 50]. In these experiments there is a form of superdeterminism driven by stationnary Faraday waves moving along a liquid surface. Yet, the development was for the moment limited to time-independent configurations like in the first Aspect’s experiments. Time varying settings would require a stronger conspiracy that can be generated by a time symmetric causality. The problem is thus to generate the right amount of conspiracy in order to reproduce quantum predictions and not look too magical or too contrived. This is exactly the solution offered in the present work with non-linear waves involving time symmetry.

  15. The error is small in the integration since \(U(f^{2})-f^{2}N(f^{2})\sim 1/r^{6}\) at large distances.

  16. This description made in the regime \(\omega _{0}T\gg 1\) is of course an approximation that neglects the transient effects associated with the discontinuities at A and B contributing to the energy balance.

  17. Of course the problem is absent if we limit the present model to neutral solitons with \(e=0\).

  18. Note that in order to have \(I_{p}< \infty \) we must have \(m>\frac{3}{2(p+1)}\) so that globally \(m> max\left[ \frac{1}{2},\frac{3}{2(p+1)}\right] \) [45].

References

  1. Bacciagaluppi, G., Valentini, A.: Quantum Theory at the Crossroads: Reconsidering the 1927 Solvay Conference. Cambridge University Press, Cambridge (2009)

    MATH  Google Scholar 

  2. Bacot, V., Labousse, M., Eddi, A., Fink, M., Fort, E.: Time reversal and holography with spacetime transformations. Nat. Phys. 12, 972–977 (2016)

    Google Scholar 

  3. Barut, A.O.: \(E=\hbar \omega \). Phys. Lett. A 143, 349–352 (1990)

    ADS  MathSciNet  Google Scholar 

  4. Bell, J.S.: On the Einstein Podolsky Rosen Paradox. Physica 1, 195–200 (1964)

    MathSciNet  Google Scholar 

  5. Bialynicki-Birula, I., Mycielski, J.: Nonlinear wave mechanics. Ann. Phys. 100, 62–93 (1976)

    ADS  MathSciNet  Google Scholar 

  6. Bohm, D.: A suggested interpretation of the quantum theory in terms of “Hidden’’ variables. Phys. Rev. 85, 166–179 (1952)

    ADS  MathSciNet  MATH  Google Scholar 

  7. Bohm, D.J., Hiley, B.J.: Nonlocality in quantum theory understood in terms of Einstein’s nonlinear field approach. Found. Phys. 11, 529–546 (1981)

    ADS  MathSciNet  Google Scholar 

  8. Bohm, D., Hiley, B.J.: The Undivided Universe. Routledge, London (1993)

    MATH  Google Scholar 

  9. Chandrasekhar, S.: Chapter 4. In: An Introduction to the Study of Stellar Structures. University of Chicago Press, Chicago (1939)

  10. Collin, S., Durt, T., Willox, R.: L. de Broglie’s double solution program: 90 years later. Ann. Fond. de Broglie 42, 19–70 (2017)

    MathSciNet  Google Scholar 

  11. Costa de Beauregard, O.: Une réponse à l’argument dirigé par Einstein, Podolsky et Rosen contre l’interprétation bohrienne des phénomènes quantiques. C. R. Acad. Sci. (Paris) 236, 1632 (1953)

    MathSciNet  MATH  Google Scholar 

  12. Cramer, J.G.: The transactional interpretation of quantum mechanics. Rev. Mod. Phys. 58, 647 (1986)

    ADS  MathSciNet  Google Scholar 

  13. De Broglie, L.: Sur la fréquence propre de l’électron. C. R. Acad. Sci. (Paris) 180, 498–500 (1925)

    MATH  Google Scholar 

  14. De Broglie, L.: Ondes et mouvements. Gauthier-Villars, Paris (1926)

    MATH  Google Scholar 

  15. De Broglie, L.: Radium. J. Phys. 8, 225–241 (1927). (Translated in: de Broglie, L., and Brillouin, L.: Selected papers on wave mechanics. Blackie and Son, Glasgow (1928))

    Google Scholar 

  16. De Broglie, L.: Une tentative d’interprétation causale et non linéaire de la mécanique ondulatoire: la théorie de la double solution. Gauthier-Villars, Paris (1956). (Translated in: de Broglie, L.: Nonlinear wave mechanics: A causal interpretation. Elsevier, Amsterdam (1960))

    MATH  Google Scholar 

  17. Derrick, G.H.: Comments on nonlinear wave equations as models for elementary particles. J. Math. Phys. 5, 1252–1254 (1964)

    ADS  MathSciNet  Google Scholar 

  18. Dirac, P.A.M.: Classical theory of radiating electrons. Proc. R. Soc. Lond. A 167, 148–169 (1938)

    ADS  MATH  Google Scholar 

  19. Drezet, A.: Quantum nonlocality of single photon state? Found. Phys. Lett. 19, 459–470 (2006)

    MATH  Google Scholar 

  20. Drezet, A.: Lorentz-invariant, retrocausal, and deterministic hidden variables. Found. Phys. 49, 1166–1199 (2019)

    ADS  MathSciNet  MATH  Google Scholar 

  21. Drezet, A.: The guidance theorem of de Broglie. Ann. Fond. de Broglie 46, 65–85 (2021)

    Google Scholar 

  22. Drezet, A.: Quantum solitodynamics: non-linear wave mechanics and pilot-wave theory. Found. Phys. 53, 31 (2023)

    ADS  MathSciNet  MATH  Google Scholar 

  23. Durnin, J., Miceli, J.J., Eberly, J.H.: Diffraction-free beams. Phys. Rev. Lett. 58, 1499–1501 (1987)

    ADS  Google Scholar 

  24. Einstein, A., Podolsky, B., Rosen, N.: Can quantum-mechanical description of physical reality be considered complete? Phys. Rev. 47, 777–780 (1935)

    ADS  MATH  Google Scholar 

  25. Fargue, D.: Permanence of the corpuscular appearance and non linearity of the wave equation. In: Diner, S., et al. (eds.) The Wave-Particle Dualism, pp. 149–172. D. Reidel Publishing, Dordrecht (1984)

    Google Scholar 

  26. Fargue, D.: Louis de Broglie’s “double solution’’, a promising but unfinished theory. Ann. Fond. de Broglie 42, 9–18 (2017)

    MathSciNet  Google Scholar 

  27. Fer, F.: Les solutions singulières des équations d’onde et la théorie de la double solution. Doctorate Thesis, Bureau de documentation minière, Paris (1957)

  28. Fer, F.: Guidage des particules, onde singulières. In: L. de Broglie, sa conception du monde physique, p. 279. Paris (1973)

  29. Fink, M.: Time reversed acoustics. Phys. Today 50, 34–40 (1997)

    Google Scholar 

  30. Fokker, A.D.: Ein invarianter variationssatz für die bewegung mehrerer elektrischer massenteilchen. Z. Phys. 58, 386–393 (1929)

    ADS  MATH  Google Scholar 

  31. Goldstone, J., Jackiw, R.: Quantization of nonlinear waves. Phys. Rev. D 11, 1486–1498 (1975)

    ADS  Google Scholar 

  32. Hall, L.A., Abouraddy, A.F.: Observation of optical de Broglie–Mackinnon wave packets. Nat, Phys. 19, 435–444 (2023)

    Google Scholar 

  33. Hatifi, M., Lopez-Fortin, C., Durt, T.: De Broglie’s double solutions: limitations of the self-gravity approach. Ann. Fond. de Broglie 43, 63–90 (2018)

    Google Scholar 

  34. Hobart, R.H.: On the instability of a class of unitary field models. Proc. Phys. Soc. 82, 201–203 (1963)

    ADS  MathSciNet  Google Scholar 

  35. Hoyle, F., Narlikar, J.V.: Cosmology and action-at-a-distance electrodynamics. Rev. Mod. Phys. 67, 113–155 (1995)

    ADS  MathSciNet  Google Scholar 

  36. Mackinnon, L.: A nondispersive de Broglie wave packet. Found. Phys. 8, 157–176 (1978)

    ADS  Google Scholar 

  37. Mie, G.: Grundlagen einer Theorie der Materie. Ann. Phys. (Berl.) 99, 1–40 (1912)

    ADS  MATH  Google Scholar 

  38. Nikolaev, V., Vervoort, L.: Aspects of superdeterminism made intuitive. Found. Phys. 53, 17 (2023)

    ADS  MathSciNet  MATH  Google Scholar 

  39. Page, L.: A generalization of electrodynamics with applications to the structure of the electron and to non-radiating orbits. Phys. Rev. 18, 292 (1921)

    ADS  Google Scholar 

  40. Papatryfonos, K., Vervoort, L., Nachbin, A., Labousse, M., Bush, J.W.M.: Bell test in a classical pilot-wave system (2022). arXiv:2208.08940

  41. Petiau, G.: Sur la représentation des corpuscules en interaction avec des champs extérieurs par des fonctions d’ondes à singularités localisées. C. R. Acad. Sci. (Paris) 239, 344–346 (1954)

    MathSciNet  MATH  Google Scholar 

  42. Petiau, G.: Quelques cas de représentation des corpuscules en intéraction avec des champs extérieurs dans la nouvelle forme de la mécanique ondulatoire (Théorie de la double solution). Sémin. L. de Broglie Théor. Phys. (Paris) 24, exposé 18 (1954–1955)

  43. Petiau, G.: Sur la détermination de fonctions d’ondes à singularités localisées mobiles décrivant des trajectoires circulaires dans le cas d’un potentiel extérieur central. C. R. Acad. Sci. (Paris) 239, 2491–2493 (1955)

    MathSciNet  MATH  Google Scholar 

  44. Rosen, G.: Particlelike solutions to nonlinear scalar wave theories. J. Math. Phys. 6, 1269–1272 (1965)

    ADS  MathSciNet  Google Scholar 

  45. Rosen, G.: Existence of particle-like solution to nonlinear field theories. J. Math. Phys. 7, 2066–2070 (1966)

    ADS  Google Scholar 

  46. Rybakov, Yu.P., Saha, R.: Soliton model of atom. Found. Phys. 25, 1723–1731 (1995)

    ADS  Google Scholar 

  47. Schwinger, J.: Electromagnetic mass revisited. Found. Phys. 13, 373–383 (1983)

    ADS  MathSciNet  Google Scholar 

  48. ’t Hooft, G.: Free will in the theory of everything (2017). arXiv:1709.02874v2

  49. Tetrode, H.: Über den Wirkungszusammenhang der Welt. Eine Erweiterung der klassischen Dynamik. Z. Phys. 10, 317–328 (1922)

    ADS  Google Scholar 

  50. Vervoort, L.: Are hidden-variable theories for pilot-wave systems possible? Found. Phys. 48, 803–826 (2018)

    ADS  MathSciNet  MATH  Google Scholar 

  51. Wharton, K.B., Argaman, N.: Colloquium—Bell’s theorem and locally mediated reformulations of quantum mechanics. Rev. Mod. Phys. 92, 021002 (2020)

    ADS  MathSciNet  Google Scholar 

  52. Wheeler, J.A., Feynman, R.P.: Interaction with the absorber as the mechanism of radiation. Rev. Mod. Phys. 17, 157–181 (1945)

    ADS  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institut NEEL, CNRS and Université Grenoble Alpes, 38000, Grenoble, France

    Aurélien Drezet

Authors
  1. Aurélien Drezet
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Aurélien Drezet.

Ethics declarations

Conflict of interest

Author declares no competing interest for this work.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Drezet, A. A Time-Symmetric Soliton Dynamics à la de Broglie. Found Phys 53, 72 (2023). https://doi.org/10.1007/s10701-023-00711-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10701-023-00711-z

Keywords

Search

Navigation