Skip to main content
SpringerLink
Log in

Information-Theoretic Interpretation of Quantum Formalism

  • Published:
Foundations of Physics Aims and scope Submit manuscript

Abstract

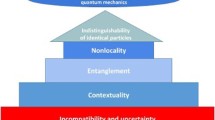
We present an information-theoretic interpretation of quantum formalism based on a Bayesian framework and devoid of any extra axiom or principle. Quantum information is construed as a technique for analyzing a logical system subject to classical constraints, based on a question-and-answer procedure. The problem is posed from a particular batch of queries while the constraints are represented by the truth table of a set of Boolean functions. The Bayesian inference technique consists in assigning a probability distribution within a real-valued probability space to the joint set of queries in order to satisfy the constraints. The initial query batch is not unique and alternative batches can be considered at will. They are enabled mechanically from the initial batch, quite simply by transcribing the probability space into an auxiliary Hilbert space. It turns out that this sole procedure leads to exactly rediscover the standard quantum information theory and thus provides an information-theoretic rationale to its technical rules. In this framework, the great challenges of quantum mechanics become simple platitudes: Why is the theory probabilistic? Why is the theory linear? Where does the Hilbert space come from? In addition, most of the paradoxes, such as uncertainty principle, entanglement, contextuality, nonsignaling correlation, measurement problem, etc., become straightforward features. In the end, our major conclusion is that quantum information is nothing but classical information processed by a mature form of Bayesian inference technique and, as such, consubstantial with Aristotelian logic.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

References

  1. Shannon, C. E.: A mathematical theory of communication, Bell System Technical Journal, 27 379–423 , 656–715. (1948), http://pespmc1.vub.ac.be/books/Shannon-TheoryComm.pdf

  2. Szilard, L.: On the Decrease of Entropy in a Thermodynamic System by the Intervention of Intelligent Beings, Zeitschrift fuer Physik, 53 840–856, (1929). http://sns.ias.edu/~tlusty/courses/InfoInBioParis/Papers/Szilard1929.pdf

  3. Brillouin, L.: Science and Information Theory, Academic Press, New-York, (1956). http://www.physics.mcgill.ca/~delrio/courses/phys559/lectures%20and%20notes/Brillouin-Information-Theory-Ch1-4.pdf

  4. Wheeler, J.A.: Information, physics, quantum: the search for links. In: Zurek, W. (ed.) Complexity, entropy, and the physics of information, pp. 3–28. Addison-Wesley, Redwood City (1990)

    Google Scholar 

  5. Landauer, R.: The physical nature of information, Physics Letters A, 217 188–193, (1996). http://cqi.inf.usi.ch/qic/64_Landauer_The_physical_nature_of_information.pdf

  6. Jaynes, E. T.: Probability Theory: the logic of science, Cambridge University Press, Cambridge, UK, (2003). http://omega.math.albany.edu:8008/JaynesBook.html

  7. Jaynes, E. T.: Clearing up mysteries, in: J. Skilling (Ed.), Maximum entropy and Baysian methods, Kluwer, Dordrecht, (1989), pp. 1–29. http://bayes.wustl.edu/etj/articles/cmystery.pdf

  8. Rovelli, C.: Relational quantum mechanics. Int. J. Theor. Phys. 35, 1637–1678 (1996). https://doi.org/10.1007/BF02302261

    Article  MathSciNet  MATH  Google Scholar 

  9. Caves, C.M., Fuchs, C.A., Schack, R.: Quantum probabilities as Bayesian probabilities. Phys. Rev. A 65(2), 22305 (2002). arXiv:quant-ph/0106133

    Article  ADS  MathSciNet  Google Scholar 

  10. Mermin, N. D.: Qbism puts the scientist back into science, Nature, 507 421–422, (2014). http://www.nature.com/polopoly_fs/1.14912!/menu/main/topColumns/topLeftColumn/pdf/507421a.pdf

  11. Fuchs, C. A.: QBism, The Perimeter of Quantum Bayesianism (2010). arXiv:1003.5209

  12. Boyd, S., Vandenberghe, L.: Convex optimization, Cambridge University Press, Cambridge, (2004). http://www.stanford.edu/~boyd/cvxbook/bv_cvxbook.pdf

  13. Cox, R. T.: Probability, frequency, and reasonable expectation, American Journal of Physics, 14 1–13, (1946). http://jimbeck.caltech.edu/summerlectures/references/ProbabilityFrequencyReasonableExpectation.pdf

  14. Maassen, H., Uffink, J.B.M.: Generalized entropic uncertainty relations. Phys. Rev. Lett. 60, 1103–1106 (1988)

    Article  ADS  MathSciNet  Google Scholar 

  15. Frank, R.L., Lieb, E.H.: Entropy and the uncertainty principle. Annales Henri Poincaré 13, 1711–1717 (2012). arXiv:1109.1209

    Article  ADS  MathSciNet  MATH  Google Scholar 

  16. Yeung, R.W.: Information theory and network coding. Springer-Verlag, New York (2008)

    MATH  Google Scholar 

  17. Murty, K.G.: Linear programming. Wiley, New York (1983)

    MATH  Google Scholar 

  18. Caticha, A.: Lectures on probability, entropy, and statistical physics, In: 28th International Workshop on Bayesian Inference and Maximum Entropy Methods in Science and Engineering, (2008). arXiv:0808.0012

  19. Feldmann, M.: New loophole for the Einstein-Podolsky-Rozen paradox. Found. Phys. Lett. 8(1), 41–53 (1995). https://doi.org/10.1007/BF02187530. arXiv:quant-ph/9904051

    Article  MathSciNet  Google Scholar 

  20. Barrett, J., Linden, N., Massar, S., Pironio, S., Popescu, S., Roberts, D.: Nonlocal correlations as an information-theoretic resource. Phys. Rev. A 71(2), 022101 (2005). arXiv:quant-ph/0404097

    Article  ADS  Google Scholar 

  21. Popescu, S., Rohrlich, D.: Quantum nonlocality as an axiom. Found. Phys. 24, 379 (1994). arXiv:quant-ph/9508009

    Article  ADS  MathSciNet  Google Scholar 

  22. Birkhoff, G., von Neumann, J.: The logic of quantum mechanics, Annals of Mathematics, 37 823, (1936). http://www.jstor.org/stable/1968621

  23. Jaynes, E. T.: Information theory and statistical mechanics. II, Phys. Rev. 108(2) 170–190, (1957). http://bayes.wustl.edu/etj/articles/theory.2.pdf

  24. Wigner, E. P.: Normal form of antiunitary operators, J. Math. Phys. 1(5) 409–413, (1960). https://link.springer.com/chapter/10.1007/978-3-662-02781-3_38

  25. Durt, T., Englert, B.-G., Bengtsson, I., Zyczkowski, K.: On mutually unbiased bases. Int. J. Quantum Info. 8, 535–640 (2010). arXiv:1004.3348

    Article  MATH  Google Scholar 

  26. Kalev, A., Gour, G.: Mutually unbiased measurements in finite dimensions, New Journal of Physics, 16(5), 053038 (2014). arXiv:1401.2706. http://stacks.iop.org/1367-2630/16/i=5/a=053038

  27. Schwinger, J.: Unitary Operator Bases, Proc. Nat. Acad. Sci. USA. 46, 560 (1960). http://www.pubmedcentral.nih.gov/picrender.fcgi?artid=222876 &blobtype=pdf

  28. Wiener, N.: The role of the observer, Philosophy of Science, 3(3), 307–319 (1936). http://www.jstor.org/stable/184668

  29. Feynman, R., Weinberg, S.: Elementary particle physics and the laws of physics. Gen. Relativ. Gravitat. 33, 615–616 (1986)

    Google Scholar 

  30. Klein, F.: A comparative review of recent researches in geometry, Gesammelte mathematische Abhandlungen, 1, 460–497 (1931). arXiv:0807.3161. http://gdz.sub.uni-goettingen.de/dms/load/img/?PPN=PPN243240503 &DMDID=dmdlog45

  31. Zuber, J.-B.: Invariances in physics and group theory, In: Conference “Lie and Klein; the Erlangen program and its impact on mathematics and physics”, Strasbourg, Sept. 2012, (2013). arXiv:1307.3970

  32. Deutsch, D.: Quantum theory, the Church-Turing principle and the universal quantum computer, Proceedings of the Royal Society of London A: Mathematical, Physical and Engineering Sciences 400(1818), 97–117 (1985). https://people.eecs.berkeley.edu/~christos/classics/Deutsch_quantum_theory.pdf

  33. Gray, J.: Plato’s Ghost: the modernist transformation of mathematics. Princeton University Press, Princeton (2008)

    Book  MATH  Google Scholar 

  34. Bouleau, N.: La jonction entre la théorie du potentiel et les probabilités, Cahiers du séminaire d’histoire des mathématiques, 8, 43–66 (1987). http://www.numdam.org/article/CSHM_1987__8__43_0.pdf

  35. Meyer, P.-A., Dellacherie, C.: Probabilité et potentiel, vol. 1 and 2, Hermann, Paris, 1975, (2008)

  36. Hiley, B. J.: Process and the implicate order: their relevance to quantum theory and mind. https://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.357.4976 &rep=rep1 &type=pdf

  37. Aerts, D., Gabora, L., Sozzo, S., Veloz, T.: Quantum structure in cognition: Fundamentals and applications, In: V. Privman, V. Ovchinnikov (Eds.), Proceedings of the Fifth International Conference on Quantum, Nano and Micro Technologies (ICQMN 2011), International Academy, Research and Industry Association, (2011), pp. 57–62. arXiv:1104.3344

  38. Busemeyer, J.R., Bruza, P.D.: Quantum models of cognition and decision. Cambridge University Press, Cambridge (2012)

    Book  Google Scholar 

  39. Bruza, P., Busemeyer, J., Gabora, L.: Introduction to the Special Issue on Quantum Cognition (2013). arXiv:1309.5673

  40. Dzhafarov, E.N., Kujala, J.V.: Selectivity in probabilistic causality: where psychology runs into quantum physics. J. Math. Psychol. 56, 54–63 (2012). arXiv:1110.2388

    Article  MathSciNet  MATH  Google Scholar 

  41. Dzhafarov, E.N., Kujala, J.V.: Quantum entanglement and the issue of selective influences in psychology: an overview. Lecture Notes Comput. Sci. 7620, 184–195 (2012). arXiv:1209.0041

    Article  MathSciNet  Google Scholar 

  42. Haven, E., Khrennikov, A.: Quantum Soc. Sci. Cambridge University Press, Cambridge (2013)

    Book  MATH  Google Scholar 

  43. Clark, S., Coecke, B., Grefenstette, E., Pulman, S., Sadrzadeh, M.: A quantum teleportation inspired algorithm produces sentence meaning from word meaning and grammatical structure (2013). arXiv:1305.0556

  44. Ionicioiu, R.: Quantum mechanics: Knocking at the gates of mathematical foundations, In: I. Parvu, G. Sandu, I. Toader (Eds.), Romanian Studies in Philosophy of Science, Vol. 313 of Boston Studies in the Philosophy and History of Science, Springer, (2015). arXiv:1506.04511

  45. Einstein, A., Born, M.: The Born-Einstein letters. Walker and Company, New-York (1971)

    Google Scholar 

  46. Feynman, R.: Lessons on Probability and Uncertainty in quantum mechanics, Cornell Messenger Lecture Archive, (2011)

  47. Fuchs, C.A.: Quantum mechanics as quantum information (and only a little more). In: Khrennikov, A. (ed.) Quantum Theory: Reconsideration of Foundations, pp. 463–543. Växjö University Press, Växjö, Sweden (2002) . arXiv:quant-ph/0205039

    Google Scholar 

  48. Articles, In: J. T. Cushing, A. Fine, S. Goldstein (Eds.), Bohmian Mechanics and Quantum Theory: An Appraisal, Kluwer, Dordrecht, (1996)

  49. Griffiths, R.B., Omnès, R.: Consistent histories and quantum measurements. Phys. Today 52(8), 26–31 (1999)

    Article  Google Scholar 

  50. Cramer, J.G.: An overview of the transactional interpretation of quantum mechanics. Int. J. Theor. Phys. 27, 227–236 (1988)

    Article  MathSciNet  Google Scholar 

  51. Ghirardi, G. C., Pearle, P.: Dynamical reduction theories: Changing quantum theory so the statevector represents reality, In: M. F. A. Fine, L. Wessels (Eds.), Proceedings of the Biennial Meeting of the Philosophy of Science Association, Philosophy of Science Association, East Lansing, MI, (1990)

  52. Zurek, W.H.: Decoherence, Einselection and the existential interpretation (The Rough Guide). Phil. Trans. R. Soc. Lond. A 356, 1793–1821 (1998). arXiv:quant-ph/9805065

    Article  ADS  MathSciNet  Google Scholar 

  53. Grangier, P.: Contextual objectivity: a realistic interpretation of quantum mechanics (2000). arXiv:quant-ph/0012122

  54. Deutsch, D.: The fabric of reality: the science of parallel universes and its implications. Allen Lane, New York (1997)

    Google Scholar 

  55. Vaidman, L.: The many-worlds interpretation of quantum mechanics, In: E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy, Stanford University, Stanford, CA, (2002). http://plato.stanford.edu/entries/qm-manyworlds/

  56. Spekkens, R.W.: Contextuality for preparations, transformations, and unsharp measurements. Phys. Rev. A 71, 052108 (2005). arXiv:quant-ph/0406166

    Article  ADS  Google Scholar 

  57. Chiribella, G., D’Ariano, G.M., Perinotti, P.: Informational derivation of quantum theory. Phys. Rev. A 84(1), 012311 (2011). arXiv:1011.6451

    Article  ADS  Google Scholar 

  58. Hardy, L.: Reconstructing quantum theory (2013). arXiv:1303.1538

  59. Moeller, M. P., Masanes, L.: Information-theoretic postulates for quantum theory (2012). arXiv:1203.4516

  60. Masanes, L., Mueller, M.P., Augusiak, R., Perez-Garcia, D.: A digital approach to quantum theory. Proc. Nat. Acad. Sci. USA 110(41), 16373 (2013). arXiv:1208.0493

    Article  ADS  Google Scholar 

  61. Chiribella, G., D’Ariano, G. M., Perinotti, P.: Quantum from principles, In: R. Spekkens, G. Chiribella (Eds.), Quantum theory: informational foundations and foils, Springer Verlag, in press. arXiv:1506.00398

  62. Oeckl, R.: A local and operational framework for the foundations of physics. arXiv:1610.09052

  63. Pitowsky, I.: Quantum mechanics as a theory of probability (2005). arXiv:quant-ph/0510095

  64. Janotta, P., Hinrichsen, H.: Generalized probability theories: what determines the structure of quantum theory? J. Phys. A Math. General 47(32), 323001 (2014). arXiv:1402.6562

    Article  MathSciNet  MATH  Google Scholar 

  65. Pawłowski, M., Paterek, T., Kaszlikowski, D., Scarani, V., Winter, A., Żukowski, M.: Information causality as a physical principle. Nature 461(7267), 1101–1104 (2009)

    Article  ADS  Google Scholar 

  66. Barnum, H., Barrett, J., Clark, L.O., Leifer, M., Spekkens, R., Stepanik, N., Wilce, A., Wilke, R.: Entropy and information causality in general probabilistic theories. N. J. Phys. 12, 033024 (2010). arXiv:0909.5075

    Article  MathSciNet  MATH  Google Scholar 

  67. Caticha, A.: Entropic dynamics: mechanics without mechanism (2017). arXiv:1704.02663

  68. Fuchs, C. A., Schack, R.: Quantum-Bayesian Coherence: The No-Nonsense Version (2013). arXiv:1301.3274

  69. Leifer, M. S., Spekkens, R. W.: Formulating Quantum Theory as a Causally Neutral Theory of Bayesian Inference (2011). arXiv:1107.5849

  70. De Raedt, H., Katsnelson, M. I., Michielsen, K.: Quantum theory as the most robust description of reproducible experiments (2013). arXiv:1303.4574

  71. Fuchs, C.A., Mermin, N.D., Schack, R.: An introduction to QBism with an application to the locality of quantum mechanics. Am. J. Phys. 82, 749–754 (2014). arXiv:1311.5253

    Article  ADS  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. AX, Paris, France

    Michel Feldmann

Authors
  1. Michel Feldmann
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Michel Feldmann.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Feldmann, M. Information-Theoretic Interpretation of Quantum Formalism. Found Phys 53, 58 (2023). https://doi.org/10.1007/s10701-023-00690-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10701-023-00690-1

Keywords

Search

Navigation