The Transition from Quantum Field Theory to One-Particle Quantum Mechanics and a Proposed Interpretation of Aharonov–Bohm Effect

Abstract

In this article, we demonstrate a sense in which the one-particle quantum mechanics (OPQM) and the classical electromagnetic four-potential arise from the quantum field theory (QFT). In addition, the classical Maxwell equations are derived from the QFT scattering process, while both classical electromagnetic fields and potentials serve as mathematical tools to approximate the interactions among elementary particles described by QFT physics. Furthermore, a plausible interpretation of the Aharonov–Bohm (AB) effect is raised within the QFT framework. We provide a quantum treatment of the source of electromagnetic potentials and argue that the underlying mechanism in the AB effect can be understood via interactions among electrons described by QFT theory where the interactions are mediated by virtual photons.

Appendix A

In this appendix, we are further stressing on the nature of classical electromagnetic fields and the relationships between classical fields with quantum fields. In classical theory, the Hamiltonian of a charged particle in the presence of classical potential $A_{\mu } (t,\varvec{x})$ can be given as

$$ H = \frac{1}{2m}[\varvec{p} - q\varvec{A}(t,\varvec{x})]^{2} + q\phi (t,\varvec{x}) $$

(A1)

This gives the Lorentz equation of motion as

$$ m\frac{{d^{2} \varvec{x}}}{{d^{2} t}} = q[ - \vec{\nabla }\phi - \frac{{\partial \varvec{A}}}{\partial t} + \vec{\nabla }(\varvec{v} \cdot \varvec{A}) - (\varvec{v} \cdot \vec{\nabla })\varvec{A}] $$

(A2)

This equation can be written in a more elegant form by introducing EMF defined in Eq. (25) as

$$ m\frac{{d^{2} \varvec{x}}}{{d^{2} t}} = q\varvec{E}(t,\varvec{x}) + q\varvec{v} \times \varvec{B}(t,\varvec{x}) $$

(A3)

Therefore, the necessity for the introduction of EMF through Eq. (25) which also induces people to believe that EMF corresponds to real entities of nature lies inside of Eq. (A3). As we can see, the fields $\varvec{E}(t,\varvec{x})$ and $\varvec{B}(t,\varvec{x})$ can be uniquely identified through Eq. (A3) with the information of the acceleration and velocity of the charged particle. This makes physicists to believe that the functions $\varvec{E}(t,\varvec{x})$ and $\varvec{B}(t,\varvec{x})$ corresponds to some real entities of nature since they seem to be uniquely valued at every space–time point. However, this follows the belief that the acceleration and velocity of the charged particle corresponds to some real quantities and can be uniquely valued at every space–time point along with the trajectory. In order to uniquely identify one quantity we need to identify another quantity uniquely since these quantities are bonded together in one equation. Measuring each quantity $\varvec{E}(t,\varvec{x})$ and $\varvec{B}(t,\varvec{x})$ precisely at every space–time point requests us to treat the particle as a single space–time point with zero size. This condition is too unrealistic to be satisfied in classical physics; it demands more internal structures of the macroscopic particle. However, we know that for microscopic particles, the velocity along a trajectory is not well defined due to the uncertainty principle. This makes Eq. (A3) as well as the bond break down in the micro-world. Therefore, we replace $\varvec{p}$ with $- i{\vec{\nabla }}$ in Eq. (A1) and obtain the Hamiltonian of an electron in the non-relativistic limit as

$$ H = \frac{1}{2m}[ - i{\vec{\nabla }} + e\varvec{A}(t,\varvec{x})]^{2} - e\phi (t,\varvec{x}) $$

(A4)

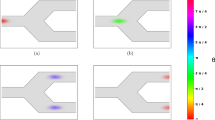
In radiation gauge, we get the wave-function of the electron as $\left| \psi \right\rangle = \exp ( - i\int_{0}^{\varvec{x}} {e\varvec{A}\text{(}\varvec{x^{\prime}}\text{)} \cdot d\varvec{x^{\prime}}} )\left| \varvec{p} \right\rangle$ in case of a non-time-varying vector potential. For the AB effect depicted in Fig. 2, the electron traveling enclosing a circle will pick up a phase shift that can be measured which is $\int_{S} {e\varvec{B}(\varvec{x})} \cdot d\varvec{S = }e\varPhi_{m}$, where $\varPhi_{m}$ is the total magnetic flux through the closed surface. However, it reminds that two wave-functions with the phase difference of $2n\pi$($n = \pm 1, \pm 2 \ldots$) still cannot be distinguished. Therefore, neither classical physics nor quantum physics can uniquely quantify $\varvec{E}(t,\varvec{x})$ and $\varvec{B}(t,\varvec{x})$ at each space–time point precisely. This comes as expected actually since we already argued that the classical potential $A_{\mu } (t,\varvec{x})$ together with classical EMF defined by Eq. (25) are emergent properties and arise from QED process. In Eq. (A3), EMF is introduced to approximate the interactions between charged particles governed by quantum physics.

At this stage, we argue that different physical quantities along with different theories arise at different spatial–temporal scales. The most fundamental nature law at the deepest level may be unique; however, the ignorance of the detailed structures at smaller scales permits physicists to create theories, which are approximately effective at larger scales. Moreover, physicists create mathematical equations which predict the evolutions of nature, and the mathematical form of the physical quantities on both sides of the equation needs to be constructed consistently in order to fit the equation form. As we can see from our derivations of the classical potentials given in Equation (21), this mathematical form of the classical four-potential is constructed to fit the framework of OPQM in order to give the same predictions with QED theory in low energy limit. Hence, in the view of physical laws from micro-world to macro-world, the emergence of $A_{\mu } (t,\varvec{x})$ follows the mathematical construction of the framework of OPQM, while the EMF follows the Lorentz force equation, or in other words we can say that these quantities are bonded with the framework of OPQM and framework of Lorentz force equation, respectively. Therefore, these quantities cannot be divorced from their frameworks, and the nature does not specify what these quantities are without referring to what roles that they are playing in the frameworks. To be more specific, let us just simply multiply by 2 on both sides of Eq. (A3) and rescale the quantities $m^{\prime} = 2m$,$\varvec{E^{\prime}}(t,\varvec{x}) = 2\varvec{E}(t,\varvec{x})$ and $\varvec{B^{\prime}}(t,\varvec{x}) = 2\varvec{B}(t,\varvec{x})$ such that the equivalence relation with the rescaled quantities still holds (that is, if we revalue the mass of every macroscopic object in our universe, the EMF has to be revalued accordingly). In this way, the new equation with the rescaled quantities works just as good as the old one, but nature does not tell us which one we should use, and which quantity that is, $\varvec{E^{\prime}}(t,\varvec{x})$ or $\varvec{E}(t,\varvec{x})$ should be defined as the real physical entity of nature. Therefore, in this case we can safely speak that, at this macroscopic scale, only the equivalence relation in Eq. (A3) is the concrete thing that we should stick with. Any transformation of Eq. (A3) with new defined quantities must mathematically maintain such equivalent relation in order to give the same physical measurement predictions. This is also what happens from Eq. (A2) to Eq. (A3). Alternatively, we can rescale the strength of EMF and the charge q instead of m in Eq. (A3), and then the above argument also applies. Moreover, quantities defined at one scale may break down at another scale, such as color or the temperature of an object which cannot be well defined at the microscopic scale since they are originally from something else that are more fundamental. The similar argument applies to the classical potential $A_{\mu } (t,\varvec{x})$ and EMF which both arise originally from low energy QED physics. In addition, EMF role is insignificant in the mathematical constructions of OPQM and QED frameworks, QED and OPQM are complete theories even without the EMF as we can see in Eqs. (1) and (5). Next we are going to provide another evidence which reaffirm our statement.

Suppose that there exists a static classical field $\varvec{E}(\varvec{x}) \ne 0$ and $\varvec{B}(t,\varvec{x}) = 0$ somewhere in the “source” free region, for simplicity, we assume that the polarization of $\varvec{E}(\varvec{x})$ is in the z direction in the reference frame $(t,\varvec{x})$, i.e., $E_{x} (\varvec{x}) = E_{y} (\varvec{x}) = 0$. Therefore, the static field $E_{z} (\varvec{x})$ can be given as

$$ E_{z} (\varvec{x}) = i\int {\frac{{d\omega d^{3} \varvec{k}}}{{(2\pi )^{3} }}\text{[}\tilde{E}_{z} (\omega ,\varvec{k})e^{{ - i(\omega t - \varvec{k} \cdot \varvec{x})}} - \tilde{E}_{z}^{\dag } (\omega ,\varvec{k})e^{{i(\omega t - \varvec{k} \cdot \varvec{x})}} ]} $$

(A5)

in order to get a non-time-varying function $E_{z} (\varvec{x})$, we require $\tilde{E}_{z} (\omega ,\varvec{k}) = \delta (\omega )\tilde{f}(\varvec{k})$ in which $\delta (\omega )$ is the Dirac-Delta function and $\tilde{f}(\varvec{k})$ is a function of wave-vector k. Next, we perform a Lorentz boost with velocity v in z direction and obtain a new field $E^{\prime}_{z} (t^{\prime},\varvec{x^{\prime}})$ written in $(t^{\prime},\varvec{x^{\prime}})$ frame with relation

$$ E^{\prime}_{z} (t^{\prime},\varvec{x^{\prime}}) = E_{z} (t,\varvec{x}) $$

(A6)

The modes expansion of $E^{\prime}_{z} (t^{\prime},\varvec{x^{\prime}})$ can be given as

$$ E^{\prime}_{z} (t^{\prime},\varvec{x^{\prime}}) = i\int {\frac{{d\omega^{\prime}{d^{3}}\varvec{k^{\prime}}}}{{(2\pi )^{3} }}\text{[}\tilde{E}^{\prime}_{z} (\omega^{\prime},\varvec{k^{\prime}})e^{{ - i(\omega^{\prime}t^{\prime} - \varvec{k^{\prime}} \cdot \varvec{x^{\prime}})}} - \tilde{E}^{\prime \dag}_{z} (\omega^{\prime},\varvec{k^{\prime}})e^{{i(\omega^{\prime}t^{\prime} - \varvec{k^{\prime}} \cdot \varvec{x^{\prime}})}} ]} $$

(A7)

In the new reference frame we have $\left\{ {\begin{array}{*{20}c} {t^{\prime} = \gamma (t + vz)} \\ {z^{\prime} = \gamma (z + vt)} \\ \end{array} } \right\}$ and $\left\{ {\begin{array}{*{20}c} {\omega^{\prime} = \gamma (\omega + vk_{z} )} \\ {k^{\prime}_{z} = \gamma (k_{z} + v\omega )} \\ \end{array} } \right\}$ with $\gamma = 1/\sqrt {1 - v^{2} }$. Therefore, we can obtain the relation $\tilde{E}_{z} (\omega ,\varvec{k}) = \tilde{E}^{\prime}_{z} (\omega^{\prime},\varvec{k^{\prime}})$ as a result of Eq. (A6) and $e^{{i(\omega^{\prime}t^{\prime} - \varvec{k^{\prime}} \cdot \varvec{x^{\prime}})}} = e^{{i(\omega t - \varvec{k} \cdot \varvec{x})}}$, i.e., the Fourier components of field $E_{z} (\varvec{x})$ does not change in the new reference frame. This result is also what we expect in quantum field theory: the probability corresponding to measurement outcomes must be a Lorentz invariant. Plug relation $\tilde{E}^{\prime}_{z} (\omega^{\prime},\varvec{k^{\prime}}) = \delta (\omega )\tilde{f}(\varvec{k})$ into Eq. (A7), after integrating over frequency $\omega^{\prime}$ we find a nonzero value at $\omega^{\prime} = \gamma vk_{z}$. Therefore, we have brought a non-time-varying field $E_{z} (\varvec{x})$ into a time-varying field $E^{\prime}_{z} (t^{\prime},\varvec{x^{\prime}})$ by a Lorentz boost. Furthermore, we noticed that the phase velocity of the modes $e^{{ - i(\omega^{\prime}t^{\prime} - \varvec{k^{\prime}} \cdot \varvec{x^{\prime}})}}$ in $E^{\prime}_{z} (t^{\prime},\varvec{x^{\prime}})$ can be given as $u^{\prime} = \frac{{\gamma vk_{z} }}{{\left| {\varvec{k^{\prime}}} \right|}}$ measured in the reference frame $(t^{\prime},\varvec{x^{\prime}})$, therefore, the modes which comprise the field $E^{\prime}_{z} (t^{\prime},\varvec{x^{\prime}})$ are propagating at speed $u^{\prime} \le v$ which is slower than light. Thus, the modes in $E^{\prime}_{z} (t^{\prime},\varvec{x^{\prime}})$ cannot be photons. In fact, neither $E^{\prime}_{z} (t^{\prime},\varvec{x^{\prime}})$ nor $E_{z} (\varvec{x})$ can be quantized. Or, at least we can say, the “quantization” of these classical fields and classical potentials do not give rise to particles behaving like photons. The reason is, as shown in our main text of this article, the EMF are emergent quantities and are not directly linked with some elementary particles, i.e., photons in this scenario.

For the FEMF introduced following Eqs. (2) and (25), we have

$$ \begin{aligned} \varvec{E}(t,\varvec{x}) = i\int {\frac{{d^{3} \varvec{k}}}{{(2\pi )^{3} }}} \sqrt {\frac{{\omega_{\varvec{k}} }}{2}} \sum\limits_{\lambda = 1,2} {[\tilde{E}_{\varvec{k}}^{\lambda }\varvec{\nu}^{\lambda } e^{{ - i(\omega_{\varvec{k}} t - \varvec{k} \cdot \varvec{x})}} - \tilde{E}_{\varvec{k}}^{\lambda \dag }\varvec{\nu}^{\lambda } e^{{i(\omega_{\varvec{k}} t - \varvec{k} \cdot \varvec{x})}} ]} \hfill \\ \varvec{B}(t,\varvec{x}) = i\int {\frac{{d^{3} \varvec{k}}}{{(2\pi )^{3} }}} \frac{1}{{\sqrt {2\omega_{\varvec{k}} } }}\sum\limits_{\lambda = 1,2} {[\tilde{B}_{\varvec{k}}^{\lambda } \varvec{k} \times\varvec{\nu}^{\lambda } e^{{ - i(\omega_{\varvec{k}} t - \varvec{k} \cdot \varvec{x})}} - \tilde{B}_{\varvec{k}}^{\lambda \dag } \varvec{k} \times\varvec{\nu}^{\lambda } e^{{i(\omega_{\varvec{k}} t - \varvec{k} \cdot \varvec{x})}} ]} \hfill \\ \end{aligned} $$

(A8)

Note that these quantities above are totally different from the EMF quantities in Eq. (A3). In Eq. (A8), the FEMF is defined from a Lorentz vector in the quantized form as Eq. (2). Therefore, the FEMF are just two different mathematical constructions that are made up of photons, and they, as a result, form a real Lorentz tensor. In addition, the FEMF also plays an essential role in the mathematical formulation of QED theory. However, there is no counterpart of EMF in quantum physics and the EMF emerges in macro-world due to the collective effects from micro-world. Now we see that the FEMF and EMF possess totally different physical meanings, the FEMF is made up of photons which are elementary particles of nature while EMF only serves as a mathematical tool in classical physics. The difference in physical meanings between FEMF and EMF originates from the differences between $\hat{A}_{\mu }$ in Eq. (1) and $A_{\mu } (\varvec{x})$ in Eq. (5) which is a derived quantity; indeed it would be less confusing if physicists historically have denoted the EMF and FEMF using two different symbols since they hold different physical meanings. Moreover, the mathematical structure of $\hat{A}_{\mu }$ and FEMF are fixed, while $A_{\mu } (\varvec{x})$ and EMF appear to be completely arbitrary in the real world since they depend on the sources. Finally, we should be more careful over the differences of their mathematical structures rather than what historically people have symbolized them since only their mathematical structures tell us what these quantities really are.