Fluid models for customer service web chat systems with interactive automated service

Abstract

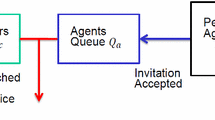
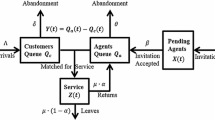
In addition to traditional call centers, companies have increasingly started developing a new type of customer contact center over the Internet, rather than using phone calls, where customers communicate with agents online through a website. Apart from lining up for an agent service, customers have the option of an interactive self-help response and thus can obtain faster service. In such a new customer contact center, managers are inclined to induce customers to use an automated service to reduce manpower costs. Under such circumstances, delay announcements play an important role in guiding customer behavior derived from an avoid-the-crowd option into a self-help choice. Motivated by this, we characterize the underlying stochastic processes by establishing a fluid model in overloaded regimes, and propose that the key to the model is a customer response resulting from system interactions based on a deterministic approximation. Furthermore, we prove that the fluid limit of the model is a unique solution to a complicated queueing system using differential equations. Based on numerical examples, the approximation is shown to be effective in overloaded regimes through comparisons with simulations.A key managerial insight is that the lower the service level is required for staffing, the more effective automated service and the guidance of delay announcement are.

Appendices

Appendix

First, for convenience regarding our analysis, the fluid scaled processes are defined as follows:

$$\begin{aligned} {{\bar{Q}}}_s^n\left( t \right)= & {} {\varPi _1}{{\left( {n\int _0^t {\mu \min \left( {s,{{\bar{\mathrm{Z}}}^n}\left( u \right) du} \right) } } \right) } \big / n}, \end{aligned}$$

(28)

$$\begin{aligned} {{\bar{Q}}}_a^n\left( t \right)= & {} {\varPi _2}{{\left( {n\int _0^t {\gamma '{{\left( {{{\bar{\mathrm{Z}}}^n}\left( u \right) - s} \right) }^{{ + }}}du} } \right) } \big / n}, \end{aligned}$$

(29)

$$\begin{aligned} {{\bar{Q}}}_A^n\left( t \right)= & {} {\varPi _3}{{\left( {n\int _0^t {\delta {{{{\bar{A}}}}^n}\left( u \right) du} } \right) } \big / n}, \end{aligned}$$

(30)

$$\begin{aligned} {{\bar{\varLambda }}} _a^n\left( t \right)= & {} \sum \limits _{r = 1}^{\varLambda ^n\left( t \right) } {{{1\left\{ {{d_r} \le {w_r}} \right\} } \big / n}}, \end{aligned}$$

(31)

$$\begin{aligned} {{\bar{\varLambda }}} _{\mathrm{A}}^n\left( t \right)= & {} \sum \limits _{r = 1}^{n{{\bar{\varLambda }}} _b^n\left( t \right) } {{{{B_r}\left( p \right) } \big / n}}. \end{aligned}$$

(32)

$$\begin{aligned} {{\bar{\varLambda }}} _{RE}^n\left( t \right)= & {} \sum \limits _{r = 1}^{n{\bar{Q}}_A^n\left( t \right) } {{{{B_r}\left( q \right) } \big / n}}, \end{aligned}$$

(33)

where $\varLambda ^n\left( \cdot \right)$ is a Poisson process of $\lambda n$. Next, we define the martingales related to the stochastic process.

$$\begin{aligned} {{\bar{M}}}_Z^n\left( t \right)= & {} \left( {{{\bar{\varLambda }}} _a^n\left( t \right) - \int _0^t {\lambda {{\left( {1 - {p^b}\left( {{{\overline{\mathrm{Z}} }^n}\left( u \right) - s} \right) } \right) }^ + }du} } \right) \nonumber \\&- \left( {{{\bar{Q}}}_s^n\left( t \right) - \int _0^t {\mu \min \left\{ {s,{{{{\bar{Z}}}}^n}\left( u \right) } \right\} du} } \right) \nonumber \\&- \left( {{{\bar{Q}}}_a^n\left( t \right) - \int _0^t {\gamma '{{\left( {{{\overline{\mathrm{Z}} }^n}\left( u \right) - s} \right) }^{{ + }}}du} } \right) \nonumber \\&+ \left( {{{\bar{\varLambda }}} _{RE}^n\left( t \right) - \int _0^t {q\delta {{{{\bar{A}}}}^n}\left( u \right) {{\left( {1 - {p^b}\left( {{{\overline{\mathrm{Z}} }^n}\left( u \right) - s} \right) } \right) }^ + }du} } \right) \end{aligned}$$

(34)

$$\begin{aligned} {{\bar{M}}}_A^n\left( t \right)= & {} \left( {{{\bar{\varLambda }}} _A^n\left( t \right) - \int _0^t {\lambda {p^b}{{\left( {{{\bar{\mathrm{Z}}}^n}\left( u \right) - s} \right) }^{{ + }}}du} } \right) - \left( {{\bar{Q}}_A^n\left( t \right) - \int _0^t {\delta {{{{\bar{A}}}}^n}\left( u \right) du} } \right) . \end{aligned}$$

(35)

Based on the definition above from Eq. (34) and (35), the fluid scaled stochastic dynamic equations can be written as follows:

$$\begin{aligned} {\bar{\mathrm{Z}}^n}\left( t \right)= & {} {\bar{\mathrm{Z}}^n}\left( 0 \right) + {{\bar{M}}}_Z^n\left( t \right) + \int _0^t {{\beta _Z}\left( {{{{\bar{Z}}}^n}} \right) \left( u \right) du}, \end{aligned}$$

(36)

$$\begin{aligned} {{{\bar{A}}}^n}\left( t \right)= & {} {{{\bar{A}}}^n}\left( 0 \right) + {\bar{M}}_A^n\left( t \right) + \int _0^t {{\beta _A}\left( {{{{{\bar{A}}}}^n}} \right) \left( u \right) du}, \end{aligned}$$

(37)

Where

$$\begin{aligned}&\int _0^t {{\beta _Z}\left( {{{{{\bar{Z}}}}^n}} \right) \left( u \right) du} \nonumber \\&\quad = \int _0^t {\lambda \left( \begin{array}{l} 1 - {p^b}{\left( {{{{{\bar{Z}}}}^n}\left( u \right) - s} \right) ^ + } - \mu \min \left\{ {s,{{{{\bar{Z}}}}^n}\left( u \right) } \right\} - \gamma '{\left( {{{{{\bar{Z}}}}^n}\left( u \right) - s} \right) ^ + }\\ + q\delta {{\bar{\mathrm{A}}}^n}\left( u \right) \left( {1 - {p^b}{{\left( {{{{{\bar{Z}}}}^n}\left( u \right) - s} \right) }^ + }} \right) \end{array} \right) } du \end{aligned}$$

(38)

$$\begin{aligned}&\int _0^t {{\beta _{\mathrm{A}}}\left( {{{{{\bar{A}}}}^n}} \right) \left( u \right) du = \int _0^t {\lambda {p^b}{{\left( {{{{{\bar{Z}}}}^n}\left( u \right) - s} \right) }^ + } - \delta } } {\bar{\mathrm{A}}^n}\left( u \right) du, \end{aligned}$$

(39)

Proof of Lemma 1

To prove the C-tightness, we show that asymptotically the scaled processes $\left\{ {{{{{\bar{Z}}}}^n}\left( \cdot \right) } \right. \left. {,{{{{\bar{A}}}}^n}\left( \cdot \right) } \right\}$ live on a compact set and have small oscillations. In addition, for the convenience of notation, let ${{\bar{X}}}_j^n\left( \cdot \right)$ simply denote the sequence of stochastic processes $\left\{ {{{{\bar{Z}}}^n}\left( \cdot \right) ,{{{{\bar{A}}}}^n}\left( \cdot \right) } \right\}$, and $j \in \left\{ {Z,A} \right\}$.

$\bullet$ The compact containment condition: for any rational $t \ge 0$ and any $\varepsilon > 0$, there exists a compact set ${K_{\varepsilon ,t}} \subset S$ such that

$$\begin{aligned} {{\underline{\lim }} _{n \rightarrow \infty }}\mathrm{P}\left\{ {{\bar{X}}_j^n\left( t \right) \in {K_{\varepsilon ,t}}} \right\} \ge 1 - \varepsilon . \end{aligned}$$

(40)

$\bullet$ The oscillation control : for any $T > 0$ and $\varepsilon > 0$, there exists a $\eta > 0$ such that

$$\begin{aligned} {{\underline{\lim }} _{n \rightarrow \infty }}\mathrm{P}\left\{ {\omega \left( {{{\bar{X}}}_j^n,T,\eta } \right) \le \varepsilon } \right\} \ge 1 - \varepsilon . \end{aligned}$$

(41)

Where, for a function ${{\bar{X}}}_j^n\left( \cdot \right) \in D\left( {{R_ + },S} \right)$

$$\begin{aligned} \omega \left( {\bar{X}}_{j}^{n}, T, \eta \right) =\sup \left\{ \max _{j \in \{Z, A\}}\left| {\bar{X}}_{j}^{n}(t)-{\bar{X}}_{j}^{n}(v)\right| : \quad v, t \in [0, T], \quad |v-t|<\eta \right\} . \end{aligned}$$

(42)

First, the compact containment condition in Eq. (40) follows in easing by the upper bound, which holds for the total number of customers in the system, but only with arrivals.

$$\begin{aligned} {\bar{\mathrm{Z}}^n}\left( t \right) + {{{\bar{A}}}^n}\left( t \right) \le {\bar{\mathrm{Z}}^n}\left( 0 \right) + {{{\bar{A}}}^n}\left( 0 \right) + {{\varPi _{\lambda n}^n\left( T \right) } \big / n} \end{aligned}$$

$\varLambda ^n\left( \cdot \right)$ is a Poisson process of $\lambda n$, so we can obtain by the law of large number (LNN),

$$\begin{aligned} {{\varLambda ^n\left( T \right) } \big / n} \Rightarrow \lambda T, \end{aligned}$$

In addition, by Assumption 1, we can show that the compact containment property in Eq. (40) indeed holds: ${{\underline{\lim }} _{n \rightarrow \infty }}\mathrm{P}\left\{ {{\bar{X}}_j^n\left( t \right) \in {K_{\varepsilon ,t}}} \right\} \ge 1 - \varepsilon$, where ${K_{\varepsilon ,t}} = \left\{ {\left( {{x_1},{x_2}} \right) \left| {{x_1} + {x_2} \le z\left( 0 \right) + a\left( 0 \right) + \lambda T{{ + }}1,{x_1},{x_2} \ge 0} \right. } \right\}$.

Second, we establish the oscillation by Eqs. (16) and (17), since $q \le 1$, $\left( {1 - {p^b}\left( {{{{\bar{Z}}}^n}\left( t \right) - s} \right) } \right) \le 1$, then we have the following:

$$\begin{aligned}&\left| {{{{{\bar{Z}}}}^n}\left( t \right) - {{{{\bar{Z}}}}^n}\left( v \right) } \right| \le {{\left| {\varLambda ^n\left( t \right) - \varLambda ^n\left( v \right) } \right| } \big / n} + {{\sum \limits _{J \in \left\{ {s,a} \right\} } {\left| {{{\bar{Q}}}_J^n\left( t \right) - {\bar{Q}}_J^n\left( v \right) } \right| } } \big / n}. \\&\left| {{{{{\bar{A}}}}^n}\left( t \right) - {{{{\bar{A}}}}^n}\left( v \right) } \right| \le {{\left| {\varLambda ^n\left( t \right) - \varLambda ^n\left( v \right) } \right| } \big / n} + {{\left| {{{\bar{Q}}}_A^n\left( t \right) - {{\bar{Q}}}_A^n\left( v \right) } \right| } \big / n}. \end{aligned}$$

By applying the last two bounds together, we can give the following upper bound:

$$\begin{aligned} \omega \left( {{{\bar{X}}}_j^n,T,\eta } \right) \le {{\left| {\varLambda ^n\left( t \right) - \varLambda ^n\left( v \right) } \right| } \big / n} + {{\sum \limits _{J \in \left\{ {s,a,\mathrm{A}} \right\} } {\left| {{{\bar{Q}}}_J^n\left( t \right) - {\bar{Q}}_J^n\left( v \right) } \right| } } \big / n}. \end{aligned}$$

Based on the compact containment property, we can get a finite value such that

$$\begin{aligned} \left( {{{{{\bar{Z}}}}^n}\left( u \right) ,{{{{\bar{A}}}}^n}\left( u \right) } \right) \le K,\quad u \in \left[ {0,T} \right] , as\quad n \rightarrow \infty . \end{aligned}$$

In this case, each part of $\left( {{{{{\bar{Z}}}}^n}\left( t \right) ,{{{{\bar{A}}}}^n}\left( t \right) } \right)$ holds for $v,t \in \left[ {0,T} \right]$. Such that $\left| {t - v} \right| \le \eta$:

$$\begin{aligned}&\int _v^t {\mu \min \left\{ {s,{{\bar{\mathrm{Z}}}^n}\left( u \right) } \right\} du \le {r_1}\eta },\quad {r_1} = \mu s. \\&\int _v^t {\gamma '{{\left( {{{\bar{\mathrm{Z}}}^n}\left( u \right) - s} \right) }^{{ + }}}du \le {r_2}\eta },\quad {r_2} = \theta {\mathrm{K}}. \\&\int _v^t {\delta {{{{\bar{A}}}}^n}\left( u \right) du \le {r_3}\eta },\quad {r_3} = \delta {\mathrm{K}}. \end{aligned}$$

Thus, we can obtain

$$\begin{aligned}&\mathrm{P}\left( {\omega \left( {{{\bar{X}}}_j^n,T,\eta } \right) } \right) \\&\quad \ge \mathrm{P}\left( {\left\{ {{{\left| {\varLambda ^n\left( t \right) - \varLambda ^n\left( v \right) } \right| } \big / n} + {{\sum \limits _{J \in \left\{ {s,a,\mathrm{A}} \right\} } {\left| {{\bar{Q}}_J^n\left( t \right) - {{\bar{Q}}}_J^n\left( v \right) } \right| } } \big / n}} \right\} \le \varepsilon } \right) . \end{aligned}$$

Finally, we can show the oscillation control property holds which a $\eta > 0$ such that $\lambda \eta < {\varepsilon \big / 4}$, ${r_J}\eta < {\varepsilon \big / 4}$, where $J \in \left( {1,2,3} \right)$.

Proof of Theorem 1

Under Assumption 1, if Lemma 1 holds, we can obtain that the limit of any convergent subsequence satisfies Eqs. (16) and (17), then the key to the proof is a unique fluid limit defined by Eqs. (16) and (17).

Next, this step of the proof could be a general result like Theorem 1 in Ding et al. (2015b). First, by Lemma 1 in Reed and Ward (2004), we can show that the mapping $\left( {{\beta _Z}\left( {{{{{\bar{Z}}}}^n}} \right) \left( t \right) ,{\beta _A}\left( {{{{{\bar{A}}}}^n}} \right) \left( t \right) } \right)$ is Lipschitz continuous, then combine with defined Eqs. (36) and (37), we can show Eqs. (43) and (44) have a unique solution with an arbitrary n,

$$\begin{aligned} {\bar{\mathrm{Z}}^n}\left( t \right) - {\bar{\mathrm{Z}}^n}\left( 0 \right) - \int _0^t {{\beta _Z}\left( {{{{{\bar{Z}}}}^n}} \right) \left( u \right) du}= & {} 0, \end{aligned}$$

(43)

$$\begin{aligned} {{{\bar{A}}}^n}\left( t \right) - {{{\bar{A}}}^n}\left( 0 \right) - \int _0^t {{\beta _A}\left( {{{{{\bar{A}}}}^n}} \right) \left( u \right) du}= & {} 0, \end{aligned}$$

(44)

Second, we may obtain that $\left( {{{\bar{M}}}_Z^n\left( t \right) ,{\bar{M}}_A^n\left( t \right) } \right) \Rightarrow 0$ using LLN (see, for example Theorem 5.10 in Chen and Yao , 2001). That is, it can be shown that each term in ${{\bar{M}}}_Z^n\left( t \right)$ and ${\bar{M}}_A^n\left( t \right)$ converges to 0, such that $\left( {{\bar{M}}_Z^n\left( t \right) ,{{\bar{M}}}_A^n\left( t \right) } \right) \Rightarrow 0$. Therefore, Eqs. (16) and (17) have a unique solution.