Subsidy policies and operational strategies for multiple competing photovoltaic supply chains

Abstract

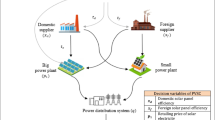
In the past decade, subsidy policies aimed at demand-side of photovoltaic (PV) supply chains have created a dilemma. While they foster the growth of the PV industry, they also induce overcapacity problems to the society. As a result, many governments have cut back subsidies to PV system users. These subsidy reductions hurt PV enterprises and their supply chains that are now facing lost business. To rescue enterprises, but not the market, an appropriate supply-side oriented subsidy policy is urgently needed. It is also important that different stakeholders on a PV supply chain develop closer collaborations to enhance their competitiveness against their rival PV supply chains. In this paper, we use game-theoretical models to investigate the impact of this new approach to policy design within the PV industry. Our findings suggest that the government should properly control the PV market entry, implement a balanced subsidy program and encourage a healthy competition among multiple PV supply chains to balance the operational performance of PV supply chains and the effects of government subsidy on the improvement of market out and social welfare. Under such a governance and subsidy program, a moderate combination of operational strategies will be the robust strategic response for multiple PV supply chains in a competitive environment.

Appendices

Appendix 1

Proofs for game-theoretical decision models for multiple competing pv supply chains with SWM

Based on the modeling notations and assumptions of Sect. 3, we formulated, analyzed and compared three game-theoretical decision models for multiple competing PV supply chains under the scenario with social welfare maximization (SWM): a Stackelberg-Cournot equilibrium decision model in Sect. 4.1, a bargaining-Cournot cooperative decision model in Sect. 4.2 and a hybrid decision model in Sect. 4.3. In the models to follow, note that the superscript or subscript e represents an equilibrium decision mode; the superscript or subscript c represents a cooperative decision mode; the superscript or subscript h represents a hybrid decision mode.

2.1 Equilibrium decision models under the scenario with SWM

In a Stackelberg-Cournot equilibrium decision mode (ED) with the government subsidy policy under the scenario with SWM, the key decision sequences are as follows: the government will first announce a subsidy factor of a PV system for every PA; then, the ith MS and the ith PA make optimal decisions in a Stackelberg way within the ith PV supply chain (von Stackelberg 1934), the ith MS chooses his optimal wholesale price ${w}_{i}$; finally, all the PAs choose their optimal ordering quantity ${q}_{i}$ under Cournot competition among the multiple PV supply chains.

In the ith decentralized PV supply chain, the optimal problem for the ith PA is formulated as follows:

$$\mathop {\max }\limits_{{q_{i} }} \;\Pi_{{PA_{i} }} \left( {\mathbf{q}} \right) = \left[ {p\left( {\mathbf{q}} \right) - c_{0i} - w_{i} + s} \right]q_{i}$$

(1)

Solving the first-order condition and the second-order derivative of the optimal problem with respect to (w.r.t.) the ordering quantity $q_{i}$ respectively, and solving the reaction function of ordering quantity $q_{i}$ w.r.t. the other ordering quantity $q_{ - i} = \left\{ {q_{1} , \ldots ,q_{i - 1} ,q_{i + 1} , \ldots ,q_{n} } \right\}$, we can obtain the equilibrium reaction function of ordering quantity $q_{i}$ w.r.t. the wholesale price ${\mathbf{w}} = \left[ {w_{1} ,w_{2} , \ldots ,w_{n} } \right]$ as follows:

$$q_{i}^{e} \left( {\mathbf{w}} \right) = \frac{{a + \sum\nolimits_{i = 1}^{n} {\left( {c_{0i} + w_{i} } \right)} }}{{b\left( {n + 1} \right)}} - \frac{{c_{0i} + w_{i} }}{b} + \frac{s}{{b\left( {n + 1} \right)}}$$

(2)

Plugging $q_{i}^{e} \left( {\varvec{w}} \right)$ into the profit function of the ith MS, we can get the optimal problem for the ith MS as follows:

$$\mathop {\max }\limits_{{w_{i} }} \;\Pi_{{MS_{i} }} \left( {\mathbf{w}} \right) = \left( {w_{i} - c_{i} } \right)q_{i}^{e} \left( {\mathbf{w}} \right)$$

(3)

Solving the first-order condition and the second-order derivative of the optimal problem w.r.t. the wholesale price ${w}_{i}$ respectively, and solving the reaction function of wholesale price ${w}_{i}$ w.r.t. the other ordering quantity $w_{ - i} = \left\{ {w_{1} , \ldots ,w_{i - 1} ,w_{i + 1} , \ldots ,w_{n} } \right\}$, we can obtain the equilibrium reaction function of wholesale price ${w}_{i}^{e}$ w.r.t. the government subsidy factor $s$ as follows:

$$w_{i}^{e} \left( s \right) = \frac{a}{n + 1} + \frac{{n\left( {c_{0i} + c_{i} } \right)}}{2n + 1} + \frac{{n\sum\nolimits_{i = 1}^{n} {\left( {c_{0i} + c_{i} } \right)} }}{{\left( {n + 1} \right)\left( {2n + 1} \right)}} - c_{0i} + \frac{s}{n + 1}$$

(4)

Plugging $w_{i}^{e} \left( s \right)$ into $q_{i} \left( {w_{i} } \right)$, we can get the equilibrium reaction function of ordering quantity $q_{i}^{e}$ w.r.t. the government subsidy factor $s$ as follows:

$$q_{i}^{e} \left( s \right) = \frac{na}{{b\left( {n + 1} \right)^{2} }} - \frac{{n\left( {c_{0i} + c_{i} } \right)}}{{b\left( {2n + 1} \right)}} + \frac{{n^{2} \sum\nolimits_{i = 1}^{n} {\left( {c_{0i} + c_{i} } \right)} }}{{b\left( {n + 1} \right)^{2} \left( {2n + 1} \right)}} + \frac{ns}{{b\left( {n + 1} \right)^{2} }}$$

(5)

Plugging ${q}_{i}^{e}\left(s\right)$ into the social welfare function $SW\left(\mathbf{q}\right)$, we can get the optimal problem for the social welfare of multiple competing PV supply chains as follows:

$$\mathop {\max }\limits_{s} \;SW\left( s \right) = \sum\nolimits_{i = 1}^{n} {\left[ {a - \left( {c_{0i} + c_{i} } \right)} \right]q_{i}^{e} \left( s \right)} - \frac{1}{2}b\left[ {\sum\nolimits_{i = 1}^{n} {q_{i}^{e} \left( s \right)} } \right]^{2}$$

(6)

Solving the first-order condition and the second-order derivative of the optimal problem w.r.t. the government subsidy factor $s$ respectively, we can obtain the optimal government subsidy factor ${s}_{e}$ as follows:

$$s_{e} = \frac{2n + 1}{{n^{3} }}\left[ {na - \sum\nolimits_{i = 1}^{n} {\left( {c_{0i} + c_{i} } \right)} } \right]$$

(7)

Hence, we can get the equilibrium wholesale price $w_{i}^{e}$, equilibrium ordering quantity $q_{i}^{e}$ and equilibrium retail price $p_{e}$ as follows:

$$w_{i}^{e} = \frac{n + 1}{{n^{2} }}a + \frac{n}{2n + 1}\left( {c_{0i} + c_{i} } \right) - c_{0i} + \frac{{\left( {n + 1} \right)\left[ {n^{2} - \left( {2n + 1} \right)} \right]}}{{n^{3} \left( {2n + 1} \right)}}\sum\nolimits_{i = 1}^{n} {\left( {c_{0i} + c_{i} } \right)}$$

(8)

$$q_{i}^{e} = \frac{a}{bn} - \frac{{n\left( {c_{0i} + c_{i} } \right)}}{{b\left( {2n + 1} \right)}} + \frac{{n^{2} - \left( {2n + 1} \right)}}{{bn^{2} \left( {2n + 1} \right)}}\sum\nolimits_{i = 1}^{n} {\left( {c_{0i} + c_{i} } \right)}$$

(9)

$$p_{e} = \frac{1}{n}\sum\nolimits_{i = 1}^{n} {\left( {c_{0i} + c_{i} } \right)}$$

(10)

Therefore, we can get the equilibrium profits of the ith PA, the ith MS and the ith PV supply chain as follows:

$$\Pi_{{PA_{i} }}^{e} = b\left( {q_{i}^{e} } \right)^{2}$$

(11)

$$\Pi_{{MS_{i} }}^{e} = \frac{n + 1}{n}b\left( {q_{i}^{e} } \right)^{2}$$

(12)

$$\Pi_{{SC_{i} }}^{e} = \frac{2n + 1}{n}b\left( {q_{i}^{e} } \right)^{2}$$

(13)

Furthermore, we can obtain the corresponding social welfare, consumer surplus and total government subsidy as follows:

$$SW_{e} = \sum\nolimits_{i = 1}^{n} {\left[ {a - \left( {c_{0i} + c_{i} } \right)} \right]q_{i}^{e} } - \frac{1}{2}b\left( {\sum\nolimits_{i = 1}^{n} {q_{i}^{e} } } \right)^{2}$$

(14)

$$CS_{e} = \frac{1}{2}b\left( {\sum\nolimits_{i = 1}^{n} {q_{i}^{e} } } \right)^{2}$$

(15)

$$TS_{e} = \sum\nolimits_{i = 1}^{n} {s_{e} q_{i}^{e} }$$

(16)

2.2 Cooperative decision models under the scenario with SWM

In a bargaining-Cournot cooperative decision mode (CD) with the government subsidy policy under the scenario with SWM, the key decision sequences are as follows: the government will first announce a subsidy factor of a PV system for every PA; then, the ith MS and the ith PA make optimal decisions in a Nash-bargaining cooperation way within the ith PV supply chain (Nash 1950), i.e., the ith MS and the ith PA bargain over the wholesale price${w}_{i}$; finally, all the PAs choose their optimal ordering quantities ${\mathbf{q}} = \left[ {q_{1} ,q_{2} , \ldots ,q_{n} } \right]$ under Cournot competition among the multiple PV supply chains.

In the ith centralized PV supply chain, the optimal problem is formulated as follows:

$$\mathop {\max }\limits_{{q_{i} }} \;\Pi_{{SC_{i} }} \left( {\mathbf{q}} \right) = \left[ {p\left( {\mathbf{q}} \right) - c_{0i} - c_{i} + s} \right]q_{i}$$

(17)

Solving the first-order condition and the second-order derivative of the optimal problem w.r.t. the ordering quantity $q_{i}$ respectively, and solving the reaction function of ordering quantity $q_{i}$ w.r.t. the other ordering quantity $q_{ - i} = \left\{ {q_{1} , \ldots ,q_{i - 1} ,q_{i + 1} , \ldots ,q_{n} } \right\}$, we can obtain the optimal reaction function of ordering quantity ${q}_{i}^{c}$ w.r.t. the government subsidy factor $s$ as follows:

$$q_{i}^{c} \left( s \right) = \frac{{a + \sum\nolimits_{i = 1}^{n} {\left( {c_{0i} + c_{i} } \right)} }}{{b\left( {n + 1} \right)}} - \frac{{c_{0i} + c_{i} }}{b} + \frac{s}{{b\left( {n + 1} \right)}}$$

(18)

Plugging ${q}_{i}^{c}\left(s\right)$ into the profit functions of the ith PA, the ith MS and the ith PV supply chain, we can get the profit reaction functions of the ith PA, the ith MS and the ith PV supply chain w.r.t. the government subsidy factor $s$ as follows:

$$\Pi_{{PA_{i} }}^{c} \left( {w_{i} ,s} \right) = b\left[ {q_{i}^{c} \left( s \right)} \right]^{2} - \left( {w_{i} - c_{i} } \right)q_{i}^{c} \left( s \right)$$

(19)

$$\Pi_{{MS_{i} }}^{c} \left( {w_{i} ,s} \right) = \left( {w_{i} - c_{i} } \right)q_{i}^{c} \left( s \right)$$

(20)

$$\Pi_{{SC_{i} }}^{c} \left( s \right) = b\left[ {q_{i}^{c} \left( s \right)} \right]^{2}$$

(21)

On this basis, the asymmetric Nash bargaining problem for bargaining over the wholesale price $w_{i}$ can be formulated as follows:

$$\begin{gathered} \mathop {\max }\limits_{{w_{i} }} \;\;\theta \left( {w_{i} } \right) = \left[ {\Pi_{{MS_{i} }}^{c} \left( {w_{i} ,s} \right)} \right]^{\tau } \left[ {\Pi_{{PA_{i} }}^{c} \left( {w_{i} ,s} \right)} \right]^{1 - \tau } \hfill \\ s.t.\;\left\{ \begin{gathered} \Pi_{{MS_{i} }}^{c} \left( {w_{i} ,s} \right) + \Pi_{{PA_{i} }}^{c} \left( {w_{i} ,s} \right) = \Pi_{{SC_{i} }}^{c} \left( s \right) \hfill \\ w_{i} > c_{i} \hfill \\ \end{gathered} \right. \hfill \\ \end{gathered}$$

(22)

Solving the first-order condition and the second-order derivative of the optimal problem w.r.t. the wholesale price $w_{i}$ respectively, we can obtain the reaction function of wholesale price $w_{i}^{c}$ w.r.t. the government subsidy factor $s$ as follows:

$$w_{i}^{c} \left( s \right) = c_{i} + \tau bq_{i}^{c} \left( s \right)$$

(23)

Plugging $q_{i}^{c} \left( s \right)$ into the social welfare function $SW\left(\mathbf{q}\right)$, we can get the optimal problem for the social welfare of multiple competing PV supply chains as follows:

$$\mathop {\max }\limits_{s} \;SW\left( s \right) = \sum\nolimits_{i = 1}^{n} {\left[ {a - \left( {c_{0i} + c_{i} } \right)} \right]q_{i}^{c} \left( s \right)} - \frac{1}{2}b\left[ {\sum\nolimits_{i = 1}^{n} {q_{i}^{c} \left( s \right)} } \right]^{2}$$

(24)

Solving the first-order condition and the second-order derivative of the optimal problem w.r.t. the government subsidy factor $s$ respectively, we can obtain the optimal government subsidy factor $s_{c}$ as follows:

$$s_{c} = \frac{1}{{n^{2} }}\left[ {na - \sum\nolimits_{i = 1}^{n} {\left( {c_{0i} + c_{i} } \right)} } \right]$$

(25)

Hence, we can get the bargaining wholesale price $w_{i}^{c}$, optimal ordering quantity $q_{i}^{c}$ and optimal retail price $p_{c}$ as follows:

$$w_{i}^{c} = c_{i} + \tau bq_{i}^{c}$$

(26)

$$q_{i}^{c} = \frac{a}{bn} - \frac{{c_{0i} + c_{i} }}{b} + \frac{n - 1}{{bn^{2} }}\sum\nolimits_{i = 1}^{n} {\left( {c_{0i} + c_{i} } \right)}$$

(27)

$$p_{c} = \frac{1}{n}\sum\nolimits_{i = 1}^{n} {\left( {c_{0i} + c_{i} } \right)}$$

(28)

Therefore, we can get the optimal(bargaining) profits of the ith PA, the ith MS and the ith PV supply chain as follows:

$$\Pi_{{PA_{i} }}^{c} = \left( {1 - \tau } \right)\Pi_{{SC_{i} }}^{c}$$

(29)

$$\Pi_{{MS_{i} }}^{c} = \tau \Pi_{{SC_{i} }}^{c}$$

(30)

$$\Pi_{{SC_{i} }}^{c} = b\left( {q_{i}^{c} } \right)^{2}$$

(31)

Furthermore, we can obtain the corresponding social welfare, consumer surplus and total government subsidy as follows:

$$SW_{c} = \sum\nolimits_{i = 1}^{n} {\left[ {a - \left( {c_{0i} + c_{i} } \right)} \right]q_{i}^{c} } - \frac{1}{2}b\left( {\sum\nolimits_{i = 1}^{n} {q_{i}^{c} } } \right)^{2}$$

(32)

$$CS_{c} = \frac{1}{2}b\left( {\sum\nolimits_{i = 1}^{n} {q_{i}^{c} } } \right)^{2}$$

(33)

$$TS_{c} = \sum\nolimits_{i = 1}^{n} {s_{c} q_{i}^{c} }$$

(34)

2.3 Hybrid decision models under the scenario with SWM

In a hybrid decision mode (CD) with the government subsidy policy under the scenario with SWM, it is assumed that there are $m$ PV supply chains adopting CD mode ($l = 1,2, \ldots ,m$) and $\left( {n - m} \right)$ PV supply chains adopt ED mode ($k = m + 1,m + 2, \ldots ,n$),$0 \le m \le n$. The key decision sequences are as follows: the government will first announce a subsidy factor of a PV system for every PA; then, the lth MS and the lth PA make optimal decisions in a Nash-bargaining cooperation way within the lth PV supply chain, i.e., the lth MS and the lth PA bargain over the wholesale price${w}_{l}$, and at the same time, the kth MS and the kth PA make optimal decisions in a Stackelberg way within the kth PV supply chain, the k^th MS chooses his optimal wholesale price${w}_{k}$; finally, all the PAs choose their optimal ordering quantities ${\mathbf{q}}_{m} = \left[ {q_{1} ,q_{2} , \ldots ,q_{m} } \right]$ and ${\mathbf{q}}_{n - m} = \left[ {q_{m + 1} ,q_{m + 2} , \ldots ,q_{n} } \right]$ under Cournot competition among the multiple PV supply chains.

For the $m$ PV supply chains adopting cooperative decision mode, the optimal problem for the l^th PV supply chain is formulated as follows:

$$\mathop {\max }\limits_{{q_{l} }} \;\Pi_{{SC_{l} }} \left( {{\mathbf{q}}_{m} ,{\mathbf{q}}_{n - m} } \right) = \left[ {p\left( {{\mathbf{q}}_{m} ,{\mathbf{q}}_{n - m} } \right) - c_{0l} - c_{l} + s} \right]q_{l}$$

(35)

Solving the first-order condition and the second-order derivative of the optimal problem w.r.t. the ordering quantity ${q}_{l}$ respectively, and solving the reaction function of ordering quantity ${q}_{l}$ w.r.t. the other ordering quantity $q_{ - l} = \left\{ {q_{1} , \ldots ,q_{l - 1} ,q_{l + 1} , \ldots ,q_{m} } \right\}$, we can obtain the reaction function of the ordering quantity $q_{l}^{hc}$ w.r.t. the ordering quantities ${\mathbf{q}}_{n - m}$ as follows:

$$q_{l}^{hc} \left( {{\mathbf{q}}_{n - m} } \right) = \frac{{a - b\sum\nolimits_{k = m + 1}^{n} {q_{k} } + \sum\nolimits_{i = 1}^{m} {\left( {c_{0l} + c_{l} } \right)} }}{{b\left( {m + 1} \right)}} - \frac{{c_{0l} + c_{l} }}{b} + \frac{s}{{b\left( {m + 1} \right)}}$$

(36)

For the $\left( {n - m} \right)$ PV supply chains adopting equilibrium decision mode, the optimal problem for the k^th PA is formulated as follows:

$$\mathop {\max }\limits_{{q_{k} }} \;\Pi_{{PA_{k} }} \left( {{\mathbf{q}}_{m} ,{\mathbf{q}}_{n - m} } \right) = \left[ {p\left( {{\mathbf{q}}_{m} ,{\mathbf{q}}_{n - m} } \right) - c_{0k} - w_{k} + s} \right]q_{k}$$

(37)

Solving the first-order condition and the second-order derivative of the optimal problem w.r.t. the ordering quantity ${q}_{k}$ respectively, and solving the reaction function of ordering quantity ${q}_{k}$ w.r.t. the other ordering quantity $q_{ - k} = \left\{ {q_{m + 1} , \ldots ,q_{k - 1} ,q_{k + 1} , \ldots ,q_{n} } \right\}$, we can obtain the reaction function of the ordering quantity $q_{k}^{he}$ w.r.t. the ordering quantities ${\mathbf{q}}_{m}$ as follows:

$$q_{k}^{he} \left( {{\mathbf{q}}_{m} } \right) = \frac{{a - b\sum\nolimits_{l = 1}^{m} {q_{l} } + \sum\nolimits_{k = m + 1}^{n} {\left( {c_{0k} + w_{k} } \right)} }}{{b\left( {n - m + 1} \right)}} - \frac{{c_{0k} + w_{k} }}{b} + \frac{s}{{b\left( {n - m + 1} \right)}}$$

(38)

Solving $q_{l}^{hc} \left( {{\mathbf{q}}_{n - m} } \right)$ and $q_{k}^{he} \left( {{\mathbf{q}}_{m} } \right)$ simultaneously, we can obtain the reaction function of the ordering quantity $q_{l}^{hc}$ and $q_{k}^{he}$ w.r.t. the wholesale prices ${\mathbf{w}}_{n-m}$ as follows:

$$q_{l}^{hc} \left( {{\mathbf{w}}_{n - m} } \right) = \frac{1}{{b\left( {n + 1} \right)}}\left[ {a + \sum\nolimits_{l = 1}^{m} {\left( {c_{0l} + c_{l} } \right)} + \sum\nolimits_{k = m + 1}^{n} {\left( {c_{0k} + w_{k} } \right)} - \left( {n + 1} \right)\left( {c_{0l} + c_{l} } \right) + s} \right]$$

(39)

$$q_{k}^{he} \left( {{\mathbf{w}}_{n - m} } \right) = \frac{1}{{b\left( {n + 1} \right)}}\left[ {a + \sum\nolimits_{l = 1}^{m} {\left( {c_{0l} + c_{l} } \right)} + \sum\nolimits_{k = m + 1}^{n} {\left( {c_{0k} + w_{k} } \right)} - \left( {n + 1} \right)\left( {c_{0k} + w_{k} } \right) + s} \right]$$

(40)

where ${\mathbf{w}}_{n - m} = \left[ {w_{m + 1} ,w_{m + 2} , \ldots ,w_{n} } \right]$.

Plugging $q_{k}^{he} \left( {{\mathbf{w}}_{n - m} } \right)$ into the profit function of the k^th MS, we can get the optimal problem for the k^th MS as follows:

$$\mathop {\max }\limits_{{w_{k} }} \;\Pi_{{MS_{k} }} \left( {{\mathbf{w}}_{n - m} } \right) = \left( {w_{k} - c_{k} } \right)q_{k}^{he} \left( {{\mathbf{w}}_{n - m} } \right)$$

(41)

Solving the first-order condition and the second-order derivative of the optimal problem w.r.t. the wholesale price $w_{k}$ respectively, we can obtain the reaction function of the equilibrium wholesale price $w_{k}^{he}$ w.r.t. the government subsidy factor $s$ as follows:

$$\begin{gathered} w_{k}^{he} \left( s \right) = \frac{1}{n + m + 1}\left[ {a + \sum\nolimits_{l = 1}^{m} {\left( {c_{0l} + c_{l} } \right)} + \frac{n}{2n + 1}\sum\nolimits_{k = m + 1}^{n} {\left( {c_{0k} + c_{k} } \right)} } \right] \\ + \frac{1}{2n + 1}\left[ {nc_{k} - \left( {n + 1} \right)c_{0k} } \right] + \frac{s}{n + m + 1} \\ \end{gathered}$$

(42)

Plugging $w_{k}^{he} \left( s \right)$ into $q_{l}^{hc} \left( {w_{k} } \right)$ and $q_{k}^{he} \left( {w_{k} } \right)$ respectively, we can obtain the reaction functions of the optimal ordering quantity $q_{l}^{hc}$ and the equilibrium ordering quantity $q_{k}^{he}$ w.r.t. the government subsidy factor $s$ as follows:

$$\begin{gathered} q_{l}^{hc} \left( s \right) = \frac{2n + 1}{{b\left( {n + 1} \right)\left( {n + m + 1} \right)}}\left[ {a + \sum\nolimits_{l = 1}^{m} {\left( {c_{0l} + c_{l} } \right)} + \frac{n}{2n + 1}\sum\nolimits_{k = m + 1}^{n} {\left( {c_{0k} + c_{k} } \right)} } \right] \\ - \frac{1}{b}\left( {c_{0l} + c_{l} } \right) + \frac{{\left( {2n + 1} \right)s}}{{b\left( {n + 1} \right)\left( {n + m + 1} \right)}} \\ \end{gathered}$$

(43)

$$\begin{gathered} q_{k}^{he} \left( s \right) = \frac{n}{{b\left( {n + 1} \right)\left( {n + m + 1} \right)}}\left[ {a + \sum\nolimits_{l = 1}^{m} {\left( {c_{0l} + c_{l} } \right)} + \frac{n}{2n + 1}\sum\nolimits_{k = m + 1}^{n} {\left( {c_{0k} + c_{k} } \right)} } \right] \\ - \frac{{n\left( {c_{0k} + c_{k} } \right)}}{{b\left( {2n + 1} \right)}} + \frac{ns}{{b\left( {n + 1} \right)\left( {n + m + 1} \right)}} \\ \end{gathered}$$

(44)

Plugging $q_{l}^{hc} \left( s \right)$ into the profit functions of the lth PA, the lth MS and the lth PV supply chain, we can get the profit reaction functions of the lth PA, the lth MS and the lth PV supply chain w.r.t. the government subsidy factor $s$ as follows:

$$\Pi_{{PA_{l} }}^{hc} \left( {w_{l} ,s} \right) = b\left[ {q_{l}^{hc} \left( s \right)} \right]^{2} - \left( {w_{l} - c_{l} } \right)q_{l}^{hc} \left( s \right)$$

(45)

$$\Pi_{{MS_{l} }}^{hc} \left( {w_{l} ,s} \right) = \left( {w_{l} - c_{l} } \right)q_{l}^{hc} \left( s \right)$$

(46)

$$\Pi_{{SC_{l} }}^{hc} \left( s \right) = b\left[ {q_{l}^{hc} \left( s \right)} \right]^{2}$$

(47)

Likewise, the asymmetric Nash bargaining problem for bargaining over the wholesale price ${w}_{l}$ can be formulated as follows:

$$\begin{gathered} \mathop {\max }\limits_{{w_{l} }} \;\;\theta \left( {w_{l} } \right) = \left[ {\Pi_{{MS_{l} }}^{hc} \left( {w_{l} ,s} \right)} \right]^{\tau } \left[ {\Pi_{{PA_{l} }}^{hc} \left( {w_{l} ,s} \right)} \right]^{1 - \tau } \hfill \\ s.t.\;\left\{ \begin{gathered} \Pi_{{MS_{l} }}^{hc} \left( {w_{l} ,s} \right) + \Pi_{{PA_{l} }}^{hc} \left( {w_{l} ,s} \right) = \Pi_{{SC_{l} }}^{hc} \left( s \right) \hfill \\ w_{l} > c_{l} \hfill \\ \end{gathered} \right. \hfill \\ \end{gathered}$$

(48)

Solving the first-order condition and the second-order derivative of the optimal problem w.r.t. the wholesale price $w_{l}$ respectively, we can obtain the reaction function of wholesale price $w_{l}^{hc}$ w.r.t. the government subsidy factor $s$ as follows:

$$w_{l}^{hc} \left( s \right) = c_{l} + \tau bq_{l}^{hc} \left( s \right)$$

(49)

Plugging $q_{l}^{hc} \left( s \right)$ and $q_{k}^{he} \left( s \right)$ into the social welfare function $SW\left( {\varvec{q}} \right)$, we can get the optimal problem for the social welfare of multiple competing PV supply chains as follows:

$$\begin{gathered} \mathop {\max }\limits_{s} \;SW\left( s \right) = \sum\nolimits_{l = 1}^{m} {\left[ {a - \left( {c_{0l} + c_{l} } \right)} \right]q_{l}^{hc} \left( s \right)} + \sum\nolimits_{k = m + 1}^{n} {\left[ {a - \left( {c_{0k} + c_{k} } \right)} \right]q_{k}^{he} \left( s \right)} \\ - \frac{1}{2}b\left[ {\sum\nolimits_{l = 1}^{m} {q_{l}^{hc} \left( s \right)} + \sum\nolimits_{k = m + 1}^{n} {q_{k}^{he} \left( s \right)} } \right]^{2} \\ \end{gathered}$$

(50)

Solving the first-order condition and the second-order derivative of the optimal problem w.r.t. the government subsidy factor $s$ respectively, we can obtain the optimal government subsidy factor $s_{h}$ as follows:

$$s_{h} = \frac{{\left( {2n + 1} \right)\left\{ {\left[ {n^{2} + \left( {n + 1} \right)m} \right]a - \left[ {\left( {2n + 1} \right)\sum\nolimits_{l = 1}^{m} {\left( {c_{0l} + c_{l} } \right)} + n\sum\nolimits_{k = m + 1}^{n} {\left( {c_{0k} + c_{k} } \right)} } \right]} \right\}}}{{\left[ {n^{2} + \left( {n + 1} \right)m} \right]^{2} }}$$

(51)

Hence, we can get the bargaining wholesale price $w_{l}^{hc}$, equilibrium wholesale price $w_{k}^{he}$, optimal ordering quantity $q_{l}^{hc}$, equilibrium ordering quantity $q_{k}^{he}$ and optimal retail price $p_{h}$ as follows:

$$w_{l}^{hc} = c_{l} + \tau bq_{l}^{hc}$$

(52)

$$\begin{aligned} w_{k}^{{he}} & = \frac{{\left( {n + 1} \right)a}}{{n^{2} + \left( {n + 1} \right)m}} + \frac{{n\left( {c_{k} + c_{{0k}} } \right)}}{{2n + 1}} - c_{{0k}} \\ & \quad + \frac{{\left( {n + 1} \right)\left\{ {\left[ {n^{2} + \left( {n + 1} \right)m} \right] - \left( {2n + 1} \right)} \right\}}}{{\left( {2n + 1} \right)\left[ {n^{2} + \left( {n + 1} \right)m} \right]^{2} }}\left[ {\left( {2n + 1} \right)\sum\nolimits_{{l = 1}}^{m} {\left( {c_{{0l}} + c_{l} } \right)} + n\sum\nolimits_{{k = m + 1}}^{n} {\left( {c_{{0k}} + c_{k} } \right)} } \right] \\ \end{aligned}$$

(53)

$$\begin{aligned} q_{l}^{{hc}} & = \frac{{\left( {2n + 1} \right)a}}{{b\left[ {n^{2} + \left( {n + 1} \right)m} \right]}} - \frac{1}{b}\left( {c_{{0l}} + c_{l} } \right) \\ & \quad + \frac{{\left[ {n^{2} + \left( {n + 1} \right)m} \right] - \left( {2n + 1} \right)}}{{b\left[ {n^{2} + \left( {n + 1} \right)m} \right]^{2} }}\left[ {\left( {2n + 1} \right)\sum\nolimits_{{l = 1}}^{m} {\left( {c_{{0l}} + c_{l} } \right)} + n\sum\nolimits_{{k = m + 1}}^{n} {\left( {c_{{0k}} + c_{k} } \right)} } \right] \\ \end{aligned}$$

(54)

$$\begin{aligned} q_{k}^{{he}} & = \frac{{na}}{{b\left[ {n^{2} + \left( {n + 1} \right)m} \right]}} - \frac{n}{{b\left( {2n + 1} \right)}}\left( {c_{{0k}} + c_{k} } \right) \\ & \quad + \frac{{n\left\{ {\left[ {n^{2} + \left( {n + 1} \right)m} \right] - \left( {2n + 1} \right)} \right\}}}{{b\left( {2n + 1} \right)\left[ {n^{2} + \left( {n + 1} \right)m} \right]^{2} }}\left[ {\left( {2n + 1} \right)\sum\nolimits_{{l = 1}}^{m} {\left( {c_{{0l}} + c_{l} } \right)} + n\sum\nolimits_{{k = m + 1}}^{n} {\left( {c_{{0k}} + c_{k} } \right)} } \right] \\ \end{aligned}$$

(55)

$$p_{h} = \frac{1}{{n^{2} + \left( {n + 1} \right)m}}\left[ {\left( {2n + 1} \right)\sum\nolimits_{l = 1}^{m} {\left( {c_{0l} + c_{l} } \right)} + n\sum\nolimits_{k = m + 1}^{n} {\left( {c_{0k} + c_{k} } \right)} } \right]$$

(56)

Therefore, we can get the optimal(bargaining) profits of the lth PA, the lth MS and the lth PV supply chain as follows:

$$\Pi_{{PA_{l} }}^{hc} = \left( {1 - \tau } \right)\Pi_{{SC_{l} }}^{hc}$$

(57)

$$\Pi_{{MS_{l} }}^{hc} = \tau \Pi_{{SC_{l} }}^{hc}$$

(58)

$$\Pi_{{SC_{l} }}^{hc} = b\left( {q_{l}^{hc} } \right)^{2}$$

(59)

Besides, we can get the equilibrium profits of the kth PA, the kth MS and the kth PV supply chain as follows:

$$\Pi_{{PA_{k} }}^{he} = b\left( {q_{k}^{he} } \right)^{2}$$

(60)

$$\Pi_{{MS_{k} }}^{he} = \frac{n + 1}{n}b\left( {q_{k}^{he} } \right)^{2}$$

(61)

$$\Pi_{{SC_{k} }}^{he} = \frac{2n + 1}{n}b\left( {q_{k}^{he} } \right)^{2}$$

(62)

Furthermore, we can obtain the corresponding social welfare, consumer surplus and total government subsidy as follows:

$$\begin{gathered} SW_{h} = \sum\nolimits_{l = 1}^{m} {\left[ {a - \left( {c_{0l} + c_{l} } \right)} \right]q_{l}^{hc} } + \sum\nolimits_{k = m + 1}^{n} {\left[ {a - \left( {c_{0k} + c_{k} } \right)} \right]q_{k}^{he} } \\ - \frac{1}{2}b\left( {\sum\nolimits_{l = 1}^{m} {q_{l}^{hc} } + \sum\nolimits_{k = m + 1}^{n} {q_{k}^{he} } } \right)^{2} \\ \end{gathered}$$

(63)

$$CS_{h} = \frac{1}{2}b\left( {\sum\nolimits_{l = 1}^{m} {q_{l}^{hc} } + \sum\nolimits_{k = m + 1}^{n} {q_{k}^{he} } } \right)^{2}$$

(64)

$$TS_{h} = \sum\nolimits_{l = 1}^{m} {s_{h} q_{l}^{hc} } + \sum\nolimits_{k = m + 1}^{n} {s_{h} q_{k}^{he} }$$

(65)

Besides, based on the previous research (Chen and Su 2018), the analytical results of the equilibrium, cooperative and hybrid decision models for multiple competing PV supply chains under the scenario without SWM (i.e., $s = 0$) are summarized and presented in Table 5 in the "Appendix" part for comparison purpose.

Comparing the key outcomes under the scenario with SWM with those under the scenario without SWM, the total profit difference and social welfare difference under three modes can be calculated as follows:

$$\Delta \Pi_{SC}^{e} = \Pi_{SC}^{e} - \Pi_{SC}^{e^{\prime}}$$

(66)

$$\Delta \Pi_{SC}^{c} = \Pi_{SC}^{c} - \Pi_{SC}^{c^{\prime}}$$

(67)

$$\Delta \Pi_{SC}^{h} = \Pi_{SC}^{h} - \Pi_{SC}^{h^{\prime}}$$

(68)

$$\Delta SW_{e} = SW_{e} - SW_{e}^{^{\prime}}$$

(69)

$$\Delta SW_{c} = SW_{c} - SW_{c}^{^{\prime}}$$

(70)

$$\Delta SW_{h} = SW_{h} - SW_{h}^{^{\prime}}$$

(71)

On this basis, the market return improvement on government subsidy (MIOS) and welfare return improvement on government subsidy (WIOS) under three scenarios can be calculated as follows:

$$MIOS_{e} = \frac{{\Delta \Pi_{SC}^{e} }}{{TS_{e} }}$$

(72)

$$MIOS_{c} = \frac{{\Delta \Pi_{SC}^{c} }}{{TS_{c} }}$$

(73)

$$MIOS_{h} = \frac{{\Delta \Pi_{SC}^{h} }}{{TS_{h} }}$$

(74)

$$WIOS_{e} = \frac{{\Delta SW_{e} }}{{TS_{e} }}$$

(75)

$$WIOS_{c} = \frac{{\Delta SW_{c} }}{{TS_{c} }}$$

(76)

$$WIOS_{h} = \frac{{\Delta SW_{h} }}{{TS_{h} }}$$

(77)

Appendix 2

See Figs. 4 and 5, Tables 5, 6 and 7.

Fig. 4

Demand-side oriented subsidy decline effect

Fig. 5

Sensitivity analysis results of the number of all PV supply chains

Table 5 Analytical results of three game-theoretical decision models for multiple competing PV supply chains without SWM

Table 6 Demand-side oriented subsidy decline effect

Table 7 Numerical analysis results of five competing PV supply chains without SWM (n = 5; m = 0, 2, 5)