A two-fluid arrangement with bounded van der Waals body forces. Part 1. The Young-Laplace equilibria. Differences from comparable gravity systems

Abstract

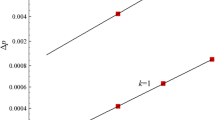
We study a conceivable two-fluid system with a layer of one fluid between a solid support and a semi-infinite layer of the other fluid in which the molecular van der Waals (vdW) forces act like gravity in well-studied cases with similar spatial arrangements. The solid support consists of two layers so that vdW forces are, similar to the gravity systems (GS), finite at the solid–fluid interface. In this Part 1, we focus on the Young–Laplace (YL) equilibrium drops of the “light” liquid with a zero angle between the solid–fluid and fluid–fluid interfaces and compare them with the YL drops in the analogous GS. We point out that there is essentially just a single GS, in contrast to a continuum of vdW systems corresponding to different values of a dimensionless Hamaker constant (DHC). We find that when the latter is above a certain threshold value, the YL drop volume cannot exceed a certain terminal value, just as it is known to be the case for GS. In contrast, in vdW systems with the smaller DHC, the YL drops may have arbitrarily large volumes, which is a major difference from GS. We introduce a certain “non-classical particle” kinetic energy and a corresponding generalized phase plane. These “energy” considerations predict interfaces with vertical tangents, which are in an excellent agreement with equilibrium drop interfaces found by numerical continuation methods. For small DHC, numerical simulations on a neutral-wave-long interval confirm the lubrication-approximation evolutions from initially flat films toward the—perpetually approached—equilibrium YL drops of the same volume. We point out that the evolution for larger DHC may be approached by the boundary integral methods suitable for the Stokes flows, which we will further investigate in the future Part 2 of this work.

Appendix A: Relation between energy and area

In view of Eqs. (54), (52) and (53) (with $x=x(h,m)$ and the subscripts h and m indicating the corresponding partial derivatives), the total energy is written as

$$\begin{aligned} E_{v}=-l+\int _{0}^{m}x(h)A_{0}(1+h)^{-3}\mathrm{d}h+\int _{0}^{m}(1+x_{h}^{2})^{1/2}\mathrm{d}h, \end{aligned}$$

(55)

where $m=h_{M}$. We have also used the expression

$$\begin{aligned} l_{s}=\int _{0}^{m}s_{h}\mathrm{d}h=\int _{0}^{m}(1+x_{h}^{2})^{1/2}\mathrm{d}h, \end{aligned}$$

where s(h) is the interfacial arc length, with $s(0)=0$. Differentiating $E_{v}$ with respect to m yields

$$\begin{aligned} \frac{\mathrm{d}}{\mathrm{d}m}E_{v}=-l_{m}+\int _{0}^{m}x_{m}A_{0}(1+h)^{-3}\mathrm{d}h+\lim _{n\rightarrow 0}\frac{\mathrm{d}}{\mathrm{d}m}\int _{0}^{m-n}(1+x_{h}^{2})^{1/2}\mathrm{d}h, \end{aligned}$$

(56)

where for the second term in the RHS, we have used Leibniz rule and the BC $x(m,m)=0$., and we have written the last term in the form of a limit since $x_{h}\rightarrow -\infty$ when $h\rightarrow m$ (and also when $h\rightarrow 0$). However, we will show that the singularities cancel and the limit in (56) turns out to be finite. With the Leibniz rule, the derivative in the last term is

$$\begin{aligned} \frac{\mathrm{d}}{\mathrm{d}m}\int _{0}^{m-n}(1+x_{h}^{2})^{1/2}\mathrm{d}h=[(1+x_{h}^{2})^{1/2}]_{h=m-n}+\int _{0}^{m-n}\frac{\partial }{\partial m}[(1+x_{h}^{2})^{1/2}]\mathrm{d}h, \end{aligned}$$

where in the first term of RHS, to leading order, $(1+x_{h}^{2})^{1/2}=-x_{h}$ when $n\rightarrow 0$, and in the second term of the RHS, the integrand $\frac{\partial }{\partial m}[(1+x_{h}^{2})^{1/2}]=(1+x_{h}^{2})^{-1/2}x_{h}x_{hm}$, so that we can integrate by parts:

$$\begin{aligned} \int _{0}^{m-n}\frac{\partial }{\partial m}[(1+x_{h}^{2})^{1/2}]\mathrm{d}h=[x_{h}x_{m}(1+x_{h}^{2})^{-1/2}]_{h=0}^{m-n}-\int _{0}^{m-n}x_{m}[x_{h}(1+x_{h}^{2})^{-1/2}]_{h}\mathrm{d}h. \end{aligned}$$

Note that $x_{h}(1+x_{h}^{2})^{-1/2}=-1$ to leading order, both for $h\rightarrow m$ and $h\rightarrow 0$. Hence, the integrated part is $[x_{h}x_{m}(1+x_{h}^{2})^{-1/2}]_{h=0}^{m-n}=-x_{m}(m-n,m)+l_{m},$ where we used the BC $x(0,m)=l(m)$. Also note that

$$\begin{aligned} {[}x_{h}(1+x_{h}^{2})^{-1/2}]_{h}=\frac{x_{hh}}{(1+x_{h}^{2})^{3/2}}=A_{0}(1+h)^{-3}-p, \end{aligned}$$

where we have used the YL equation. As a result, there is cancellation of terms with l and $A_{0}$ in (56), yielding

$$\begin{aligned} \frac{\mathrm{d}}{\mathrm{d}m}E_{v}=\lim _{n\rightarrow 0}([-x_{h}-x_{m}]_{h=m-n})+\int _{0}^{m}px_{m}\mathrm{d}h. \end{aligned}$$

(57)

In view of the expression for the area, $\alpha =\int _{0}^{m}(x)\mathrm{d}h,$ (and using again the BC $x=0$ at $h=m$), it is clear that $\int _{0}^{m}px_{m}\mathrm{d}h=p\alpha _{m}$. One can see also that the limit term in (57) equals zero. The latter fact follows from writing to leading order $m-h=a(m)x^{2}$, or $x=(m-h)^{1/2}/a^{1/2}$, where $h=m-n$. Hence, $x_{h}=-(m-h)^{-1/2}/a^{1/2}$ and $x_{m}=(m-h)^{-1/2}/a^{1/2}-(1/2)(m-h)^{1/2}a_{m}/a^{3/2}$, and noting that $a_{m}$ is finite, we find $[-x_{h}-x_{m}]_{h=m-n}=O(n^{1/2})\rightarrow 0$ when $n\rightarrow 0$.

As a result, we arrive at

$$\begin{aligned} \frac{\mathrm{d}}{\mathrm{d}m}E_{v}=p\frac{\mathrm{d}}{\mathrm{d}m}\alpha . \end{aligned}$$

(58)

Actually, more generally, we can write

$$\begin{aligned} \mathrm{d}E_{v}=p\mathrm{d}\alpha , \end{aligned}$$

which is clear from simple physical considerations.