On the acoustic trapped modes and their symmetry properties in a circular cylindrical waveguide with a cavity

Abstract

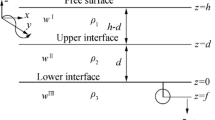
The paper is concerned with a partly analytical, partly numerical study of acoustic trapped modes in a cylindrical cavity (expansion chamber), placed in between two semi-infinite pipes acting as a waveguide. Trapped mode solutions are expressed in terms of Fourier–Bessel series, with the expansion coefficients determined from a determinant condition. The roots of the determinant, expressed in terms of the real wavenumber k, correspond to trapped modes. For a shallow cavity and for low values of the circumferential mode number it is found that there is just one trapped mode in the allowable wave number domain, and this mode is symmetric about a radial axis in the center of the cavity. As the circumferential mode number is increased, more and more trapped modes, placed between two cutoff frequencies, come into play, and they alternate between symmetric and antisymmetric modes. An analytical explanation of the mechanism behind the mode increasing and mode alternation is given via asymptotic expressions of the determinant condition. Numerical computations are done for verification of the analytical results and for consideration of less shallow cavities. Also for these cases, similar phenomena of an increasing number of trapped modes, and alternation between symmetric and antisymmetric modes, are found.

Appendices

Appendices

Comments on the numerical approach

The determinant (58) is evaluated via an LU decomposition [57]. Following this decomposition, the determinant is simply the product of the diagonal elements. As a check, in some cases the determinant was also evaluated directly from the definition [58], up to $N = 7$. In terms of computational time, a direct evaluation is not practical, however, for larger values of N since it involves $4N\!\cdot \!(4N + 4)!$ multiplications and $(4N + 4)!$ summations. Whenever numerical values are given (for the location of trapped modes in terms of the parameter s) these have been determined by employing the bisection method. The numerical values of the Bessel function zeros (8) are taken from Ref. [51], where the first twenty zeros are given with six significant digits, for circumferential mode numbers m up to $m = 8$. For larger zeros than these, and for cases of circumferential mode numbers larger than $m = 8$, McMahon’s expansions are used [51, p. 371].

Convergence study

A verification of convergence to the values of s for which trapped modes are located (e.g., the spikes in Fig. 5) is shown in Table 1. It is noted that the convergence is rapid for small values of $\alpha ^{-1}$, as would be expected. For larger values of $\alpha ^{-1}$, relatively many terms are needed for convergence.

Table 1 Convergence study for the placement $s_{*}$ of the trapped mode, in terms of the parameter s defined by (44), for various values of $\alpha ^{-1} = r_1/r_0$

Convergence to a trapped mode solution for a shallow cavity

The functions ${\mathcal {F}}_{1mqn}$ and ${\mathcal {F}}_{2mqn}$, defined by (29) and (30), can be written

$$\begin{aligned} {\mathcal {F}}_{1mqn} = \frac{2 \alpha ^2 j_{mn}^2}{j_{mn}^2 - m^2} \frac{{\tilde{k}}_{1mn}^{-1}}{J_m^2 \left( j_{mn}\right) } \int _0^1 J_{m}(\alpha j_{mn}{\tilde{r}}) J_m(j_{mq} {\tilde{r}}) {\tilde{r}} \,\mathrm{d}{\tilde{r}} \end{aligned}$$

(59)

and

$$\begin{aligned} {\mathcal {F}}_{2mqn} = \frac{2 j_{mn}^2}{j_{mn}^2 - m^2} \frac{{\tilde{k}}_{0mn}}{J_m^2 \left( j_{mn}\right) } \int _0^1 J_{m}(j_{mn}{\tilde{r}}) J_m(\alpha j_{mq} {\tilde{r}}) {\tilde{r}} \,\mathrm{d}{\tilde{r}}, \end{aligned}$$

(60)

respectively. Employing the expansion (37) with $\epsilon = 1 - \alpha ^2$, we can write

$$\begin{aligned} {\mathcal {F}}_{1mqn}= & {} \alpha ^{m+2} {\tilde{k}}_{1mn}^{-1} \Bigg \{ \delta _{qn} + \frac{2 \alpha ^2 j_{mn}^2}{j_{mn}^2 - m^2} \frac{1}{J_m^2 \left( j_{mn}\right) } \nonumber \\&\times \sum _{p=1}^\infty \frac{\epsilon ^p}{p\,!} \left( {\textstyle \frac{1}{2}} j_{mn} \right) ^p \int _0^1 J_{m+p}(j_{mn}{\tilde{r}}) J_m(j_{mq} {\tilde{r}}) {\tilde{r}}^{p+1} \mathrm{d}{\tilde{r}} \Bigg \}, \end{aligned}$$

(61)

and

$$\begin{aligned} {\mathcal {F}}_{2mqn}= & {} \alpha ^m {\tilde{k}}_{0mn} \Bigg \{ \delta _{qn} + \frac{2 \alpha ^2 j_{mn}^2}{j_{mn}^2 - m^2} \frac{1}{J_m^2 \left( j_{mn}\right) } \nonumber \\&\times \sum _{p=1}^\infty \frac{\epsilon ^p}{p\,!} \left( {\textstyle \frac{1}{2}} j_{mq} \right) ^p \int _0^1 J_m (j_{mn}{\tilde{r}}) J_{m+p}(j_{mq} {\tilde{r}}) {\tilde{r}}^{p+1} \mathrm{d}{\tilde{r}} \Bigg \}. \end{aligned}$$

(62)

It is here assumed that $0 < \epsilon \ll 1$. From [47, p. 219] we have that

$$\begin{aligned}\int _0^1 J_{m+p}(j_{mn}{\tilde{r}}) J_m(j_{mq} {\tilde{r}}) {\tilde{r}}^{p+1} \mathrm{d}{\tilde{r}} \le M/j_{mp},\end{aligned}$$

where M is a constant and $p = \min (n, q)$. It is noted, also, that $J_m(x) \le 2^{-\frac{1}{2}}$ for $m \ge 1 \,$ [59, p. 379]. Furthermore, $J_m(z) \sim (2\pi m)^{-\frac{1}{2}} \left( e\,z/2m \right) ^m$ for $m \gg 1$ [51, p. 365] and $j_{mn} \sim \left( n + \frac{m}{2} - \frac{3}{4} \right) \pi$ for $n \gg 1$ [51, p. 371]. Employing D’Alembert’s ratio test [59, p. 22] it follows that the series in (61), (62) are absolutely convergent for any values of m, q, and n.

It was shown in Sect. 4 that, for the symmetric case, the truncated determinant (42) has a real root, in terms of the real wavenumber ${\tilde{k}}$ as the root. It needs to be shown that this is also the case for the original system (36). In the following we will assume a real ${\tilde{k}}$ and show that the real and the imaginary parts of the finite but arbitrarily large determinant $\Delta _N = |a_{qn}|_0^N$, N large, have identical zeros. Written out for the symmetric case, the determinant for (36) takes the form

$$\begin{aligned} \Delta = \left| \begin{array}{ccccc} 1 &{} -{\mathcal {F}}_{1m00}(1 + \mathrm {e}^{\mathrm {i} {\tilde{k}}_{1m0}{\tilde{l}}}) &{} 0 &{} - {\mathcal {F}}_{1m01} (1 + \mathrm {e}^{\mathrm {i}{\tilde{k}}_{1m1}{\tilde{l}}}) &{} \cdots \\ {\mathcal {F}}_{2m00} &{} 1 - \mathrm {e}^{\mathrm {i} {\tilde{k}}_{1m0}{\tilde{l}}} &{} {\mathcal {F}}_{2m01} &{} 0 &{} \cdots \\ 0 &{} -{\mathcal {F}}_{1m10}(1 + \mathrm {e}^{\mathrm {i} {\tilde{k}}_{1m0}{\tilde{l}}}) &{} 1 &{} - {\mathcal {F}}_{1m11}(1 + \mathrm {e}^{\mathrm {i} {\tilde{k}}_{1m1}{\tilde{l}}}) &{} \cdots \\ {\mathcal {F}}_{2m10} &{} 0 &{} {\mathcal {F}}_{2m11} &{} 1 - \mathrm {e}^{ \mathrm {i} {\tilde{k}}_{1m1}{\tilde{l}}} &{} \cdots \\ \vdots &{} \vdots &{} \vdots &{} \vdots &{} \ddots \\ \end{array} \right| . \end{aligned}$$

(63)

Let $\alpha j_{m0}< {\tilde{k}} < j_{m0}$, ${\tilde{k}}$ real. Then, employing (24) and noticing the numerical gaps between the roots of the differentiated Bessel functions $J'_{m}(z)$—denoted by $j_{m0}$, $j_{m1}$, ..., cf. (8), for $m = 1$, $j_{10} = 1.8412$, $j_{11} = 5.3315$, ...; $j_{20} = 3.0542$, $j_{21} = 6.7061$, etc. [51, p. 411]—it will be seen that ${\tilde{k}}_{1m0}$ is real while ${\tilde{k}}_{1mn}$ is purely imaginary for $n \ge 1$; also, ${\tilde{k}}_{0mn}$ is purely imaginary for all $n \ge 0$. Accordingly, from (29) and (30) (or from (61) and (62)), ${\mathcal {F}}_{1mq0}$ is real while ${\mathcal {F}}_{1mqn}$ is purely imaginary for $n \ge 1$; and ${\mathcal {F}}_{2mqn}$ is purely imaginary for $n \ge 0$. It is convenient, then, to write ${\tilde{k}}_{1mn} = \mathrm {i} {\tilde{k}}^{*}_{1mn}$ for $n \ge 1$, ${\mathcal {F}}_{1mqn} = -\mathrm {i}{\mathcal {F}}^{*}_{1mqn}$, also for $n \ge 1$, and ${\mathcal {F}}_{2mqn} = \mathrm {i}{\mathcal {F}}^{*}_{2mqn}$ for all $n \ge 0$. Using this ‘asterisk notation,’ (63) may be written

$$\begin{aligned} \Delta = \left| \begin{array}{ccccc} 1 &{} -{\mathcal {F}}_{1m00}(1 + \mathrm {e}^{\mathrm {i} {\tilde{k}}_{1m0}{\tilde{l}}}) &{} 0 &{} \mathrm {i}{\mathcal {F}}^{*}_{1m01} (1 + \mathrm {e}^{- {\tilde{k}}^{*}_{1m1}{\tilde{l}}}) &{} \cdots \\ \mathrm {i} {\mathcal {F}}^{*}_{2m00} &{} 1 - \mathrm {e}^{\mathrm {i} {\tilde{k}}_{1m0}{\tilde{l}}} &{} \mathrm {i} {\mathcal {F}}^{*}_{2m01} &{} 0 &{} \cdots \\ 0 &{} -{\mathcal {F}}_{1m10}(1 + \mathrm {e}^{\mathrm {i} {\tilde{k}}_{1m0}{\tilde{l}}}) &{} 1 &{} \mathrm {i}{\mathcal {F}}^{*}_{1m11}(1 + \mathrm {e}^{- {\tilde{k}}^{*}_{1m1}{\tilde{l}}}) &{} \cdots \\ \mathrm {i} {\mathcal {F}}^{*}_{2m10} &{} 0 &{} \mathrm {i} {\mathcal {F}}^{*}_{2m11} &{} 1 - \mathrm {e}^{-{\tilde{k}}^{*}_{1m1}{\tilde{l}}} &{} \cdots \\ \vdots &{} \vdots &{} \vdots &{} \vdots &{} \ddots \\ \end{array} \right| . \end{aligned}$$

(64)

In this notation, any coefficient in (64) is real and any imaginary quantity is proceeded by an $\mathrm {i}$. In order to show that the real and imaginary parts of (64) have identical zeros (roots), it is instructive to first evaluate the truncated determinant directly in a low-order case. Recall that the truncated determinant $\Delta _N$, with the present consideration of symmetry, is of order $2(N + 1) \times 2(N + 1)$, $N = 0, 1, \dots$. Consider first the smallest possible truncation, with $N = 0$. Then

$$\begin{aligned} \Delta _0= & {} \, 1 - \mathrm {e}^{\mathrm {i} {\tilde{k}}_{1m0}{\tilde{l}}} + \mathrm {i} {\mathcal {F}}_{1m00} {\mathcal {F}}^{*}_{2m00} \left( 1 + \mathrm {e}^{\mathrm {i} {\tilde{k}}_{1m0}{\tilde{l}}}\right) \nonumber \\= & {} \, 1 - \cos \left( {\tilde{k}}_{1m0}{\tilde{l}}\right) - {\mathcal {F}}_{1m00} {\mathcal {F}}^{*}_{2m00}\sin \left( {\tilde{k}}_{1m0}{\tilde{l}}\right) \nonumber \\&+ \; \mathrm {i} \left[ - \sin \left( {\tilde{k}}_{1m0}{\tilde{l}}\right) + {\mathcal {F}}_{1m00} {\mathcal {F}}^{*}_{2m00} \left\{ 1 + \cos \left( {\tilde{k}}_{1m0}{\tilde{l}}\right) \right\} \right] . \end{aligned}$$

(65)

It is noted that (65) is equivalent to (42) since, from (61) and (62) it follows that the real quotient ${\mathcal {F}}_{1m00} {\mathcal {F}}^{*}_{2m00}$ can be written as

$$\begin{aligned} {\mathcal {F}}_{1m00} {\mathcal {F}}^{*}_{2m00} = \alpha ^{2(m+1)}\frac{\mathrm{Im}({\tilde{k}}_{0m0})}{{\tilde{k}}_{1m0}} + O(\epsilon ) \quad \hbox {as} \quad \epsilon \rightarrow 0. \end{aligned}$$

(66)

The ‘correction’ term ($O(\epsilon )$) is also real (cf. (61), (62)). Setting real and imaginary parts of (65) equal to zero gives

$$\begin{aligned} \frac{1 - \cos \left( {\tilde{k}}_{1m0}{\tilde{l}}\right) }{\sin \left( {\tilde{k}}_{1m0}{\tilde{l}}\right) } = {\mathcal {F}}_{1m00} {\mathcal {F}}^{*}_{2m00} \quad \hbox {and} \quad \frac{\sin \left( {\tilde{k}}_{1m0}{\tilde{l}}\right) }{1 + \cos \left( {\tilde{k}}_{1m0}{\tilde{l}}\right) } = {\mathcal {F}}_{1m00} {\mathcal {F}}^{*}_{2m00}, \end{aligned}$$

(67)

respectively. The left-hand sides as well as the right-hand sides are identities, that is, the real and the imaginary part have identical roots. The example discussed in Sect. 4.1 can now be employed, together with (66), to show directly that each of the equations in (67) has a zero when the real wavenumber ${\tilde{k}} \in (\alpha j_{m0}, j_{m0})$. (This remains true also when the said correction term is present.)

Consider now the determinant $\Delta _N$, of order $2(N + 1) \times 2(N + 1)$, with N large. Except for the diagonal elements and the elements in the second column, any non-zero element is purely imaginary. Multiplying all elements of any row (or any column) by $\mathrm {i}$ corresponds to multiplying the resultant determinant by $\mathrm {i}$ (cf., e.g., [60], p. 25). We can, then, multiply each row (or each column) by $\mathrm {i}$, which will correspond to multiplying the determinant by $\mathrm {i}^{2(N + 1)}$. Then all elements that are non-diagonal and not in the second column will be real.

Next, we expand the determinant in terms of the second column. It is noted that the second term of the diagonal, $a_{22}$, is proportional to $1 - \mathrm {e}^{\mathrm {i} {\tilde{k}}_{1m0}{\tilde{l}}}$; any other term $a_{j2}$, $j \ne 2$, is proportional to $1 + \mathrm {e}^{\mathrm {i} {\tilde{k}}_{1m0}{\tilde{l}}}$. The minor multiplying the element $a_{j2}$ of the second column will contain an element of any row, except row number j, and an element of any column, except column number 2. Thus, for any N, the determinant $\Delta _N$ will take the form

$$\begin{aligned} \Delta _N = \mathrm {i}^{(2(N + 1) + 1)} \left\{ \left( 1 - \mathrm {e}^{\mathrm {i} {\tilde{k}}_{1m0}{\tilde{l}}} \right) {\mathcal {A}}_N + \mathrm {i} \left( 1 + \mathrm {e}^{\mathrm {i} {\tilde{k}}_{1m0}{\tilde{l}}} \right) {\mathcal {B}}_N \right\} , \end{aligned}$$

(68)

where ${\mathcal {A}}_N$ and ${\mathcal {B}}_N$ are real quantities. Setting real and imaginary parts equal to zero gives

$$\begin{aligned} \frac{1 - \cos \left( {\tilde{k}}_{1m0}{\tilde{l}}\right) }{\sin \left( {\tilde{k}}_{1m0}{\tilde{l}}\right) } = \frac{{\mathcal {B}}_N}{{\mathcal {A}}_N}, \qquad \frac{\sin \left( {\tilde{k}}_{1m0}{\tilde{l}}\right) }{1 + \cos \left( {\tilde{k}}_{1m0}{\tilde{l}}\right) } = \frac{{\mathcal {B}}_N}{{\mathcal {A}}_N}, \end{aligned}$$

(69)

equivalently to (67). Thus, for any truncation N, the real and imaginary parts of the determinant equation $\Delta _N = 0$ have identical (coinciding) roots at a certain (real) value of ${\tilde{k}}$ in its allowed domain.

Numerical verification

Table 2 shows placements of trapped modes (in terms of the placement of the roots s of $\Delta _N(s) = 0$ and the corresponding values of ${\tilde{k}}$) for a cavity with parameters $\alpha ^{-1} = r_1/r_0 = 3$ and $-\tilde{z_1} = {\tilde{z}}_2 = -z_1/r_0 = z_2/r_0 = 5 \Rightarrow {\tilde{l}} = 10$, which corresponds to $h/D = 1$ and $\ell /D = 5$ in the notation of Hein and Koch [25], where h is the difference between the cavity radius and the pipe radius ($r_1 - r_0$ in our notation), D is the pipe diameter ($2r_0$ in our notation), and $\ell = z_2 - z_1$. In Ref. [25], $K = \omega D/c_0$, which corresponds to $2{\tilde{k}}$ in our notation. It should be emphasized that $(m, n, \rho )$ is used in the notation of Ref. [25], where m is the circumferential mode number, n is the number of nodal lines in the axial direction, and $\rho$ is the number of nodal lines in the radial direction. (It is noted that the meanings of n and $\rho$ are different in the present paper.)

The table shows that the values $2{\tilde{k}}/2\pi$ obtained via the present analysis (using expansion into 20 modes, i.e., $N = 19$) are in good agreement with values of $K/2\pi$ from Fig. 18 in Ref. [25]. Included in the last column of the table are the modal numbers $(m, n, \rho )$ in the notation of Ref. [25], where m is the circumferential mode number, n is number of nodal lines in the axial (z) direction, and $\rho$ is number of nodal lines in the radial (r) direction.

Table 2 The first five (out of eight) trapped modes and comparison of wavenumber values with those of Ref. [25] [Sect. 4.1, Fig. 18]