Energy Decay in the Dynamics of Complex Bodies with Spreading Microstructures Represented by $3D$ Vectors

Abstract

We analyze complex bodies with active microstructure described by a vector field with values $\nu \in \mathbb{R}^{3}$, which complements the displacement $u\in \mathbb{R}^{3}$. We consider linear elastic constitutive structures for macroscopic and microstructural stresses, diffusion of microstructures, and a viscoelastic-substrate-type effect, which has a regularizing influence that we eliminate at a later stage of our analysis. For weak solutions of the balance equations, we prove decay inequalities in $W^{1,2}$ and also for the $p$-power of the norms of $u$ and $\nu$ in $L^{p}$ spaces under slip-without-friction-type boundary conditions.

1 Introduction

In certain material classes, events at spatial scales lower than the macroscopic one at which we accept a continuum description of condensed matter show features hardly representable in the standard format of continuum mechanics. Examples are manifold; their list include polarization in ferroelectrics, atomic shifts in quasicrystals, phase transitions of various nature, relative strain of polymers with respect to a ground fluids in which are scattered, diffusion into soft matter as it occurs when ions flow into muscles or proteins trans-locate trough tissues, etc.

In the presence of microstructural diffusion a question goes as follows: Does such an effect influence or even determine an overall energy decay in a dynamic regime when the macroscopic behavior is elastic while the microscopic one is slightly dissipative? If so, how?

In linear elastostatics, proving the energy decay along cylinders with special boundary conditions is at the roots of Saint-Venant’s classical theory: the basis of beam analyses in all engineering applications. In dynamics we have results for viscoelastic flows or thermoelastic behavior. The analysis of energy decay in the dynamics of micro-to-macro interactions in materials with active microstructure – so-called complex to remind the circumstance – requires appropriate work. Here we make some steps along the pertinent path.

To account for the morphology of microstructures, we do not look at the body geometry only in terms of the macroscopic region it may occupy in the ambient space: a point space commonly identified with $\mathbb{R}^{3}$. Rather we include appropriate descriptors of microstructural features that characterize the specific material class under analysis. Circumstances and even taste suggest the adoption of different nominal choices for them: morphological descriptors, order-parameters, phase fields; in all these choices we refer to variables that are sensitive to changes in observers.¹ True interactions are associated with such fields; they are defined by the power that they perform in the time rate of the microstructural descriptors. Invariance requirements for different entities (external power, the relative one [21], the balance of energy, a mechanical dissipation inequality) allow one to deduce appropriate balances to be satisfied by the microstructural actions ([9], [19], [21]). Such an aspect does not depend on the specific mathematical nature of the descriptor chosen: we just need to say, in general, that the microstructural descriptors belong to a finite-dimensional differentiable manifold not necessarily embedded into a linear space [7], [19].

Here, we reduce generality and refer to descriptors of the microstructure that are three-dimensional vectors, at times called directors. Sure, mechanics of continua accounting for them can be constructed per se ([30], [12], [14]). In any case, the resulting theory is a chapter of the general model-building framework for the mechanics of complex materials ([7], [19], [21]). It reveals itself as a non-marginal chapter when we look at the prominence of material classes the phenomenology of which suggests to adopt such a scheme in the pertinent models. Examples range from the low-dimensional description of beams and shells [2] to models of ferroelectric behavior [17], to the description of distributed micro-cracking [18] or to the mechanics of quasicrystals ([16], [25], [26], [28], [20], [23]). In order to clarify the issue, we describe the last case with more details. Quasicrystals are aluminum-based alloys characterized by intrinsically quasi-periodic arrangements of atoms in space. They were discovered in 1982 when in electron diffraction experiments a distribution of Bragg’s peaks with ten-fold symmetry was recorded [27]. It constituted an incompatible scenario with lattice periodicity, later recognized not due to twin structures. Quasi-periodic lattices can be seen as projections of a higher-dimensional periodic lattice on an incommensurate subspace with lower dimension. So, a displacement in the hyper-lattice has two components: one in the space over which we project – it corresponds to the standard displacement – and another component in the portion of the hyperspace that is orthogonal to the subspace where we project. We obtain an analogous picture if we look at the mass of a three-dimensional quasi-periodic lattice: its expansion in Fourier series implies six-dimensional wave vectors. The three additional degrees of freedom can be interpreted as those exploited by atoms to rearrange themselves as to assure quasi-periodicity [16]. We then collect these additional degrees of freedom into a vector field, which is indifferent to rigid translations of observers in the ambient physical space because such degrees of freedom are relative to the generic material element collapsed at a point in the continuum representation. However, relative rotations affect the way different observers evaluate the orientation of such a vector field. In this sense we say that it is an observable entity. For this reason the entities that are dual to its time rate and the rate of its gradient are true interactions that affect the overall power over a body and have to be balanced (see pertinent papers referenced above). Also, scattering experiments on quasicrystals record only three sound-like branches [28], those referred just to the standard displacement field. Consequently, in this case we do not attribute peculiar kinetic energy to the degrees of freedom described by the additional vector field, leaving to it a role during diffusion and at equilibrium or even including only rotational inertia [23], an issue – this last one – on which we come back below in this section.

We consider a body that occupies in the three-dimensional ambient space a bounded connected region $\mathcal{B}\subseteq \mathbb{R}^{3}$, endowed with piecewise $C^{1}$-boundary (even if, in most of the situations, Lipschitz regularity for the boundary will be enough). We define two differentiable fields, depending on space $x\in \mathcal{B}$ and time $t\in [0,T]$, $T>0$, variables, namely the displacement $u:=\tilde{u}(t,x)\in \mathbb{R}^{3}$ and the microstructural descriptor $\nu :=\tilde{\nu}(t,x)\in \mathbb{R}^{3}$.

Interactions are defined by the power that they perform in the time rate of what describes the body morphology. In the present case, the two fields involved in such a description are $\tilde{u}$ and $\tilde{\nu}$.

Standard bulk and contact actions are defined by the power that they develop in the macroscopic velocity. Microstructural actions appear as conjugated with the time rate of $\tilde{\nu}$ and they are subdivided into bulk and contact families, the former including external actions and inner self-actions, the latter being represented by a microstress field. Both classes of actions require to be balanced. We derive such balance equations from an invariance requirement, according to a procedure explained in references [19] and [21].

Then, we specialize them to a scenario defined as follows:

• We consider small strain regime.

• We omit viscous components of the standard stress and the microstress.

• We attribute dissipation only to the microstructural self-action, which drives microstructural diffusion (as it occurs in quasicrystals, which modeling falls within the present scheme [20], [26]).

• We do not consider the possibility of gyroscopic inertia attributed to $\nu$, a non-linear effect investigated in reference [6] by including regularizing terms due to second-neighbor interactions not included in the present scheme.

• We consider at first the body on a viscoelastic substrate. So, a damping term $\tilde{\gamma}u_{t}$ in the balance of standard forces accounts for the circumstance. Terms like $\tilde{\gamma}u_{t}$ also appear when we consider the Coriolis force as, for instance, in reference [11] or account for sticky viscosity in the presence of rough boundaries ([13], [4]), or when we refer to capillary effects as in Brinkman-Forchheimer’s scheme ([31], [15]). We obtain a first result with $\tilde{\gamma}\neq 0$. Then, we analyze the limit $\tilde{\gamma}=0$.

In this setting, the balance equations reduce to

$$ \begin{aligned} & \rho u_{tt} + \tilde{\gamma} u_{t} -\mu \Delta u = \xi \nabla \mathrm{div} u + \kappa \Delta \nu + \bar{\xi}\nabla \mathrm{div} \nu , & \textrm{ in } [0,T]\times \mathcal{B}, \\ &\varsigma \nu _{t} - \zeta \Delta \nu = \gamma \nabla \mathrm{div} \nu + \kappa \Delta u +\bar{\xi}\nabla \mathrm{div} u -\kappa _{0} \nu , & \textrm{ in } [0,T]\times \mathcal{B}, \\ &u|_{t=0}=u_{0}, \,\, u_{t}|_{t=0}=\dot{u}_{0},\,\, \nu |_{t=0}=\nu _{0}, & \textrm{ on } \mathcal{B}, \end{aligned} $$

(1.1)

where subscripts indicate partial derivatives. Also, $\rho$, $\mu$, $\xi$, $\kappa$, $\bar{\xi}$, $\varsigma$, $\zeta$, $\gamma$ and $\kappa _{0}$ are positive constants. We also set $\tilde{\gamma}\ge 0$, discussing the two cases $\tilde{\gamma}=0$ or $\tilde{\gamma}>0$ (see Theorems 3.1 and 3.2).

We consider slip-without-friction-like boundary conditions given by

$$ \left \{ \textstyle\begin{array}{l@{\quad}l} \displaystyle u\cdot n = 0, \,\, \mathrm{curl}\,u\times n = 0,& \textrm{ on } [0,T]\times \partial \mathcal{B}, \\ \nu \cdot n =0, \,\, \mathrm{curl}\,\nu \times n = 0, & \textrm{ on } [0,T] \times \partial \mathcal{B}. \end{array}\displaystyle \right . $$

(1.2)

We take the region ℬ to be simply connected.

We show energy decay for weak solutions of the above balance equations (Theorems 3.1 and 3.2 below).

Derivation of the balance equations under analysis here and the pertinent constitutive structures specifying them into system (1.1) are in references [20], [23]. For the sake of clarity, we sketch in Sect. 2 the path leading to them. Deriving the balance equations considered from a fully nonlinear setting allows us to put them into a wide perspective.

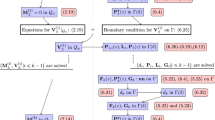
The main results are in Sect. 3, that is, Theorems 3.1 and 3.2. In Sect. 4, after preliminary notations and lemmas, we provide low-order a priori estimates for solutions of (1.1)-(1.2). Thanks to the assumed regularity on the initial data, we also obtain a decay estimate for $\|u_{t}\|$ (see Lemma 4.2).

Section 5 is devoted to high-order estimates for solutions to system (1.1), which lead also to a decay estimate for $\|\nabla u_{t}\|$ (see Lemma 5.1). Collecting the results from Sects. 4–5, we also provide the proof of Theorem 3.1. Finally, in Sect. 6, we prove the crucial inequality to obtain Theorem 3.2.

2 On the Origin of the Balance Equations Considered

2.1 Body Morphology and Generalized Motions

Take $\hat{\mathbb{R}}^{3}$ and a copy of it, say $\mathbb{R}^{3}$, connected just by the identification map $\iota :\hat{\mathbb{R}}^{3}\longrightarrow \mathbb{R}^{3}$. We define two non-singular metrics: $\hat{g}$ in $\hat{\mathbb{R}}^{3}$ and $g$ in $\mathbb{R}^{3}$ We select ℬ in $\hat{\mathbb{R}}^{3}$, considering it as a macroscopic reference shape. Deformed configurations are reached in $\mathbb{R}^{3}$ through orientation-preserving differentiable one-to-one maps $x\longmapsto y:=\tilde{y}(x)\in \mathbb{R}^{3}$. We indicate by $F$ the derivative $D\tilde{y}(x)$; its link with the gradient $\nabla \tilde{y}(x)$ is given by the relation $\nabla \tilde{y}(x)=D\tilde{y}(x)\hat{g}^{-1}$; so the two can be identified when $\hat{g}^{-1}$ is flat, i.e., it refers to an orthonormal frame; for this reason we adopt the classical terminology for $F$ and call it the deformation gradient.² Two linear operators are associated with $F$: its formal adjoint $F^{\ast}$ and its transpose $F^{\mathsf{T}}$; the two are connected by the relation $F^{\mathsf{T}}=\hat{g}^{-1}F^{\ast}g$; they coincide when both metrics are flat. We also define the displacement field by $u=\tilde{u}(x):=\tilde{y}(x)-\iota (x)$, so that $F=I+D\tilde{u}(x)$, with $I$ the shifter from $\hat{\mathbb{R}}^{3}$ to $\mathbb{R}^{3}$, with components $\delta ^{i}_{\;A}$, where capital indices refer to coordinates in $\hat{\mathbb{R}}^{3}$, while the others to their counterparts in $\mathbb{R}^{3}$.

The field $\tilde{\nu}:\mathcal{B} \longrightarrow \mathbb{R}^{3}$ already mentioned is considered to be differentiable. It accounts for microstructural features. We indicate by $N$ its spatial derivative $D\tilde{\nu}(x)$. The pair $(\tilde{y}(x,t), \tilde{\nu}(x,t))$ is what we consider as a generalized motion, with the time $t$ varying into the interval $[0,T]$. We assume both maps to be differentiable with respect to time, so that velocities are given by $\dot{y}=\frac{\mathrm{d}\tilde{y}(x,t)}{\mathrm{d}t}$ and $\dot{\nu}=\frac{\mathrm{d}\tilde{\nu}(x,t)}{\mathrm{d}t}$. Of course, we have $\dot{y}=\dot{u}$.

2.2 Power of Standard and Microstructural Actions, and Invariance

We say that $\mathfrak{b}\subseteq \mathcal{B}$ is a part of ℬ when it is connected, endowed with non-vanishing volume and a piecewise Lipschitz boundary.

On every part, we recognize bulk and contact (i.e., boundary) actions exerted by the rest of the body and the environment. They are defined by the external power $\mathcal{P}^{\mathit{ext}}_{\mathfrak{b}}$ that they develop on any rate of change of the body morphology, namely on any pair $(\dot{y}, \dot{\nu})$. In this view, we define the external power by

$$ \mathcal{P}_{\mathfrak{b}}^{\mathit{ext}}\left ( \dot{y},\dot{\nu}\right ) := \int _{\mathfrak{b}}\left ( b^{\ddagger }\cdot \dot{y}+\beta ^{\ddagger } \cdot \dot{\nu}\right ) \mathrm{d}\mu (x)+\int _{\partial \mathfrak{b}}\left ( \mathfrak{t}_{\partial}\cdot \dot{y}+\tau _{\partial} \cdot \dot{\nu}\right ) \mathrm{d}\bar{\mathcal{H}}^{2}(x), $$

where $\mathrm{d}\mu (x)$ is the standard volume measure, $\mathrm{d}\bar{\mathcal{H}}^{2}(x)$ the Hausdorff one, and the dot indicated duality pairing, identified with the scalar product when the metrics considered are flat. Subscript $\partial$ associated with the contact actions indicates that we presume dependence of $\mathfrak{t}$ and $\tau$ on the boundary $\partial \mathfrak{b}$. We subordinate $\mathcal{P}^{\mathit{ext}}_{\mathfrak{b}}$ to invariance under isometry-based changes in observers defined by

$$ \dot{y}^{\diamond }:=\mathfrak{c}+q\times \left ( y-y_{0}\right ) + \dot{y} \qquad \text{and}\qquad \dot{\nu}^{\diamond}=\dot{\nu}+ \mathcal{A}\left ( \nu \right ) q, $$

where $\mathfrak{c}$ is a translational velocity, $q$ a rotational one, both depending on time only, and × indicates the vector product; the linear operator $\mathcal{A}\left ( \nu \right )$ is given by $\mathcal{A} (\,\cdot \,) =-\nu \times (\, \cdot \, )$. The rate $\dot{\nu}$ is independent of the rigid translation of frames in space because $\nu$ represents a relative shift inside each material element. At variance, it is sensitive to rotations of observers (i.e., frames of reference).

The requirement of invariance reads $\mathcal{P}_{\mathfrak{b}}^{\mathit{ext}}\left ( \dot{y},\dot{\nu}\right ) = \mathcal{P}_{\mathfrak{b}}^{\mathit{ext}}\left ( \dot{y}^{\diamond },\dot{\nu}^{\diamond } \right )$, presumed valid for any choice of $\mathfrak{b}$, $\mathfrak{c}$, and $q$; their arbitrariness implies first the validity of the two integral balances

$$ \int _{\mathfrak{b}}b^{\ddagger }\;\;\mathrm{d}\mu (x)+\int _{\partial \mathfrak{b}}\mathfrak{t}_{\partial}\;\;\mathrm{d} \bar{\mathcal{H}}^{2}(x)=0, $$

$$ \int _{\mathfrak{b}}\left ( \left ( y-y_{0}\right ) \times b^{\ddagger }+ \mathcal{A}^{\ast }\beta ^{\ddagger }\right ) \mathrm{d}\mu (x)+\int _{\partial \mathfrak{b}}\left ( \left ( y-y_{0}\right ) \times \mathfrak{t}_{ \partial}+\mathcal{A}^{\ast }\tau _{\partial} \right ) \mathrm{d} \bar{\mathcal{H}}^{2}(x)=0, $$

where $\mathcal{A}^{\ast }$ is the formal adjoint of $\mathcal{A}$.

The first integral equation is the standard balance of forces, those developing power in the time rate of a gross deformation. The second one is a non-standard balance of couples.

• If $\left \vert b^{\ddagger }\right \vert$ is bounded over $\mathcal{B}\$ and $\mathfrak{t}_{\partial}$ depends continuously on $x$, the action-reaction principle holds first on flat boundaries, and, on its basis, one may further show that $\mathfrak{t}_{\partial}$ depends on $\mathfrak{\partial \mathfrak{b}}$ only through the normal $n$ to it in all points where it is well-defined and extends there the action-reaction property, i.e., $\mathfrak{t}_{\partial}=\mathfrak{t}:=\tilde{\mathfrak{t}}\left ( x,n \right ) =-\tilde{\mathfrak{t}}\left ( x,-n\right )$. Also, as a function of $n$, $\tilde{\mathfrak{t}}$ is homogeneous and additive, i.e., there exists a second-rank tensor field $x\longmapsto P\left ( x\right )$ such that $\tilde{\mathfrak{t}}\left ( x,n\right ) =P\left ( x\right ) n\left ( x \right )$. This is the standard Cauchy theorem preceded by the Hamel-Noll result; $P$ is the first Piola-Kirchhoff stress.

• Since ℬ is bounded, as above selected, we can choose the arbitrary point $y_{0}$ in a way such that the boundedness of $| b^{\ddagger }|$ implies the same of $| (y-y_{0})\times b^{\ddagger }|$. If in addition $\left \vert \mathcal{A}^{\ast }\beta ^{\ddagger }\right \vert$ is bounded over ℬ and $\tau _{\partial}$ depends continuously on $x$, the microstructural contact action $\tau _{\partial}$ satisfies a non-standard action-reaction principle and depends on $\mathfrak{\partial \mathfrak{b}}$ only through the normal $n$ to it in all points where it is well-defined; we have, in fact, $\mathcal{A}^{\ast }\left ( \tilde{\tau} \left ( x,n\right ) + \tilde{\tau} \left ( x,-n\right ) \right ) =0$. Also, as a function of $n$, $\tilde{\tau}$ is homogeneous and additive, i.e., there exists a second-rank tensor field $x\longmapsto \mathcal{S}\left ( x\right )$, so called microstress, such that $\tilde{\tau} \left ( x,n\right ) =\mathcal{S}\left ( x\right ) n \left ( x\right )$.

• If both stress fields are in $C^{1}\left ( \mathcal{B}\right ) \cap C\left ( \bar{\mathcal{B}} \right )$ and the bulk actions $x\longmapsto b$, $x\longmapsto \beta ^{\ddagger }$ are continuous over ℬ, the point-wise balance of forces

  $$ \mathrm{Div}P+b^{\ddagger }=0 $$

  (2.2)

  holds and there exists a field $x\longmapsto z\left ( x\right ) \in T_{\nu }^{\ast }\mathcal{M}$ such that

  $$ \mathrm{Div}\mathcal{S}+\beta ^{\ddagger }-z=0\;,\qquad \mathrm{skw}\mathit{PF}^{ \ast }=\frac{1}{2}\mathsf{e}\left ( \mathcal{A}^{\ast }z+\left ( D \mathcal{A}^{\ast }\right ) \mathcal{S}\right )\;; $$

  (2.3)

  moreover,

  $$ \mathcal{P}_{\mathfrak{b}}^{\mathit{ext}}\left ( \dot{y},\dot{\nu}\right ) = \int _{\mathfrak{b}}\left ( P\cdot \dot{F}+z\cdot \dot{\nu}+\mathcal{S} \cdot \dot{N}\right ) \mathrm{d}\mu (x)\;, $$

  (2.4)

  with the right-hand side integral called internal (or inner) power.

As usual, we presume that $b^{\ddagger }$ admits a decomposition into inertial and non-inertial components, namely $b^{\ddagger }=b^{in}+b$, the former defined to be such that the negative of its power on any part of the body and any velocity field equals the time rate of the kinetic energy pertaining to the same part. Velocity arbitrariness implies the identification $b^{in}=-\rho \ddot{y}=-\rho \ddot{u}$. Since $x$ is fixed, we have $\ddot{u}=u_{tt}$. For the purposes of this paper, we choose a non-inertial live load $b=\tilde{\gamma} u_{t}$ as already mentioned in the introduction, and we also set $\beta ^{\ddagger }=0$ (for more general analyses on $\beta ^{\ddagger }$ see references [8], [19], [21]).

2.3 Mechanical Dissipation Inequality and Constitutive Structures

The Clausius-Duhem inequality, a form of the second law, written here in isothermal setting by involving the above form of internal power, furnishes a priori constitutive restrictions. In its abstract version (see any of the treatises mentioned above), the Clausius-Duhem inequality in its isothermal version reads as follows:

$$ \frac{d}{dt}(\text{free energy of}\; \mathfrak{b})-( \text{rate at which the work is performed in} \;\mathfrak{b})\leq 0. $$

It is required that the inequality holds for any choice of the rate fields involved, each one considered independent from the other.³ Thus a point is what explicit expression for the power (i.e., the rate at which ... etc.) comes into play. In our treatment the inner power involves the microstructural interactions, so it is not only the standard integral of $P\cdot \dot{F}$, rather its density is $P\cdot \dot{F}+z\cdot \dot{\nu}+\mathcal{S}\cdot \dot{N}$ (see also [7]).

Then, explicitly, the inequality reads

$$ \frac{\mathrm{d}}{\mathrm{d}t}\int _{\mathfrak{b}}\psi \;\;\mathrm{d} \mu (x)-\int _{\mathfrak{b}}\left ( P\cdot \dot{F}+z\cdot \dot{\nu}+\mathcal{S} \cdot \dot{N}\right ) \mathrm{d}\mu (x)\leq 0\;, $$

where $\psi$ is the free energy density. It is presumed to hold true for any choice of the time rates involved. The arbitrariness of $\mathfrak{b}$ implies the local dissipation inequality

$$ \dot{\psi}-P\cdot \dot{F}-z\cdot \dot{\nu}-\mathcal{S}\cdot \dot{N} \leq 0\;,$$

which is assumed to hold for any (independent) choice of the rates involved. As constitutive functional dependence on state variables, we assume

$$ \psi =\tilde{\psi}(F,\nu , N)\;\qquad P=\tilde{P}(F,\nu , N)\;\qquad \mathcal{S}=\tilde{\mathcal{S}}(F,\nu , N)$$

and

$$ z=\tilde{z}^{e}(F,\nu , N)+\tilde{z}^{d}(F,\nu , N, \dot{\nu})\;.$$

The assumptions about first Piola-Kirchhoff stress and microstress imply that we are considering them only with energetic nature. The assumption on $z$ (a microstructural self-action, indeed) represents it as the sum of an energetic component and a purely dissipative one (an analogous decomposition holds for the first Piola-Kirchhoff stress in classical viscoelasticity, which is involved here). By inserting into the inequality and exploiting the arbitrariness of $\dot{F}$, $\dot{\nu}$, and $\dot{N}$, we get

$$ P=\frac{\partial \psi}{\partial F}\;,\;\;\;z^{e}= \frac{\partial \psi}{\partial \nu}\;, \;\;\;\mathcal{S}= \frac{\partial \psi}{\partial N}\;,\;\;\;z^{d}\cdot \dot{\nu}\geq 0. $$

The last inequality is compatible with

$$ z=\frac{\partial \psi}{\partial \nu}+a(\dots )\dot{\nu}\;, $$

with $a(\dots )$ a real-positive-valued state function, here chosen to be a scalar $\varsigma$ only for the sake of simplicity.

Remark 2.1

The inequality $z^{d}\cdot \dot{\nu}\geq 0$ also is compatible with $z^{d}=A(\dots )\dot{\nu}$, with $A$ a second-rank positive definite tensor-valued function of state variables and, possibly, their gradients. We choose the form $a(\dots )\dot{\nu}$ to avoid possible problems associated with the tensor structure, as pointed out in reference [3]. Here, the choice $a(\dots )\dot{\nu}$ describes local diffusion (which can be also interpreted as a dissipation of the microstructure inside each material element).

Remark 2.2

We could also adopt an analogous decomposition into energetic and dissipative components for both the microstress $\mathcal{S}$ (as it seems to be appropriate for the case of bi-atomic media [15]) and the first Piola-Kirchhoff stress $P$, imagining in this last case that even macroscopic viscosity would occur. Both choices would in fact have a regularizing role and would assure a faster decay of the energy. Our aim is to show that even a minimal dissipative behavior of the microstructure influence non-trivially the energy decay. For this reason, we restrict ourselves to the less regularized setting in which the microstructural dissipation is due only to $z^{d} = \zeta\dot{\nu}$, where $\zeta$ is a positive constant.

The actions occurring in the materials (stresses and self-action), that we have represented so far as fields defined over ℬ, i.e., by using a Lagrangian representation, have their counterparts defined on the current configuration $\mathcal{B}_{c}:=\tilde{y}(\mathcal{B},t)$. They are given by

$$ \sigma =\frac{1}{\mathrm{det} F}\frac{\partial \psi}{\partial F}F^{ \ast}\;,\;\;\;z_{c} =\frac{1}{\mathrm{det} F}\bigg( \frac{\partial \psi}{\partial \nu}+a(\dots )\dot{\nu}\bigg)\;,\;\;\; \mathcal{S}_{c}=\frac{1}{\mathrm{det} F} \frac{\partial \psi}{\partial N}F^{\ast}, $$

where $\sigma$ is the standard Cauchy stress, while $z_{c}$ and $\mathcal{S}_{c}$ are respectively self-action and microstress in Eulerian representation.

The condition $|\nabla u|\ll 1$ defines small strain regime. We accept it here with the consequence that we may avoid to distinguish between referential (ℬ) and current ($\mathcal{B}_{c}$) configurations. It implies also the approximations

$$ \sigma \approx P\;,\;\;\;z_{c}\approx z\;,\;\;\;\mathcal{S}_{c} \approx \mathcal{S}, $$

and the possibility of choosing for the free energy density $\psi$ a quadratic dependence on its entries, which would be otherwise forbidden because in large strain regime convexity of the free energy with respect to the deformation gradient is incompatible with the requirement of objectivity for the energy density, which is a requirement of invariance under rigid-body type changes in observers ($\mathit{SO}(3)$ invariance). Such a limitation does not affect the small strain regime accepted here, so that, by referring to flat metrics, we select (for the derivation of such an expression under symmetry conditions see reference [23])

$$ \begin{aligned} \psi =&\frac{1}{2}\lambda \left ( \mathrm{sym} Du \cdot I\right )^{2} + \mu \, \mathrm{sym} Du \cdot \mathrm{sym}Du \\ &+\frac{1}{2}k_{1}\left ( N\cdot I\right )^{2} +k_{2}\,\mathrm{sym}N \cdot \mathrm{sym}N+k_{2}^{\prime }\,\mathrm{skw}N\cdot \mathrm{skw}N \\ &+k_{3}\left ( \mathrm{sym}Du \cdot I\right ) \left ( N\cdot I\right ) +k_{3}^{\prime }\,\mathrm{sym}N\cdot \mathrm{sym}Du +\frac{1}{2} \kappa _{0}\left \vert \nu \right \vert ^{2} \end{aligned} $$

where $I$ is here the unit tensor, and the operators $\mathrm{sym}$ and $\mathrm{skw}$ extract, respectively, symmetric and skew-symmetric components of their arguments. $\lambda$ and $\mu$ are the standard Lamé constants, the other coefficients indicated by $k$ with various decorations have constitutive nature. They are such that the energy is positive definite. Specifically, we have $\mu > 0$, $k_{2} > 0$, $2 k_{2} + 3k_{1} > 0$, $k_{2}' > 0$, $k_{3}' < 2\sqrt{\mu k_{2}}$, $3 k_{3} + k_{3}' < \sqrt{(2\mu +3\lambda )(2k_{2}+3k_{1})}$, $\kappa _{0}\geq 0$.

Once restricting to the small strain setting and taking the previous expression of the energy, we eventually get

$$ \begin{aligned} & P\approx \boldsymbol{\sigma} =\lambda \left ( \mathrm{tr}\, ( \mathrm{sym}\nabla u) \right ) I+2\mu \mathrm{sym}\nabla u +k_{3} \left ( \mathrm{tr}\nabla \nu \right ) I+k_{3}^{\prime }\mathrm{sym} \nabla \nu +\epsilon \nabla \dot{u}\;, \\ &z\approx z_{c}=\kappa _{0}\nu +\varsigma \dot{\nu}\;, \\ & \!\! \textstyle\begin{array}{l@{\quad}l} \mathcal{S}\approx \mathcal{S}_{c}= &k_{1}\left ( \mathrm{tr}\nabla \nu \right ) I+2k_{2}\mathrm{sym}\nabla \nu +2k_{2}^{\prime }\mathrm{skw}\nabla \nu +k_{3}\left ( \mathrm{tr}(\mathrm{sym}\nabla u) \right ) I \\ & \qquad \qquad \qquad \qquad \qquad +2k_{2}^{\prime }\mathrm{skw} \nabla \nu +k_{3}\left ( \mathrm{tr}(\mathrm{sym}\nabla u) \right ) I +k_{3}^{ \prime }\mathrm{sym}\nabla u \end{array}\displaystyle \end{aligned} $$

When we insert such constitutive structures in the balance equations, we also set $\xi = \lambda + \mu$, $\bar{\xi}=k_{3} + \frac{1}{2} k'_{3}$, $\zeta = k_{2} + k'_{2}$, $\gamma = k_{1} + k_{2} - k'_{2}$, $\kappa =\frac{1}{2} k'_{3}$.

Eventually, we choose $\beta ^{\ddagger}=0$ and admit a live load $b=\tilde{\gamma}u_{t}$. In our analyses we also consider the case $\tilde{\gamma}=0$, in agreement with the aim expressed in Remark 2.2.

This is the ground from which system (1.1) emerges.

3 Weak Solutions and Energy Decay

For the sake of short-hand notation, from now on we will omit (in most of the cases) to specify a measure under integral signs being sure that the domain of integration will clarify the setting. Similarly, we will also avoid distinguishing by a tilde a function from its value.

Definition 3.1

Weak solutions

We say that a pair $(u,\nu )$ such that

$$\begin{gathered} u \in W^{1,2}(0,T; L^{2}(\mathcal{B})) \cap L^{2}(0,T; W^{1,2}( \mathcal{B})), \\ \nu \in L^{2}(0,T; W^{1,2}(\mathcal{B})), \end{gathered}$$

and

$$\begin{gathered} ( \mathrm{curl}\, u \times n)|_{\partial \mathcal{B}} =( \mathrm{curl}\,\nu \times n)|_{\partial \mathcal{B}} =0\,\, \textrm{ in the weak sense}, \end{gathered}$$

is a weak (distributional) solution of the balance equations (1.1) if, for $T>0$, we have

$$\begin{aligned} & \begin{aligned} -\rho \int _{0}^{T} & \int _{\mathcal{B}} u_{t}\, \cdot w_{t} + \tilde{\gamma}\int _{0}^{T} \int _{\mathcal{B}} u_{t}\, \cdot w + \mu \int _{0}^{T} \int _{\mathcal{B}} \big(\mathrm{curl}\, u \cdot \mathrm{curl}\, w + \mathrm{div} u \cdot \mathrm{div} w\big) \\ &\qquad + \kappa \int _{0}^{T} \int _{\mathcal{B}} \big(\mathrm{curl} \, \nu \cdot \mathrm{curl}\, w + \mathrm{div} \nu \cdot \mathrm{div} w \big) \\ & \quad = -\xi \int _{0}^{T} \int _{\mathcal{B}} \mathrm{div} u \cdot \mathrm{div} w - \bar{\xi}\int _{0}^{T} \int _{\mathcal{B}} \mathrm{div} \nu \cdot \mathrm{div} w, \end{aligned}\!\!\!\!\! \\ & \begin{aligned} - \varsigma \int _{0}^{T} & \int _{\mathcal{B}} \nu \cdot \omega _{t} + \kappa _{0} \int _{0}^{T} \int _{\mathcal{B}} \nu \cdot \omega + \zeta \int _{0}^{T} \int _{\mathcal{B}} \big( \mathrm{curl}\, \nu \cdot \mathrm{curl}\, \omega + \mathrm{div} \nu \cdot \mathrm{div} \omega \big) \\ & \qquad + \kappa \int _{0}^{T} \int _{\mathcal{B}} \big( \mathrm{curl}\, u \cdot \mathrm{curl}\, \omega + \mathrm{div}\, u \cdot \mathrm{div}\, \omega \big) \\ &\quad = -\gamma \int _{0}^{T} \int _{\mathcal{B}} \mathrm{div}\, \nu \cdot \mathrm{div}\, \omega - \bar{\xi}\int _{0}^{T} \int _{\mathcal{B}} \mathrm{div}\,u \cdot \mathrm{div} \,\omega , \end{aligned} \hspace{-0.5 cm} \end{aligned}$$

for every $w, \omega \in C^{\infty}([0,T]\times \mathcal{B})$ satisfying $w(0,\cdot ) = w(T,\cdot ) =\omega (0,\cdot ) = \omega (T,\cdot )=0$ in ℬ, and $w\cdot n = \omega \cdot n =0$ on $[0, T ]\times \partial \mathcal{B}$.

Choices of the initial data will allow us to manage more regular solutions than those in the above definition.

Consider initial data $\text{(1.1)}_{3}$. We need to assume $u_{0}, \nu _{0}\in W^{1,2}(\mathcal{B})$, with $u_{0}$ and $\nu _{0}$ satisfying conditions (1.2), and $\dot{u}_{0}\in W^{1,2}(\mathcal{B})$ with $\dot{u}_{0}\cdot n =0$ on $\partial \mathcal{B}$ (in the weak sense). In this case and in the above conditions, existence and uniqueness of weak solutions to balances (1.1), can be obtained, up to minor changes, by following the same steps in the proof presented in reference [6, Theorem 3.2].

3.1 Main Results

In the sequel, we need improved regularity for the solutions, so we take initial data in higher order Sobolev spaces to prove our results. As a consequence, due to the reached uniqueness (see [6]), weak solutions will be more regular than those in Definition 3.1. Since we assume $(u_{0}, \nu _{0}) \in W^{2,2}(\mathcal{B})$, conditions (1.2) shall be meant in the sense of traces. Also, for $\|\cdot \|_{k,p}$ we mean the usual norm in $W^{k,p}(\mathcal{B})$.

Theorem 3.1

Assume $\tilde{\gamma}> 0$ and $\mu \ge \kappa$, $\zeta \ge \kappa$, $\xi \ge \bar{\xi}$ and $\gamma \ge \bar{\xi}$. Let $u_{0}, \nu _{0}\in W^{2,2}(\mathcal{B})$ satisfy the boundary conditions (1.2), and $\dot{u}_{0}\in W^{1,2}(\mathcal{B})$ with $\dot{u}_{0}\cdot n =0$ on $\partial \mathcal{B}$. Let $(u, \nu )$ be the weak solution of problem (1.1)-(1.2).

Then,

$$ u, \nu \in L^{\infty}(0, +\infty ; W^{2,2}(\mathcal{B})), $$

(3.1)

$$ u_{t} \in C([0,+\infty ); L^{2}\left (\mathcal{B}\right ))\cap L^{ \infty}(0,+\infty ; W^{1,2}(\mathcal{B}))\cap L^{2}(0,+\infty ; W^{1,2}( \mathcal{B})) $$

(3.2)

and

$$ \nu _{t} \in L^{2}(0, +\infty ; W^{1,2}\left (\mathcal{B}\right )). $$

(3.3)

Moreover, we have

$$ \| u_{t} (t)\|^{2}_{1,2} \le \|\dot{u}_{0}\|_{1,2}^{2} e^{- \frac{\tilde{\gamma}}{\rho}t} + K_{1}, $$

(3.4)

where $K_{1}>0$ depends only on the constitutive parameters and on $\|u_{0}\|_{2,2}$, $\|\nu _{0}\|_{2,2}$, and $\|\dot{u}_{0}\|_{1,2}$.

The inclusions (3.1) and (3.3) still hold under the assumption $\tilde{\gamma}=0$, namely when we have no damping term in the balance $\text{(1.1)}_{1}$, while the inclusion (3.2) does not emerge in this case. Indeed, if $\tilde{\gamma}=0$ we only obtain $u_{t} \in L^{\infty}(0,+\infty ; W^{1,2}(\mathcal{B}))$.

Theorem 3.2

Assume $\tilde{\gamma}\ge 0$ and the same assumptions of Theorem 3.1. Then, for $t\geq 0$ and $p> 2$, $(u, \nu )$ verifies

$$ \|u(t)\|^{p}_{p} + \|\nu (t)\|^{p}_{p} \leq \big(\|u_{0}\|_{p}^{p} + \|\nu _{0}\|_{p}^{p}\big) e^{-\Lambda t} + K_{2}, $$

(3.5)

where $\Lambda >0$ depends only on the constitutive parameters, on $p$ and $K_{2}>0$ depends on the constitutive parameters, $p$ and on $\|u_{0}\|_{2,2}$, $\|\nu _{0}\|_{2,2}$, $\|\dot{u}_{0}\|_{1,2}$.

The constant $\Lambda$ accrues from (6.15) and (6.17) below.

As regards $\|u\|_{p}$, we shall use a result on second order differential equations (see Theorem 4.1) in the same spirit of reference [33] where, at variance, the authors considered in a $n$-dimensional ambient space only elliptic regime both in space and time (that is $\mu <0$ in the balance $\text{(1.1)}_{1}$) with a forcing term ($g$ in [33, (0.1)]). For $p>n+1$, a decay behavior for large times that is similar to the inequality (3.5) was obtained but for $\|u\|_{2,p}$ (see [33, (0.6)] where the forcing term is taken of class $L^{p}_{\mathit{loc}}(0,+\infty ; L^{p})$), due to the additional regularity at disposal. Remarkably, the corresponding $K_{2}$ in [33, (0.6)] was only related to the forcing term $g$ and not on the initial data. This fact, in particular, makes the author able to find an attractor, at variance of what we can do here.

4 Notations and Preliminaries to the Decay Analysis

For $p\geq 1$, $L^{p}(\mathcal{B})$ indicates the Lebesgue space of $p$-power summable functions, endowed with norm $\|\cdot \|_{p}$. When $p=2$, we shorten the notation to $\|\cdot \|=\|\cdot \|_{2}$. Moreover, for $k$ a non-negative integer and $p$ as above, $W^{k,p}(\mathcal{B})$ and $\|\cdot \|_{k,p}$ indicate a Sobolev space and its norm, respectively. We write $W^{1,p}_{0}(\mathcal{B})$ for the closure of $C_{0}^{\infty}(\mathcal{B})$ in $W^{1,p}(\mathcal{B})$ and $W^{-1, p'}(\mathcal{B})$, $p' = p/(p -1)$, for its dual endowed with norm $\|\cdot \|_{-1, p'}$.

Let $X$ be a real Banach space with norm $\|\cdot \|_{X}$. We will use the spaces $W^{k,p}(0, T;X)$, with norm denoted by $\|\cdot \|_{W^{k,p}(0,T;X)}$. Specifically, $W^{0,p}(0, T;X)=L^{p}(0, T;X)$ is a standard Bochner space.

Also, with $f$ and $g$ two square-integrable fields defined on ℬ, the symbol $(f\, ,\, g)$ will indicate the standard $L^{2}$-product, i.e., the integral $\int _{\mathcal{B}}f\cdot g$, where the dot means duality pairing, which coincides with the scalar product in orthonormal frames.

We also define the space $\mathcal{H}^{1}$ by

$$\begin{aligned} \mathcal{H}^{1} :=\big\{ v\in W^{1,2}(\mathcal{B})\, :\, ( v \cdot n)_{ \vert _{\partial \mathcal{B}}}= 0\big\} , \end{aligned}$$

equipped with the $W^{1,2}$-norm (denoted by $\|\, \cdot \,\|_{1,2}$) and its dual by $\mathcal{H}^{-1}$. We have analogous definitions for the higher order spaces $\mathcal{H}^{n}$, $n\in \mathbb{N}$, with norms $\|\, \cdot \,\|_{n,2}$, and for $\mathcal{H}^{1/2}$ with norm $\|\, \cdot \, \|_{\frac{1}{2}, 2}$.

In the sequel, positive constants not depending on $(u,\nu )$ but only on the constitutive constants and the initial data will be indicated generically by $c$ or $C$, without further specifications.

4.1 Tools from the Theory of Ordinary Differential Equations

Theorem 4.1

[33, Theorem 1.1]

For given $\ell \in \mathbb{R}$ and $\varpi >0$, let $y(t)$ be a solution of

$$ \left \{ \textstyle\begin{array}{l} y''(t)+2 \ell y'(t) -\varpi ^{2}y(t) =h(t), \,\,\, t\geq 0, \\ y(0)=y_{0}, \end{array}\displaystyle \right . $$

that is bounded as $t \to +\infty$. Assume that

$$ h(t) \in L^{1}_{\mathit{loc}}(\mathbb{R}_{+}),\,\, \textrm{ and }\,\, |h|_{a} := \underset{t\in \mathbb{R}^{+}}{\sup} \|h\|_{L^{1}(t, t+1)}< \infty . $$

(4.1)

Then, the following estimate for $y(t)$ holds true:

$$ y(t) \leq |y_{0}|e^{-\alpha t} + C|h_{-}|_{a},\,\, \textrm{ where }\, \, h_{-}(t) = \max \{-h(t), 0\}, \,\, \textrm{ and } \,\, \alpha > 0. $$

(4.2)

The inclusion (4.1) tells us that $h$ is uniformly $L^{1}$ over compact sets; in short $h\in L^{1}_{\mathit{uloc}}(0,+\infty )$. Also, if $h \in L^{\infty}(0,+\infty )$ then $h$ fulfills (4.1). Further on, we have $\alpha = \ell + \sqrt{\ell ^{2}+\varpi ^{2}}$ (see [33]).

4.2 Poincaré Type Inequalities

Lemma 4.1

[5, Lemma 8.5]

Let $v \in (W^{1,p}(\mathcal{B}))^{3}$, $1 < p < +\infty$ be given, with $\mathcal{B} \subset \mathbb{R}^{3}$ bounded and endowed with Lipschitz boundary $\partial \mathcal{B}$. Then, there exists $C > 0$ such that

$$ \|v\|_{p} \leq C \| \nabla v\|_{p}, \,\, \textit{ for each } \ v \textrm{ such that } (v \cdot n)|_{\partial \mathcal{B}} =0. $$

Further assumptions allow the use of $\|\mathrm{curl}\, v \|_{p}$ and $\|\mathrm{div} v \|_{p}$ to define a semi-norm equivalent to that of $\|\nabla v \|_{p}$, $1< p <+\infty$. We state the next result obtained by von Wahl.

Theorem 4.2

[34, Theorem 3.2]

Let $1 < p < +\infty$ be given. Let ℬ be such that $b_{1}(\mathcal{B}) =0$, i.e. the first Betti number of ℬ vanishes. Then, there exists $C$, which depends only on $p$ and ℬ, such that

$$ \|\nabla v\|_{p}\leq C\big(\|\mathrm{div}\, v\|_{p} +\|\mathrm{curl} \, v \|_{p}\big), $$

(4.3)

for all $v \in (W^{1,p}(\mathcal{B}))^{3}$ satisfying $( v\cdot n)|_{\partial \mathcal{B}} = 0$.

In order to keep the notion concise, in the sequel we avoid to indicate the dimension: we’ll use the more compact notation $W^{k,p}(\mathcal{B})$ in place of $\big(W^{k,p}(\mathcal{B})\big)^{3}$, without inducing confusion.

4.3 A-Priori Estimates

Theorems 3.1-3.2 require for the weak solutions considered here an improved regularity with respect to the one that is implicit in Definition 3.1. Indeed, we lack the needed regularity to test equations directly. So, we proceed formally at a first glance because a suitable Galerkin approximation scheme, as adopted in reference [6], allows us to get the energy estimates above anticipated.

By multiplying the equations in (1.1) by $u_{t}$ and $\nu _{t}$ in $L^{2}(\mathcal{B})$, respectively, due to the relations $u_{t}\cdot n=0$, $\nu _{t}\cdot n=0$, and $\Delta u = \nabla \mathrm{div} u - \mathrm{curl}\,\, \mathrm{curl}\, u$ as well as $\Delta \nu = \nabla \mathrm{div} \nu - \mathrm{curl}\,\, \mathrm{curl}\, \nu$, integration by parts leads to

$$\begin{aligned} &\begin{aligned} \frac{\rho}{2} \frac{\mathrm{d}}{\mathrm{d}t} &\| u_{t} \|^{2} + \frac{\mu}{2}\frac{\mathrm{d}}{\mathrm{d}t}\|\mathrm{curl}\, u\|^{2} + \frac{\mu + \xi}{2}\frac{\mathrm{d}}{\mathrm{d}t}\|\mathrm{div} u\|^{2} + \tilde{\gamma}\|u_{t}\|^{2} \\ & = - \kappa \int _{\mathcal{B}} \mathrm{div} \nu \cdot \mathrm{div} u_{t} - \kappa \int _{\mathcal{B}} \mathrm{curl}\, \nu \cdot \mathrm{curl} \, u_{t} - \bar{\xi}\int _{\mathcal{B}} \mathrm{div} \nu \cdot \mathrm{div} u_{t}, \end{aligned} \\ &\begin{aligned} \varsigma \|\nu _{t}\|^{2} &+ \frac{\kappa _{0}}{2} \frac{\mathrm{d}}{\mathrm{d}t}\|\nu \|^{2} + \frac{\zeta}{2} \frac{\mathrm{d}}{\mathrm{d}t}\|\mathrm{curl}\, \nu \|^{2} + \frac{\zeta + \gamma}{2}\frac{\mathrm{d}}{\mathrm{d}t}\|\mathrm{div} \nu \|^{2} \\ &= - \kappa \int _{\mathcal{B}} \mathrm{div} \,u \cdot \mathrm{div}\, \nu _{t} - \kappa \int _{\mathcal{B}} \mathrm{curl}\, u \cdot \mathrm{curl}\,\nu _{t} - \bar{\xi}\int _{\mathcal{B}} \mathrm{div}\, u \cdot \mathrm{div}\, \nu _{t}. \end{aligned} \end{aligned}$$

Hence, by adding them, we get

$$ \begin{aligned} \frac{\rho}{2} \frac{\mathrm{d}}{\mathrm{d}t} &\| u_{t} \|^{2} + \tilde{\gamma} \|u_{t}\|^{2}+ \varsigma \|\nu _{t}\|^{2} + \frac{\kappa _{0}}{2}\frac{\mathrm{d}}{\mathrm{d}t}\|\nu \|^{2} + \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}\big(\mu \|\mathrm{curl}\, u \|^{2} + \zeta \|\mathrm{curl}\, \nu \|^{2}\big) \\ &\qquad \,\,\, +\frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}\big( (\mu + \xi )\|\mathrm{div} \,u\|^{2} + (\zeta +\gamma ) \|\mathrm{div} \,\nu \|^{2} \big) \\ & = -\kappa \frac{\mathrm{d}}{\mathrm{d}t}\int _{\mathcal{B}} \mathrm{curl}\, u\cdot \mathrm{curl}\, \nu -(\kappa + \bar{\xi}) \frac{\mathrm{d}}{\mathrm{d}t}\int _{\mathcal{B}} \mathrm{div}\, u \cdot \mathrm{div} \,\nu , \end{aligned} $$

(4.4)

so that, integration over $[0, t]$ and the use of Hölder’s and Young’s inequalities imply

$$ \begin{aligned} \frac{\rho}{2} & \|u_{t} \|^{2} + \int _{0}^{t}\Big( \tilde{\gamma} \|u_{t}\|^{2} + \varsigma \|\nu _{t}\|^{2}\Big) + \frac{\kappa _{0}}{2}\|\nu \|^{2} + \Big(\frac{\mu}{2}\|\mathrm{curl}\, u \|^{2} + \frac{\zeta}{2}\|\mathrm{curl}\, \nu \|^{2}\Big) \\ &\qquad \,\,\, + \Big( \frac{\mu +\xi}{2}\|\mathrm{div}\, u\|^{2} + \frac{\zeta +\gamma }{2} \|\mathrm{div}\, \nu \|^{2}\Big) \\ & \leq c_{0} + \frac{\kappa}{2}\|\mathrm{curl}\, u\|^{2} + \frac{\kappa}{2}\|\mathrm{curl}\, \nu \|^{2} + \frac{\kappa + \bar{\xi}}{2}\|\mathrm{div} \,u\|^{2} + \frac{\kappa + \bar{\xi}}{2}\|\mathrm{div} \,\nu \|^{2}, \end{aligned} \hspace{-0.3 cm} $$

with $c_{0}=c(\|u_{0}\|_{1,2}, \|\nu _{0}\|_{1,2}, \|\dot{u}_{0}\|)$ independent on time $t$.

Hence, for every $t \ge 0$, we get

$$ \begin{aligned} \frac{\rho}{2}\|u_{t} \|^{2} + \int _{0}^{t}\Big( \tilde{\gamma}\|u_{t} \|^{2} + \varsigma \|\nu _{t}\|^{2}\Big) &+ \frac{\kappa _{0}}{2}\| \nu \|^{2} + \frac{\mu -\kappa}{2}\|\mathrm{curl}\,u\|^{2} \\ &+ \frac{\mu +\xi -\kappa -\bar{\xi}}{2}\|\mathrm{div}\,u\|^{2} + \frac{\zeta -\kappa}{2}\|\mathrm{curl}\,\nu \|^{2} \\ & + \frac{\zeta +\gamma -\kappa -\bar{\xi}}{2}\|\mathrm{div}\,\nu \|^{2} \leq c_{0}. \end{aligned} \hspace{-0.2 cm} $$

(4.5)

Remark 4.1

Thanks to Theorem 4.2 with $v=u$ and $p=2$, the assumed simple connectedness of ℬ allows us to infer from inequality (4.5) the inclusion $\nabla u \in L^{\infty}(0,+\infty ; L^{2}\left (\mathcal{B}\right ))$. Then, Theorem 4.2 guarantees the inclusion $u \in L^{\infty}(0,+\infty ;\mathcal{H}^{1}\left (\mathcal{B}\right ))$. We have also $\nu \in L^{\infty}(0,+\infty ; L^{2}\left (\mathcal{B}\right ))$ from (4.5). When $p=2$ and $v=\nu$, an analogous reasoning allows us to get from inequality (4.5) the inclusion $\nu \in L^{\infty}(0,+\infty ; \mathcal{H}^{1}\left (\mathcal{B} \right ))$. Moreover, $u_{t}\in L^{2}(0, +\infty ; L^{2}(\mathcal{B}))\cap L^{\infty}(0, + \infty ; L^{2}(\mathcal{B}))$ and, through embedding [1], we get $u\in C([0, +\infty ]; L^{2}(\mathcal{B}))$, since $u_{tt} \in L^{2}_{loc}(0,+\infty ;\mathcal{H}^{-1})$, and $\nu _{t} \in L^{2}(0, +\infty ; L^{2}(\mathcal{B}))$.

Lemma 4.2

Under the regularity assumptions of Theorem 3.1, the inequality

$$ \|u_{t}(t)\|^{2}\le \|\dot{u}_{0}\|^{2} e^{-\frac{2\tilde{\gamma}}{\rho}t} + C, $$

(4.6)

holds true for some $C>0$ which, up to scaling by a constant, is $c_{0}$, as in the inequality (4.5).

Proof

From (4.4) we have, for every $t \ge 0$,

$$ \begin{aligned} \frac{\rho}{2} \frac{\mathrm{d}}{\mathrm{d}t} &\| u_{t} \|^{2} + \tilde{\gamma} \|u_{t}\|^{2} \le \\ & - \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}\big(\mu \|\mathrm{curl} \, u\|^{2} - \zeta \|\mathrm{curl}\, \nu \|^{2}\big) +\frac{1}{2} \frac{\mathrm{d}}{\mathrm{d}t}\big( (\mu +\xi )\|\mathrm{div}\, u\|^{2} + (\zeta +\gamma ) \|\mathrm{div} \,\nu \|^{2}\big) \\ & -\kappa \frac{\mathrm{d}}{\mathrm{d}t}\int _{\mathcal{B}} \mathrm{curl}\, u\cdot \mathrm{curl}\, \nu -(\kappa + \bar{\xi}) \frac{\mathrm{d}}{\mathrm{d}t}\int _{\mathcal{B}} \mathrm{div}\, u \cdot \mathrm{div} \,\nu , \end{aligned} $$

(4.7)

which is

$$ \frac{\rho}{2}\frac{\mathrm{d}}{\mathrm{d}t}\|u_{t} \|^{2} + \tilde{\gamma} \|u_{t}\|^{2} \le \frac{\mathrm{d}}{\mathrm{d}t} \omega (t)=:\dot{\omega}(t), $$

where $\omega$ is clearly deduced by the right-hand side of the inequality (4.7) and is of class $L^{\infty}(0,+\infty )$, in virtue of the estimate (4.5). Hence, by direct computations, we obtain

$$ \begin{aligned} e^{\frac{2\tilde{\gamma}}{\rho}t}\|u_{t}\|^{2}-\|\dot{u}_{0}\|^{2}&\le \int _{0}^{t} e^{\frac{2\tilde{\gamma}}{\rho}s} \dot{\omega}(s)\, \mathrm{d}s \\ &= \omega (t)e^{\frac{2\tilde{\gamma}}{\rho}t} - \omega (0)- \frac{2\tilde{\gamma}}{\rho}\int _{0}^{t} \omega (s) e^{ \frac{2\tilde{\gamma}}{\rho}s}\,\mathrm{d}s. \end{aligned} $$

Hence, for every $t\ge 0$,

$$ \|u_{t}\|^{2}(t) \le \|\dot{u}_{0}\|^{2} e^{- \frac{2\tilde{\gamma}}{\rho}t} + \omega (t)-\omega (0)e^{- \frac{2\tilde{\gamma}}{\rho}t} -\frac{2\tilde{\gamma}}{\rho}e^{- \frac{2\tilde{\gamma}}{\rho}t}\int _{0}^{t} \omega (s) e^{ \frac{2\tilde{\gamma}}{\rho}s}\,ds, $$

which implies the inequality (4.6). □

5 Higher-Order Estimates

By multiplying the first equation in (1.1) by $\Delta u_{t}$ in $L^{2}(\mathcal{B})$, and using the boundary conditions (1.2), we find

$$\begin{aligned} \int _{\mathcal{B}}u_{tt}\cdot \Delta u_{t} = & \int _{\mathcal{B}} u_{tt} \cdot \big( \nabla \mathrm{div} u_{t} - \mathrm{curl}\,\, \mathrm{curl}\, u_{t}\big) \\ = & -\int _{\mathcal{B}}\mathrm{div}\, u_{tt}\cdot \mathrm{div}\, u_{t} + \int _{\partial \mathcal{B}} \mathrm{div}\, u_{t} (u_{tt} \cdot n) \\ &- \int _{\mathcal{B}}\mathrm{curl}\, u_{tt} \cdot \mathrm{curl}\, u_{t} + \int _{\partial \mathcal{B}} \mathrm{curl}\, u_{t} \times n \cdot u_{tt} \\ = & -\frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t} \|\mathrm{div}\, u_{t}\|^{2} - \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}\|\mathrm{curl}\, u_{t}\|^{2}. \end{aligned}$$

Indeed, the boundary condition (1.2) imply

$$ \int _{\partial \mathcal{B}} \mathrm{div} u_{t} (u_{tt} \cdot n)= \int _{\partial \mathcal{B}} \mathrm{curl}\, u_{t} \times n \cdot u_{tt} =0. $$

Similarly, we have

$$ \int _{\mathcal{B}} u_{t} \cdot \Delta u_{t} = - \|\mathrm{curl}\, u_{t} \|^{2}- \|\mathrm{div} u_{t}\|^{2}, $$

so that we may compute

$$\begin{aligned} \int _{\mathcal{B}} \nabla \mathrm{div}\, u \cdot \Delta u_{t} = & \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t} \|\nabla \mathrm{div} u\|^{2} + \int _{\partial \mathcal{B}} \mathrm{curl}\, u_{t} \times n \cdot \nabla \mathrm{div} u \\ = &\frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t} \|\nabla \mathrm{div}\, u \|^{2} \end{aligned}$$

and

$$\begin{aligned} \int _{\mathcal{B}} \nabla \mathrm{div} \,\nu \cdot \Delta u_{t} = & \int _{\mathcal{B}}\nabla \mathrm{div}\, \nu \cdot \nabla \mathrm{div}\, u_{t}+ \int _{\partial \mathcal{B}} \mathrm{curl}\, u_{t} \times n \cdot \nabla \mathrm{div}\, \nu \\ = & \int _{\mathcal{B}}\nabla \mathrm{div}\, \nu \cdot \nabla \mathrm{div}\, u_{t}. \end{aligned}$$

Hence, we get

$$\begin{aligned} &\begin{aligned} \frac{\rho}{2}\frac{\mathrm{d}}{\mathrm{d}t}\| &\mathrm{curl}\, u_{t} \|^{2} + \frac{\rho}{2}\frac{\mathrm{d}}{\mathrm{d}t}\| \mathrm{div}\, u_{t} \|^{2} + \frac{\mu}{2}\frac{\mathrm{d}}{\mathrm{d}t}\|\Delta u\|^{2} \\ & + \tilde{\gamma}\| \mathrm{curl}\, u_{t} \|^{2} + \tilde{\gamma}\| \mathrm{div}\, u_{t} \|^{2}= -\frac{\xi}{2} \frac{\mathrm{d}}{\mathrm{d}t} \| \nabla \mathrm{div}\, u \|^{2} \\ &- \kappa \int _{\mathcal{B}} \Delta \nu \cdot \Delta u_{t} - \bar{\xi}\int _{\mathcal{B}} \nabla \mathrm{div}\, \nu \cdot \nabla \mathrm{div}\, u_{t}. \end{aligned} \end{aligned}$$

(5.1)

Then, by multiplying in $L^{2}(\mathcal{B})$ the second equation in system (1.1) by $\Delta \nu _{t}$, since

$$ \int _{\mathcal{B}}\nu \cdot \Delta \nu _{t} = -\frac{1}{2} \frac{\mathrm{d}}{\mathrm{d}t}\|\mathrm{curl}\, \nu \|^{2} - \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}\|\mathrm{div}\, \nu \|^{2}, $$

we get

$$\begin{aligned} &\begin{aligned} \varsigma \|\mathrm{curl}\, &\,\nu _{t}\|^{2} + \varsigma \| \mathrm{div} \,\nu _{t}\|^{2} + \frac{\kappa _{0}}{2} \frac{\mathrm{d}}{\mathrm{d}t}\|\mathrm{curl}\, \nu \|^{2} + \frac{\kappa _{0}}{2}\frac{\mathrm{d}}{\mathrm{d}t}\|\mathrm{div}\, \nu \|^{2} + \frac{\zeta}{2}\frac{\mathrm{d}}{\mathrm{d}t}\|\Delta \nu \|^{2}= \\ & - \frac{\gamma}{2}\frac{\mathrm{d}}{\mathrm{d}t} \| \nabla \mathrm{div}\, \nu \|^{2} - \kappa \int _{\mathcal{B}} \Delta u \cdot \Delta \nu _{t} - \bar{\xi}\int _{\mathcal{B}} \nabla \mathrm{div} \,u \cdot \nabla \mathrm{div}\, \nu _{t}. \end{aligned} \end{aligned}$$

(5.2)

By adding the two identities (5.1) and (5.2), we obtain

$$\begin{aligned} &\begin{aligned} \frac{\rho}{2}&\frac{\mathrm{d}}{\mathrm{d}t}\left (\| \mathrm{curl} \, u_{t} \|^{2} + \| \mathrm{div}\, u_{t} \|^{2}\right ) + \frac{\mu}{2}\frac{\mathrm{d}}{\mathrm{d}t}\|\Delta u\|^{2} + \frac{\zeta}{2}\frac{\mathrm{d}}{\mathrm{d}t} \|\Delta \nu \|^{2} \\ & + \tilde{\gamma}\left (\| \mathrm{curl}\, u_{t} \|^{2} +\| \mathrm{div}\, u_{t}\|^{2}\right ) + \varsigma \left ( \|\mathrm{curl}\, \nu _{t}\|^{2} + \|\mathrm{div}\, \nu _{t}\|^{2}\right ) \\ &+ \frac{\kappa _{0}}{2}\frac{\mathrm{d}}{\mathrm{d}t}\left (\| \mathrm{curl}\, \nu \|^{2} + \|\mathrm{div} \,\nu \|^{2} \right ) + \frac{\xi}{2} \frac{\mathrm{d}}{\mathrm{d}t} \| \nabla \mathrm{div}\, u \|^{2} + \frac{\gamma}{2} \frac{\mathrm{d}}{\mathrm{d}t} \| \nabla \mathrm{div}\, \nu \|^{2} \\ =& - \kappa \frac{\mathrm{d}}{\mathrm{d}t} \int _{\mathcal{B}} \Delta \nu \cdot \Delta u - \bar{\xi}\frac{\mathrm{d}}{\mathrm{d}t} \int _{\mathcal{B}} \nabla \mathrm{div} \nu \cdot \nabla \mathrm{div} \,u. \end{aligned} \end{aligned}$$

(5.3)

For every $t\ge 0$, integration on $(0, t)$ gives

$$ \begin{aligned} \frac{\rho}{2} &\big(\| \mathrm{curl}\, u_{t} \|^{2} + \| \mathrm{div}\, u_{t} \|^{2}\big) + \frac{\kappa _{0}}{2} \big(\| \mathrm{curl}\, \nu \|^{2} + \|\mathrm{div}\, \nu \|^{2}\big) \\ &+ \frac{\mu -\kappa}{2} \|\Delta u\|^{2} + \frac{\zeta -\kappa}{2} \|\Delta \nu \|^{2}+ \frac{\xi -\bar{\xi}}{2}\|\nabla \mathrm{div}\,u\|^{2} + \frac{\gamma -\bar{\xi}}{2} \|\nabla \mathrm{div}\,{\nu}\|^{2} \\ &+ \varsigma \int _{0}^{t} \big(\| \mathrm{curl}\,\nu _{t}\|^{2}+ \| \mathrm{div}\, \nu _{t}\|^{2})+ \tilde{\gamma} \int _{0}^{t}\big( \| \mathrm{curl}\, u_{t}\|^{2} +\| \mathrm{div}\, u_{t}\|^{2}\big) \leq C_{0}, \end{aligned} \hspace{-0.6 cm} $$

(5.4)

with $C_{0}=c(\|u_{0}\|_{2,2}, \|\nu _{0}\|_{2,2}, \|\dot{u}_{0}\|_{1,2})$ a bound from above for $c_{0}$ in the estimate (4.5).

Remark 5.1

Assuming that $u_{0}$ and $\nu _{0}$ are bounded in $\mathcal{H}^{2}(\mathcal{B})$, they satisfy the boundary conditions (1.2), and $\dot{u}_{0}\in \mathcal{H}^{1}(\mathcal{B})$ with $\dot{u}_{0}\cdot n =0$ on $\partial \mathcal{B}$ in weak sense, implies $\Delta u, \Delta \nu \in L^{\infty}(0, +\infty ; L^{2}(\mathcal{B}))$ and, using Theorem 4.2 and Lemma 4.1, $\nabla u_{t} \in L^{\infty}(0, +\infty ; L^{2}(\mathcal{B}))\cap L^{2}(0, +\infty ;L^{2}(\mathcal{B}))$ and $\nabla \nu _{t} \in L^{2}(0, +\infty ; L^{2}(\mathcal{B}))$.

Remark 5.2

Since $u$ and $\nu$ are bounded in $L^{\infty}(0, T; \mathcal{H}^{2}(\mathcal{B}))$, Sobolev’s embeddings imply directly the boundedness of $u$ and $\nu$ in any $L^{\infty}(0, T; L^{p}(\mathcal{B}))$ for any $1\leq p\leq \infty$.

Lemma 5.1

Under the regularity assumptions of Theorem 3.1, the following inequality

$$ \|\nabla u_{t} (t) \|^{2} \le c \|\nabla u_{0}\|^{2} e^{- \frac{2\tilde{\gamma}}{\rho}t} +C $$

(5.5)

holds true. The constant $c$ depends on ℬ as the one on the right-hand side of the inequality (4.3) and $C$, up to scaling by a constant, is $C_{0}$ in the inequality (5.4).

Proof

From the identity (5.3), we obtain

$$\begin{aligned} &\begin{aligned} \frac{\rho}{2}&\frac{\mathrm{d}}{\mathrm{d}t}\left (\| \mathrm{curl} \, u_{t} \|^{2} + \| \mathrm{div}\, u_{t} \|^{2}\right ) + \tilde{\gamma}\left (\| \mathrm{curl}\, u_{t} \|^{2} +\| \mathrm{div}\, u_{t} \|^{2}\right ) \\ \le &- \frac{\kappa _{0}}{2}\frac{\mathrm{d}}{\mathrm{d}t}\left (\| \mathrm{curl}\, \nu \|^{2} - \|\mathrm{div}\, \nu \|^{2} \right ) + \frac{\xi}{2} \frac{\mathrm{d}}{\mathrm{d}t} \| \nabla \mathrm{div}\, u \|^{2} - \frac{\gamma}{2} \frac{\mathrm{d}}{\mathrm{d}t} \| \nabla \mathrm{div}\, \nu \|^{2} \\ =&- \frac{\mu}{2}\frac{\mathrm{d}}{\mathrm{d}t}\|\Delta u\|^{2} - \frac{\zeta}{2}\frac{\mathrm{d}}{\mathrm{d}t} \|\Delta \nu \|^{2} \\ & - \kappa \frac{\mathrm{d}}{\mathrm{d}t} \int _{\mathcal{B}} \Delta \nu \cdot \Delta u - \bar{\xi}\frac{\mathrm{d}}{\mathrm{d}t} \int _{ \mathcal{B}} \nabla \mathrm{div}\, \nu \cdot \nabla \mathrm{div} \,u=: \frac{\mathrm{d}}{\mathrm{d}t} \omega _{2}(t), \end{aligned} \end{aligned}$$

where $\omega _{2}$ is bounded in virtue of the inequality (5.4). Hence, by proceeding as in the proof of Lemma 4.2, for every $t \ge 0$ we obtain

$$ \|\nabla u_{t}(t) \|^{2} \le c \left (\|\mathrm{curl} \,\dot{u}_{0}\|^{2} + \|\mathrm{div} \,\dot{u}_{0}\|^{2}\right ) e^{- \frac{2\tilde{\gamma}}{\rho}t} +C, $$

which implies the result (5.5). □

We have now the ingredients to prove Theorem 3.1.

Proof of Theorem 3.1

The regularity claimed in (3.1), (3.2) and (3.3) are proved putting together Remarks 4.1 and 5.1.

The decay estimate in (3.4) instead follows from Lemmas 4.2 and 5.1, with $K_{1}$ given by $C_{0}$. □

Remark 5.3

We claim that $\Delta u, \Delta \nu \in L^{2} (0,+\infty ; L^{2}(\mathcal{B}))$. By multiplying by $\Delta u$ the first equation in (1.1) and integrating in both space and time, we obtain

$$\begin{aligned} &\begin{aligned} \int _{0}^{t} \int _{\mathcal{B}} u_{tt}\cdot \Delta u &= -\int _{0}^{t} \int _{\mathcal{B}}(\mathrm{div} \,u_{tt}) (\mathrm{div}\,u) - \int _{0}^{t} \int _{\mathcal{B}} (\mathrm{curl}\,u_{tt})\cdot (\mathrm{curl}\, u) \\ &= -\int _{\mathcal{B}}(\mathrm{div}\, u_{t})(\mathrm{div}\, u) -\int _{ \mathcal{B}} (\mathrm{curl}\, u_{t})\cdot (\mathrm{curl} \, u) + \int _{\mathcal{B}}(\mathrm{div}\,\dot{u}_{0})(\mathrm{div}\, u_{0}) \\ &+\int _{\mathcal{B}} (\mathrm{curl} \, \dot{u}_{0})\cdot ( \mathrm{curl}\, u_{0}) + \int _{0}^{t} \left (\|\mathrm{div} \, u_{t} \|^{2}+\|\mathrm{curl}\, u_{t}\|^{2}\right ), \end{aligned} \end{aligned}$$

which is of class $L^{\infty}(0,+\infty )$, by means of (5.4). Also,

$$ \int _{0}^{t} \int _{\mathcal{B}} u_{t} \cdot \Delta u = -\frac{1}{2} \left (\|\mathrm{div}\, u\|^{2}+ \|\mathrm{curl}\, u\|^{2}\right ), $$

which is bounded from (4.5). We also have

$$ \begin{aligned} -\xi \int _{0}^{t} \int _{\mathcal{B}} \nabla (\mathrm{div}\,u) \cdot \Delta u -&\bar{\xi}\int _{0}^{t} \int _{\mathcal{B}} \nabla ( \mathrm{div}\,\nu ) \cdot \Delta u \\ &=-\xi \int _{0}^{t} \|\nabla (\mathrm{div}\,u)\|^{2}+\bar{\xi}\int _{0}^{t} \int _{\mathcal{B}}\nabla (\mathrm{div}\,u) \cdot \nabla ( \mathrm{div}\,\nu ), \end{aligned} $$

and

$$ \begin{aligned} -\xi \int _{0}^{t} \int _{\mathcal{B}} \nabla (\mathrm{div}\,u) \cdot \Delta u -&\bar{\xi}\int _{0}^{t} \int _{\mathcal{B}} \nabla ( \mathrm{div}\,\nu ) \cdot \Delta u -\kappa \int _{0}^{t} \int _{ \mathcal{B}}\Delta \nu \cdot \Delta u \\ &\le - \xi \int _{0}^{t} \|\nabla (\mathrm{div}\,u)\|^{2}+ \frac{\bar{\xi}}{2} \int _{0}^{t} \|\nabla (\mathrm{div}\,u)\|^{2} \\ &+ \frac{\bar{\xi}}{2}\int _{0}^{t} \| \nabla (\mathrm{div}\,\nu )\|^{2} + \frac{\kappa}{2} \int _{0}^{t} \|\Delta \nu \|^{2} + \frac{\kappa}{2}\int _{0}^{t} \|\Delta u\|^{2}. \end{aligned} $$

Hence, from the previous estimates we get

$$\begin{aligned} &\begin{aligned} \left (\mu - \frac{\kappa}{2}\right ) \int _{0}^{t} \|& \Delta u\|^{2} -\frac{\kappa}{2} \int _{0}^{t} \|\Delta \nu \|^{2} \\ &+\left (\xi -\frac{\bar{\xi}}{2}\right ) \int _{0}^{t} \|\nabla ( \mathrm{div}\,u)\|^{2} -\frac{\bar{\xi}}{2} \int _{0}^{t} \|\nabla ( \mathrm{div}\,\nu )\|^{2} \le C_{0} \end{aligned} \end{aligned}$$

(5.6)

where $C_{0}$ is given in (5.4).

Analogously, by testing (1.1)₂ in $L^{2}(\mathcal{B}\times (0,t))$ by $-\Delta \nu$, we get

$$\begin{aligned} \begin{aligned} \frac{\varsigma}{2}\big(\|\mathrm{div}\,\nu \|^{2}+&\|\mathrm{curl}\, \nu \|^{2}\big) + \kappa _{0}\int _{0}^{t} \left (\|\mathrm{div}\, \nu \|^{2}+\|\mathrm{curl}\, \nu \|^{2} \right ) \\ &+ \left (\zeta -\frac{\kappa}{2}\right ) \int _{0}^{t} \|\Delta \nu \|^{2} -\frac{\kappa}{2} \int _{0}^{t} \|\Delta u\|^{2} \\ &+\left (\gamma - \frac{\bar{\xi}}{2}\right ) \int _{0}^{t} \|\nabla ( \mathrm{div}\,\nu )\|^{2} - \frac{\bar{\xi}}{2} \int _{0}^{t} \|\nabla ( \mathrm{div}\,u)\|^{2} \\ &\le \frac{\varsigma}{2}\left ( \|\mathrm{div}\, \nu _{0}\|^{2}+\| \mathrm{curl}\,\nu _{0}\|^{2}\right ), \end{aligned} \end{aligned}$$

(5.7)

so that, by adding (5.6) and (5.7), we deduce, for every $t \ge 0$,

$$\begin{aligned} &\begin{aligned} \frac{\varsigma}{2}\left (\|\mathrm{div}\,\nu \|^{2}+\|\mathrm{curl} \, \nu \|^{2}\right ) +& \kappa _{0}\int _{0}^{t} \left (\| \mathrm{div}\,\nu \|^{2}+\|\mathrm{curl}\, \nu \|^{2} \right ) \\ & (\mu -\kappa )\int _{0}^{t} \|\Delta u\|^{2} + (\zeta - \kappa ) \int _{0}^{t} \|\Delta \nu \|^{2} \\ &+ (\xi -\bar{\xi}) \int _{0}^{t} \|\nabla (\mathrm{div}\,u)\|^{2} \\ &+ (\gamma -\bar{\xi}) \int _{0}^{t} \|\nabla (\mathrm{div}\,\nu )\|^{2} \le C_{0}. \end{aligned} \end{aligned}$$

This last estimate proves the claim.

6 Proof of Theorem 3.2

In the following we leave explicit a number of entities, which are however bounded in view of the regularity obtained in the previous sections, in order to keep the provided energy estimates as sharp as possible.

Fix $2 < p <+\infty$. By multiplying $\text{(1.1)}_{1}$ by $u|u|^{p-2}$ and integrating over ℬ, we get

$$\begin{aligned} &\begin{aligned} \rho \big(u_{tt}, u|u|^{p-2}\big) + & \tilde{\gamma}(\partial _{t} u, u|u|^{p-2}) - \frac{4\mu (p-2)}{p^{2}} \|u\|^{p}_{p} \\ &\qquad + \frac{4 \mu (p-2)}{p^{2}} \big\| |u|^{\frac{p}{2}}\big\| _{1,2}^{2} + \frac{\mu}{2}\int _{\mathcal{B}} |u|^{p-2} |\nabla u|^{2} \\ & = \mu \bigg(\int _{\partial \mathcal{B}}|u|^{p-2}(u\cdot \nabla ) u \cdot n \bigg)-\kappa \big(\nabla \nu , \nabla \big(u|u|^{p-2}\big)\big) \\ & \qquad + \kappa \bigg(\int _{\partial \mathcal{B}}|u|^{p-2}(u\cdot \nabla ) \nu \cdot n\bigg) -\overline{\xi }\big(\mathrm{div}\, \nu , \mathrm{div} \,\big(u|u|^{p-2}\big)\big) \\ & \qquad - \xi \big(\mathrm{div} u, \mathrm{div} \big(u|u|^{p-2}\big) \big), \end{aligned} \hspace{-1 cm} \end{aligned}$$

(6.1)

where we made use of $u\cdot n=0$ on $\partial \mathcal{B}$, to give

$$ \bar{\xi}\int _{\partial \mathcal{B}}|u|^{p-2} \mathrm{div}\, \nu (u \cdot n) +\xi \int _{\partial \mathcal{B}}|u|^{p-2} \mathrm{div} \,u (u \cdot n) =0. $$

Moreover, by multiplying $\text{(1.1)}_{2}$ by $\nu |\nu |^{p-2}$ and integrating on ℬ, we obtain

$$\begin{aligned} &\begin{aligned} \varsigma \big(\nu _{t}, & \nu |\nu |^{p-2}\big) + \kappa _{0} \|\nu \|_{p}^{p} + \frac{4\zeta (p-2)}{p^{2}} \big\| \nabla |\nu |^{ \frac{p}{2}}\big\| ^{2} + \frac{\zeta}{2}\int _{\mathcal{B}} |\nu |^{p-2} |\nabla \nu |^{2} \\ & = \zeta \bigg(\int _{\partial \mathcal{B}}|\nu |^{p-2}(\nu \cdot \nabla ) \nu \cdot n \bigg) -\overline{\xi}\big(\mathrm{div}\, u, \mathrm{div}\, \big( \nu |\nu |^{p-2}\big)\big) \\ & \qquad - \kappa \big(\nabla u, \nabla \big(\nu |\nu |^{p-2}\big) \big) + \kappa \bigg(\int _{\partial \mathcal{B}}|\nu |^{p-2}(\nu \cdot \nabla ) u \cdot n \bigg) \\ &\qquad - \gamma \big(\mathrm{div}\, \nu , \mathrm{div}\, \big(\nu |\nu |^{p-2} \big)\big). \end{aligned} \hspace{-1 cm} \end{aligned}$$

(6.2)

The identities

$$ \frac{\mathrm{d}}{\mathrm{d}t}\|u(t)\|_{p}^{p} = \frac{\mathrm{d}}{\mathrm{d}t}\||u(t)|^{\frac{p}{2}}\|^{2} = p\big( \partial _{t} u(t), u(t)|u(t)|^{p-2}\big) $$

and

$$ \frac{\mathrm{d}^{2}}{\mathrm{d}t^{2}} \|u (t)\|_{p}^{p} = p\big( \partial ^{2}_{t} u, u|u|^{p-2}\big) +p\big\| \partial _{t} u | u|^{ \frac{p-2}{2}}\big\| ^{2} + p(p-2)\int _{\mathcal{B}} |u|^{p-4 }( \partial _{t} u \cdot u)^{2}, $$

allow us to express the balance (6.1) as

$$\begin{aligned} \begin{aligned} \frac{\rho}{p} \frac{\mathrm{d}^{2}}{\mathrm{d}t^{2}} &\| u\|^{p}_{p} + \frac{\tilde{\gamma}}{p} \frac{\mathrm{d}}{\mathrm{d}t}\| u\|^{p}_{p} - \frac{4 \mu (p-2)}{p^{2}} \|u\|^{p}_{p} \\ &\qquad +\frac{4 \mu (p-2)}{p^{2}} \big\| |u|^{\frac{p}{2}}\big\| _{1,2}^{2} + \frac{\mu}{2}\int _{\mathcal{B}} |u|^{p-2} |\nabla u|^{2} \\ = & \rho \big\| \partial _{t} u | u|^{(p-2)/2}\big\| ^{2} + \rho (p-2) \int _{\mathcal{B}} |u|^{p-4 }(\partial _{t} u \cdot u)^{2} \\ & \qquad -\overline{\xi }\big(\mathrm{div} \nu , \mathrm{div} \big(u|u|^{p-2} \big)\big) -\kappa \big(\nabla \nu , \nabla \big(u|u|^{p-2}\big)\big) \\ & \qquad - \xi \big(\mathrm{div} u, \mathrm{div} \big(u|u|^{p-2}\big) \big) + \Gamma ^{p}_{u}, \end{aligned} \end{aligned}$$

(6.3)

where $\Gamma ^{p}_{u}$ indicates boundary terms. Analogously, equation (6.2) can be rephrased as

$$\begin{aligned} \begin{aligned} \frac{\varsigma}{p} \frac{\mathrm{d}}{\mathrm{d}t} &\|\nu \|^{p}_{p} + \kappa _{0}\|\nu \|_{p}^{p} + \frac{4\zeta (p-2)}{p^{2}} \big\| \nabla |\nu |^{\frac{p}{2}}\big\| ^{2} + \frac{\zeta}{2}\int _{ \mathcal{B}} |\nu |^{p-2} |\nabla \nu |^{2} \\ = & \, -\overline{\xi}\big(\mathrm{div} u, \mathrm{div} \big(\nu | \nu |^{p-2}\big)\big) - \kappa \big(\nabla u, \nabla \big(\nu |\nu |^{p-2} \big)\big) \\ & - \gamma \big(\mathrm{div} \nu , \mathrm{div} \big(\nu |\nu |^{p-2} \big)\big) + \Gamma ^{p}_{\nu}, \end{aligned} \end{aligned}$$

(6.4)

where $\Gamma ^{p}_{\nu}$ indicates boundary terms.

6.1 Decay Estimate for $\|\nu \|_{p}^{p}$

The first term on the right-hand side of equation (6.4) can be estimated as

$$ \begin{aligned} \bar{\xi}\left |\big(\mathrm{div} u, \mathrm{div}\, \big(\nu |\nu |^{p-2} \big)\big)\right | &=\bar{\xi}\left |\big(\partial _{i} u_{i}, \partial _{i} \big(\nu |\nu |^{p-2}\big)_{i}\big) \right | \\ &= \bar{\xi}\left |\big(\partial _{i} u_{i}, \partial _{i} \nu |\nu |^{p-2} + (p-2)\nu _{i} |\nu |^{p-4}\partial _{i}\nu \cdot \nu \big)\right | \\ &\leq C \left |\big( \partial _{i} u_{i}, \partial _{i} \nu |\nu |^{p-2} \big)\right | \\ &=C \left |\big( \partial _{i} u_{i} |\nu |^{\frac{p-2}{2}}, \partial _{i} \nu _{i} |\nu |^{\frac{p-2}{2}} \big)\right | \\ &\leq \epsilon \|\nabla \nu |\nu |^{\frac{p-2}{2}}\|^{2} + c_{ \epsilon }\|\nabla u |\nu |^{\frac{p-2}{2}}\|^{2}. \end{aligned} $$

For the second term, we have

$$ \begin{aligned} \|\nabla u |\nu |^{\frac{p-2}{2}}\|^{2}\leq &\|\nabla u\|_{L^{4}}^{2} \| |\nu |^{\frac{p-2}{2}}\|_{L^{4}}^{2} \\ \leq & C \|\nabla u\|_{L^{4}}^{2} \| |\nu |^{\frac{p}{2}}\|_{L^{4}}^{ \frac{2(p-2)}{p}} \\ \leq & C \| \nabla u\|^{\frac{1}{2}}\| \Delta u\|^{\frac{3}{2}}\| | \nu |^{\frac{p}{2}}\|_{L^{4}}^{\frac{2(p-2)}{p}} \\ \leq & C \| \nabla u\|^{\frac{1}{2}}\| \Delta u\|^{\frac{3}{2}}\| | \nu |^{\frac{p}{2}}\|^{\frac{(p-2)}{2p}}\| \nabla |\nu |^{\frac{p}{2}} \|^{\frac{3(p-2)}{2p}} \\ \leq & C \| \nabla u\|^{\frac{1}{2}}\| \Delta u\|^{\frac{3}{2}} \big( \| |\nu |^{\frac{p}{2}}\|^{\frac{2(p-2)}{p}} + \| \nabla |\nu |^{ \frac{p}{2}}\|^{\frac{2(p-2)}{p}}\big) \\ = & C \| \nabla u\|^{\frac{1}{2}}\| \Delta u\|^{\frac{3}{2}} \| \nu \|_{p}^{(p-2)} \\ &\quad + C \| \nabla u\|^{\frac{1}{2}}\| \Delta u\|^{\frac{3}{2}}\| \nabla |\nu |^{\frac{p}{2}}\|^{\frac{2(p-2)}{p}} =: \tilde{I}_{1} + \tilde{I}_{2}, \end{aligned} \hspace{-1 cm} $$

(6.5)

where we used Gagliardo-Nirenberg’s and Young’s inequalities omitting, as we do below, the lower order terms, that however can be controlled on the left-hand side with the same type of computation.

Since

$$ \tilde{I}_{1}\leq \varepsilon \| \nu \|_{p}^{p} + C_{\varepsilon} \| \nabla u\|^{\frac{p}{4}}\| \Delta u\|^{\frac{3p}{4}}\,\, \text{ and } \,\, \tilde{I}_{2} \leq \delta \|\nabla |\nu |^{\frac{p}{2}}\|^{2} + C_{ \delta}\|\nabla u\|^{\frac{p}{4}}\|\Delta u\|^{\frac{3p}{4}} $$

(6.6)

from the inequality (6.5) we obtain

$$ \|\nabla u |\nu |^{\frac{p-2}{2}}\|^{2}\leq \varepsilon \| \nu \|_{p}^{p} + \delta \|\nabla |\nu |^{\frac{p}{2}}\|^{2} + C_{\delta , \varepsilon}\|\nabla u\|^{\frac{p}{4}}\|\Delta u\|^{\frac{3p}{4}} $$

and

$$ \begin{aligned} \bar{\xi}\left |\big(\mathrm{div} \nu , \mathrm{div} \big(\nu |\nu |^{p-2} \big)\big) \right | \leq \epsilon \int _{\mathcal{B}} |\nu |^{p-2} | \nabla \nu |^{2} &+ \varepsilon \| \nu \|_{p}^{p} + \delta \|\nabla | \nu |^{\frac{p}{2}}\|^{2} \\ & +C_{\delta , \varepsilon , \epsilon}\|\nabla u\|^{\frac{p}{4}}\| \Delta u\|^{\frac{3p}{4}}. \end{aligned} $$

(6.7)

In (6.6) and in the next, the values of $\varepsilon$ and $\delta$ can be chosen arbitrary small. Depending on them, Young’s inequality gives $C_{\varepsilon}$ and $C_{\delta}$.

The second addendum in the right-hand side of (6.4) can be controlled exactly as done above, that is

$$ \kappa \left |\big(\nabla u, \nabla \big(\nu |\nu |^{p-2}\big)\big) \right | \le \varepsilon \| \nabla \nu |\nu |^{\frac{p-2}{2}}\|^{2} +c_{ \varepsilon }\|\nabla u |\nu |^{\frac{p-2}{2}}\|^{2}. $$

Also, by using again the estimate (6.5) (and substituting $u$ with $\nu$), we get

$$ \begin{aligned} \gamma \left |\big(\mathrm{div} \nu , \mathrm{div} \big(\nu |\nu |^{p-2} \big)\big) \right |& \leq \gamma \|\mathrm{div} \nu |\nu |^{ \frac{p-2}{2}}\|^{2} +\gamma (p-2)\int _{\mathcal{B}} |\mathrm{div} \nu | |\nabla \nu | |\nu |^{p-2} \\ & \leq \gamma (p-1)\| \nabla \nu |\nu |^{\frac{p-2}{2}}\|^{2} \\ & \leq \varepsilon \| \nu \|_{p}^{p} + \delta \|\nabla |\nu |^{ \frac{p}{2}}\|^{2} + C_{\delta , \varepsilon}\|\nabla \nu \|^{ \frac{p}{4}}\|\Delta \nu \|^{\frac{3p}{4}}. \end{aligned} $$

(6.8)

Consider the boundary terms in $\Gamma _{\nu}^{p}$. We compute

$$ \begin{aligned} \left |\int _{\partial \mathcal{B}} |\nu |^{p-2} (\nu \cdot \nabla ) \nu \cdot n\right | &\leq \int _{\partial \mathcal{B}} |\nu |^{p-1} | \nabla \nu | \\ & \leq \||\nu |^{p-1}\|_{L^{2}(\partial \mathcal{B})} \|\nabla \nu \|_{L^{2}( \partial \mathcal{B})} \\ & \leq C\| |\nu |^{p-1}\|_{\frac{1}{2}, 2} \|\nabla \nu \|_{ \frac{1}{2}, 2} \\ & \leq C \| |\nu |^{p-1}\|_{1,2}\|\nabla \nu \|^{\frac{1}{2}}\| \Delta \nu \|^{\frac{1}{2}} \\ &\leq C \big(\| |\nu |^{p-1}\| + \| \nabla |\nu |^{p-1}\| \big)\| \nabla \nu \|^{\frac{1}{2}}\|\Delta \nu \|^{\frac{1}{2}}, \end{aligned} $$

(6.9)

by exploiting Hölder’s inequality on $\partial \mathcal{B}$ and Gagliardo-Nirenberg’s inequality on ℬ.

In such an estimate, we have

$$ \begin{aligned} \| |\nu |^{p-1}\| \|\nabla \nu \|^{\frac{1}{2}}\|\Delta \nu \|^{ \frac{1}{2}} & = \| |\nu |^{\frac{p-1}{2}}\|_{L^{4}}^{2} \|\nabla \nu \|^{\frac{1}{2}}\|\Delta \nu \|^{\frac{1}{2}} \\ & \leq C\| |\nu |^{\frac{p}{2}}\|_{L^{4}}^{\frac{2(p-1)}{p}} \| \nabla \nu \|^{\frac{1}{2}} \|\Delta \nu \|^{\frac{1}{2}} \\ & \leq C\big( \| \nu \|_{p}^{(p-1)} + \| \nabla |\nu |^{\frac{p}{2}} \|^{\frac{2(p-1)}{p}}\big) \|\nabla \nu \|^{\frac{1}{2}} \|\Delta \nu \|^{\frac{1}{2}} \\ &\leq \varepsilon \|\nu \|_{p}^{p} + \delta \| \nabla |\nu |^{ \frac{p}{2}}\|^{2}+ C_{\delta , \varepsilon} \left \{\|\nabla \nu \| \|\Delta \nu \|\right \}^{\frac{p}{2}}, \end{aligned} $$

(6.10)

as it follows from the same steps leading to the inequality (6.5). For the second term in the last line of the estimate (6.9), we find

$$ \begin{aligned} \| \nabla |\nu |^{p-1}\|= \left (\int _{\mathcal {B}}\big|\nabla |\nu |^{p-1} \big|^{2}\right )^{\frac{1}{2}}& \leq (p-1)\left (\int _{\mathcal {B}}| \nu |^{2(p-2)}|\nabla \nu |^{2}\right )^{\frac{1}{2}} \\ &\leq (p-1) \|\nu \|_{L^{\infty}}^{\frac{p-2}{2}}\left (\int _{ \mathcal {B}}|\nu |^{(p-2)}|\nabla \nu |^{2}\right )^{\frac{1}{2}} \\ &\leq C \| \nabla \nu \|_{L^{4}} \| |\nu |^{\frac{p-2}{2}}\|_{L^{4}} \|\nu \|_{L^{\infty}}^{\frac{p-2}{2}} \\ &\leq C \| \nabla \nu \|^{\frac{1}{4}}\| \Delta \nu \|^{\frac{3}{4}} \| |\nu |^{\frac{p}{2}}\|_{L^{4}}^{\frac{p-2}{p}} \|\nu \|_{L^{\infty}}^{ \frac{p-2}{2}} \\ &\leq C \| \nabla \nu \|^{\frac{1}{4}}\| \Delta \nu \|^{\frac{3}{4}} \| |\nu |^{\frac{p}{2}}\|_{1,2}^{\frac{(p-2)}{p}} \|\nu \|_{L^{\infty}}^{ \frac{p-2}{2}} \end{aligned} $$

and hence

$$ \begin{aligned} \| \nabla |\nu |^{p-1}\|& \|\nabla \nu \|^{\frac{1}{2}}\|\Delta \nu \|^{\frac{1}{2}} \leq C \| |\nu |^{\frac{p}{2}}\|_{1,2}^{ \frac{p-2}{p}} \left \{ \| \nabla \nu \|^{\frac{3}{4}}\| \Delta \nu \|^{\frac{5}{4}}\| \|\nu \|_{L^{\infty}}^{\frac{p-2}{2}}\right \} \\ &\leq C \big(\| \nu \|_{p}^{\frac{p-2}{2}} + \|\nabla |\nu |^{ \frac{p}{2}}\|^{\frac{(p-2)}{p}}\big) \left \{ \| \nabla \nu \|^{ \frac{3}{4}}\| \Delta \nu \|^{\frac{5}{4}}\| \|\nu \|_{L^{\infty}}^{ \frac{p-2}{2}}\right \} \\ & \leq \varepsilon \|\nu \|_{p}^{p} + \delta \| \nabla |\nu |^{ \frac{p}{2}}\|^{2} + C_{\delta , \varepsilon}\left \{ \| \nabla \nu \|^{\frac{3}{4}}\| \Delta \nu \|^{\frac{5}{4}}\| \|\nu \|_{L^{\infty}}^{ \frac{p-2}{2}}\right \}^{\frac{2p}{p+2}}, \end{aligned} $$

(6.11)

as a consequence of Gagliardo-Nirenberg’s and Poincaré’s inequalities. By inserting the estimates (6.10) and (6.11) into inequality (6.9), we get

$$ \begin{aligned} \left |\int _{\partial \mathcal{B}} |\nu |^{p-2} (\nu \cdot \nabla ) \nu \cdot n\right | \,\leq\, &2\varepsilon \|\nu \|_{p}^{p} + 2 \delta \| \nabla |\nu |^{\frac{p}{2}}\|^{2} + C_{\delta , \varepsilon} \left (\|\nabla \nu \|\|\Delta \nu \|\right )^{\frac{p}{2}} \\ & \quad +C_{\delta , \varepsilon}\left ( \| \nabla \nu \|^{ \frac{3}{4}}\| \Delta \nu \|^{\frac{5}{4}}\| \|\nu \|_{L^{\infty}}^{ \frac{p-2}{2}}\right )^{\frac{2p}{p+2}}. \end{aligned} \hspace{-0.5 cm} $$

(6.12)

By virtue of Remark 5.1, the last addendum in the inequality (6.12) is a function of class $L^{\infty}(0,+\infty )$ whose $\|\cdot \|_{\infty}$ norm is bound by $C_{0}$ of (5.4), and depends on the initial data, along with the values of $p$ and the other fixed parameters. Also, due to the fact that $\nu \in L^{\infty}(0,+\infty ;\mathcal{H}^{2})$, the right-hand side of (6.12) (and similar ones below involving $u$) is in $L^{1}_{\mathit{uloc}}(0, +\infty )$.

For the mixed boundary term, we have

$$ \begin{aligned} \left |\int _{\partial \mathcal{B}}|\nu |^{p-2}(\nu \cdot \nabla ) u \cdot n\right | & \leq \int _{\partial \mathcal{B}}|\nu |^{p-1} | \nabla u| \\ & \leq \| |\nu |^{p-1}\|_{L^{2}(\partial \mathcal{B})} \|\nabla u\|_{L^{2}( \partial \mathcal{B})} \\ &\leq \| |\nu |^{p-1}\|_{\frac{1}{2},2}\|\nabla u\|_{\frac{1}{2},2} \\ &\leq C\| |\nu |^{p-1}\|_{1, 2} \|\nabla u\|^{\frac{1}{2}}\|\Delta u \|^{\frac{1}{2}} \\ &\leq C \| |\nu |^{p-1}\| \|\nabla u\|^{\frac{1}{2}}\|\Delta u\|^{ \frac{1}{2}} \\ & \qquad + C\| \nabla |\nu |^{p-1}\| \|\nabla u\|^{\frac{1}{2}}\| \Delta u\|^{\frac{1}{2}}, \end{aligned} $$

and using the same estimates in the inequalities (6.10) and (6.11), we obtain

$$ \begin{aligned} \left |\int _{\partial \mathcal{B}}|\nu |^{p-2}(\nu \cdot \nabla ) u \cdot n\right |& \leq 2\varepsilon \|\nu \|_{p}^{p} + 2\delta \| \nabla |\nu |^{\frac{p}{2}}\|^{2} \\ &\quad + C_{\delta , \varepsilon}\left (\|\nabla u\|\|\Delta u\| \right )^{\frac{p}{2}} \\ & \quad +C_{\delta , \varepsilon} \left (\|\nabla \nu \|^{ \frac{p-1}{2}} \|\Delta \nu \|^{\frac{p+1}{2}} \|\nabla u\|\|\Delta u \|\right )^{\frac{p}{p+2}}. \end{aligned} $$

(6.13)

Putting together the inequalities (6.7), (6.8), (6.12) and (6.13), from equation (6.4) we obtain

$$ \begin{aligned} \frac{\varsigma}{p}\frac{\mathrm{d}}{\mathrm{d}t}\|\nu \|^{p}_{p} + ( \kappa _{0}-7\varepsilon )\|\nu \|_{p}^{p} &+ \Big( \frac{4 \zeta (p-2)}{p^{2}}-7\delta \Big) \big\| \nabla |\nu |^{ \frac{p}{2}}\big\| ^{2} \\ &+ \Big(\frac{\zeta}{2}-2\epsilon \Big)\||\nu |^{\frac{p-2}{2}} \nabla \nu \|^{2} \leq C \end{aligned} $$

(6.14)

where $C=C_{\epsilon , \varepsilon , \delta ,C_{0}}$ and $C_{0}$ is given in the inequality (5.4). In particular, by choosing $\varepsilon = \frac{\kappa _{0}}{14}$, we obtain, for every $t \ge 0$,

$$ \frac{\mathrm{d}}{\mathrm{d}t}\|\nu \|^{p}_{p} + \frac{2 p \kappa _{0}}{\varsigma}\|\nu \|_{p}^{p} \leq C, $$

from which, by standard arguments, we deduce

$$ \|\nu (t)\|^{p}_{p} \leq \|\nu _{0}\|^{p}_{p} e^{- \frac{2 p \kappa _{0}}{\varsigma} t} + C. $$

(6.15)

Remark 6.1

From (6.14), directly, we also get

$$ \|\nabla |\nu |^{\frac{p}{2}}\|, \||\nu |^{\frac{p-2}{2}} \nabla \nu \|\in L^{2}_{uloc}(0, +\infty )\;.$$

6.2 Decay Estimate for $\|u\|_{p}^{p}$

For the first two terms on the right-hand side of the equation (6.3) we compute

$$ \begin{aligned} \rho \big\| u_{t} | u|^{(p-2)/2}\big\| ^{2} + & \rho (p-2)\int _{ \mathcal{B}} |u|^{p-4 }( u_{t} \cdot u)^{2} \\ &\leq \rho \int _{\mathcal{B}} |u_{t}|^{2} |u|^{p-2} + \rho (p-2) \int _{\mathcal{B}} |u_{t}|^{2} |u|^{p-2} \\ & = \rho (p-1) \int _{\mathcal{B}} | u_{t}|^{2} |u|^{p-2} \\ & \leq \rho (p-1) \|u\|^{p-2}_{L^{\infty}} \|u_{t}\|^{2}. \end{aligned} $$

The remaining terms on the right-hand side of the identity (6.3), i.e.,

$$ \overline{\xi}\big(\mathrm{div} u, \mathrm{div} \big(\nu |\nu |^{p-2} \big)\big), \,\,\, \kappa \big(\nabla u, \nabla \big(\nu |\nu |^{p-2} \big)\big), \,\,\textrm{ and }\,\, \xi \big(\mathrm{div} u, \mathrm{div} \big(u|u|^{p-2}\big)\big), $$

can be estimated as done in the case of the corresponding terms in the equation (6.4): In fact, these terms, up to exchange $\nu$ with $u$ are exactly the ones previously controlled. For the boundary terms in $\Gamma _{u}^{p}$, the integrals

$$ \mu \int _{\partial \mathcal{B}}|u|^{p-2}(u\cdot \nabla ) u \cdot n \,\, \textrm{ and }\,\, \kappa \int _{\partial \mathcal{B}}|u|^{p-2}(u \cdot \nabla ) \nu \cdot n $$

can be estimated as done in the inequalities (6.12)-(6.13).

Eventually, from (6.3) we get

$$\begin{array}{c}|\frac{\rho }{p}\frac{d^2}{d{t}^2}{\parallel u\parallel }_p^p+\frac{\tilde{\gamma }}{p}\frac{\mathrm{d}}{\mathrm{d}t}{\parallel u\parallel }_p^p-\frac{4\mu (p-2)}{p^2}{\parallel u\parallel }_p^p|\hfill \\ \hfill \le (\frac{4\mu (p-2)}{p^2}+11\delta ){\parallel {|u|}^{\frac{p}{2}}\parallel }_{1,2}^2+(\frac{\mu }{2}+2ϵ){\int }_{\mathcal{B}}{|u|}^{p-2}{|\mathrm{\nabla }u|}^2+C_{\delta ,ϵ,\epsilon },\end{array}$$

where $C_{\delta , \epsilon , \varepsilon}$ is related to $C_{0}$ in the inequality (5.4).

Since $u$ is of class $L^{\infty}(0,\infty ;L^{\infty}(\mathcal{B}))$ (see Remark 5.2), we deduce that

$$ \||u|^{\frac{p-2}{2}}|\nabla u|\| \le \|u\|_{\infty }^{\frac{p-2}{2}} \|\nabla u\|\le C. $$

(6.16)

Analogously, we can bound the first term in the right-hand side, observing that

$$ \left |\nabla |u|^{\frac{p}{2}}\right |^{2}\le \frac{p^{2}}{4}|u|^{p-2}| \nabla u|^{2}, $$

and then using (6.16) again. Thus, for every $t \ge 0$, we obtain

$$ \left |\frac{d^{2}}{dt^{2}}\| u\|^{p}_{p} + \frac{\tilde{\gamma}}{\rho} \frac{\mathrm{d}}{\mathrm{d}t}\| u\|^{p}_{p} - \frac{8 \mu (p-2)}{\rho p} \|u\|^{p}_{p} \right |\le C. $$

Thanks to this control, by applying Theorem 4.1, and exploiting again the boundedness in time of $\|u(t)\|_{\infty}$, we finally obtain

$$ \|u(t)\|_{p}^{p} \le \|u_{0}\|_{p}^{p} e^{-\alpha t} + C, $$

(6.17)

where $\alpha =\frac{1}{\rho}\left (\tilde{\gamma}+\sqrt{\tilde{\gamma}^{2} + 16 \mu ^{2}(p-2)^{2}/p^{2}}\right )$. In turn, this result implies the estimate (3.5) and, along with (6.15), concludes the proof of Theorem 3.2.

7 Additional Remarks

The results obtained here show how dissipative microstructural changes – in particular diffusion – may influence the overall energy decay in the linear dynamics of otherwise elastic complex bodies with active microstructures described by a vector field. From a physical viewpoint the key result is the one dealing with the condition $\tilde{\gamma}=0$; in that case, in fact, the only dissipative mechanism is at microstructural level, i.e., it is a low-spatial-scale mechanism. Microscopic-to-macroscopic coupling amplifies effects crossing spatial scales up to generate the global energy decay.