Geometric Variational Principles for Computational Homogenization

Abstract

The homogenization of periodic elastic composites is addressed through the reformulation of the local equations of the mechanical problem in a geometric functional setting. This relies on the definition of Hilbert spaces of kinematically and statically admissible tensor fields, whose orthogonality and duality properties are recalled. These are endowed with specific energetic scalar products that make use of a reference and uniform elasticity tensor. The corresponding strain and stress Green’s operators are introduced and interpreted as orthogonal projection operators in the admissibility spaces. In this context and as an alternative to classical minimum energy principles, two geometric variational principles are investigated with the introduction of functionals that aim at measuring the discrepancy of arbitrary test fields to the kinematic, static or material admissibility conditions of the problem. By relaxing the corresponding local equations, this study aims in particular at laying the groundwork for the homogenization of composites whose constitutive properties are only partially known or uncertain. The local fields in the composite and their macroscopic responses are computed through the minimization of the proposed geometric functionals. To do so, their gradients are computed using the Green’s operators and gradient-based optimization schemes are discussed. A FFT-based implementation of these schemes is proposed and they are assessed numerically on a canonical example for which analytical solutions are available.

Appendices

Appendix A: Mathematical Definitions

1.1 A.1 Periodic Fields

Consider a unit-cell $\mathcal{V}$ allowing to fill the space $\mathbb{R}^{d}$ by translation along $d$ vectors ${\boldsymbol{Y}}_{1}, \dots, {\boldsymbol{Y}}_{d}$. The lattice ℛ generated by these vectors is defined as

$$ \mathcal{R} = \Biggl\{ {\boldsymbol{Y}},\; {\boldsymbol{Y}} = \sum _{j=1}^{d} n_{j} {\boldsymbol{Y}}_{j}, \; n_{j} \in\mathbb{Z} \Biggr\} . $$

Define spaces of periodic scalar functions, vector fields and tensor fields as:

1.2 A.2 Fourier Transforms

The Fourier transform $\hat{f}$ of $f$ is defined as:

$$ \hat{f}({\boldsymbol{\xi}}) = \mathscr{F}[f]({\boldsymbol{\xi}}) = \frac{1}{| \mathcal{V}|} \int_{\mathcal{V}} f( {\boldsymbol{x}} ) e^{-\mathrm{i} {\boldsymbol{\xi}} \cdot{\boldsymbol{x}}} \,\text{d} { \boldsymbol{x}},\quad\text{where } \mathrm{i} = \sqrt{-1}. $$

Let $\mathcal{R}^{*}$ denote the reciprocal lattice of ℛ generated by the vectors

$$ {\boldsymbol{Y}}^{*}_{i} = \frac{2 \pi}{|\mathcal{V}|} { \boldsymbol{Y}}_{j} \wedge {\boldsymbol{Y}}_{k}, $$

where $(i,j,k)$ is a direct circular permutation. Then, according to Plancherel’s theorem:

$$ \frac{1}{|\mathcal{V}|} \int_{\mathcal{V}} \bigl|f({\boldsymbol{x}}) \bigr|^{2} \ d { \boldsymbol{x}} = \sum_{{\boldsymbol{\xi}} \in\mathcal{R}^{*}} |\hat{f}( {\boldsymbol{ \xi}}) |^{2}, $$

and therefore

$$ f \in L^{2}_{\text{per}}\! (\mathcal{V} ) \quad \Leftrightarrow \quad\sum_{{\boldsymbol{\xi}} \in\mathcal{R}^{*}} \bigl|\hat{f}({\boldsymbol{\xi}})\bigr|^{2} < + \infty. $$

The original periodic function $f$ in $L^{2}_{\text{per}}\! (\mathcal{V} )$ can be reconstructed from its Fourier transform by

$$ f({\boldsymbol{x}}) = \mathscr{F}^{-1}[\hat{f}]({\boldsymbol{x}}) = \sum_{{\boldsymbol{\xi}} \in\mathcal{R}^{*}} \hat{f}({\boldsymbol{\xi}}) e^{ \mathrm{i} {\boldsymbol{\xi}} \cdot{\boldsymbol{x}}}. $$

Appendix B: Properties of the Green’s Operators

Lemma 3

The strain Green’s operator${\boldsymbol{\varGamma}}_{0}$satisfies the following properties:

• 1. ${\boldsymbol{\varGamma}}_{0}$satisfies the reciprocity identity:

  

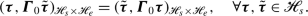  (65)

• 2. The kernel of${\boldsymbol{\varGamma}}_{0}$coincides with the subspace:

  

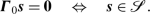  (66)

• 3. ${\boldsymbol{\varGamma}}_{0}$is such that:

  $$ {\boldsymbol{\varGamma}}_{0} {\boldsymbol{L}}_{0} { \boldsymbol{\varGamma}}_{0} = {\boldsymbol{\varGamma}} _{0}. $$

  (67)

Properties (66) and (67) were proved in [13]. The additional property (65) derives from the identity

(68)

To prove (68), note that, by definition of ${\boldsymbol{\varGamma}} _{0}$, a stress field ${\boldsymbol{s}}={\boldsymbol{L}}_{0} {\boldsymbol{\varGamma}}_{0}{\boldsymbol {\tau}}- {\boldsymbol{\tau}}$ in can be associated with ${\boldsymbol{\tau}}$ through (17). By Lemma 1:

which, according to the definition (9) of the Riesz mapping, proves (68). Note that similar properties can be proved for the stress Green’s operator ${\boldsymbol{\Delta}}_{0}$ owing to the duality principle, see [14].

Remark 6

If one defines the Green’s operator from , endowed with the standard $L^{2}$-scalar product, into itself then the reciprocity identity (65) amounts in the self-adjointness of ${\boldsymbol{\varGamma}}_{0}$, a property which is known since [10].