Abstract
The precautionary principle has emerged as a leading guide to public decision-making about environmental risks under irreversibility and uncertainty. In this paper, we adopt a two-period model with irreversibility and agents differentiated by their degree of optimism to characterize the conditions under which the precautionary principle applies. We show in particular that it is more often applied if the decision-maker has an intermediate optimism index, if scientific research is more effective at reducing uncertainty, and if the same decision-maker makes the decisions for both periods. Moreover, we show that socially optimal decisions lead to apply the precautionary principle more often than an elected decision-maker does.





Similar content being viewed by others
Notes
As described in Berggren (2012).
In the literature on decision theory, ambiguity and uncertainty are not always distinguished, and we will use both terms in this paper.
Non-expected utility models do not satisfy dynamic consistency in general. However, in our specific framework, decisions are comonotonic (the outcomes of all decisions are higher when the product is harmless than when it is harmful) and consequently, dynamic consistency holds when the same decision maker takes the decisions in the two periods of time.
K is the cost (in terms of welfare) if the product is harmful, pK is the average cost if the product has probability p of being harmful.
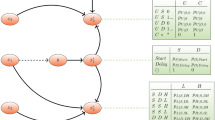
AA (resp. II) denotes the set of points \((\alpha ,U)\) such that \(D^{*}=(A,A)\) (resp. \(D^{*}=(I,I)\)), and AR (resp. IR) denotes the set of points \((\alpha ,U)\) such that \(D_{1}^{*}=A\) (resp. \(D_{1}^{*}=I\)) and \(D_{2}\) depends on the results of research
The loss of welfare linked to a wrong authorization in second-period (with prohibition in first-period) is a concave function. This loss for an average individual is greater than the average of the losses for all the individuals.
References
Arrow KJ, Fisher AC (1974) Environmental preservation, uncertainty, and irreversibility. Q J Econ 88(2):312–319
Arrow KJ, Hurwicz L (1972) An optimality criterion for decision-making under ignorance. In: Carter C, Ford J (eds) Uncertainty and expextations in economics. B. Blackwell, Oxford, pp 1–11
Asano T (2010) Precautionary Principle and the optimal timing of environmental policy under ambiguity. Environ Resour Econ 47:173–196
Basili M, Chateauneuf A, Fontini F (2008) Precautionary principle as a rule of choice with optimism on windfall gains and pessimism on catastrophic losses. Ecol Econ 67:485–491
Berggren N (2012) Time for behavioral political economy? An analysis of articles in behavioral economics. Rev Austrian Econ 25:199–221
Bouglet T, Lanzi T, Vergnaud J-C (2006) Incertitude scientifique et décision publique : le recours au Principe de pré caution. Rech Econ Louvain 72(2):109–127
Chateauneuf A, Eichberger J, Grant S (2007) Choice under uncertainty with the best and the worst in mind: neo-additive capacities. J Econ Theory 137:538–567
Courbage C, Rey B, Treich N (2013) Prevention and precaution. In: Dionne G (ed) Handbook of insurance. Springer, New-York, pp 185–204
Epstein LG (1980) Decision making and the temporal resolution of uncertainty. Int Econ Rev 21(2):269
Gilboa I, Schmeidler D (1989) Maxmin expected utility with non-unique prior. J Math Econ 18–2:141–153
Gollier C, Jullien B, Treich N (2000) Scientific progress and irreversibility: an economic interpretation of the Precautionary Principle. J Public Econ 75:229–253
Gollier C, Treich N (2003) Decision making under scientific uncertainty: the economics of the Precautionary Principle. J Risk Uncertain 27(1):77–103
Grant S, Quiggin J (2013) Bounded awareness, heuristics and the precautionary principle. J Econ Behav Organ 93:17–31
Henry C (1974) Investment decisions under uncertainty: the irreversibility effect. Am Econ Rev 64(6):1006–12
Immordino G (2003) Looking for a guide to protect the environment: the development of the precautionary principle. J Econ Surv 17(5):629–643
Jeleva M, Rossignol S (2009) Political management of risk : the role of trust. Public Choice 139–1:83–104
Klibanoff P, Marinacci M, Mukerji S (2005) A smooth model of decision making under ambiguity. Econometrica 73:1849–1892
Lange A, Treich N (2008) Uncertainty, learning and ambiguity in economic models on climate policy: some classical results and new directions. Clim Change 89:7–21
Ulph A, Ulph D (1997) Global warming, irreversibility and learning. Econ J 107:636–650
Acknowledgements
Preliminary versions of this paper were presented at the European Public Choice Society Meeting, at the International Conference on Public Economic Theory, and at LED seminar in St-Denis; we are grateful to all participants for their comments. We thank also two anonymous referees who helped us to improve the paper.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix 1: Proofs Without Irreversibility
Proof of Proposition 1
If \(D_{1}=I\), to determine \(D_{2}^{*},\) it is necessary to compare the individual evaluations of (I, I) and (I, A), taking into account the fact that the evaluation of the pair (I, A) depends on the result of the research.
Combining Table 1 and Eq. (8) gives:
Consequently, \(D_{2}^{*}(I)=A\Leftrightarrow q_{R}(\alpha )<\frac{\Delta V}{K}.\)
If \(D_{1}=A\), to determine \(D_{2}^{*},\) it is necessary to compare the individual evaluations of (A, I) and (A, A), taking into account the fact that both evaluations depend on the research result:
Consequently, \(D_{2}^{*}(A)=A\Leftrightarrow q_{R}(\alpha )<\frac{\Delta V}{K}.\)
Then \(q_{1}(\alpha )>q_{0}(\alpha )\) implies the proposition results. \(\square\)
Proof of Proposition 2
According to Equation (4), the welfare at the beginning of Period 1 if decisions \(D_{1}\) and \(D_{2}\) are taken is:
We want to determine the value \(D_{1}^{*}\) of \(D_{1}\) which maximizes \(W_{\alpha }(D_{1},D_{2}^{*})\), where \(D_{2}^{*}\) is given by Proposition 1. Since \(D_{2}^{*}\) does not depend on \(D_{1}\) when there is no irreversibility (Proposition 1), it is equivalent maximizing \(h(D_{1})=W_{1}(D_{1})-Kq(\alpha )1_{D_{1}=A}\)
-
\(h(A)=V-Kq(\alpha )\) and \(h(I)=V_{I}\), thus:
-
\(h(A)>h(I)\Leftrightarrow V-Kq(\alpha )>V_{I}\)
-
\(h(A)>h(I)\Leftrightarrow U>q(\alpha )\)
We can conclude that \(D_{1}^{*}=A\) if \(U>q(\alpha )\), and \(D_{1}^{*}=I\) if \(U<q(\alpha )\). \(\square\)
Appendix 2: Proofs with Irreversibility
Proof of Proposition 3
-
(a)
If \(D_{1}=A\) then\(\;D_{2}^{*}=A\) by irreversibility.
-
(b)
If \(D_{1}=I\) both \(D_{2}^{*}=A\) and \(D_{2}^{*}=I\) are possible. To determine the preferred one, it is necessary to compare the corresponding individual evaluations, taking into account the fact that the evaluation of the pair (I, A) depends on the result of the research.
$$\begin{aligned} W_{\alpha ,R}(I,I)& = 2V_{I} \\ W_{\alpha ,R}(I,A)& = q_{R}(\alpha )W(B,I,A)+(1-q_{R}(\alpha ))W(G,I,A) \\& = V_{I}+V-Kq_{R}(\alpha ) \end{aligned}$$Consequently, \(D_{2}^{*}(I)=A\Leftrightarrow q_{R}(\alpha )<\frac{\Delta V}{K}.\)
Then \(q_{1}(\alpha )>q_{0}(\alpha )\) implies the results. \(\square\)
Proof of Proposition 4
To determine \(D_{1}^{*}\), we have to compare \(W_{\alpha }(I,D_{2}^{*}(I))\) and \(W_{\alpha }(A,D_{2}^{*}(A)).\)
Due to irreversibility, \(W_{\alpha }(A,D_{2}^{*}(A))=W_{\alpha }(A,A)=2(V-Kq(\alpha ))\) where \(q(\alpha )=\alpha q_{0}(\alpha )+(1-\alpha )q_{1}(\alpha )\) is the first period (ex ante) perception of the probability of the product being harmful.
\(D_{2}^{*}(I)\) depends on the values of the parameters as shown in Proposition 3. Thus,
-
1.
If \(q_{0}(\alpha )>U\) then \(D_{2}^{*}(I)=I\) for any R and \(W_{\alpha }(I,D_{2}^{*}(I))=W_{\alpha }(I,I)=2V_{I};\)
-
2.
If \(q_{1}(\alpha )<U\) then \(D_{2}^{*}(I)=A\) for any R and \(W_{\alpha }(I,D_{2}^{*}(I))=W_{\alpha }(I,A)=V_{I}+V-Kq(\alpha );\)
-
3.
If \(q_{0}(\alpha )<U<q_{1}(\alpha )\) then \(D_{2}^{*}(I)=I\) if \(R=1\) and \(D_{2}^{*}(I)=A\) if \(R=0\) and according to Eq. (11) we have:
$$\begin{aligned} W_{\alpha }(D_{1},D_{2}^{*}(D_{1}))& = W_{\alpha }(I,D_{2}^{*}(I))=\alpha W_{\alpha ,0}(I,A)+(1-\alpha )W_{\alpha ,1}(I,I)\\& = \alpha \left[ V_{I}+V-Kq_{0}(\alpha )\right] +(1-\alpha )\left[ 2V_{I} \right] =(2-\alpha )V_{I}+\alpha (V-Kq_{0}(\alpha )). \end{aligned}$$
Comparing \(W_{\alpha }(A,A)\) and \(W_{\alpha }(I,D_{2}^{*}(I))\) in each of the previous cases, we obtain:
-
1.
If \(q_{0}(\alpha )>U\) then \(D_{1}^{*}=A\Leftrightarrow 2V-2Kq(\alpha )>2V_{I}\), i.e., \(\frac{\Delta V}{K}>q(\alpha );\)
-
2.
If \(q_{1}(\alpha )<U\) then \(D_{1}^{*}=A\Leftrightarrow 2V-2Kq(\alpha )>V_{I}+V-Kq(\alpha )\)
i.e., \(D_{1}^{*}=A\Leftrightarrow \frac{\Delta V}{K}>q(\alpha );\)
-
3.
If \(q_{0}(\alpha )<U<q_{1}(\alpha )\) then \(D_{1}^{*}=A\Leftrightarrow 2V-2Kq(\alpha )>(2-\alpha )V_{I}+\alpha (V-Kq_{0}(\alpha ))\),
i.e., \(D_{1}^{*}=A\Leftrightarrow \frac{\Delta V}{K}>\frac{2q(\alpha )-\alpha q_{0}(\alpha )}{2-\alpha }=M(\alpha )\)
Since \(q(\alpha )=\alpha q_{0}(\alpha )+(1-\alpha )q_{1}(\alpha )\), and \(q_{0}(\alpha )\le q_{1}(\alpha )\),we have \(q(\alpha )\in \left[ q_{0}(\alpha ),q_{1}(\alpha )\right]\).
This implies that \(\left[ q_{0}(\alpha )>\frac{\Delta V}{K}\text { and }\frac{ \Delta V}{K}>q(\alpha )\right]\) is impossible, and that \(q_{1}(\alpha )< \frac{\Delta V}{K}\) implies \(\frac{\Delta V}{K}>q(\alpha )\).
Consequently,
-
1.
If \(q_{0}(\alpha )>U\) then \(D_{1}^{*}=I;\)
-
2.
If \(q_{1}(\alpha )<U\) then \(D_{1}^{*}=A;\)
-
3.
If \(q_{0}(\alpha )<U<q_{1}(\alpha )\) then \(D_{1}^{*}=A\)\(\Leftrightarrow \frac{\Delta V}{K}>M(\alpha ).\)
It is easy to show that \(M(\alpha )=\frac{2q(\alpha )-\alpha q_{0}(\alpha )}{ 2-\alpha }\in \left[ q_{0}(\alpha ),q_{1}(\alpha )\right]\) which gives the first two results of the proposition.
Moreover,
\(\square\)
Appendix 3: Political Decision
Proof of Proposition 5
We have to compare \(W_{\alpha _{1}}(I,D_{2}^{*}(\alpha _{2},I))\) and \(W_{\alpha _{1}}(A,D_{2}^{*}(\alpha _{2},A)).\)
Due to irreversibility, \(W_{\alpha _{1}}(A,D_{2}^{*}(\alpha _{2},A))=W_{\alpha _{1}}(A,A)=2(V-Kq(\alpha _{1}))\) where \(q(\alpha _{1})=\alpha _{1}q_{0}(\alpha _{1})+(1-\alpha _{1})q_{1}(\alpha _{1})\) is the first period (ex ante) perception of the probability of the product being harmful.
\(D_{2}^{*}(\alpha _{2},I)\) depends on the values of the parameters as shown in Proposition 3 with \(\alpha =\alpha _{2}\). Thus,
-
1.
If \(q_{0}(\alpha _{2})>U\) then \(D_{2}^{*}(\alpha _{2},I)=I\) for any R and \(W_{\alpha _{1}}(I,D_{2}^{*}(\alpha _{2},I))=W_{\alpha _{1}}(I,I)=2V_{I};\)
-
2.
If \(q_{1}(\alpha _{2})<U\) then \(D_{2}^{*}(\alpha _{2},I)=A\) for any R and \(W_{\alpha _{1}}(I,D_{2}^{*}(\alpha _{2},I))=W_{\alpha _{1}}(I,A)=V_{I}+V-Kq(\alpha _{1});\)
-
3.
If \(q_{0}(\alpha _{2})<U<q_{1}(\alpha _{2})\) then \(D_{2}^{*}(\alpha _{2},I)=I\) if \(R=1\) and \(D_{2}^{*}(\alpha _{2},I)=A\) if \(R=0\) thus \(W_{\alpha _{1}}(I,D_{2}^{*}(\alpha _{2},I))=\alpha _{1}W_{\alpha _{1},0}(I,A)+(1-\alpha _{1})W_{\alpha _{1},1}(I,I)\)\(=\alpha _{1}\left[ V_{I}+V-Kq_{0}(\alpha _{1})\right] +(1-\alpha _{1})\left[ 2V_{I}\right] =(2-\alpha _{1})V_{I}+\alpha _{1}(V-Kq_{0}(\alpha _{1})).\)
Comparing \(W_{\alpha _{1}}(A,A)\) and \(W_{\alpha _{1}}(I,D_{2}^{*}(\alpha _{2},I))\) in each of the previous cases, we obtain:
-
1.
If \(q_{0}(\alpha _{2})>U\) then \(D_{1}^{*}=A\Leftrightarrow 2V-2Kq(\alpha _{1})>2V_{I}\), i.e., \(\frac{\Delta V}{K}>q(\alpha _{1});\)
-
2.
If \(q_{1}(\alpha _{2})<U\) then \(D_{1}^{*}=A\Leftrightarrow 2V-2Kq(\alpha _{1})>V_{I}+V-Kq(\alpha _{1})\),
i.e., \(D_{1}^{*}=A\Leftrightarrow \frac{\Delta V}{K}>q(\alpha _{1});\)
-
3.
If \(q_{0}(\alpha _{2})<U<q_{1}(\alpha _{2})\) then
$$\begin{aligned} D_{1}^{*}& = A\Leftrightarrow 2V-2Kq(\alpha _{1})>(2-\alpha _{1})V_{I}+\alpha _{1}(V-Kq_{0}(\alpha _{1})),~~\hbox {i.e.,}~~ \\ D_{1}^{*}& = A\Leftrightarrow \frac{\Delta V}{K}>\frac{2q(\alpha _{1})-\alpha _{1}q_{0}(\alpha _{1})}{2-\alpha _{1}}=M(\alpha _{1}) \end{aligned}$$As \(q(\alpha _{1})<M(\alpha _{1})\), we can deduce then in the three cases:
\(\frac{\Delta V}{K}<q(\alpha _{1})\) implies \(D_{1}^{*}=I\)
\(\frac{\Delta V}{K}>M(\alpha _{1})\) implies \(D_{1}^{*}=A\)
If \(q(\alpha _{1})<\frac{\Delta V}{K}<M(\alpha _{1})\), then:
which gives the result of the proposition. \(\square\)
Proof of Corollary 3
For \(\alpha _{2}\) given, \(0<\alpha _{2}<1\), we define \(\alpha ^{\prime }\) and \(\alpha ''\) by \(q_{0}(\alpha _{2})=q(\alpha ^{\prime })=M(\alpha '')\)
We know that \(q_{0}\), q, and M are decreasing functions, with \(q_{0}<q<M\) , so \(\alpha _{2}<\alpha ^{\prime }<\alpha ''\).
Assume \(\alpha _{1}\in \left( \alpha ^{\prime },\alpha ''\right)\); we have:
-
If \(U<q(\alpha _{1})\), then \(D_{1}^{*}=I\) according to Proposition 5.
-
If \(U>M(\alpha _{1})\), then \(D_{1}^{*}=A\) according to Proposition 5.
-
Assume that \(q(\alpha _{1})<U<M(\alpha _{1})\).
We have \(q(\alpha _{1})<q_{0}(\alpha _{2})<M(\alpha _{1})\) since:
and
We can then distinguish two sub-cases:
First sub-case: \(q(\alpha _{1})<U<q_{0}(\alpha _{2})\), then \(D_{1}^{*}=A\) according to Proposition 5.
Second sub-case: \(q_{0}(\alpha _{2})<U<M(\alpha _{1})\), then \(D_{1}^{*}=I\) according to Proposition 5. \(\square\)
Proof of Propositions 6 and 7
-
(a)
Majority voting on \(D_{2}\)
For \(D_{1}\) given, Proposition 3 gives the preferences of an agent \(\alpha\) on \(D_{2}\).
-
If \(D_{1}=A\), we have unanimity for \(D_{2}^{*}(\alpha )=A\) since there is no other choice.
-
If \(D_{1}=I\) and \(R=0\), then \(D_{2}^{*}(\alpha )=I\) iff \(U<q_{0}(\alpha )\)
-
If \(D_{1}=I\) and \(R=1\), then \(D_{2}^{*}(\alpha )=I\) iff \(U<q_{1}(\alpha )\)
\(q_{0}\) and \(q_{1}\) are affine functions, thus the median voter theorem applies, and \(D_{2}^{**}=D_{2}^{*}(\alpha _{m_{2}})\)
-
-
(b)
Majority voting on \(D_{1}\)
Proposition 5 gives the preferences of an agent \(\alpha _{1}\) on \(D_{1}\) if \(\alpha _{2}=\alpha _{m_{2}}\)Three cases can be distinguished:
-
Case 1: If \(U<q_{0}(\alpha _{2})\), then \(D_{1}^{*}(\alpha _{1})=I\) iff \(U<q(\alpha _{1})\)
-
Case 2: If \(U>q_{1}(\alpha _{2})\), then \(D_{1}^{*}(\alpha _{1})=I\) iff \(U<q(\alpha _{1})\)
-
Case 3: If \(q_{0}(\alpha _{2})<U<q_{1}(\alpha _{2})\), then \(D_{1}^{*}(\alpha _{1})=I\) iff \(U<M(\alpha _{1})\)
-
In each of the three cases, the median voter theorem can be applied because q and M are continuous decreasing functions. Thus \(D_{1}^{**}=D_{1}^{*}(\alpha _{m_{1}})\).
The median voter theorem applies for each period. Proposition 6 is then obtained by Corollary 2 with \(\alpha =\alpha _{m}\). Proposition 7 is obtained by Proposition 3 with \(\alpha =\alpha _{m_{2}}\) and Proposition 5 with \(\alpha _{1}=\alpha _{m_{1}}\) and \(\alpha _{2}=\alpha _{m_{2}}\). \(\square\)
Proof of Proposition 8
The political decision is given in Proposition 6 with \(\alpha _{m}=\overline{ \alpha }\).
The socially optimal decisions are evaluated with a utilitarian criterion:
-
(a)
Second-period socially optimal decisions
-
If \(D_{1}=A\) then \(D_{2}^{opt}(A)=A\) due to irreversibility;
-
If \(D_{1}=I\), then to determine the optimal decision it is necessary to take into account the fact that the evaluation of the decision (I, A) depends on the result of the research.
$$\begin{aligned} {\mathcal {U}}_{R}(I,I)& = \dfrac{1}{n}\sum _{i=1}^{n}W_{\alpha (i),R}(I,I)=2V_{I}=W_{{\overline{\alpha }},R}(I,I) \\ {\mathcal {U}}_{R}(I,A)& = \dfrac{1}{n}\sum _{i=1}^{n}W_{\alpha (i),R}(I,A)=\dfrac{ 1}{n}\sum _{i=1}^{n}\left[ V_{I}+V-Kq_{R}(\alpha (i))\right] \\& = V_{I}+V-Kq_{R}({\overline{\alpha }})=W_{{\overline{\alpha }} ,R}(I,A) \end{aligned}$$
due to the linearity of \(q_{R}\) with respect to \(\alpha .\)
It follows that given \(D_{1}=I\), the utilitarian second-period decisions are the same as the political decisions when the median voter has an optimism index \({\overline{\alpha }}.\)
From Proposition 3,
-
1.
If \(q_{0}({\overline{\alpha }})>U\) then \(D_{2}^{opt}(I)=D_{2}^{**}(I)=I\) for any R;
-
2.
If \(q_{1}({\overline{\alpha }})<U\) then \(D_{2}^{opt}(I)=D_{2}^{**}(I)=A\) for any R;
-
3.
If \(q_{0}({\overline{\alpha }})<U<q_{1}({\overline{\alpha }})\) then \(D_{2}^{opt}(I)=D_{2}^{**}(I)=I\) if \(R=1\) and \(D_{2}^{opt}(I)=D_{2}^{ **}(I)=A\) if \(R=0\)
(b) First-period optimal decisions.
We have to compare \({\mathcal {U}}(A,D_{2}^{opt}(A))\) and \({\mathcal {U}} (I,D_{2}^{opt}(I)).\)
Due to irreversibility,
From (a), \({\mathcal {U}}(I,D_{2}^{opt}(I))\) depends on U, \(q_{0}(\overline{ \alpha })\) and \(q_{1}({\overline{\alpha }}).\)
More specifically:
-
1.
If \(q_{0}({\overline{\alpha }})>U\) then \(D_{2}^{opt}(I)=D_{2}^{**}(I)=I\) for any R and \({\mathcal {U}}(I,D_{2}^{opt}(I))={\mathcal {U}} (I,I)=2V_{I}=W_{{\overline{\alpha }}}(I,I)\)
\({\mathcal {U}}(A,D_{2}^{opt}(A))-{\mathcal {U}}(I,D_{2}^{opt}(I))=W_{\overline{ \alpha }}(A,A)-W_{{\overline{\alpha }}}(I,I)=2V-2Kq({\overline{\alpha }} )-2V_{I}<0\) if \(q_{0}({\overline{\alpha }})>U\),
thus \(D_{1}^{opt}=D_{1}^{**}=I\); then \(D^{opt}=D^{**}=(I,I)\).
-
2.
If \(q_{1}({\overline{\alpha }})<U\) then \(D_{2}^{opt}(I)=D_{2}^{**}(I)=A\) for any R and \({\mathcal {U}}(I,D_{2}^{opt}(I))=\dfrac{1}{n} \sum _{i=1}^{n}W_{\alpha (i)}(I,A)=\dfrac{1}{n}\sum _{i=1}^{n}\left( V_{I}+V-Kq(\alpha (i))\right) =V_{I}+V-Kq({\overline{\alpha }})=W_{\overline{ \alpha }}(I,A)\)
\({\mathcal {U}}(A,D_{2}^{opt}(A))-{\mathcal {U}}(I,D_{2}^{opt}(I))=W_{\overline{ \alpha }}(A,A)-W_{{\overline{\alpha }}}(I,A)\)
\(=2V-2Kq({\overline{\alpha }})-V_{I}-V+Kq({\overline{\alpha }})=V-V_{I}-Kq( {\overline{\alpha }})>0\) if \(q_{1}({\overline{\alpha }})<U.\)
thus \(D_{1}^{opt}=D_{1}^{**}=A\); then \(D^{opt}=D^{**}=(A,A)\).
-
3.
If \(q_{0}({\overline{\alpha }})<U<q_{1}({\overline{\alpha }})\) then \(D_{2}^{opt}(I)=D_{2}^{**}(I)=I\) if \(R=1\) and \(D_{2}^{opt}(I)=D_{2}^{ **}(I)=A\) if \(R=0\). Moreover, according to Equation (11)
$$\begin{aligned}&{\mathcal {U}}(I,D_{2}^{opt}(I))=\dfrac{1}{n}\sum _{i=1}^{n}W_{\alpha (i)}(I,D_{2}^{opt}(I)) \\&=\dfrac{1}{n}\sum _{i=1}^{n}\left[ \alpha (i)W_{\alpha (i),0}(I,D_{2}^{opt}(I))+(1-\alpha (i))W_{\alpha (i),1}(I,D_{2}^{opt}(I)) \right] \\&=\dfrac{1}{n}\sum _{i=1}^{n}\left[ \alpha (i)W_{\alpha (i),0}(I,A)+(1-\alpha (i))W_{\alpha (i),1}(I,I)\right] \\&=\dfrac{1}{n}\sum _{i=1}^{n}\left[ \alpha (i)\left( V_{I}+V-Kq_{0}(\alpha (i))\right) +(1-\alpha (i))\left( 2V_{I}\right) \right] \\&=\dfrac{1}{n}\sum _{i=1}^{n}\left[ \alpha (i)(V-V_{I})+2V_{I}-K\alpha (i)q_{0}(\alpha (i))\right] \\&={\overline{\alpha }}(V-V_{I})+2V_{I}-K\dfrac{1}{n}\sum _{i=1}^{n}\alpha (i)q_{0}(\alpha (i)) \\&{\mathcal {U}}(A,D_{2}^{opt}(A))={\mathcal {U}}(A,A)=2\left[ V-Kq(\overline{ \alpha })\right] =W_{{\overline{\alpha }}}(A,A) \end{aligned}$$
Moreover
Thus
Since \(U=\frac{V-V_{I}}{K}\), and setting \(S=\frac{1}{2-{\overline{\alpha }}} \left[ 2q({\overline{\alpha }})-\dfrac{1}{n}\sum _{i=1}^{n}\alpha (i)q_{0}(\alpha (i))\right]\), we have:
To achieve the proof of Proposition 8, it is sufficient to set \({\widehat{M}} =\min (S;q_{1}({\overline{\alpha }}))\) and to prove that \(S>M(\overline{\alpha })\).
Setting \(g(\alpha )=\alpha (1-\alpha )\), because \(q_{0}(\alpha )=(1-\delta )p+\delta ^{\prime }(1-\alpha )\),
and \(\left[ g({\overline{\alpha }})-\dfrac{1}{n}\sum _{i=1}^{n}g(\alpha (i)) \right] >0\) since g is a concave function. \(\square\)
Appendix 4: Proofs with Costly Research
Proof of Proposition 9
-
a.
If \(D_{1}=A\) then \(D_{2}^{*}=A\) by irreversibility of authorization.
-
b.
If \(D_{1}=I^{res}\), to determine \(D_{2}^{*}\) we compare \(W_{\alpha ,R}(I^{res},I)\) and \(W_{\alpha ,R}(I^{res},A)\).
$$\begin{aligned}&W_{\alpha ,R}(I^{res},I)=2V_{I}-C \\&W_{\alpha ,R}(I^{res},A)=q_{R}(\alpha )(V_{I}+V-K-C)+(1-q_{R}(\alpha ))(V_{I}+V-C) \\&=V_{I}+V-C-Kq_{R}(\alpha )\\&D_{2}^{*}(I^{res})=A\Leftrightarrow V_{I}+V-C-Kq_{R}(\alpha )>2V_{I}-C\\&D_{2}^{*}(I^{res})=A\Leftrightarrow U>q_{R}(\alpha )\,{\rm since} \,U=\frac{ V-V_{I}}{K} \end{aligned}.$$ -
c.
If \(D_{1}=I^{no}\), to determine \(D_{2}^{*}\) we compare \(W_{\alpha }(I^{no},I)\) and \(W_{\alpha }(I^{no},A)\).
$$\begin{aligned}&W_{\alpha }(I^{no},I)=2V_{I} \\&W_{\alpha }(I^{no},A)=q(\alpha )(V_{I}+V-K)+(1-q(\alpha ))(V_{I}+V)=V_{I}+V-Kq(\alpha ) \\&D_{2}^{*}(I^{no})=A\Leftrightarrow V_{I}+V-Kq(\alpha )>2V_{I} \\&D_{2}^{*}(I^{no})=A\Leftrightarrow U>q(\alpha ) \end{aligned}$$\(\square\)
Proof of Proposition 10
We set \(w_{\alpha }(D_{1})=W_{\alpha }(D_{1},D_{2}^{*}(D_{1})\). To determine \(D_{1}^{*}\), we have to compare \(w_{\alpha }(A)\), \(w_{\alpha }(I^{res})\) and \(w_{\alpha }(I^{no})\).
According to Proposition 9,
Moreover, \(w_{\alpha }(I^{res})=W_{\alpha }(I^{res},D_{2}^{*}(I^{res}))\) thus:
-
If \(U<q_{0}(\alpha )\), then \(w_{\alpha }(I^{res})=W_{\alpha }(I^{res},I)=2V_{I}-C\)
-
If \(U>q_{1}(\alpha )\), then \(w_{\alpha }(I^{res})=W_{\alpha }(I^{res},A)=V_{I}+V-Kq(\alpha )-C\)
-
If \(q_{0}(\alpha )<U<q_{1}(\alpha )\), then \(w_{\alpha }(I^{res})=\alpha W_{\alpha ,0}(I^{res},A)+(1-\alpha )W_{\alpha ,1}(I^{res},I)\)\(=\alpha \left[ V_{I}+V-C-Kq_{0}(\alpha )\right] +(1-\alpha )\left[ 2V_{I}-C \right] =(2-\alpha )V_{I}+\alpha (V-Kq_{0}(\alpha ))-C\)
We have to study the three cases \(U<q_{0}(\alpha )\), \(U>q_{1}(\alpha )\) and \(q_{0}(\alpha )<U<q_{1}(\alpha )\).
-
1.
If \(U<q_{0}(\alpha )\), then \(w_{\alpha }(I^{res})=2V_{I}-C<2V_{I}=w_{\alpha }(I^{no})\)
$$\begin{aligned}&D_{1}^{*}=I^{no}\Leftrightarrow w_{\alpha }(I^{no})>w_{\alpha }(A) \\&D_{1}^{*}=I^{no}\Leftrightarrow 2V_{I}>2V-2Kq(\alpha ) \\&D_{1}^{*}=I^{no}\Leftrightarrow q(\alpha )>U\,\,\hbox {true here since} \,\,q(\alpha )>q_{0}(\alpha ) \end{aligned}$$Conclusion: \(D_{1}^{*}=I^{no}\) if \(U<q_{0}(\alpha )\).
-
2.
If \(U>q_{1}(\alpha )\), then \(w_{\alpha }(I^{res})=V_{I}+V-Kq(\alpha )-C<V_{I}+V-Kq(\alpha )=w_{\alpha }(I^{no})\)
$$\begin{aligned}&D_{1}^{*}=A\Leftrightarrow w_{\alpha }(A)>w_{\alpha }(I^{no}) \\&D_{1}^{*}=A\Leftrightarrow 2V-2Kq(\alpha )>V_{I}+V-Kq(\alpha ) \\&D_{1}^{*}=A\Leftrightarrow U>q(\alpha )\,\, \hbox {true here because} \,\, q_{1}(\alpha )>q(\alpha )\\ \end{aligned}$$Conclusion: \(D_{1}^{*}=A\) if \(U>q_{1}(\alpha )\).
-
3.
If \(q_{0}(\alpha )<U<q_{1}(\alpha )\), then:
$$w_{\alpha }(A)>w_{\alpha }(I^{no})\Leftrightarrow U>q(\alpha )$$and
$$\begin{aligned}&w_{\alpha }(A)>w_{\alpha }(I^{res})\Leftrightarrow 2V-2Kq(\alpha )>(2-\alpha )V_{I}+\alpha (V-Kq_{0}(\alpha ))-C \\&w_{\alpha }(A)>w_{\alpha }(I^{res})\Leftrightarrow (2-\alpha )(V-V_{I})>2Kq(\alpha )-\alpha Kq_{0}(\alpha )-C \\&w_{\alpha }(A)>w_{\alpha }(I^{res})\Leftrightarrow U>M_{C}(\alpha ), \end{aligned}$$setting \(M_{C}(\alpha )=\frac{1}{(2-\alpha )}\left[ 2q(\alpha )-\alpha q_{0}(\alpha )-\frac{C}{K}\right] =M(\alpha )-\frac{C}{(2-\alpha )K} =q(\alpha )+\frac{\alpha (1-\alpha )(\delta -\delta ^{\prime })-\frac{C}{K}}{ 2-\alpha }\)
and if \(U<q(\alpha )\):
This implies that if \(q_{0}(\alpha )<U<q_{1}(\alpha )\):
To find \(D_{1}^{*}\), we just need to compare \(M_{C}(\alpha )\), \(q(\alpha )\) and \(q_{0}(\alpha )+\frac{C}{\alpha K}\).
First Case. We assume that research is very expensive, i.e., \(C>(\frac{ \delta -\delta ^{\prime }}{4})K.\)
Comparing \(q(\alpha )\) and \(M_{C}(\alpha )\):
Let \(h(\alpha )=\alpha (1-\alpha )(\delta -\delta ^{\prime })\) on \(\alpha \in [0;1]\). Then \(\max _{0\le \alpha \le 1}h(\alpha )=h(\frac{1}{2})= \frac{\delta -\delta ^{\prime }}{4}\)
As we are in the first case, \(\max _{0\le \alpha \le 1}h(\alpha )<\frac{C}{K }\), i.e., \(h(\alpha )<\frac{C}{K}\), \(\forall \alpha\).
This implies that \(M_{C}(\alpha )<q(\alpha )\), \(\forall \alpha\).
Comparing \(q(\alpha )\) and \(q_{0}(\alpha )+\frac{C}{\alpha K}\):
This implies that \(q(\alpha )<q_{0}(\alpha )+\frac{C}{\alpha K}\), \(\forall \alpha\).
Conclusion: in the first case, \(M_{C}(\alpha )<q(\alpha )<q_{0}(\alpha )+ \frac{C}{\alpha K}\), \(\forall \alpha\).
Thus \(D_{1}^{*}=A\) if \(U>q(\alpha )\), and \(D_{1}^{*}=I^{no}\) if \(U<q(\alpha )\).
Second Case. We assume that research is not very expensive, i.e., \(C\le ( \frac{\delta -\delta ^{\prime }}{4})K.\)
Comparing \(q(\alpha )\) and \(M_{C}(\alpha )\):
Here \(\max _{0\le \alpha \le 1}h(\alpha )=h(\frac{1}{2})=\frac{\delta -\delta ^{\prime }}{4}\ge \frac{C}{K}\).
Then there exists \(\beta \in [0;\frac{1}{2}]\) with: \(h(\alpha )\ge \frac{C}{K}\) if and only if \(\alpha \in \left[\frac{1}{2}-\beta ;\frac{1}{2 }+\beta \right]\).
This means here that \(M_{C}(\alpha )\ge q(\alpha )\Leftrightarrow \alpha \in \left[\frac{1}{2}-\beta ;\frac{1}{2}+\beta \right]\).
Comparing \(q(\alpha )\) and \(q_{0}(\alpha )+\frac{C}{\alpha K}\):
This means here that \(q(\alpha )\ge q_{0}(\alpha )+\frac{C}{\alpha K} \Leftrightarrow \alpha \in \left[\frac{1}{2}-\beta ;\frac{1}{2}+\beta \right]\)
Conclusion: in the second case,
Thus for \(\alpha \notin \left[\frac{1}{2}-\beta ;\frac{1}{2}+\beta \right]\), \(D_{1}^{*}=A\) if \(U>q(\alpha )\), and \(D_{1}^{*}=I^{no}\) if \(U<q(\alpha )\).
For \(\alpha \in \left[\frac{1}{2}-\beta ;\frac{1}{2}+\beta \right]\):
\(\square\)
Rights and permissions
About this article
Cite this article
Jeleva, M., Rossignol, S. Optimists, Pessimists, and the Precautionary Principle. Environ Resource Econ 74, 367–396 (2019). https://doi.org/10.1007/s10640-019-00322-1
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10640-019-00322-1