Optimal Pollution Standards and Non-compliance in a Dynamic Framework

Abstract

In this paper we present a Stackelberg differential game to study the dynamic interaction between a polluting firm and a regulator who sets pollution limits overtime. At each time, the firm settles emissions taking into account the fine for non-compliance with the pollution limit, and balances current costs of investments in a capital stock which allows for future emission reductions. We derive two main results. First, we show that the optimal pollution limit decreases as the capital stock increases, while both emissions and the level of non-compliance decrease. Second, we find that offering fine discounts in exchange for firm’s capital investment is socially desirable. We numerically obtain the optimal value of such discount, which crucially depends on the severity of the fine. In the limiting scenario with a very large severity of the fine, the optimal discount implies that no penalties are levied, since the firm shows adequate adaptation progress through capital investment.

Appendix

1.1 Proof of Proposition 1

This proof characterizes the stagewise feedback Stackelberg equilibrium strategies (see, for example Haurie et al. 2012, p. 278). Under the assumption of stationary strategies, it involves the resolution of two Hamilton–Jacobi–Bellman equations:

$$\begin{aligned} \rho V_{{{\mathrm{F}}}}^{b}(K)= & {} Y(K,\phi ^{{{\mathrm{E}}}}(K)) -C(\phi ^{{{\mathrm{I}}}}(K))-f\frac{(\phi ^{{{\mathrm{E}}}}(K)-\phi ^{{{\mathrm{L}}}}(K))^{2}}{2}+(V_{{{\mathrm{F}}}}^{b})^{\prime }(K)(\phi ^{{{\mathrm{I}}}}(K)-\delta K), \\ \rho V_{{{\mathrm{R}}}}^{b}(K)= & {} Y(K,\phi ^{{{\mathrm{E}}}}(K)) -C(\phi ^{{{\mathrm{I}}}}(K))-D(\phi ^{{{\mathrm{E}}}}(K))-hf\frac{(\phi ^{{{\mathrm{E}}}}(K)-\phi ^{{{\mathrm{L}}}}(K))^{2}}{2} \\&+(V_{{{\mathrm{R}}}}^{b})^{\prime }(K)(\phi ^{{{\mathrm{I}}}}(K)-\delta K), \end{aligned}$$

where $(\varvec{\phi _{{{\mathrm{F}}}}}(K),\phi _{{{\mathrm{R}}}}(K))$ is a feedback strategy pair, with $\varvec{\phi _{{{\mathrm{F}}}}}(K)=(\phi ^{{{\mathrm{E}}}}(K),\phi ^{{{\mathrm{I}}}}(K))$ and $\phi _{{{\mathrm{R}}}}(K)=\phi ^{{{\mathrm{L}}}}(K)$. To obtain this pair, we first compute the best reaction functions of the firm as the solution to:

$$\begin{aligned} \max _{I,E}\left\{ Y(K,E)-C(I)-f\frac{(E-\phi ^{{{\mathrm{L}}}}(K))^{2}}{2}+(V_{{{\mathrm{F}}}}^{b})^{\prime }(K)(I-\delta K)\right\} . \end{aligned}$$

In view of the linear-quadratic structure of the problem we conjecture a quadratic value function for the firm in K:

$$\begin{aligned} V_{{{\mathrm{F}}}}^{b}(K)=\frac{a_{{{\mathrm{F}}}}^{b}}{2}K^{2}+b_{{{\mathrm{F}}}}^{b}K+c_{{{\mathrm{F}}}}^{b}. \end{aligned}$$

(27)

Therefore, the best-response functions of the firm are then given by:

$$\begin{aligned} \hat{E}^{b}(K;\phi ^{{{\mathrm{L}}}}(K))= & {} \underline{E}^{b}+E^{{{\mathrm{L}}}b}\phi ^{{{\mathrm{L}}}}(K)+E^{{{\mathrm{K}}}b}K,\quad \nonumber \\ \hat{I}^{b}(K;\phi ^{{{\mathrm{L}}}}(K))= & {} \hat{I}^{b}(K)=\underline{I}^{b}+I^{{{\mathrm{L}}}b}\phi ^{{{\mathrm{L}}}}(K)+I^{{{\mathrm{K}}}b}K, \end{aligned}$$

(28)

$$\begin{aligned} \underline{E}^{b}=\frac{\sigma }{\sigma ^{2}+f},\;\;E^{{{\mathrm{L}}}b}= \frac{f}{\sigma }\underline{E}^{b},\;\;E^{{{\mathrm{K}}}b}=-\underline{E} ^{b},\quad \underline{I}^{b}=\frac{b_{{{\mathrm{F}}}}^{b}}{c},\;\;I^{{{\mathrm{L}}}b}=0,\;\;I^{{{\mathrm{K}}}b}=\frac{a_{{{\mathrm{F}}} }^{b}}{c}. \end{aligned}$$

Knowing the best-reaction functions of the firm presented in (28), now the regulator fixes the pollution limit, to maximize:

$$\begin{aligned}&\max _{L}\left\{ Y(K,\hat{E}^{b}(K;L))-C(\hat{I}^{b}(K;L))-D(\hat{E} ^{b}(K;L))-hf\frac{[\hat{E}^{b}(K;L)-L]^{2}}{2}\right. \\&\quad \quad \quad \left. +\, (V_{{{\mathrm{R}}}}^{b})^{\prime }(K)(\hat{I}^{b}(K;L)-\delta K)\right\} . \end{aligned}$$

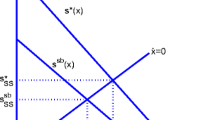
Notice, however that $I^{{{\mathrm{L}}}b}=0$ and therefore, $\hat{I} ^{b}(K;L)$ does not actually depend on L. The regulator cannot directly influence investment, and hence behaves as a static player. His optimal strategy is then to set an emission limit which satisfies:

$$\begin{aligned} Y_{E}(K,\hat{E}^{b}(K;L))=D^{\prime }(\hat{E}^{b}(K;L))+hf[\hat{E} ^{b}(K;L)-L]\frac{E^{{{\mathrm{L}}}b}-1}{E^{{{\mathrm{L}}}b}}. \end{aligned}$$

From this condition, the optimal emission limit is the affine function of the stock of capital, $L^{*b}(K)$, given in (5). As long as $h<1$, it follows that $\left( L_{0}^{*b}\right) _{f}>0$.

From the optimal emission limit in (5) and the best-response functions of the firm, the optimal emissions and investment are given by the expressions in (6) and (7). And it is easy to see that $\left( E_{0}^{*b}\right) _{f}<0$ for all f. Further, from the Riccati equations the coefficients which define the firm’s value function, $V_{{{\mathrm{F}}}}^{b}(K)$, defined in (27) can now be computed and are given by (8) and:

$$\begin{aligned} c_{{{\mathrm{F}}}}^{b} = \frac{(b_{{{\mathrm{F}}}}^{b})^{2}+c}{2c\rho }-\frac{d^{2}f(f+\sigma ^{2})}{2\rho \Psi ^{2}}>0. \end{aligned}$$

(29)

Once the optimal investment strategy is known, integrating the differential equation which defines the capital stock dynamics, the optimal time path of the capital stock is given by (10).

1.2 Proof of Proposition 2

We first characterize the optimal strategies for the case of no regulation and the first-best scenario, and then we compare these two cases with the stagewise feedback Stackelberg equilibrium characterized in Proposition 1.

First, with no regulator the Hamilton–Jacobi–Bellman equation for problem (17) is:

$$\begin{aligned} \rho V_{{{\mathrm{F}}}}^{{{\mathrm{NR}}}}(K)=\max _{I,E}\left\{ K+\sigma E-\frac{(K+\sigma E)^{2}}{2}-c\frac{I^{2}}{2}+(V_{{{\mathrm{F}}}}^{{{\mathrm{NR}}}})^{\prime }(K)(I-\delta K)\right\} . \end{aligned}$$

We again conjecture a quadratic value function in K, denoted by $V_{{{\mathrm{F}}}}^{{{\mathrm{NR}}}}(K)$:

$$\begin{aligned} V_{{{\mathrm{F}}}}^{{{\mathrm{NR}}}}(K)=\frac{a_{{{\mathrm{F}}}}^{{{\mathrm{NR}}}}}{2}K^{2}+b_{{{\mathrm{F}}}}^{{{\mathrm{NR}}}}K+c_{{{\mathrm{F}}}}^{{{\mathrm{NR}}}}, \end{aligned}$$

and $a_{{{\mathrm{F}}}}^{{{\mathrm{NR}}}},b_{{{\mathrm{F}}}}^{{{\mathrm{NR}}}},c_{{{\mathrm{F}}}}^{{{\mathrm{NR}}}}$ are unknowns to be determined. The only feasible solution for these unknowns associated with a convergent time path of the capital stock is: $a_{{{\mathrm{F}}}}^{{{\mathrm{NR}}}}=b_{{{\mathrm{F}}}}^{{{\mathrm{NR}}}}=0,c_{{{\mathrm{F}}}}^{{{\mathrm{NR}}}}=1/(2\rho )$. Therefore, the solution under no regulation is the following:

$$\begin{aligned} I^{*{{\mathrm{NR}}}}=0,\quad E^{*{{\mathrm{NR}}}}(K)=\frac{1}{\sigma }(1-K),\quad \hbox {and}\quad K^{{{\mathrm{NR}}}}(t)=k_{0}e^{-\delta t}, \end{aligned}$$

(30)

where the optimal time path of capital is obtained by solving (2) once the optimal investment strategy has been replaced.

In the particular case $k_{0}=0$, then $K(t)=0$, $E(t)=1/\sigma$ for any $t\ge 0$ and the social welfare is $V^{{{\mathrm{NR}}}}(0)=1/(2\rho )-d/(2\rho \sigma ^{2})$.

The first-best solution to problem (18) can be found by solving the following Hamilton–Jacobi–Bellman equation:

$$\begin{aligned} \rho V^{{{\mathrm{FB}}}}(K)=\max _{I,E}\left\{ (K+\sigma E)-\frac{(K+\sigma E)^{2}}{2}-c\frac{I^{2}}{2}-d\frac{E^{2}}{2}+(V^{{{\mathrm{FB}}}})^{\prime }(K)(I-\delta K)\right\} , \end{aligned}$$

where $V^{{{\mathrm{FB}}}}(K)$ denotes the value function of the problem. Following the same procedure as above, we then easily obtain the following optimal strategies:

$$\begin{aligned} I^{*{{\mathrm{FB}}}}(K)=\frac{b^{{{\mathrm{FB}}}}}{c}+\frac{a^{{{\mathrm{FB}}}}}{c}K,\quad E^{*{{{\mathrm{FB}}}}}(K)=\frac{\sigma }{d+\sigma ^{2}}(1-K), \end{aligned}$$

(31)

where $V^{{{\mathrm{FB}}}}(K)=a^{{{\mathrm{FB}}}}K^{2}/2+b^{{{\mathrm{FB}}}}K+c^{{{\mathrm{FB}}}}$, with

$$\begin{aligned}&a^{{{\mathrm{FB}}}} = \frac{c(\rho + 2\delta ) - \sqrt{ \Delta ^{{{\mathrm{FB}}}}}}{2}<0,\quad b^{{{\mathrm{FB}}}}=\frac{2cd}{(d+\sigma ^{2})\left[ c\rho +\sqrt{\Delta ^{{{\mathrm{FB}}}}}\right] }>0, \\&c^{{{\mathrm{FB}}}} = \frac{(b^{{{\mathrm{FB}}}})^{2}}{2c\rho }+\frac{\sigma ^{2}}{2\rho (d+\sigma ^{2})}>0,\quad \Delta ^{{{\mathrm{FB}}}}=c^{2}(\rho +2\delta )^{2}+\frac{4cd}{d+\sigma ^{2}}, \end{aligned}$$

and the optimal time-path of the capital stock is:

$$\begin{aligned} K^{{{\mathrm{FB}}}}(t)=(k_{0}-\bar{K}^{{{\mathrm{FB}}}})e^{\theta ^{{{\mathrm{FB}}}}t}+\bar{K}^{{{\mathrm{FB}}}}, \end{aligned}$$

(32)

$$\begin{aligned} \bar{K}^{{{\mathrm{FB}}}}=\frac{d}{d+c(d+\sigma ^{2})\delta (\delta +\rho )}>0,\quad \theta ^{{{\mathrm{FB}}}}=\frac{c\rho - \sqrt{ \Delta ^{{{\mathrm{FB}}}}}}{2c}<0, \end{aligned}$$

where $\bar{K}^{{{\mathrm{FB}}}}$ is the long-run value of the capital stock, and $|\theta ^{{{\mathrm{FB}}}}|$ is the speed of convergence towards this value.

The corresponding long-run values of emissions and investment read:

$$\begin{aligned} \bar{E}^{{{\mathrm{FB}}}}=\frac{\sigma }{d+\sigma ^{2}}\left( 1-\bar{K}^{{{\mathrm{FB}}}}\right) =\frac{c\delta (\delta +\rho )\sigma }{d+c(d+\sigma ^{2})\delta (\delta +\rho )},\quad \bar{I}^{{{\mathrm{FB}}} }=\delta \bar{K}^{{{\mathrm{FB}}}}=\frac{d\delta }{d+c(d+\sigma ^{2})\delta (\delta +\rho )}. \end{aligned}$$

Finally, we compare the optimal time paths under no regulation, the first best and the base model.

• 1. Since $f\ge d,$ it follows that $\bar{K}^{{{\mathrm{FB}}}}> \bar{K}^{b}$ and both are positive. Moreover, under this condition it is also true that $\Delta ^{{{\mathrm{FB}}}}>\Delta ^{b}>0$, hence inequality $\theta ^{{{\mathrm{FB}}}}<\theta ^{b}$ follows. It is immediate to verify that $\theta ^{b}<-\delta$. Then $K^{{{\mathrm{FB}}}}(t)>K^{b}(t)>K^{{{\mathrm{NR}}}}(t)$ for all $t>0$ follows.

• 2. $\bar{I}^{{{\mathrm{FB}}}}>\bar{I}^{b}>0$ follows straightforwardly from part (1). Since $f\ge d$, then $a^{{{\mathrm{FB}}}}<a_{{{\mathrm{F}}}}^{b}<0$ immediately follows from $\Delta ^{{{\mathrm{FB}}}}>\Delta ^{b}$. Moreover, proving $b^{{{\mathrm{FB}}}}>b_{{{\mathrm{F}}}}^{b}$ is equivalent to proving:

  $$\begin{aligned} \frac{\Delta ^{{{\mathrm{FB}}}}-c^{2}(\rho +2\delta )^{2}}{2cd(c\rho + \sqrt{\Delta ^{{{\mathrm{FB}}}}})}>\frac{\Delta ^{b}-c^{2}(\rho +2\delta )^{2}}{2cd(c\rho +\sqrt{\Delta ^{b}})}. \end{aligned}$$

  Provided that $f(x)=(x-c^{2}(\rho +2\delta )^{2})/(c\rho +\sqrt{x})$ is an increasing function and $\Delta ^{{{\mathrm{FB}}}}>\Delta ^{b}$, $b^{{{\mathrm{FB}}}}>b_{{{\mathrm{F}}}}^{b}$ follows. Thus, $I^{*{{\mathrm{FB}}}}(0)=b^{{{\mathrm{FB}}}}/c>b_{{{\mathrm{F}}} }^{b}/c=I^{*b}(0)>0$. However, nothing can be said of the comparison between $I^{b}(t)$ and $I^{{{\mathrm{FB}}}}(t)$ for $t>0$.

• 3. The results immediately follow from part (1), the expressions of $E^{*{{\mathrm{NR}}}}(K),$ $E^{*{{\mathrm{FB}}}}(K)$, $E^{*b}(K)$, and inequalities:

  $$\begin{aligned} \frac{1}{\sigma }>\frac{\sigma (f+h\sigma ^{2})}{\Psi }>\frac{\sigma }{d+\sigma ^{2}}. \end{aligned}$$

1.3 Proof of Proposition 3

• 1. From (8), (29) and (11) it follows that sign$|\theta ^{b}|_{f}=$sign$(\bar{K}^{b})_{f}=$sign$(\bar{I}^{b})_{f}$ is given by:

  $$\begin{aligned} \hbox {sign}(h\sigma ^{2}(2f+\sigma ^{2})-(d+\sigma ^{2})f)= \hbox {sign}(2h\sigma ^{2}(f+\sigma ^{2})-\Psi ). \end{aligned}$$

  (33)

  Here and henceforth we will be assuming $d+\sigma ^{2}(1-2h)>0$, i.e. $h<(d+\sigma ^{2})/(2\sigma ^{2})=h_{\max }$. Under this condition, the sign in (33) is positive for $f\in [d,\hat{f}_{K}^{b})$, and negative for $f>\hat{f}_{K}^{b}$, with $\hat{f}_{K}^{b}$ given in (19).³⁷

  The effect on emissions is obtained from (13),

  $$\begin{aligned} (\bar{E}^{b})_{f}=-\frac{dh\sigma ^{3}}{\Psi ^{2}}\left( 1-\bar{K}^{b}\right) -\frac{\sigma (f+h\sigma ^{2})}{\Psi }(\bar{K}^{b})_{f}. \end{aligned}$$

  In consequence, if $(\bar{K}^{b})_{f}>0$ then $(\bar{E}^{b})_{f}<0$, implying $\hat{f}_{E}^{b}>\hat{f}_{K}^{b}>0$ for all $h>0$. To prove that $\hat{f}_{E}^{b}$ is finite it is sufficient to see than $(\bar{E}^{b})_{f}$ is positive for sufficiently large values of f: $\lim _{f\rightarrow \infty }(\bar{E}^{b})_{f}=\infty$.

• 2. The effect of f on the emission limits can be computed from (12):

  $$\begin{aligned} (\bar{L}^{b})_{f}=\frac{d\sigma (d+(1-h)\sigma ^{2})}{\Psi ^{2}}\left( 1-\bar{K}^{b}\right) -\frac{\sigma (-d+f+h\sigma ^{2})}{\Psi }(\bar{K}^{b})_{f}, \end{aligned}$$

  (34)

  where $1-\bar{K}^{b}$ can be written as a function of $(\bar{K}^{b})_{f}$, and then:

  $$\begin{aligned} (\bar{L}^{b})_{f}=\left\{ \frac{[d+(1-h)\sigma ^{2}][d^{2}f(f+\sigma ^{2})+c\delta (\delta +\rho )\Psi ^{2}]}{\Psi d\sigma (2h\sigma ^{2}(f+\sigma ^{2})-\Psi )}-\frac{\sigma (f+h\sigma ^{2}-d)}{\Psi }\right\} (\bar{K}^{b})_{f}. \end{aligned}$$

  We know that $(\bar{K}^{b})_{f}<0$ if and only if $f>\hat{f}_{K}^{b}$, i.e. $2h\sigma ^{2}(f+\sigma ^{2})-\Psi <0$. Then, a sufficient condition for the expression in brackets to be negative is $f+h\sigma ^{2}-d>0$. In consequence, if $\hat{f}_{K}^{b}>d$, then $(\bar{K}^{b})_{f}<0$ implies $(\bar{L}^{b})_{f}>0$ and therefore $\hat{f}_{L}^{b}<\hat{f}_{K}^{b}$. It is easy to prove that

  $$\begin{aligned} \hat{f}_{K}^{b}>d\Leftrightarrow h>\frac{2d}{2d+\sigma ^{2}}\frac{d+\sigma ^{2}}{2\sigma ^{2}}\equiv h_{min}. \end{aligned}$$

  Thus $(\bar{L}^{b})_{f}>0$ for all $f>\hat{f}_{L}^{b}$. To study the sign of $(\bar{L}^{b})_{f}$ for $f\in [0,\hat{f}_{L}^{b})$, expression (34) can be re-written as an expression which sign is given by a second-order convex polynomial in f. Depending on whether this polynomial has no real roots or it has two roots with different or equal sign, the different behaviour in footnote 23 appear.

• 3. The derivative $(\bar{E}^{b}-\bar{L}^{b})_{f}$ can be computed and proved negative for any positive h.

1.4 Proof of Proposition 4

For the dynamic maximization problem for the firm described in (3), assuming a quadratic value function for the firm, $V_{{{\mathrm{F}}}}(K)$ in (24), and following the same reasoning as in the proof of Proposition 1, the best-response functions of the firm are given by:

$$\begin{aligned} \hat{E}(K;\zeta ^{{{\mathrm{L}}}}(K))=\underline{E}+E^{{{\mathrm{L}}}}\zeta ^{{{\mathrm{L}}}}(K)+E^{{{\mathrm{K}}}}K,\quad \hat{I}(K;\zeta ^{{{\mathrm{L}}}}(K))=\underline{I}+I^{{{\mathrm{L}}}}\zeta ^{{{\mathrm{L}}}}(K)+I^{{{\mathrm{K}}}}K, \end{aligned}$$

(35)

where $(\varvec{\zeta _{{{\mathrm{F}}}}}(K),\zeta _{{{\mathrm{R}}}}(K))$ is a feedback strategy pair, with $\varvec{\zeta _{{{\mathrm{F}}}}}(K)=(\zeta ^{{{\mathrm{E}}}}(K),\zeta ^{{{\mathrm{I}}}}(K))$ and $\zeta _{{{\mathrm{R}}}}(K)=\zeta ^{{{\mathrm{L}}}}(K)$ and:

$$\begin{aligned}&E^{{{\mathrm{L}}}}=\frac{cf}{\Omega },\quad \underline{E}=\frac{\sigma }{f}E^{{{\mathrm{L}}}}+\frac{f\beta (b_{{{\mathrm{F}}}}+\beta \sigma )}{\Omega },\quad E^{{{\mathrm{K}}}}=\frac{E^{{{\mathrm{L}}}}-1}{\sigma }+\frac{f\beta (a_{{{\mathrm{F}}}}-c\delta )}{\Omega }, \\&I^{{{\mathrm{L}}}}=-\frac{f\beta \sigma ^{2}}{\Omega },\quad \underline{I}=-\frac{I^{{{\mathrm{L}}}}}{\sigma }+\frac{b_{{{\mathrm{F}}}}(f+\sigma ^{2})}{\Omega },\quad I^{{{\mathrm{K}}}}=\frac{I^{{{\mathrm{L}}}}(1-\beta \delta \sigma )}{\sigma }+ \frac{a_{{{\mathrm{F}}}}(f+\sigma ^{2})}{\Omega }, \end{aligned}$$

with $\Omega =cf+(c+f\beta ^{2})\sigma ^{2},$ $E^{{{\mathrm{L}}}}\in (0,1)$, and $I^{{{\mathrm{L}}}}<0$. This last inequality is the main difference with the base model with $\beta =0$ where $I^{{{\mathrm{L}}}b}=0$ (see (28)). Therefore, the regulator, taking into account the best-response functions in (35), now behaves as a dynamic player. It fixes the pollution limit in order to maximize:

$$\begin{aligned}&\max _{L} \left\{ Y(K,\hat{E}(K;L)) - C(\hat{I}(K;L)) - D(\hat{E}(K;L))\right. \\&\left. -\,\, hf\frac{[\hat{E}(K;L) - (L + \beta (\hat{I}(K;L) - \delta K))]^{2}}{2} +(V_{{{\mathrm{R}}}}^{b})^{\prime }(K)(\hat{I}(K;L)-\delta K)\right\} . \end{aligned}$$

Assuming a quadratic value function for the regulator, $V_{{{\mathrm{R}}}}(K)$ in (24), the optimal emission standard strategy, $L^*(K)$ in (23) follows. The optimal emissions and investment strategies, $E^*(K)$ and $I^*(K)$ in (23) immediately follow from the best-response functions in (35) once $L^*(K)$ is known.

From the optimal investment strategy in (23) and the capital stock dynamics in (2), the optimal time path of the capital stock can be written as in (25).

1.5 Proof of Proposition 5

For $g(f,\eta )=f$, the optimal strategies of the problem in Sect. 5 are:

$$\begin{aligned}&E^{{{\mathrm{S}}}}(K)=\sigma \frac{(f+h\sigma ^{2})(1-K)-\eta fh\sigma }{\Psi },\quad I^{{{\mathrm{S}}}}(K)=\frac{(V_{{{\mathrm{F}}}}^{{{\mathrm{S}}}})^{\prime }(K)}{c}, \end{aligned}$$

(36)

$$\begin{aligned}&L^{{{\mathrm{S}}}}(K)=\frac{(f-d+h\sigma ^{2})(1-K)+\eta f(d+(1-h)\sigma ^{2})}{\Psi }, \end{aligned}$$

(37)

with $V_{{{\mathrm{F}}}}^{{{\mathrm{S}}}}(K)$ being the value function of the firm when a subsidy for over-compliance exists. The optimal time path of the capital stock reads:

$$\begin{aligned} K^{{{\mathrm{S}}}}(t)=(k_{0}-\bar{K}^{{{\mathrm{S}}}})e^{\theta ^{b}t}+\bar{K}^{{{\mathrm{S}}}},\quad \bar{K}^{{{\mathrm{S}}}}=\frac{df(f+\sigma ^{2})(d+h\eta \sigma ^{3})}{d^{2}f(f+\sigma ^{2})+4c\delta (\rho +\delta )\Psi ^{2}}>\bar{K}^{b}. \end{aligned}$$

(38)

The capital stock grows higher than in the case without a subsidy, and the speed of convergence is identical, $\theta ^{b}$. Assuming the same initial value, $k_{0}$, it follows that $K^{b}(t)<K^{{{\mathrm{S}}}}(t)<\bar{K}^{{{\mathrm{S}}}}$ for all $t>0$.

From (36), an upper bound for $\eta$ ensures non-negative emissions. This bound is minimum for the long-run capital stock. Hence, a necessary and sufficient condition which guarantees positive emissions along the whole planning horizon is:

$$\begin{aligned} \eta \le \bar{\eta }\equiv \frac{c\delta (\rho +\delta )(f+h\sigma ^{2})\Psi }{fh\sigma [d(f+\sigma ^{2})+c\delta (\rho +\delta )\Psi ]}, \end{aligned}$$

with $\bar{\eta }$ being the value of $\eta$ for which emissions are null at the steady state. This condition also ensures that $\bar{K}^{{{\mathrm{S}}}}<1$.

Focusing on the starting date, we define $\eta ^0$ as the value of $\eta$ for which full compliance initially holds, that is, $N(k_0,\eta ^0)=0$:

$$\begin{aligned} \eta ^0=\frac{d\sigma (1-k_0)}{f(d+\sigma ^2)}. \end{aligned}$$

Since $N^{{{\mathrm{S}}}}_\eta <0$, then the firm initially over-complies if $\eta >\eta ^0$ and vice versa. Furthermore, since $N^{{{\mathrm{S}}}}_K<0$ and K grows with time, then if the firm initially over-complies, $\eta >\eta ^0$, it will over-comply henceforth.

Focusing on the steady state, $\bar{K}^{{{\mathrm{S}}}}(\eta )$, we define $\tilde{\eta }$ as the value of $\eta$ for which full compliance holds, that is, $N(\bar{K}^{{{\mathrm{S}}}}({\tilde{\eta }}),{\tilde{\eta }})=0$:

$$\begin{aligned} {\tilde{\eta }} =\frac{dc\delta (\rho +\delta )\sigma \Psi }{f[d^2(f+\sigma ^2)+c\delta (\rho +\delta )(\sigma ^2+d)\Psi ]}<\eta ^0. \end{aligned}$$

Since $dN^{{{\mathrm{S}}}}/d\eta <0$, then at the steady state the firm over-complies if $\eta >{\tilde{\eta }}$ and vice versa. Further, since $N^{{{\mathrm{S}}}}_K<0$ and K grows with time, then if the firm ends-up with non-compliance, $\eta \le {\tilde{\eta }}$, the firm does not comply along the whole period.

From the behaviour at the initial time and at the steady state, and the fact that ${\tilde{\eta }}<\min \left\{ \eta ^0,\bar{\eta }\right\}$, the results in Proposition 5 follow.

1.6 Proof of Proposition 6

This proof distinguishes two cases depending on the value of $\eta :$

• (a) $\eta \le \tilde{\eta }\quad$ (Non-compliance for all $t\ge 0$)

  $$\begin{aligned} m(K^{b}(t),K^{{{\mathrm{S}}}}(t),\eta )=N^{{{\mathrm{S}}}}(K^{b}(t),0)-N^{{{\mathrm{S}}}}(K^{{{\mathrm{S}}}}(t),\eta )=\frac{d\sigma (K^{{{\mathrm{S}}}}(t)-K^{b}(t))}{\Psi }+\eta \frac{f(d+\sigma ^{2})}{\Psi }. \end{aligned}$$

  Since $K^{{{\mathrm{S}}}}(t)>K^{b}(t)$ for all $t>0$, then $m(K^{b}(t),K^{{{\mathrm{S}}}}(t),\eta )>0$ for all $t\ge 0$ and the consideration of the subsidy increases the effectiveness of the enforcement mechanism along the whole period.

• (b) ${\tilde{\eta }}<\eta$

  • (b1) $\tilde{\eta }<\eta <\eta ^{0}$ (Non-compliance for $t\in [0,\hat{t})$ and over-compliance for $t\ge \hat{t}$)Within the interval $[0,\hat{t})$ effectiveness increases following the argument in case a).

    Within the interval $[\hat{t},\infty )$ function m reads:

    $$\begin{aligned} m(K^{b}(t),K^{{{\mathrm{S}}}}(t),\eta )= & {} N^{{{\mathrm{S}}}}(K^{b}(t),0)+N^{{{\mathrm{S}}}}(K^{{{\mathrm{S}}}}(t),\eta )\nonumber \\= & {} \frac{d\sigma (2-K^{{{\mathrm{S}}}}(t)-K^{b}(t))}{\Psi }-\eta \frac{f(d+\sigma ^{2})}{\Psi }. \end{aligned}$$

    (39)

    At time $\hat{t}$, $N^{{{\mathrm{S}}}}(K^{{{\mathrm{S}}}}(\hat{t}),\eta )=0$ and therefore, $m(K^{b}(\hat{t}),K^{{{\mathrm{S}}}}(\hat{t}),\eta )>0$. Taking into account (10) and (38), it immediately follows that $m(K^{b}(t),K^{{{\mathrm{S}}}}(t),\eta )$ is monotonously decreasing with time. Finally, at the steady state $m(\bar{K}^{b},\bar{K}^{{{\mathrm{S}}}}(\eta ),\eta )$ is a decreasing function of $\eta$ which vanishes for $\eta =2\tilde{\eta }$. Therefore:

    • 1. If $\eta \le 2{\tilde{\eta }}$, then $m(\bar{K}^{b},\bar{K}^{{{\mathrm{S}}}}(\eta ),\eta )\ge 0$ and since m is decreasing with time, $m(\bar{K}^{b},\bar{K}^{{{\mathrm{S}}}}(\eta ),\eta )>0$ for all $t\in [\hat{t},\infty )$. In consequence, the effectiveness of the enforcement mechanism increases for the whole period.

    • 2. If $\eta >2\tilde{\eta }$, then $m(\bar{K}^{b},\bar{K}^{{{\mathrm{S}}}}(\eta ),\eta )<0$ but, at time $\hat{t},$ $m(K^{b}(\hat{t}),K^{{{\mathrm{S}}}}(\hat{t}),\eta )>0$. In consequence, the effectiveness is improved within a first interval longer than $[0,\hat{t})$, but worsens from a given time on.

  • (b2) ${\tilde{\eta }}<\eta ^0\le \eta \quad$(Over-compliance for all $t> 0$)

    Function m is given in Eq. (39). At time 0, $m(k_{0},k_{0},\eta )$ is positive if and only if $\eta <2\eta ^{0}$. Under this condition, the same reasoning as in the case b1) for the interval $[\hat{t},\infty )$ now applies for $[0,\infty )$. Therefore either the effectiveness of the enforcement mechanism increases for the whole period if $\eta \le 2\tilde{\eta }$, or it increases within a first period but worsens henceforth, if $\eta >2\tilde{\eta }$.Finally, $\eta >2\eta ^{0}$ implies $m(k_{0},k_{0},\eta )<0$ and since this function decreases with time it will remain negative. Therefore, the consideration of the subsidy would imply a reduction in the effectiveness of the enforcement mechanism along the whole period.