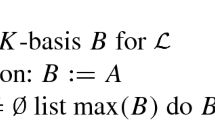Skip to main content
SpringerLink
Log in

Evaluation codes and their basic parameters

  • Published:
Designs, Codes and Cryptography Aims and scope Submit manuscript

Abstract

The aim of this work is to give degree formulas for the generalized Hamming weights of evaluation codes and to show lower bounds for these weights. In particular, we give degree formulas for the generalized Hamming weights of Reed–Muller-type codes, and we determine the minimum distance of toric codes over hypersimplices, and the 1st and 2nd generalized Hamming weights of squarefree evaluation codes.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

References

  1. Aigner M.: Combinatorial Theory. Springer, Berlin (1997).

    MATH  Google Scholar 

  2. Atiyah M.F., Macdonald I.G.: Introduction to Commutative Algebra. Addison-Wesley, Reading, MA (1969).

    MATH  Google Scholar 

  3. Ballico E., Elia M., Sala M.: On the evaluation of multivariate polynomials over finite fields. J. Symb. Comput. 50, 255–262 (2013).

    MathSciNet  MATH  Google Scholar 

  4. Becker T., Weispfenning V.: Gröbner Bases A Computational Approach to Commutative Algebra, in Cooperation with Heinz Kredel. Graduate Texts in Mathematics, vol. 141. Springer, New York (1993).

  5. Beelen P.: A note on the generalized Hamming weights of Reed–Muller codes. Appl. Algebra Eng. Commun. Comput. 30(3), 233–242 (2019).

    MathSciNet  MATH  Google Scholar 

  6. Beelen P., Datta M.: Generalized Hamming weights of affine Cartesian codes. Finite Fields Appl. 51, 130–145 (2018).

    MathSciNet  MATH  Google Scholar 

  7. Bosma W., Cannon J., Playoust C.: The Magma algebra system. I. The user language. J. Symb. Comput. 24, 235–265 (1997).

    MathSciNet  MATH  Google Scholar 

  8. Carvalho C.: On the second Hamming weight of some Reed–Muller type codes. Finite Fields Appl. 24, 88–94 (2013).

    MathSciNet  MATH  Google Scholar 

  9. Carvalho C., Lopez Neumann V.G., López H.H.: Projective nested cartesian codes. Bull. Braz. Math. Soc. 48(2), 283–302 (2017).

    MathSciNet  MATH  Google Scholar 

  10. Cooper S.M., Seceleanu A., Tohǎneanu S.O., Vaz Pinto M., Villarreal R.H.: Generalized minimum distance functions and algebraic invariants of Geramita ideals. Adv. Appl. Math. 112, 101940 (2020).

    MathSciNet  MATH  Google Scholar 

  11. Cox D., Little J., O’Shea D.: Ideals, Varieties, and Algorithms. Springer, Berlin (1992).

    MATH  Google Scholar 

  12. Duursma I.M., Rentería C., Tapia-Recillas H.: Reed–Muller codes on complete intersections. Appl. Algebra Eng. Commun. Comput. 11(6), 455–462 (2001).

    MathSciNet  MATH  Google Scholar 

  13. Geil O.: Evaluation codes from an affine variety code perspective. In: Advances in Algebraic Geometry Codes. Series on Coding Theory and Cryptology, vol. 5, pp. 153–180. World Scientific Publishing, Hackensack, NJ (2008).

  14. Geil O., Høholdt T.: Footprints or generalized Bezout’s theorem. IEEE Trans. Inf. Theory 46(2), 635–641 (2000).

    MathSciNet  MATH  Google Scholar 

  15. Geil O., Pellikaan R.: On the structure of order domains. Finite Fields Appl. 8(3), 369–396 (2002).

    MathSciNet  MATH  Google Scholar 

  16. Geramita A.V., Kreuzer M., Robbiano L.: Cayley–Bacharach schemes and their canonical modules. Trans. Am. Math. Soc. 339(1), 163–189 (1993).

    MathSciNet  MATH  Google Scholar 

  17. Ghorpade S.: A note on Nullstellensatz over finite fields. In: Contributions in Algebra and Algebraic Geometry. Contemporary Mathematics, vol. 738, pp. 23–32. American Mathematical Society, Providence, RI (2019).

  18. González-Sarabia M., Camps E., Sarmiento E., Villarreal R.H.: The second generalized Hamming weight of some evaluation codes arising from a projective torus. Finite Fields Appl. 52, 370–394 (2018).

    MathSciNet  MATH  Google Scholar 

  19. González-Sarabia M., Martínez-Bernal J., Villarreal R.H., Vivares C.E.: Generalized minimum distance functions. J. Algebr. Comb. 50(3), 317–346 (2019).

    MathSciNet  MATH  Google Scholar 

  20. González-Sarabia M., Rentería C., Hernández de la Torre M.: Minimum distance and second generalized Hamming weight of two particular linear codes. Congr. Numer. 161, 105–116 (2003).

    MathSciNet  MATH  Google Scholar 

  21. González-Sarabia M., Rentería C., Tapia-Recillas H.: Reed–Muller-type codes over the Segre variety. Finite Fields Appl. 8(4), 511–518 (2002).

    MathSciNet  MATH  Google Scholar 

  22. Grayson D., Stillman M.: Macaulay\(2\). Available at http://www.math.uiuc.edu/Macaulay2/ (1996).

  23. Greuel G.M., Pfister G.: A Singular Introduction to Commutative Algebra, 2nd extended edn. Springer, Berlin (2008).

    Google Scholar 

  24. Hansen J.: Toric surfaces and error-correcting codes. In: Coding Theory, Cryptography, and Related Areas, pp. 132–142. Springer, Berlin (2000).

  25. Heijnen P., Pellikaan R.: Generalized Hamming weights of \(q\)-ary Reed–Muller codes. IEEE Trans. Inf. Theory 44(1), 181–196 (1998).

    MathSciNet  MATH  Google Scholar 

  26. Helleseth T., Kløve T., Mykkelveit J.: The weight distribution of irreducible cyclic codes with block lengths \(n_1((q^l-1)/N)\). Discret. Math. 18, 179–211 (1977).

    MATH  Google Scholar 

  27. Huffman W.C., Pless V.: Fundamentals of Error-Correcting Codes. Cambridge University Press, Cambridge (2003).

    MATH  Google Scholar 

  28. Jacobson N.: Basic Algebra I, 2nd edn. W. H. Freeman and Company, New York (1996).

    MATH  Google Scholar 

  29. Johnsen T., Verdure H.: Generalized Hamming weights for almost affine codes. IEEE Trans. Inf. Theory 63(4), 1941–1953 (2017).

    MathSciNet  MATH  Google Scholar 

  30. Joyner D.: Toric codes over finite fields. Appl. Algebra Eng. Commun. Comput. 15(1), 63–79 (2004).

    MathSciNet  MATH  Google Scholar 

  31. Koblitz N.: A Course in Number Theory and Cryptography, 2nd edn. Graduate Texts in Mathematics, vol. 114. Springer, New York (1994).

  32. Kreuzer M., Robbiano L.: Computational Commutative Algebra 2. Springer, Berlin (2005).

    MATH  Google Scholar 

  33. Kreuzer M., Robbiano L.: Computational Linear and Commutative Algebra. Springer, Cham (2016).

    MATH  Google Scholar 

  34. Lachaud G.: The parameters of projective Reed–Muller codes. Discret. Math. 81(2), 217–221 (1990).

    MathSciNet  MATH  Google Scholar 

  35. Little J.: Remarks on generalized toric codes. Finite Fields Appl. 24, 1–14 (2013).

    MathSciNet  MATH  Google Scholar 

  36. López H.H., Matthews G., Soprunov I.: Monomial-Cartesian codes and their duals, with applications to LCD codes, quantum codes, and locally recoverable codes. Des. Codes Cryptogr. 88(8), 1673–1685 (2020).

    MathSciNet  MATH  Google Scholar 

  37. López H.H., Rentería C., Villarreal R.H.: Affine cartesian codes. Des. Codes Cryptogr. 71(1), 5–19 (2014).

    MathSciNet  MATH  Google Scholar 

  38. López H.H., Sarmiento E., Vaz Pinto M., Villarreal R.H.: Parameterized affine codes. Stud. Sci. Math. Hung. 49(3), 406–418 (2012).

    MathSciNet  MATH  Google Scholar 

  39. MacWilliams F.J., Sloane N.J.A.: The Theory of Error-Correcting Codes. North-Holland, Amsterdam (1977).

    MATH  Google Scholar 

  40. Martínez-Bernal J., Pitones Y., Villarreal R.H.: Minimum distance functions of graded ideals and Reed–Muller-type codes. J. Pure Appl. Algebra 221, 251–275 (2017).

    MathSciNet  MATH  Google Scholar 

  41. Martínez-Bernal J., Pitones Y., Villarreal R.H.: Minimum distance functions of complete intersections. J. Algebra Appl. 17(11), 1850204 (2018).

    MathSciNet  MATH  Google Scholar 

  42. Martínez-Bernal J., Valencia-Bucio M.A., Villarreal R.H.: Linear codes over signed graphs. Des. Codes Cryptogr. 88(2), 273–296 (2020).

    MathSciNet  MATH  Google Scholar 

  43. Mercier D.J., Rolland R.: Polynômes homogènes qui s’annulent sur l’espace projectif \({\rm P}^m({ F}_q)\). J. Pure Appl. Algebra 124(1–3), 227–240 (1998).

    MathSciNet  MATH  Google Scholar 

  44. O’Carroll L., Planas-Vilanova F., Villarreal R.H.: Degree and algebraic properties of lattice and matrix ideals. SIAM J. Discret. Math. 28(1), 394–427 (2014).

    MathSciNet  MATH  Google Scholar 

  45. Patanker N., Singh S.K.: Generalized Hamming weights of toric codes over hypersimplices and square-free affine evaluation codes. Preprint (2020). arXiv:2002.10920 [math.AC].

  46. Ruano D.: On the structure of generalized toric codes. J. Symb. Comput. 44(5), 499–506 (2009).

    MathSciNet  MATH  Google Scholar 

  47. Sarmiento E., Vaz Pinto M., Villarreal R.H.: The minimum distance of parameterized codes on projective tori. Appl. Algebra Eng. Commun. Comput. 22(4), 249–264 (2011).

    MathSciNet  MATH  Google Scholar 

  48. Seidenberg A.: Constructions in algebra. Trans. Am. Math. Soc. 197, 273–313 (1974).

    MathSciNet  MATH  Google Scholar 

  49. Soprunov I.: Lattice polytopes in coding theory. J. Algebra Comb. Discret. Struct. Appl. 2(2), 85–94 (2015).

    MathSciNet  MATH  Google Scholar 

  50. Sørensen A.: Projective Reed–Muller codes. IEEE Trans. Inf. Theory 37(6), 1567–1576 (1991).

    MathSciNet  MATH  Google Scholar 

  51. Stanley R.: Hilbert functions of graded algebras. Adv. Math. 28, 57–83 (1978).

    MathSciNet  MATH  Google Scholar 

  52. Stanley R.: Enumerative Combinatorics, vol. 1, 2nd edn. Cambridge Studies in Advanced Mathematics, vol. 49. Cambridge University Press, Cambridge (2012).

  53. Stichtenoth H.: Algebraic Function Fields and Codes, 2nd edn. Graduate Texts in Mathematics, vol. 254. Springer, Berlin (2009).

  54. Sturmfels B.: Gröbner Bases and Convex Polytopes, University Lecture Series, vol. 8. American Mathematical Society, Rhode Island (1996).

  55. Tsfasman M., Vladut S., Nogin D.: Algebraic Geometric Codes: Basic Notions. Mathematical Surveys and Monographs, vol. 139. American Mathematical Society, Providence, RI (2007).

  56. Villarreal R.H.: Monomial Algebras, 2nd edn. Monographs and Research Notes in Mathematics. Chapman and Hall/CRC, Boca Raton, FL (2015).

  57. Wei V.K.: Generalized Hamming weights for linear codes. IEEE Trans. Inf. Theory 37(5), 1412–1418 (1991).

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors thank Nupur Patanker for pointing out an error in the previous statements of Corollary 4.6 and Proposition 5.1. We thank the referees for a careful reading of the paper and for the improvements suggested. We also thank Hiram López for helpful comments about the computation of radicals over finite fields. Computations with Magma [7] and Macaulay2 [22] were important to give examples and to have a better understanding of evaluation codes.

Author information

Authors and Affiliations

  1. Departamento de Matemáticas, Centro de Investigación y de Estudios Avanzados del IPN, Apartado Postal 14–740, 07000, Mexico City, Mexico

    Delio Jaramillo & Rafael H. Villarreal

  2. Departamento de Matemática, Instituto Superior Técnico, Universidade de Lisboa, Avenida Rovisco Pais, 1, 1049-001, Lisbon, Portugal

    Maria Vaz Pinto

Authors
  1. Delio Jaramillo
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Maria Vaz Pinto
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Rafael H. Villarreal
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Rafael H. Villarreal.

Additional information

Communicated by G. Korchmaros.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Delio Jaramillo was supported by a scholarship from CONACYT, Mexico. Maria Vaz Pinto was partially supported by the Center for Mathematical Analysis, Geometry and Dynamical Systems of Instituto Superior Técnico, Universidade de Lisboa. Rafael H. Villarreal was supported by SNI, Mexico.

Appendix A: Procedures for Magma and Macaulay2

Appendix A: Procedures for Magma and Macaulay2

In this section we give procedures, over finite fields, for Magma [7] and Macaulay2 [22] that are used to compute generalized Hamming weights and degree-footprint lower bounds of evaluation codes. In Procedure A.4 we use a pure lexicographical order. In all other procedures we use the graded reverse lexicographical order [56, p. 343], which is the default order.

Procedure A.1

Computing the generalized Hamming weights and footprints of an evaluation code \({\mathcal {L}}_X\) on X using Theorem 3.4, Proposition 3.5 and its constructive proof, and Theorem 3.9. The input for this procedure is a generating set for \({\mathcal {L}}\) and the vanishing ideal I of X. This procedure corresponds to Example 6.1. For the case \({\mathcal {L}}=S_d\), see Procedure A.2 below.

figure a

Procedure A.2

Given an evaluation code \({\mathcal {L}}_X\) on X and a monomial order \(\prec \) on S. Using Proposition 3.5 and its constructive proof, this procedure computes a linear subspace \(\widetilde{{\mathcal {L}}}\) of S such that \(\widetilde{{\mathcal {L}}}_X\) is a standard evaluation code on X and \(\widetilde{{\mathcal {L}}}_X={\mathcal {L}}_X\). Then, using Theorem 3.4, it computes the r-generalized Hamming weight of a projective Reed–Muller-type code on X of degree d. This procedure corresponds to Example 6.2. In this procedure \({\mathcal {L}}\) is the linear subspace \(S_d\) of S of homogeneous polynomials of degree d together with the zero vector. To compute \(\delta _r({\mathcal {L}}_X)\) for an evaluation code \({\mathcal {L}}_X\) replace basis(d,S) by the matrix of a K-basis of the linear space \({\mathcal {L}}\) (see Procedure A.1).

figure b

Procedure A.3

Computing the generalized Hamming weights of a Reed–Muller-type code \(C_X(d)\) using Corollary 3.6. This procedure corresponds to Example 6.3. Other examples can be computed changing the finite field and the set of points of X.

figure c

Procedure A.4

Computing the quotient and the remainder in the multivariate division algorithm [11, Theorem 3, p. 63]. This procedure corresponds to Example 6.4.

figure d

Procedure A.5

Computing the minimum distance of a Reed–Muller-type code \(C_X(d)\) of degree d using Corollary 3.7. This procedure corresponds to Example 6.5.

figure e

Procedure A.6

Computing the footprint of a Reed–Muller-type code \(C_X(d)\). This procedure corresponds to Example 6.6. To compute other examples just change the finite field and the vanishing ideal I of X.

figure f

Procedure A.7

Computing the length, dimension, and minimum distance of a Reed–Muller-type code \(C_X(d)\) over an affine variety \(X=V_{{\mathbb {A}}^s}(G)\) defined by a given set of multivariate polynomials G. This procedure uses Lemma 2.5, Corollary 3.7, and Hilbert’s Nullstellensatz of Theorem 3.12. As an illustration we use the Hermitian curve g of Example A.7.

figure g

Procedure A.8

Computing the number of points of an affine variety over a finite field using Lemma 2.8. This procedure corresponds to the elliptic curve of Example 6.8. To compute other examples just change the finite field and the set of polynomials F that define the affine variety.

figure h

Procedure A.9

Computing the radical of an ideal over a finite field using Magma [7]. This procedure corresponds to Example 6.9.

figure i

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Jaramillo, D., Vaz Pinto, M. & Villarreal, R.H. Evaluation codes and their basic parameters. Des. Codes Cryptogr. 89, 269–300 (2021). https://doi.org/10.1007/s10623-020-00818-8

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10623-020-00818-8

Keywords

Mathematics Subject Classification

Search

Navigation