Tobin Tax, Carry Trade, and the Exchange Rate Dynamics

Abstract

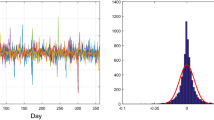
We propose a heterogeneous agent model including the fundamentalists, chartists, and carry traders, to describe the dynamics of exchange rates, and examine the effects of Tobin Tax on the equilibrium exchange rates. A theoretical analysis shows that an increase of Tobin tax always reduces the trading volume, and its impact on the equilibrium exchange rate depends on the agents’ expectations of exchange rate movements and their weights in the markets. Our simulation exercise shows that an increase in Tobin tax may lead to an increase in volatility and price distortion and a decrease in the kurtosis and the trading volume. Our results suggest that the Tobin tax could be an emergency measure, but not a long-term policy tool to suppress speculation.

Appendices

Appendix 1: Derivation of the Optimal Foreign Currency Holding

The purpose of the foreign exchange market trader is to optimize the portfolio of assets on domestic and foreign assets to maximize the expected utility. Suppose the trader’s expected utility function is:

$$ L = U_{i,\;t} \left( {W_{i,\,t + 1} } \right) = E_{i,\;t} \left( {W_{i,\;t + 1} } \right) - \frac{1}{2}\mu_{i} \,\upsilon_{i,\;t} \left( {W_{i,\;t + 1} } \right) $$

(17)

We substitute $W_{i,\;t + 1}$ into the above formula and get

$$ L = \left( {1 + r_{d,\;t} } \right)\left( {W_{t}^{i} - s_{t} \,f_{t}^{i} } \right) + E_{t} \left( {s_{t + 1} } \right)\left( {1 + r_{f,\;t} } \right)f_{t}^{i} $$

$$ - \tau \left| {f_{t}^{i} - f_{t - 1}^{i} } \right|s_{t} - \frac{1}{2}\lambda \left( {1 + r_{f,t} } \right)^{2} \left( {f_{t}^{i} } \right)^{2} v_{i,t} \left( {s_{t + 1} } \right) $$

(18)

If $\Delta f_{t}^{i} < 0$, the trader is a seller. From the first order condition $\frac{\partial L}{{\partial f_{t}^{i} }} = 0$, we can get

$$ f_{t}^{i} \left( {s_{t} } \right) = \frac{{E_{t}^{i} \left( {s_{t + 1} } \right)\left( {1 + r_{f,\;t} } \right) - s_{t} \left( {1 + r_{d,\;t} - \tau } \right)}}{{\lambda v_{t}^{i} \left( {s_{t + 1} } \right)\left( {1 + r_{f,\;t} } \right)^{2} }} $$

(19)

Since $f_{t}^{i} < f_{t - 1}^{i}$, we have

$$ s_{t} > \frac{{E_{t}^{i} \left( {s_{t + 1} } \right)\left( {1 + r_{f,\;t} } \right) - \lambda v_{t}^{i} \left( {s_{t + 1}^{2} } \right)\left( {1 + r_{f,\;t} } \right)^{2} f_{t - 1}^{i} }}{{1 + r_{d,\;t} - \tau }} = s_{2} $$

(20)

Similarly, if $\Delta f_{t}^{i} > 0$, the trader is a buyer. The first order condition implies that

$$ f_{t}^{i} \left( {s_{t} } \right) = \frac{{E_{t}^{i} \left( {s_{t + 1} } \right)\left( {1 + r_{f,\;t} } \right) - s_{t} \left( {1 + r_{d,\;t} + \tau } \right)}}{{\lambda \,v_{t}^{i} \left( {s_{t + 1} } \right)\left( {1 + r_{f,\;t} } \right)^{2} }} $$

(21)

Since $f_{t}^{i} > f_{t - 1}^{i}$, we have

$$ s_{t} < \frac{{E_{t}^{i} \left( {s_{t + 1} } \right)\left( {1 + r_{f,\;t} } \right) - \lambda \,v_{t}^{i} \left( {s_{t + 1}^{2} } \right)\left( {1 + r_{f,\;t} } \right)^{2} f_{t - 1}^{i} }}{{1 + r_{d,\;t} + \tau }} = s_{1} $$

(22)

Finally if $\Delta \,f_{t}^{i} = 0$, the investor decides not to trade. Because $L$ is constant, $\frac{\partial L}{{\partial f_{t}^{i} }} = 0$ always holds when $s_{1} \le s_{t} \le s_{2}$.

Proof of Proposition 1

The market-clearing condition showed as Eq. (12) implies that

$$ \mathop \sum \limits_{{i \in \left\{ {f,c,r} \right\}}} w_{t}^{i} \frac{{df_{t}^{i} }}{d\tau } = 0 $$

Substitute Eq. (6) into the above equation, then we can have

$$ \frac{ds}{{d\tau }} = \frac{{ - s_{t} \mathop \sum \nolimits_{{i\, \in \left\{ {f,\;c,\;r} \right\}}} w_{t}^{i} {\text{sgn}} \left( {\Delta \,f_{t}^{i} } \right)/G_{i} }}{{\mathop \sum \nolimits_{{i \in \left\{ {f,\;c,\;r} \right\}}} w_{t}^{i} \left( {1 + r_{d,\;t} + \tau {\text{sgn}} \left( {\Delta \,f_{t}^{i} } \right)} \right)/G_{i} }} $$

along with $\frac{{\partial f_{t}^{i} }}{\partial \tau } = - \frac{{s_{t} {\text{sgn}} \left( {\Delta \,f_{t}^{i} } \right)}}{{G_{i} }}$ and $\frac{{\partial f_{t}^{i} }}{\partial s} = - \frac{{1 + r_{d,t} + \tau {\text{sgn}} \left( {\Delta f_{t}^{i} } \right)}}{{G_{i} }}$, where $G_{i} = \lambda v_{t}^{i} \left( {1 + r_{f,t} } \right)^{2}$.

Thus we prove Proposition 1.

Proof of Proposition 2

The equilibrium response of $f_{t}^{i}$ to a change in the tax $\tau$ is given by

$$ \frac{{df^{i} }}{d\tau } = \frac{{\partial f^{i} }}{\partial \tau } + \frac{{\partial f^{i} }}{\partial s}\frac{ds}{{d\tau }} $$

(23)

By substituting $\frac{{\partial f^{i} }}{\partial \tau }$, $\frac{{\partial f^{i} }}{\partial s}$ and $\frac{ds}{{d\tau }}$ into Eq. (23), we have

$$ \frac{{df_{t}^{i} }}{d\tau } = \frac{{\partial f_{t}^{i} }}{\partial \tau }\left\{ {1 - \left[ {\left( {1 + r_{d,t} } \right){\text{sgn}} \left( {\Delta f_{t}^{i} } \right) + \tau } \right]\frac{{\mathop \sum \nolimits_{{i \in \left\{ {f,c,r} \right\}}} w_{t}^{i} {\text{sgn}} \left( {\Delta f_{t}^{i} } \right)/G_{i} }}{{\mathop \sum \nolimits_{{i \in \left\{ {f,c,r} \right\}}} w_{t}^{i} \left( {1 + r_{d,t} + \tau {\text{sgn}} \left( {\Delta f_{t}^{i} } \right)} \right)/G_{i} }}} \right\} $$

Let $e_{i}$ represent the expression in the curly braces, and it can be rewritten as:

$$ e_{i} = 1 - \left[ {\left( {1 + r_{d,t} } \right){\text{sgn}} \left( {\Delta f_{t}^{i} } \right) + \tau } \right]\frac{{1 - L^{*} }}{{\left( {1 + r_{d,t} + \tau } \right) + \left( {1 + r_{d,t} - \tau } \right)L}} $$

where $L^{*} = \frac{{\sum_{i \in S} w_{t}^{i} /G_{i} }}{{\sum_{i \in B} w_{t}^{i} /G_{i} }} \in \left( {0,\;\infty } \right)$ is the ratio of sellers’ risk aversion to buyers’ risk aversion. It is straightforward to show that $e_{i} > 0$ for both buyers and sellers.

Therefore, the sign of $\frac{{df_{t}^{i} }}{d\tau }$ is determined by that of $\frac{{\partial f_{t}^{i} }}{\partial \tau }$. According to Eq. (6), we can gain that $\frac{{\partial f_{t}^{i} }}{\partial \tau } = \frac{{ - s_{t} {\text{sgn}} \left( {\Delta f_{t}^{i} } \right)}}{{G^{i} }}$. When $\Delta f_{t}^{i} > 0$, which implies that the trader is a buyer and $i \in B$, we can get $\frac{{df_{t}^{i} }}{d\tau } < 0$. Otherwise, when $\Delta f_{t}^{i} < 0$ which implies that the trader is a seller and $i \in S$, we can get $\frac{{df_{t}^{i} }}{d\tau } > 0$. Thus we can prove Proposition 2a).

Let $vol_{t}^{i} = \left| {f_{t}^{i} - f_{t - 1}^{i} } \right|$, we have $\frac{{dvol_{t} }}{d\tau } = \sum w_{t}^{i} \frac{{dvol_{t}^{i} }}{d\tau } = \mathop \sum \limits_{i \in B} w_{t}^{i} \frac{{df_{t}^{i} }}{d\tau } - \mathop \sum \limits_{i \in S} w_{t}^{i} \frac{{df_{t}^{i} }}{d\tau }$. So Proposition 2b) is proved as ${ }\mathop \sum \limits_{i \in B} w_{t}^{i} \frac{{df_{t}^{i} }}{d\tau } < 0$ and $\mathop \sum \limits_{i \in S} w_{t}^{i} \frac{{df_{t}^{i} }}{d\tau } > 0$.