A Finite Difference Scheme for Pairs Trading with Transaction Costs

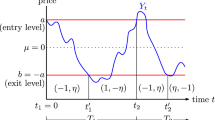
Abstract

We consider a pairs trading stochastic control problem with transaction costs and constraints on the gross market exposure, and propose a new monotone Finite Difference scheme approximating the viscosity solution of the Hamilton–Jacobi–Bellman equation characterizing the optimal trading strategies. Given a fixed time horizon and a portfolio of two cointegrated assets, the agent trades the spread between the two assets and the trading strategy is defined as the possibly negative portfolio weight maximizing the expected exponential utility derived from terminal wealth. Furthermore, trades incur transaction costs comprised of explicit transactions fees and commissions and the implicit cost due to slippage. These costs are modeled as a linear or square root function of the trading rate and respectively added or subtracted from the observable asset price at the time when a buy or a sell order enters the market. Our main contribution is the derivation of a robust approximation for the nonlinear transaction cost term in the Hamilton–Jacobi–Bellman equation. Finally, we combine our monotone Finite Difference scheme with a Monte Carlo sampling method to analyze the effects of transaction fees and slippage on the trading policies’ performance.

Appendix: The Finite Difference Scheme for the model with price impact

In this appendix, we derive a monotone approximation for the viscosity solution of (68), (69). We will use the standard notations

$$\begin{aligned} D_z^+G_{i,j}^n&= \frac{G_{i+1,j}^n-G_{i,j}^n}{\varDelta z},&D_z^-G_{i,j}^n&= \frac{G_{i,j}^n-G_{i-1,j}^n}{\varDelta z},\\ D_{\pi }^+G_{i,j}^n&= \frac{G_{i,j+1}^n-G_{i,j}^n}{\varDelta \pi },&D_{\pi }^-G_{i,j}^n&= \frac{G_{i,j}^n-G_{i,j-1}^n}{\varDelta \pi },\\ D_{z}^+D_z^-G_{i,j}^n&= \frac{G_{i+1,j}^n-2G_{i,j}^n+G_{i-1,j}^n}{(\varDelta z)^2}. \end{aligned}$$

As in Sect. 5, we split (68) into two operators:

$$\begin{aligned} g_{\tau } -a(z,\pi )g - b(z,\pi ) g_z - \frac{1}{2}\sigma _{\beta }^2 g_{zz} =0, \end{aligned}$$

(70)

and

$$\begin{aligned} g_{\tau }+\sup \limits _{v\in A}\big \{ -\gamma \lambda v^2 g +\kappa \gamma \pi vg - \kappa vg_z - vg_{\pi } \big \}=0. \end{aligned}$$

(71)

Next, we proceed as in Sect. 5 to derive a scheme for (71) in the interior of the domain by writing

$$\begin{aligned}&\frac{G_{i,j}^{n+1}-G_{i,j}^n}{\varDelta t}\nonumber \\&\quad =\min (\inf _{b_{i,j}+\kappa v>=0, v>=0} \{(a_{i,j}-\kappa \gamma \pi _j v+\lambda \gamma v^2)G_{i,j}^n+(b_{i,j}+\kappa v)D_z^+G_{i,j}^n+\frac{1}{2}\sigma _{\beta }^2D_{z}^+D_z^-G_{i,j}^n+vD_{\pi }^+G_{i,j}^n\},\nonumber \\&\quad \inf _{b_{i,j}+\kappa v>=0, v<0} \{(a_{i,j}-\kappa \gamma \pi _j v+\lambda \gamma v^2)G_{i,j}^n+(b_{i,j}+\kappa v)D_z^+G_{i,j}^n+\frac{1}{2}\sigma _{\beta }^2D_z^+D_z^-G_{i,j}^n+vD_{\pi }^-G_{i,j}^n\},\nonumber \\&\quad \inf _{b_{i,j}+\kappa v<0, v>=0} \{(a_{i,j}-\kappa \gamma \pi _j v+\lambda \gamma v^2)G_{i,j}^n+(b_{i,j}+\kappa v)D_z^-G_{i,j}^n+\frac{1}{2}\sigma _{\beta }^2D_z^+D_z^-G_{i,j}^n+vD_{\pi }^+G_{i,j}^n\},\nonumber \\&\quad \inf _{b_{i,j}+\kappa v<0, v<0} \{(a_{i,j}-\kappa \gamma \pi _j v+\lambda \gamma v^2)G_{i,j}^n+(b_{i,j}+\kappa v)D_z^-G_{i,j}^n+\frac{1}{2}\sigma _{\beta }^2D_z^+D_z^-G_{i,j}^n+vD_{\pi }^-G_{i,j}^n\}). \end{aligned}$$

(72)

We can solve the above optimization problem in closed form.

• For the first infimum, the critical point is

  $$\begin{aligned} v^{++} = \frac{\kappa \gamma \pi _j G_{i,j}^n-\kappa D_z^+G_{i,j}^n-D_{\pi }^+G_{i,j}^n}{2\lambda \gamma G_{i,j}^n}. \end{aligned}$$

  The infimum is attained at $v_1^*$ defined by

  • if $b_{i,j}+\kappa v \ge 0, v \ge 0$, $v_1^*=v^{++}$

  • if $b_{i,j}+\kappa v \ge 0, v < 0$, $v_1^*=0$

  • if $b_{i,j}+\kappa v < 0, v \ge 0$, $v_1^*=-\frac{b_{i,j}}{\kappa }$.

• For the second infimum, the critical point is

  $$\begin{aligned} v^{+-} = \frac{\kappa \gamma \pi _j G_{i,j}^n-\kappa D_z^+G_{i,j}^n-D_{\pi }^-G_{i,j}^n}{2\lambda \gamma G_{i,j}^n}. \end{aligned}$$

  The solution of the minimization problem is given by

  • if $b_{i,j}+\kappa v \ge 0, v < 0$, $v_2^*=v^{+-}$

  • if $b_{i,j}+\kappa v \ge 0, v \ge 0$, $v_2^*=0$

  • if $b_{i,j}+\kappa v< 0, v < 0$, $v_2^*=-\frac{b_{i,j}}{\kappa }$.

• For the third infimum, the critical point is

  $$v^{-+} = \frac{\kappa \gamma \pi _j G_{i,j}^n-\kappa D_z^-G_{i,j}^n-D_{\pi }^+G_{i,j}^n}{2\lambda \gamma G_{i,j}^n},$$

  and the solution of the optimization problem is

  • if $b_{i,j}+\kappa v < 0, v \ge 0$, $v_3^*=v^{-+}$

  • if $b_{i,j}+\kappa v< 0, v < 0$, $v_3^*=0$

  • if $b_{i,j}+\kappa v \ge 0, v \ge 0$, $v_3^*=-\frac{b_{i,j}}{\kappa }$

• Finally, for the forth infimum, the critical point is

  $$\begin{aligned} v^{--} = \frac{\kappa \gamma \pi _j G_{i,j}^n-\kappa D_z^-G_{i,j}^n-D_{\pi }^-G_{i,j}^n}{2\lambda \gamma G_{i,j}^n}, \end{aligned}$$

  and the solution of the minimization problem is given by

  • if $b_{i,j}+\kappa v< 0, v < 0$, $v_4^*=v^{--}$

  • if $b_{i,j}+\kappa v < 0, v \ge 0$, $v_4^*=0$

  • if $b_{i,j}+\kappa v \ge 0, v < 0$, $v_4^*=-\frac{b_{i,j}}{\kappa }$

For instance, when the minimum in (72) is realized for the first infimum and achieved at the critical point inside the interval, the resulting Finite Difference scheme is given by

$$\begin{aligned} G_{i,j}^{n+1}= & {} =1+\varDelta t[\frac{1}{2}\sigma _{\beta }^2 D^+_z D^-_z G_{i,j}^n+ (b_{i,j}+\frac{\kappa ^2\pi _j}{2\lambda })D^+_z G_{i,j}^n\nonumber \\&-\frac{\kappa ^2}{4\lambda \gamma G_{i,j}^n}(D^+_z G_{i,j}^n)^2 +\frac{\kappa }{2\lambda }\pi _j D^+_{\pi } G_{i,j}^n+(a_{i,j}\nonumber \\&-\frac{\kappa ^2\gamma }{4\lambda }\pi _j^ 2) G_{i,j}^n-\frac{\kappa }{2\lambda \gamma }\frac{D^+_z G_{i,j}^n D^+_{\pi } G_{i,j}^n}{G_{i,j}^n} -\frac{1}{4\lambda \gamma }\frac{(D^+_{\pi } G_{i,j}^n)^2}{G_{i,j}^n}]. \end{aligned}$$

(73)

When, the minimum is realized for one of the other infima and achieved inside the associated interval, on must replace in the above scheme the forward Finite Differences $D^+_z G_{i,j}^n$ and $D^+_{\pi } G_{i,j}^n$ by the appropriate pair of Finite Differences, which are respectively $D^+_z G_{i,j}^n, D^-_{\pi } G_{i,j}^n$ for the second infimum, $D^-_z G_{i,j}^n, D^+_{\pi } G_{i,j}^n$ for the third, and $D^-_z G_{i,j}^n, D^-_{\pi } G_{i,j}^n$ for the forth.

When the minimum holds for $v^*=0$, (73) degenerates to

$$\begin{aligned} G_{i,j}^{n+1}= & {} =G_{i,j}^n. \end{aligned}$$

(74)

Finally, we leave to the reader the case when $v^*=-\frac{b_{i,j}}{\kappa }$.

Note that, as in Sect. 5, we also need to modify the scheme to accommodate the state constraint at the edges $\pi _{{\min }}$ and $\pi _{{\max }}$. We leave this straightforward modification to the reader as well.

Since (73) is explicit, the scheme is only convergent under the following CFL condition derived from verifying the monotonicity condition.

$$\begin{aligned}&1+\varDelta t (a_{i,j}-\frac{\kappa ^2\pi _j^2\gamma }{4\lambda }-\frac{\sigma _{\beta }^2}{\varDelta z^2}-\frac{(b_{i,j}+\frac{\kappa ^2\pi _j}{2\lambda })}{\varDelta z} -\frac{\kappa \pi _j}{2\lambda \varDelta \pi }-\frac{\kappa }{2\lambda \gamma \varDelta \pi \varDelta z}\frac{(G_{i,j}^n)^2-G_{i+1,j}^n G_{i,j+1}^n}{(G_{i,j}^n)^2}\nonumber \\&\quad +\frac{\kappa ^2}{4\lambda \gamma \varDelta z^2}\frac{(G_{i+1,j}^n)^2-(G_{i,j}^n)^2}{(G_{i,j}^n)^2}+\frac{1}{4\lambda \gamma \varDelta \pi ^2}\frac{(G_{i,j+1}^n)^2-(G_{i,j}^n)^2}{(G_{i,j}^n)^2} ) \ge 0,\nonumber \\&\quad {\text{ for all}} \; i,j. \end{aligned}$$

(75)

Note that when other combinations of the forward and backward Finite Differences arise at the minimum, we need to modify (75) accordingly. Since these changes are straightforward, we leave them to the reader.