Evaluation of flexibility in adaptation projects for climate change

Abstract

Climate change adaptation inherently entails investment decision-making under the high levels of uncertainty. To address this issue, a single fixed large investment can be divided into two or more sequential investments. This reduces the initial investment cost and adds flexibility about the size and timing of subsequent investment decisions. This flexibility enables future investment decisions to be made when further information about the magnitude of climate change becomes available. This paper presents a real option analysis framework to evaluate adaptations including flexibility to reduce both the risk and uncertainty of climate change, against increasing coastal flooding due to sea-level rise as an example. The paper considers (i) how to design the sequence of adaptation options under growing risk of sea-level rise, and (ii) how to make the efficient use of flexibility included in adaptations for addressing uncertainty. A set of flexibilities (i.e. wait or future growth) are incorporated into single-stage investments (i.e. raising coastal defence from 2.5 mAOD to 3.5mAOD or 4.0 mAOD) in stages so that multiple-stage adaptations with different heights are created. The proposed method compares these sequentially growing adaptations in economic terms, including optimisation, providing additional information on the efficiency of flexible adaptation strategies given the uncertainty of climate change. The results from the evaluation enable decision-makers to identify long-lasting robust adaptation against the uncertainty of climate change.

Introduction

Climate change adaptation inherently entails investment decision-making under the high levels of uncertainty (Dawson et al. 2018). To tackle uncertainty, flexible adaptation options that can be deferred or extended in the future are widely used in climate change adaptation, in particular, flood risk management. Such adaptations have some advantages in addressing both risk and uncertainty. Firstly, they provide a high degree of freedom in making investment decisions under uncertain future states. As long as flexibility is alive in adaptation options, there is a choice either to invest or to wait for option holders at a given time (Bellman 1952). Secondly, option holders can part investment costs by stages in designing adaptation options so that an initial action can be facilitated at a relatively low cost. In addition, if the remaining option is proved to be unnecessary in the future, the option holders can drop it and save the due cost in the future. Lastly, the flexible adaptation options enable us to adjust actions or plans in response to the unexpected future states (Linquiti and Vonortas 2012). After a large amount of budgets are spent on infrastructure, it will be difficult to adjust its capacity and size in response to unexpected conditions. However, if the costly infrastructure is engineered to flexibly grow in many stages, it enables us to take adjustable actions in response to the future. These types of adaptation approaches are referred to as diverse terms such as ‘adaptation pathways approach (Ranger et al. 2013; Nicholls et al. 2014)’, ‘dynamic adaptation (Hallegatte 2009; Haasnoot et al. 2013)’ or ‘real options approach (Neufville 2003; Woodward et al. 2014)’.

Real option analysis (ROA) is an assessment tool to value flexibility included in investment options (Dixit and Pindyck 1994). The theoretical background is based upon an assumption that flexibility given to decision-makers is the right, but not the obligation (Dixit and Pindyck 1994; Park 2002; Neufville 2003). ROA has been widely used to address the uncertainty of future market states (e.g. the price of product or stock) in finance. This concept is now vigorously used in climate change realms where uncertainty is deep and everywhere (Dobes 2010; Woodward et al. 2014; Hino and Hall 2017; Trigeorgis and Tsekrekos 2018). Although ROA and dynamic adaptation (DY) have much in common in the use of flexibility under uncertainty, their applications differ depending on the condition of adaptation options. ROA focuses on adaptation options that are flexible and irreversible, while DY focuses on a group of adaptations that are sequentially and flexibly connected to deal with uncertainty. Thus, DY includes soft adaptations such as land use planning and flood alarm in its application whereas ROA addresses hard adaptation options such as defence heightening that incur a large investment cost.

As coastal adaptations require a large investment cost, flood risk analysts or decision-makers are left in the face of challenging tough investment decisions. For this reason, flexibility is currently introduced to resolve investment issues under uncertainty. Flexibility takes place in coastal adaptations with diverse forms: (1) wait, (2) future growth or (3) both. The inclusion of such flexibilities in coastal adaptations enables decision-makers to invest by stages. Thus, a way to exercise the flexibility in the climate change adaptation has effect on reduction in the risk of climate change and, subsequently, the value of adaptation options under various climate change scenarios (Linquiti and Vonortas 2012; Woodward et al. 2014). The previous studies on real options suggest that an adjustable adaptation option in the future is a more robust strategy under the uncertainty of climate change, if the adaptation option is not limited by further development or land use (Dobes 2010; Linquiti and Vonortas 2012; Woodward et al. 2014; Hino and Hall 2017; Haasnoot et al. 2021).

In terms of the robustness against uncertainty, real option analysis is frequently compared to robust decision-making (Dittrich et al. 2016). However, they are inherently different in methodology and application. Robust decision-making is intended to find an optimal trade-off between decrease in the expected performance and increase in the performance for the worst case among possible adaptation options, whereas real option analysis focuses on the evaluation of flexibility that leads to increase in the robustness of the adaptation options. However, the recent studies on robust decision-making provide methods to evaluate flexible adaptation strategies at the minimum loss of their expected performance (Mcinerney et al. 2012; Wreford and Topp 2020).

Recently, real option analysis employs diverse ways to assess flexible adaptation options, or strategies, against uncertainty in climate change adaptation. Some studies have quantified the efficiency of flexible adaptations in flood risk management under the combination of socio-economy changes and climatic changes (Hino and Hall 2017; Manocha and Babovic 2017). One study incorporates qualitative drivers (e.g. adaptive capacity, information accessibility, decision-making process) in real option evaluation framework so as to stimulate long-term actions against climate change (Lawrence et al. 2019). One study focuses on structuring the process of designing climate adaptation policies that can flexibly respond to the unfold futures (Buurman and Babovic 2016). Other studies evaluate a portfolio of coastal adaptation paths which grow in response to the possible future states by NPV (net present value) method (Linquiti and Vonortas 2012; Woodward et al. 2014). The investment timing for implementing adaptation options has been investigated to maximise the economy efficiency of coastal adaptations under various sea-level rise scenarios (Kim et al. 2018). Most studies suggest that, rather than non-flexible adaptations, incremental and adjustable approaches to the upgrade of infrastructure are more economically efficient for the long-lasting infrastructure (Manocha and Babovic 2017; Smet 2017).

As in the previous studies, when comparing to a traditional cost-benefit analysis, real option analysis requires a very complicated process to assess adaptation options under various future scenarios (e.g. climate change scenarios and socio-economic scenarios) (Kind et al. 2018). In addition, the possible futures are manifold so that the adaptation options need to be evaluated under various future states which may require the statistical understanding of the future states (Kwakkel 2020). Furthermore, the incorporation of flexibility entails additional issues on when and how to use the flexibility against uncertainty.

In such contexts, this paper is aimed to provide the framework of assessing multiple-stage adaptations which incorporate wait or future growth, as flexibility, in themselves. This research applies the real option analysis framework in coastal defences (i.e. heightening) which are intended to protect the vulnerable coastal area from coastal flooding the risk of which will be increased by future sea-level rise. To understand the efficiency of flexibility, we consider a simple case where a coastal defence will be raised against coastal flooding and sea-level rise. When designing the coastal defence, the height of coastal defence and the number of stages by which the coastal defence can be upgraded are set to be flexibility so as to create a portfolio of multiple-stage adaptations. Thus, the decision is to select the most efficient one among the considered multiple-stage adaptations against the uncertainty of sea-level rise. Lymington is chosen as a vulnerable area to coastal flooding and sea-level rise (Ruocco et al. 2011; Wadey et al. 2012), and the coastal flood adaptation has been planned to improve the capacity of the current coastal defence against the risk of coastal flooding (Fig. 1).

Fig. 1.

The case study site of Lymington with a 1-in-200 year flood risk zone indicated by Environment Agent (UK) from OS maps

This paper uses the past UKCP 09 data (UK climate projection 09) because this research had been conducted when the UKCP09 was available (Lowe et al. 2009). Now, these dataset have been upgraded as UKCP 18. The present and future flood risks and the corresponding adaptation measures (a single-stage adaptation) have been well-understood by the previous studies (Wadey et al. 2013). Thus, the results from the analysis enable us to make the efficient use of flexibility of wait or growth under the uncertainty and risk of sea-level rise. This analysis allows decision-makers to choose a flexible and robust adaptation in terms of economy efficiency under given conditions (e.g. types of coastal adaptations, adaptation costs, the uncertainty of SLR scenarios, the risk of coastal flooding).

This paper consists of four sections. The next section explains the overall framework to value multiple-stage adaptation options. In the following section, we provide results from the evaluations of all the possible adaptations with changes to the costs of flexibility under each SLR scenario. For more information, the detailed analysis on the option evaluation has been included in supplementary documents (1, 2 and 3). The quantitative comparisons of the multiple- and single-stage adaptations are undertaken to provide an economically efficient strategy under the uncertainty of SLR scenarios. Finally, this paper makes a conclusion with discussions on the results and its implications for climate change adaptation.

Method

Evaluation of deferable adaptation options and investment timing

If an adaptation option is deferable, two values exist (Dixit and Pindyck 1994). A value for wait is called a continuation value, while a value for investment is a termination value (Bellman 1952; Dixit and Pindyck 1994; Yang and Blyth 2007; Kim et al. 2018). A higher one of both values in any given year t is an option value at that time defined by equation (1).

$${F}_t=\max \left[{F}_{con,t},{F}_{ex,t}\right]$$

(1)

Here, F_con,t is a continuation value in year t, F_ex,t is a termination value in year t and F_t is an option value in the year t, which is the higher one of the two values. If a continuation value (F_con,t) is greater than a termination value (F_ex,t), it suggests that waiting is preferable to investing and vice versa (Bellman 1952). The termination value in year t is an option value when the investment is made at year t. Thus, it can be defined by Equation (2).

$${F}_{ex,t}={\sum\nolimits}_{i=t+1}^{L+t}\frac{EAB_i}{{\left(1+r\right)}^i}-\frac{I}{{\left(1+r\right)}^t}$$

(2)

Here, EAB_i is the expected annual benefit of a project with the investment cost of I at year i, r is discount rate and L is the project life (= 100 years). A continuation value (F_con,t) at year t is the higher one of continuation and termination values at year t+1 discounted by a discounting factor 1/(1+r). Thus, a continuation value is defined by equation (3).

$${F}_{con,t}=\frac{1}{\left(1+r\right)}\times \mathit{\operatorname{Max}}\left[{F}_{ex,t+1},{F}_{con,t+1}\right]$$

(3)

These two equations (i.e. F_ex,t, F_con,t) can determine whether to defer or to invest at any year. Thus, the investment timing for an adaptation option is considered by finding an optimal investment time. As in the previous study (Kim et al. 2018), the investment timing is associated with the threshold value of sea-level rise. The calculation of a continuation and a termination value starts from the end year of sea-level rise (SLR) projection by a backward induction method (Kim et al. 2018). This evaluation process can be extended to a multiple-stage adaptation by estimating the option value and optimal investment time for each stage of a multiple-stage adaptation option as shown in Fig. 2. For option evaluation, this analysis adopts the national discount rates (r) from the Green Book (HM Treasury 2003) (i.e. 3.5% for the first 30 years, 3.0% for the next 45 years and 2.5% afterwards). For simplicity, socio-economic change is excluded in the option evaluation because it entirely multiplies the option values of coastal adaptations, leading to no changes in the relative rankings of the coastal adaptations in terms of option value.

Fig. 2.

The framework of option evaluations for multiple-stage adaptations

Reduction in flood damage by any adaptation measure is benefit that decision-makers expect to gain from the investment. This study evaluates expected annual benefit (EAB) at a given year—the annual performance of an adaptation measure—which depends on sea-level rise and ways to upgrade coastal defence in an area of interest. To estimate changes in EAB for any adaptation measure across sea-level rise, a pair of impact curves for the initial and upgraded defence conditions have been chosen. The changes in EAB across sea-level rise for any adaptation measure show the unique response of the coastal area to sea-level rise. The case study area considers six SLR scenarios in which we will evaluate temporal changes in EAB of each adaptation measure (refer to Supplement 1 for the estimation of EAB). The trajectories of sea-level rise by different scenarios for Lymington are drawn during the twenty-first century in Fig. 3.

Fig. 3.

Mean sea-level rise (relative to 1990) scenarios for Lymington: 2008 to 2100 (Lowe et al. 2009)—the H++ MSLR is derived from the global scale sea-level rise data (Nicholls et al. 2014) by scaling-techniques and the historical trend of sea-level rise (1.4 mm/year) is from the Southampton tidal gauge (Haigh et al. 2009). H1 SLR scenario is created in the middle between H++ and High SLR scenarios.

Description of terms of single- and multiple-stage adaptations

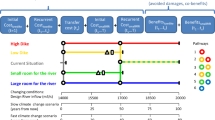
A single-stage investment has been transformed into multiple-stage sequential investments so that the possible sets of the adaptation pathways are conceptualised in Fig. 4. 0.5m or 1m increase is considered for coastal defence upgrade against the rising sea-level in Lymington. The current crest level of the coastal defence is around 2.5 meter Above Ordnance Datum which is, hereafter, termed mAOD. The coastal defence can be raised to 3.0, 3.5 or 4.0 mAOD in one or more stages. Totally, 7 pathways are made for an experimental setting (refer to Supplement 1). This paper denotes U_i → j to an adaptation measure of raising the crest of coastal defence from the initial height (i) to the upgraded height (j). This terminology also represents multiple-stage adaptations by putting terms together. For example, a two-stage defence upgrade from the current leve—it is termed (c) in the paper—through 3.0 mAOD to 3.5 mAOD is denoted by U_{c → 3.0m} × U_{3.0m → 3.5m}.

Fig. 4.

Examples of real options for the case of coastal defences: a option to wait; b option to grow; and c option to invest now. The coastal defences have been upgraded according to sea-level rise. The dash-lined coastal defence is the upgrade scheme whereas the solid-lined coastal defence means the upgraded defence.

This paper only considers the raising of coastal defence because the real option analysis is applicable when the option is irreversible. Thus, reversible adaptation options such as flood alarming and land use planning are not considered in this research. In practice, these types of adaptations can be jointly planned with coastal defence upgrade to enhance the adaptive capacity of the coastal defence, as a whole system, against coastal flooding.

Estimation of costs of multiple-stage adaptations

The additional costs should be paid for including flexibility in adaptation options. If not, adaptation options with flexibility would be always more preferable than non-flexible one. It is because the future uncertainty will be addressed with the high degree of freedoms induced by the flexibility at no cost. Thus, real option analysis assumes for the practical and theoretical reasons that the flexibility should be priced (Dixit and Pindyck 1994). The division of a single investment into two sequential investments increases the overall investment cost as shown in equation (4) and (5).

$${\displaystyle \begin{array}{c}{\boldsymbol{I}}_{\boldsymbol{o}}+{\boldsymbol{I}}_{\boldsymbol{f}}=\left({\boldsymbol{I}}_{\boldsymbol{o},\mathbf{1}}+{\boldsymbol{I}}_{\boldsymbol{o},\mathbf{2}}\right)+\left(\ \frac{\mathbf{1}}{\mathbf{2}}{\boldsymbol{I}}_{\boldsymbol{f}}+\frac{\mathbf{1}}{\mathbf{2}}{\boldsymbol{I}}_{\boldsymbol{f}}\right)\\ {}=\left({\boldsymbol{I}}_{\boldsymbol{o},\mathbf{1}}+\frac{\mathbf{1}}{\mathbf{2}}{\boldsymbol{I}}_{\boldsymbol{f}}\right)+\left({\boldsymbol{I}}_{\boldsymbol{o},\mathbf{2}}+\frac{\mathbf{1}}{\mathbf{2}}{\boldsymbol{I}}_{\boldsymbol{f}}\right)\end{array}}$$

(4)

$${\boldsymbol{I}}_{\mathbf{1}}={\boldsymbol{I}}_{\boldsymbol{o},\mathbf{1}}+\frac{\mathbf{1}}{\mathbf{2}}{\boldsymbol{I}}_{\boldsymbol{f}},{\boldsymbol{I}}_{\mathbf{2}}={\boldsymbol{I}}_{\boldsymbol{o},\mathbf{2}}+\frac{\mathbf{1}}{\mathbf{2}}{\boldsymbol{I}}_{\boldsymbol{f}}$$

(5)

Here, I_o is the cost of a single-stage adaptation; I_f is the cost of flexibility (termed, hereafter, flexibility premium); I_{o, 1} and I_{o, 2} are the net costs of the first- and the second-stage adaptations—both of which are ideally made by dividing a single-stage adaptation into sequential adaptations; I₁ and I₂ are the investment costs of the first- and the second-stage adaptation, respectively. For simplicity, it is assumed that the cost of flexibility is evenly distributed to each stage (i.e. ${I}_f=\frac{1}{2}{I}_f+\frac{1}{2}{I}_f$). The investment cost of upgrading coastal defence (i.e. impermeable revetments and seawalls) is set to be £ 64.2 million, which is an indicative cost for upgrading 15km-long coastal defence up to 3.5 mAOD level (NFDC 2010). The estimation of the costs for different heightening follows a linear relation between height and cost by the previous study (Jonkman et al. 2013) and this distribution rule (Eqs. (4) and (5)) is applied for each stage adaptation. The investment cost for each adaptation is explained in Supplement 2.

Economy efficiency of flexible adaptations under uncertainty

Quantifications of single- and multiple-stage adaptations

At the early stage of planning a coastal adaptation, option holders can take either single- or multiple-stage adaptation path. This decision affects a way to incorporate flexibility in the considered adaptation option, subsequently leading to change in economy efficiency. After the coastal adaptation is designed, the economy efficiency of the adaptation depends on how to use the flexibility. The efficiency of a single-stage adaptation can be maximised by the optimal investment based on the observation of sea-level rise. On the other hand, the size of an adaptation and the number of stages for the upgrade have effects on the efficiency of the adaptation - which is represented as option value (NPV_opt). Table 1 shows the option values of all the adaptation paths including single-stage and multiple-stage adaptations. The sets of option values for coastal adaptations are the maximum values that decision-makers can gain by the current and future decisions. The option evaluation process is explained in Supplement 3. In addition, the optimal investment times and option values for each adaptation are shown by different SLR scenarios and different flexibility premiums in the supplementary document.

Table 1. The possible option values for each adaptation pathway according to different sea-level rise scenarios by flexibility premiums

Effect of flexibility costs on economy efficiency

For comparisons between the single-stage adaptations and the multiple-stage adaptations, the option values of each adaptation path are plotted across the flexibility premiums under each SLR scenario as shown in Fig. 5.

Fig. 5.

Option values (i.e. NPV_opt) of optimal investments for each of the adaptation pathways across the premium costs by different SLR scenarios

For the H++ SLR scenario, the adaptation pathway U_{c → 4.0m} shows the highest option value among all the adaptation pathways. The single-stage adaptation path of U_{c → 3.5m} has the second-highest option value in a range of flexibility cost from 30 to 50%. The single-stage adaptation paths are more efficient than the multiple-stage adaptation paths in the extreme SLR scenario. The risk of the coastal flooding is very high and sea level is fast-growing at the rate of 2.54cm/year in the H++ SLR scenario so that an interval between the first adaptation and the next adaptation is relatively short (e.g. 30 to 40 years later). Thus, splitting the investment is inefficient in the worst-case SLR scenario. Multiple-stage adaptations including the smallest size of adaptation component (i.e. U_{c → 3.0m}) are ranked low in the H++ SLR scenario.

On the contrary, the adaptation paths extendable up to 4.0 mAOD show lower performance than the adaptation paths up to 3.0mAOD or 3.5mAOD in the mild SLR scenarios. The adaptation paths including the small size of coastal defence upgrade (i.e. U_{c → 3.0m}) are considered to be more efficient than those including the large size of coastal defence upgrade (i.e. U_{c → 3.5m} or U_{c → 4.0m}) in these cases. As these scenarios are mild comparing to the H++ SLR scenario in the risk of coastal flooding, the high standard of the coastal defence is not yet needed for Lymington. Thus, the single large investment (U_{c → 4.0m}) shows much lower efficiency, as shown in Fig. 5(c), (d) and (e), than the multiple-stage adaptation paths or the single small investment (i.e. U_{c → 3.0m}) because the high standard-of-protection coastal defence is considered as an excessive adaptation in such mild SLR scenarios.

U _{c → 3.0m} × U_{3.0m → 3.5m} shows the second-highest economy efficiency in managing coastal flood risk under most SLR scenarios except the H++ SLR scenario. In the H++ SLR scenario, U_{c → 3.0m} × U_{3.0m → 3.5m} is ranked the fourth after U_{c → 4.0m}, U_{c → 3.5m} and U_{c → 3.5m} × U_{3.5m → 4.0m}. If the mild SLR scenarios are expected to realise, only U_{c → 3.0m} will be made during the twenty-first century. Thus, the further investment will not be made if sea-level rise does not exceed the trigger value 55 cm—beyond which the implementation of U_{3.0m → 3.5m} is optimal (refer to Table 3.4 in Supplement 3). In this regard, U_{c → 3.0m} × U_{3.0m → 3.5m} is considered to be an efficient and robust strategy against the uncertain conditions of sea-level rise.

For further protection, a set of adaptations that can be raised up to 4.0 mAOD (e.g. U_{c → 3.0m} × U_{3.0m → 3.5m} × U_{3.5m → 4.0m}, U_{c → 3.0m} × U_{3.0m → 4.0m,} U_{c → 3.5m} × U_{3.5m → 4.0m} and U_{c → 4.0m}) could be taken as adaptation strategies for Lymington. However, these types of adaptations show lower performance than those extendable up to 3.5mAOD under these mild SLR scenarios. Increase in the overall costs for further protection under mild SLR scenarios leads to the inefficiency or redundancy of the overall adaptation. Nevertheless, these adaptations may be more proper to option holders who prefer to address all the range of sea-level rise.

As shown in Fig. 5(a), under the H++ SLR scenario, U_{c → 3.5m} is relatively a better strategy in the high cost of flexibility (40 to 50%) than the equivalent level of a multiple-stage adaptation (i.e. U_{c → 3.0m} × U_{3.0m → 3.5m}). It is because the high cost of flexibility increases the overall investment cost of U_{c → 3.0m} × U_{3.0m → 3.5m}. Thus, flexibility does not lead to an increase in economic efficiency under the high cost of flexibility. With this in mind, flexible coastal defence should be designed to lower the flexibility cost.

Compared in Fig. 5, the single-stage large investment may be the best option in the most extreme SLR scenario (i.e. the H++ SLR scenario). However, these types of options show the lowest performance in other mild SLR scenarios (e.g. high to low SLR scenarios). On the contrary, the single small investment (U_{c → 3.0m}) which shows the highest performance in the high to low SLR scenarios is the least adaptive to the H++ SLR scenario as it shows the lowest option value in this extreme SLR scenario. Thus, a robust decision against uncertainty is to take a multiple-stage adaptation that can perform relatively well across all the possible future scenarios.

Economy efficiency of different types of adaptations under different SLR scenarios

For an illustrative purpose, changes in option value for all the adaptation paths are visualised across the SLR scenarios in 20% and 50% flexibility premium scenarios (Fig. 6). These curves enable us to understand how to trade-off between efficiency and robustness in choosing an adaptation option under the uncertainty of sea-level rise. The process of option trade-off is detailed by the comparison of option values as below.

Fig. 6.

Changes in the option values of the adaptation paths across SLR scenarios by different flexibility premiums – Note that the SLR scenarios on the x-axis are distanced according to sea-level rise in 2100.

• (1) As U_{c → 3.0m}, U_{c → 3.5m} and U_{c → 4.0m} are all the single-stage adaptations, there is no flexibility premiums in these types of adaptations. When comparing U_{c → 3.5m} and U_{c → 4.0m}, U_{c → 3.5m} is more efficient than U_{c → 4.0m} in a range from the Historical SLR scenario to the H1 SLR scenario. On the contrary, in a range between the H1 SLR scenario and the H++ SLR scenario, the option value of U_{c → 4.0m} significantly increases to be higher than that of U_{c → 3.5m}. Nevertheless, U_{c → 4.0m} is less efficient than U_{c → 3.5m} if sea-level rise in 2100 is under 1.6m. As sea − level rise over 1.6m is physically possible but less likely, U_{c → 3.5m} would be more likely to be chosen as an efficient adaptation option when making a choice between U_{c → 3.5m} and U_{c → 4.0m}.

• (2) U _{c → 3.5m} is a more efficient option than U_{c → 3.5m} × U_{3.5m → 4.0m}. Regardless of flexibility costs, U_{c → 3.5m} gives higher option value across all the SLR scenarios than U_{c → 3.5m} × U_{3.5m → 4.0m}. Thus, U_{c → 3.5m} × U_{3.5m → 4.0m} should be rejected in option choice when comparing to U_{c → 3.5m}.

• (3) When the flexibility cost is low (e.g. 20%), U_{c → 3.0m} × U_{3.0m → 4.0m} and U_{c → 3.0m} × U_{3.0m → 3.5m} × U_{3.5m → 4.0m} may be better strategies than U_{c → 3.5m} in the low rates of SLR scenarios. On the contrary, when the flexibility cost is higher than 20%, the economic efficiency of U_{c → 3.0m} × U_{3.0m → 4.0m} and U_{c → 3.0m} × U_{3.0m → 3.5m} × U_{3.5m → 4.0m} is less than that of U_{c → 3.5m} across all the SLR scenarios. The choice of multiple stages of adaptation paths is an efficient decision when the flexibility cost is low. In the opposite cases, U_{c → 3.0m} × U_{3.5m → 4.0m} and U_{c → 3.0m} × U_{3.0m → 3.5m} × U_{3.5m → 4.0m} are less useful than U_{c → 3.5m} because a low standard-of-protection measure (i.e. U_{c → 3.0m}) in the first stage makes less efficient such high standard-of-protection adaptations (i.e. coastal adaptations up to 4.0mAOD). A low-level coastal defence before a high-level coastal adaptation seems to be less efficient combination in the design of a multiple-stage adaptation.

• (4) In terms of U_{c → 3.5m} and U_{c → 3.0m} × U_{3.0m → 3.5m}, we can see that U_{c → 3.0m} × U_{3.0m → 3.5m} is much more efficient than U_{c → 3.5m} in most of the SLR scenarios. Only in the H++ SLR scenario, U_{c → 3.5m} is more efficient than U_{c → 3.0m} × U_{3.0m → 3.5m} because the lifespan of the first stage adaptation is relatively short in the high rate of sea-level rise. As the H++ SLR scenario is a low-probability case, it is less likely that the option value of U_{c → 3.5m} is higher than that of U_{c → 3.0m} × U_{3.0m → 3.5m}. Thus, U_{c → 3.0m} × U_{3.0m → 3.5m} is likely to be a more efficient adaptation strategy than U_{c → 3.5m}.

• (5) As seen in Fig. 6(a) and (b), U_{c → 3.0m} gives the highest option value between the historical SLR scenario and the high SLR scenario. This adaptation provides protection for Lymington at the lowest cost. However, its option value is the lowest in the worst-case SLR scenario. This also implies that the least costly adaptation is very sensitive to the uncertainty of SLR scenarios. In addition, U_{c → 3.0m} is a less efficient adaptation than U_{c → 3.0m} × U_{3.0m → 3.5m} beyond the H1 SLR scenario. This adaptation option seems to be the most vulnerable to the extreme SLR scenario.

• (6) Lastly, the option evaluation of U_{c → 3.25m} × U_{3.25m → 4.0m} is included for comparison to coastal adaptations with different increments (i.e. 0.5m, 0.75m and 1m). In the relatively mild SLR scenarios (i.e. historical trend to high SLR scenarios), single- or multiple-stage adaptations starting with U_{c → 3.0m} show higher option values than U_{c → 3.25m} × U_{3.25m → 4.0m} because the low-level coastal defence, when it is set in the first stage, is more efficient than the high-level coastal defence. Likewise, U_{c → 3.25m} × U_{3.25m → 4.0m} is estimated to be more efficient than U_{c → 3.5m} × U_{3.5m → 4.0m} in the high to historical SLR scenarios. However, U_{c → 3.25m} × U_{3.25m → 4.0m} becomes less efficient than U_{c → 3.5m} × U_{3.5m → 4.0m} if sea-level rise in 2100 is over 0.7m. U_{c → 3.25m} × U_{3.25m → 4.0m} is estimated to be more efficient than U_{c → 4.0m} when sea-level rise in 2100 is below 1.5m for 20% flexibility premium. As more severe coastal flooding is expected in the extreme SLR scenario, a high level coastal defence is more effective in defending the coastal areas.

Implications of option evaluations for applications

Compared by option values, in the most extreme SLR scenario, high-level coastal defence upgrade in single stage is better than low-level coastal defence upgrade in many stages, whereas, in the mild SLR scenarios, low-level coastal defence upgrade in many stages is better than high-level coastal defence upgrade in fewer stage. For the given SLR scenarios and coastal defence conditions in Lymington, U_{c → 3.0m} × U_{3.0m → 3.5m} is considered as the most efficient adaptation to perform relatively well across all the SLR scenarios.

There are some important notions in applications to climate change adaptations. Firstly, the possible range of sea-level rise in the future has an effect on the option choice. If the possible range of sea-level rise was narrow, no change in the ranking of adaptation options might occur. In this case, an adaptation with the highest option value would be chosen within the given range of sea-level rise. For example, if the possible range of sea-level rise in 2100 was between 0.1m and 0.6m (Fig. 6(a)), the optimal option would be a small single-stage adaptation (i.e. U_{c − 3.0m}). In the other hand, if the possible range was within 0.8m to 1.4m, the two-stage adaptation of U_{c − 3.0m} × U_{3.0m − 3.5m} would be an optimal choice for protection. Thus, when the option choices are considered under uncertainty, all the adaptations need to be assessed within the uncertainty range of sea-level rise in the future. This provides an important implication as the effort towards narrowing the uncertainty range may be more helpful in the option choice than considering all the possible future states.

As the flexibility premium is a cost, it also changes the option values of the flexible adaptations. Thus, the relative orders of adaptation options in option value differ depending on how the flexibility is included in the adaptation options. The higher the flexibility cost is, the lower the option value is. However, the option values of non-flexible adaptations are constant over the flexibility premiums as they do not include the flexibility. The high flexibility premium leads to narrowing a range within which flexible adaptations are preferable to non-flexible adaptations. By comparing it with the possible range of sea-level rise, an optimal(robust) and flexible adaptation can be chosen among all the adaptations.

Thirdly, this research restricts the application of real option analysis only to physical (irreversible) types of coastal adaptations. If sea-level keeps increasing beyond the certain level, the option values of coastal adaptations start to decrease (as seen in Fig. 6). This is due to the limitation of physical capacity of coastal adaptations against extreme coastal flooding caused by sea-level rise. If such extreme coastal flooding materialize, other types of adaptations should be considered to protect coastal areas. For example, retreat from a flood zone or building new types of coastal defence may be possible adaptations which can be added to the already-made adaptation options.

Fourthly, the option values of multiple-stage adaptations reflect future learning from the future generation. The designing of coastal adaptations at the cost of the flexibility enables the future generation to resolve the uncertainty with more information. Subsequently, the remaining adaptation options provide an opportunity to make a suboptimal decision with the future information, leading to the most use of flexibility in response to the future state. There was a need to explain how to reduce the uncertainty of the future sea-level rise with real option analysis in this research. This will be met by using flexibility included in adaptation options in the future.

The impact curves that represent the relations between climatic variables and flood damages in the case study area were made in experimental setting. The accuracy of the impact curves needs to be improved for the implementation level of the decision. In addition, if the lead time of 4 to 5 years was considered for option evaluations, the investment should occur earlier. The effect of the lead time on the option value is assessed to be quantitatively small and unidirectional for all the adaptation paths when compared to other factors (e.g. sea-level rise, investment costs) because the investment cost is spread over the construction time and the benefit would occur after the construction (4 to 5 years). Generally, the spread costs increase the option value of an adaptation option while the delayed benefits decrease it. The effect of the lead time is excluded in order to simplify the option evaluation. However, this is a very practical issue when we make an investment decision. Thus, a way to find an investment time for a coastal adaptation needs to be investigated in association with a lead time for future research. This study has upgraded the coastal defence height up to 4.0 mAOD in one or two stages. We could further increase the number of stages, if appropriate. Although it provides more flexibility for adaptation pathways, it does not seem to be an efficient option because the overall investment cost may significantly rise due to the flexibility premiums.

Conclusions

This study demonstrates a real option-based framework to assess a set of adaptation pathways under a variety of SLR scenarios with alternations to flexibility premiums. Through the quantification of both single-stage and multiple-stage coastal adaptations including flexibility, we can have important implications in incorporating flexibility into adaptations.

Firstly, the multiple-stage adaptations are not always efficient than the single-stage adaptations. The efficiency of flexibility depends on both environmental and investment conditions. The single-stage adaptations with high crest levels are more likely to be efficient only in the extreme or worst cases whereas the multiple-stage adaptations can work efficiently both in the extreme and the mild SLR scenarios. As noted, the size and cost of the first-stage adaptation are crucial for the efficiency of multiple-stage adaptations. As the first-stage adaptation can reduce the present risk of coastal flooding, the benefits from the first-stage investment are monetarily realized in the short-term. Thus, taking an adaptation earlier than later is more efficient for the overall option value.

Secondly, the flexibility of wait included in coastal adaptations increases the economic efficiency of coastal adaptations. The flexibility helps decision-makers learn and observe the future. This flexibility obviously separates the future decisions from the current decisions. The future decisions (e.g. implementing the remaining adaptations) will be made with more information based on the learning and observation. On the other hand, the current decisions concern how to devise adaptation strategies now. The current decisions are of whether to choose single-stage or multiple-stage adaptation or what size of adaptation option is needed. If such decisions are made in the present, the future decisions will be also affected by the current decisions. On the other hand, the uncertainty we are concerned about now will be reduced by the future decision. Thus, the option values of the coastal adaptations will be achieved by the current and future decisions.

Lastly, there are a myriad of possible SLR scenarios one of which will materialize in the future. It is also possible that none of them will not occur in the future. In this regard, the SLR scenarios used in this analysis are our expectations towards the future from the current perspective. In addition, the uncertainty about what SLR scenario we will be on cannot be resolved by real option-based analysis. However, the decision based on the real option analysis leaves coastal adaptations open to various future states. Also, the real option evaluation process provides a basis upon which the coastal adaptations are quantitatively compared under all the future states. Thus, the future generation will be able to resolve the risk of coastal flooding by using the remaining coastal adaptations in an economically efficient way, even though the uncertainty of coastal flooding is deep in the present.