An analytical model for tidal evolution in co-orbital systems. I. Application to exoplanets

Abstract

Close-in co-orbital planets (in a 1:1 mean-motion resonance) can experience strong tidal interactions with the central star. Here, we develop an analytical model adapted to the study of the tidal evolution of those systems. We use a Hamiltonian version of the constant time-lag tidal model, which extends the Hamiltonian formalism developed for the point-mass case. We show that co-orbital systems undergoing tidal dissipation favour either the Lagrange or the anti-Lagrange configurations, depending on the system parameters. However, for all range of parameters and initial conditions, both configurations become unstable, although the timescale for the destruction of the system can be larger than the lifetime of the star. We provide an easy-to-use criterion to determine whether an already known close-in exoplanet may have an undetected co-orbital companion.

Appendices

Appendices

Notations

We gather here for convenience the notations used throughout this work.⁵

Table 4 Notations

Coefficients of the Hamiltonian $\displaystyle {\mathcal {H}_4}$ and $\displaystyle {\mathcal {H}_t^j}$

First, we give the coefficients depending on $\displaystyle {\xi }$ of the Hamiltonian $\displaystyle {\mathcal {H}_4}$. We recall that $\displaystyle {\mathcal {H}_4}$ is expressed

$$\begin{aligned} \begin{aligned} \mathcal {H}_4&=\frac{1}{4}\frac{m}{m_0}\left\{ {\,}D_h\left( X_1^2\bar{X}_1^2+X_2^2\bar{X}_2^2\right) +E_hX_1^2\bar{X}_2^2+\bar{E}_hX_2^2\bar{X}_1^2\right. \\&\quad \left. +\,F_h\left( X_1X_2\bar{X}_1^2+\bar{X}_1\bar{X}_2X_2^2\right) +\bar{F}_h\left( \bar{X}_1\bar{X}_2X_1^2+X_1X_2\bar{X}_2^2\right) +G_hX_1X_2\bar{X}_1\bar{X}_2{\,}\right\} . \end{aligned}\nonumber \\ \end{aligned}$$

(71)

The coefficients read $\displaystyle {\left( \text {recall that }\varDelta =\sqrt{2-2\cos \xi }\right) }$

$$\begin{aligned} \begin{aligned} D_h&=\frac{7}{16}\cos \xi +\frac{1}{4\varDelta ^9}\left( -\frac{3951}{32}+115\cos \xi +\frac{293}{8}\cos 2\xi -27\cos 3\xi -\frac{37}{32}\cos 4\xi \right) , \\ G_h&=\cos \xi +\varDelta ^{-9}\left( -\frac{4491}{32}+139\cos \xi +\frac{233}{8}\cos 2\xi -27\cos 3\xi -\frac{25}{32}\cos 4\xi \right) ,\\ E_h&=\frac{1}{32}\left( e^{-i\xi }+81e^{-3i\xi }\right) +\frac{e^{-6i\xi }}{32\varDelta ^9}P_E\left( e^{i\xi }\right) ,\\ F_h&=-\frac{7}{4}e^{2i\xi }+\frac{e^{-3i\xi }}{4\varDelta ^9}P_F\left( e^{i\xi }\right) ,\\ P_E\!\left( X\right)&=-\frac{9}{8}\!+\!15X\!-\!\frac{349}{2}X^2\!+\!171X^3\!+\!\frac{2889}{4}X^4\!-\!1571X^5\!+\!\frac{2007}{2}X^6\!-\!87X^7\!-\!\frac{625}{8}X^8,\\ P_F\!\left( X\right)&=\frac{207}{32}\!+\!\frac{303}{8}X\!-\!\frac{577}{4}X^2\!+\!\frac{603}{8}X^3\!+\!\frac{2511}{16}X^4\!-\!\frac{1475}{8}X^5\!+\!45X^6\!+\!\frac{57}{8}X^7\!-\!\frac{5}{32}X^8. \end{aligned} \end{aligned}$$

(72)

We now give the expressions of the coefficients appearing in the tidal Hamiltonian (41) for the second and fourth order in eccentricity. We have

$$\begin{aligned} \begin{aligned}&B_t^j=\frac{3}{8}-\frac{15}{8}\cos 2\left( \Delta \lambda _j-\Delta \theta _j \right) ,\\&C_t^j=\frac{3}{32}e^{i\left( \Delta \lambda _j-2\Delta \theta _j \right) }+\frac{9}{16}e^{-i\Delta \lambda _j}+\frac{147}{32}e^{-i\left( 3\Delta \lambda _j-2\Delta \theta _j \right) },\\&D_t^j=\frac{3}{8}+\frac{69}{64}\cos 2\left( \Delta \lambda _j-\Delta \theta _j\right) ,\\&G_t^j=\frac{9}{16}+\frac{75}{16}\cos 2\left( \Delta \lambda _j-\Delta \theta _j\right) ,\\&E_t^j=\frac{81}{64}e^{-2i\Delta \lambda _j}+\frac{867}{32}e^{-2i\left( 2\Delta \lambda _j-\Delta \theta _j\right) },\\&F_t^j=\frac{9}{16}e^{i\Delta \lambda _j}-\frac{3}{128}e^{-i\left( \Delta \lambda _j-2\Delta \theta _j\right) }-\frac{1365}{128}e^{i\left( 3\Delta \lambda _j-2\Delta \theta _j\right) }, \end{aligned} \end{aligned}$$

(73)

with

$$\begin{aligned} \Delta \lambda _j=\lambda _j-\lambda _j^{\bigstar }\;\;\text { and }\;\;\Delta \theta _j=\theta _j-\theta _j^{\bigstar }. \end{aligned}$$

(74)

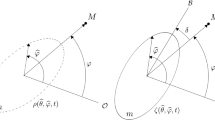
Lagrange and anti-Lagrange in horseshoe-shaped orbits

Here, we show that the Lagrange and anti-Lagrange proper modes correspond to aligned and anti-aligned pericentres in horseshoe-shaped orbits. The matrix of the variational equations (27), once averaged over the semi fast dynamics and according to the geometrical considerations stated at the end of Sect. 2.3, reads

$$\begin{aligned} \mathcal {M}_0=-i\begin{pmatrix}\frac{m_2}{m_0}\underline{A}_h&{}\quad \frac{m_2}{m_0}\underline{B}_h\\ \frac{m_1}{m_0}\underline{B}_h&{}\quad \frac{m_1}{m_0}\underline{A}_h \end{pmatrix}, \end{aligned}$$

(75)

where both $\displaystyle {\underline{A}_h}$ and $\displaystyle {\underline{B}_h}$, average of $\displaystyle {A_h}$ and $\displaystyle {B_h}$ over the semi-fast dynamics, are real. The eigenvectors of $\displaystyle {\mathcal {M}_0}$ show that for the Lagrange configuration

$$\begin{aligned} \arg \left( \frac{X_1}{X_2}\right) =\varpi _1-\varpi _2=0, \end{aligned}$$

(76)

while for the anti-Lagrange configuration

$$\begin{aligned} \arg \left( \frac{X_1}{X_2}\right) =\varpi _1-\varpi _2=\pi . \end{aligned}$$

(77)

This corresponds to aligned and anti-aligned pericentres.

Conservation of the total angular momentum

Here, we show that the set of equations (51) is consistent with the conservation of the total angular momentum of the system. The normalized⁶ total angular momentum $\displaystyle {\mathcal {C}}$ reads (Robutel and Pousse 2013)

(78)

From (51), we get

$$\begin{aligned} \dot{\mathcal {C}}=\sum _{j\in \left\{ 1,2\right\} }3\frac{q_j}{Q_j}\frac{m_0}{m}\mathcal {R}_j^{-13}X_j\bar{X}_j\left\{ {\,}h_2^j-k_2^j+p_2^j+\mathcal {R}_j^{-1}X_j\bar{X}_j\left( h_4^j-k_4^j+p_4^j \right) {\,}\right\} , \end{aligned}$$

(79)

and the total angular momentum is conserved since we have

$$\begin{aligned} \begin{aligned}&h_2^j-k_2^j+p_2^j=0 \quad \text { and}\\&h_4^j-k_4^j+p_4^j=0. \end{aligned} \end{aligned}$$

(80)

Diagonalization of a perturbed matrix

We show here the method that we use to obtain the eigenvalues of a perturbed matrix once a diagonal basis of the principal matrix is known. Indeed, the computation of the eigenvalues (60) is equivalent to finding the roots of the characteristic polynomial of $\displaystyle {\mathcal {Z}_0+\mathcal {Z}_1}$, given in Eqs. (94) and (95). Even when the degree of this polynomial is reduced to four using the $\displaystyle {0}$ eigenvalue, it is hard to obtain its roots in a convenient form. The method we use here, briefly presented by Laskar et al. (2012), gives the eigenvalues and eigenvectors very easily.

Let $\displaystyle {\mathcal {M}=\mathcal {M}_0+\zeta \mathcal {M}_1\in \mathcal {M}_n\left( \mathbb {C}\right) }$ be a $\displaystyle {n\times n}$ complex matrix where $\displaystyle {\zeta }$ is a small quantity with respect to $\displaystyle {1}$. Assume that we know a diagonal basis for $\displaystyle {\mathcal {M}_0}$

$$\begin{aligned} \mathcal {D}_0=P_0^{-1}\mathcal {M}_0P_0=\text {diag}\left( \lambda _i\right) , \end{aligned}$$

(81)

where the columns of $\displaystyle {P_0}$ are the eigenvectors of $\displaystyle {\mathcal {M}_0}$ and the $\displaystyle {\lambda _i}$ its eigenvalues, which are not assumed to be of multiplicity one but which are assumed to be sorted by value, that is, equal eigenvalues are consecutive. This does not restrict the generality, as any permutation can be applied on the columns of $\displaystyle {P_0}$ to achieve that. We now define

$$\begin{aligned} \mathcal {Q}_1=P_0^{-1}\mathcal {M}_1P_0. \end{aligned}$$

(82)

If $\displaystyle {P}$ is the matrix of the eigenvectors of $\displaystyle {\mathcal {D}_0+\zeta \mathcal {Q}_1}$, and since $\displaystyle {\mathcal {D}_0+\zeta \mathcal {Q}_1}$ is near diagonal, we write

$$\begin{aligned} P=I_n+\zeta P_1+\mathcal {O}\left( \zeta ^2\right) . \end{aligned}$$

(83)

We have

$$\begin{aligned} P^{-1}\left( \mathcal {D}_0+\zeta \mathcal {Q}_1\right) P=\mathcal {D}_0+\zeta \left( {\,}\mathcal {Q}_1+\left[ \mathcal {D}_0,P_1\right] {\,}\right) +\mathcal {O}\left( \zeta ^2\right) , \end{aligned}$$

(84)

where $\displaystyle {\left[ \mathcal {D}_0,P_1\right] =\mathcal {D}_0P_1-P_1\mathcal {D}_0}$. Thus, the cohomological equation

$$\begin{aligned} \mathcal {Q}_1+\left[ \mathcal {D}_0,P_1\right] =\mathcal {D}_1, \end{aligned}$$

(85)

where $\displaystyle {\mathcal {D}_1=\text {diag}\left( q_{i,i}\right) }$ is the diagonal matrix composed of the diagonal terms of $\displaystyle {\mathcal {Q}_1}$. The solution of the cohomological equation is

$$\begin{aligned} p_{i,j}=\left\{ \begin{array}{ll} \frac{q_{i,j}}{\lambda _j-\lambda _i}&{}\quad \text { if }\lambda _i\ne \lambda _j, \\ 0&{}\quad \text { else},\end{array}\right. \end{aligned}$$

(86)

where

$$\begin{aligned} \mathcal {Q}_1=\left( q_{i,j}\right) _{1\le i,j\le n}\;\;\;\;\;\text {and}\;\;\;\;\;P_1=\left( p_{i,j}\right) _{1\le i,j\le n}. \end{aligned}$$

(87)

The matrix $\displaystyle {\mathcal {M}_0+\zeta \mathcal {M}_1}$ is now block diagonal:

$$\begin{aligned} P^{-1}P_0^{-1}\left( \mathcal {M}_0+\zeta \mathcal {M}_1\right) P_0P=\text {diag}\left( \mathcal {B}_0^1+\zeta \mathcal {B}_1^1,\,...\,,\mathcal {B}_0^r+\zeta \mathcal {B}_1^r\right) ,\;\;\;\;\;r\le n \end{aligned}$$

(88)

where $\displaystyle {\forall i\le r\;\;\exists k\le n}$ such that

$$\begin{aligned} \mathcal {B}_0^i=\lambda _kI_{m(k)}, \end{aligned}$$

(89)

and $\displaystyle {m(k)}$ denotes the multiplicity of $\displaystyle {\lambda _k}$ and thus the size of the block. The computation of the eigenvalues of $\displaystyle {\mathcal {M}_0+\zeta \mathcal {M}_1}$ is reduced to the computation of the eigenvalues of the blocks $\displaystyle {\mathcal {B}_0^i+\zeta \mathcal {B}_1^i}$ who are hopefully all of small size and whose eigenvalues are then analytically easily found.

Linearization

Near the fixed points given by (53), the linear system reads

$$\begin{aligned} \dot{\mathcal {X}}=\left( \mathcal {Q}_0+\mathcal {Q}_1\right) \mathcal {X}, \end{aligned}$$

(90)

where $\displaystyle {\mathcal {X}}$ is defined in Sect. 3.2.2. We have

$$\begin{aligned} \mathcal {Q}_0=\begin{pmatrix}\mathcal {Z}_0&{}\quad 0_{5,2}\\ 0_{2,5}&{}\quad \mathcal {M}_0\end{pmatrix}\;\;\text { and }\;\;\mathcal {Q}_1=\begin{pmatrix}\mathcal {Z}_1&{}\quad 0_{5,2}\\ 0_{2,5}&{}\quad \mathcal {M}_1\end{pmatrix}, \end{aligned}$$

(91)

where

$$\begin{aligned} \mathcal {M}_0= & {} \frac{27}{8}i\begin{pmatrix}\frac{m_2}{m_0}&{}-\frac{m_2}{m_0}e^{i\pi /3}\\ -\frac{m_1}{m_0}e^{-i\pi /3}&{}\frac{m_1}{m_0}\end{pmatrix}, \end{aligned}$$

(92)

$$\begin{aligned} \mathcal {M}_1= & {} -\frac{21}{2}\text {diag}\left\{ {\,}q_1\frac{m_0}{m_1}\left( \eta \Delta t_1-\frac{5}{7}i\right) ,q_2\frac{m_0}{m_2}\left( \eta \Delta t_2-\frac{5}{7}i\right) {\,}\right\} , \end{aligned}$$

(93)

$$\begin{aligned} \mathcal {Z}_0= & {} \begin{pmatrix} 0&{}\quad 0&{}\quad 0&{}\quad 0&{}\quad 0\\ 0&{}\quad 0&{}\quad 0&{}\quad 0&{}\quad 0\\ 0&{}\quad 0&{}\quad 0&{}\quad -3\gamma &{}\quad 0\\ 0&{}\quad 0&{}\quad \frac{1}{3}\gamma ^{-1}\nu ^2&{}\quad 0&{}\quad 0\\ 0&{}\quad 0&{}\quad 0&{}\quad 0&{}\quad 0 \end{pmatrix}, \end{aligned}$$

(94)

$$\begin{aligned} \mathcal {Z}_1= & {} \begin{pmatrix} -d_1 &{}\quad 0 &{}\quad 0 &{}\quad 3\gamma ^{-1}\delta ^{-1}d_1 &{}\quad 3\gamma ^{-1}d_1 \\ 0 &{}\quad -d_2 &{}\quad 0 &{}\quad -3\gamma \delta d_2 &{}\quad 3\gamma ^{-1}d_2 \\ 0 &{}\quad 0 &{}\quad 0 &{}\quad -\gamma \left( \delta c_2+\left( 1-\delta \right) c_1\right) &{}\quad \gamma ^{-1}\left( c_2-c_1\right) \\ -\left( 1-\delta \right) b_1 &{}\quad \delta b_2 &{}\quad 0 &{}\quad 3\gamma \left( \delta ^2b_2+\left( 1-\delta \right) ^2b_1 \right) &{}\quad 3\gamma ^{-1}\left[ \left( 1-\delta \right) b_1-\delta b_2\right] \\ -b_1 &{}\quad -b_2 &{}\quad 0 &{}\quad 3\gamma ^{-1}\delta ^{-1}b_1-3\gamma \delta b_2 &{}\quad 3\gamma ^{-1}\left( b_1+b_2 \right) \end{pmatrix},\nonumber \\ \end{aligned}$$

(95)

with

(96)

Near $\displaystyle {L_{4,5}}$, the eigenvectors of $\displaystyle {\mathcal {M}_0+\mathcal {M}_1}$, computed using results from “Appendix E” reveal that the Lagrange configuration corresponds to

$$\begin{aligned} \begin{aligned}&\varpi _1-\varpi _2=\frac{\pi }{3}+\frac{28}{9}\frac{m_0^2\left( m_1q_2/Q_2+m_2q_1/Q_1\right) }{m_1m_2\left( m_1+m_2\right) },\\ {}&\frac{e_1}{e_2}=1+\frac{20}{9}\frac{m_0^2\left( q_2m_1-q_1m_2\right) }{m_1m_2\left( m_1+m_2\right) }, \end{aligned} \end{aligned}$$

(97)

while the anti-Lagrange configuration complies with

$$\begin{aligned} \begin{aligned}&\varpi _1-\varpi _2=\frac{4\pi }{3}-\frac{28}{9}\frac{m_0^2\left( m_1q_2/Q_2+m_2q_1/Q_1\right) }{m_1m_2\left( m_1+m_2\right) },\\&\frac{e_1}{e_2}=\frac{m_2}{m_1}\left( 1-\frac{20}{9}\frac{m_0^2\left( q_2m_1-q_1m_2\right) }{m_1m_2\left( m_1+m_2\right) }\right) . \end{aligned} \end{aligned}$$

(98)

Direct 3-body model

The complete equations of motion governing the tidal evolution of a three-body system in an astrocentric frame using a linear constant time-lag tidal model are given by (Mignard 1979)

$$\begin{aligned} \begin{aligned} \frac{\mathrm{d}^2{\vec {r}}_1}{\mathrm{d}t^2}&= -\frac{\mu _1}{r_1^3}\vec {r}_1+ \mathcal {G} m_2\left( \frac{\vec {r}_2-\vec {r}_1}{|\vec {r}_2-\vec {r}_1|^3}-\frac{\vec {r}_2}{r_2^3}\right) + \frac{\vec {f}_1}{\beta _1} + \frac{\vec {f}_2}{m_0}, \\ \frac{\mathrm{d}^2{\vec {r}}_2}{\mathrm{d}t^2}&= -\frac{\mu _2}{r_2^3}\vec {r}_2+ \mathcal {G} m_1\left( \frac{\vec {r}_1-\vec {r}_2}{|\vec {r}_1-\vec {r}_2|^3}-\frac{\vec {r}_1}{r_1^3}\right) + \frac{\vec {f}_2}{\beta _2} + \frac{\vec {f}_1}{m_0}, \\ \frac{\mathrm{d}^2{\theta }_i}{\mathrm{d}t^2}&= - \frac{(\vec {r}_i \times \vec {f}_i)\cdot \vec {k}}{C_i} = - 3 \frac{\kappa _{2, i} {{\mathcal {G}}} m_0^2 R_i^3}{\alpha _i m_i r_i^{8}} \Delta t_i \left[ \frac{\mathrm{d}{\theta }_i}{\mathrm{d}t} \, r_i^2 - \left( \vec {r}_i \times \frac{\mathrm{d}{\vec {r}}_i}{\mathrm{d}t} \right) \cdot \vec {k} \right] , \end{aligned} \end{aligned}$$

(99)

where $\vec {r}_i$ and $\theta _i$ are the astrocentric position vector and the rotation angle of the planet i, respectively, $\vec {k}$ is the unit vector normal to the orbital plane of the planets and $\vec {f}_i$ is the force arising from the tidal potential energy created by the deformation of each planet [Eq. (33)]

$$\begin{aligned} \vec {f}_i = - 3 \frac{\kappa _{2, i} {{\mathcal {G}}} m_0^2 R_i^5}{r_i^{8}} \vec {r}_i -3 \frac{\kappa _{2, i} {{\mathcal {G}}} m_0^2 R_i^5}{r_i^{10}} \Delta t_i \left[ 2 \left( \vec {r}_i \cdot \frac{\mathrm{d}{\vec {r}}_i}{\mathrm{d}t} \right) \vec {r}_i + r_i^2 \left( \frac{\mathrm{d}{\theta }_i}{\mathrm{d}t} \, \vec {r}_i \times \vec {k} + \frac{\mathrm{d}{\vec {r}}_i}{\mathrm{d}t} \right) \right] .\nonumber \\ \end{aligned}$$

(100)