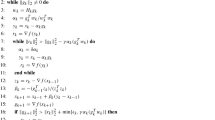Skip to main content
SpringerLink
Log in

Iterative Methods to Calculate Weighted Pseudoinverses with Mixed Weights

  • SYSTEMS ANALYSIS
  • Published:
Cybernetics and Systems Analysis Aims and scope

Abstract

The authors have obtained and analyzed the expansions of weighted pseudoinverses with mixed weights (one of the weight matrices is positive definite and the other is nonsingular indefinite) into matrix power series with positive exponents. Iterative methods for calculation of weighted pseudoinverces with mixed weights have been generated and investigated on the basis of the obtained expansions of weighted pseudoinverses. Different variants of weighted pseudoinverces with mixed nonsingular weights are analyzed and developed into matrix power series.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. J. S. Chipman, “On least squares with insufficient observation,” J. Amer. Statist. Assoc., Vol. 59, No. 308, 1078–1111 (1964).

    Article  MathSciNet  Google Scholar 

  2. R. D. Milne, “An oblique matrix pseudoinverse,” SIAM J. Appl. Math., Vol. 16, No. 5, 931–944 (1968).

    Article  MathSciNet  Google Scholar 

  3. J. F. Ward, T. L. Boullion, and T. O. Lewis, “A note on the oblique matrix pseudoinverse,” SIAM J. Appl. Math., Vol. 20, No. 2, 173–175 (1971).

    Article  MathSciNet  Google Scholar 

  4. J. F. Ward, T. L. Boullion, and T. O. Lewis, “Weighted pseudoinverses with singular weights,” SIAM J. Appl. Math., Vol. 21, No. 3, 480–482 (1971).

    Article  MathSciNet  Google Scholar 

  5. E. F. Galba, V. S. Deineka, and I. V. Sergienko, “Weighted pseudoinverses and weighted normal pseudosolutions with singular weights,” Comp. Math. Math. Physics, Vol. 49, No. 8, 1281–1297 (2009).

    Article  Google Scholar 

  6. I. V. Sergienko, E. F. Galba, and V. S. Deineka, “Existence and uniqueness of weighted pseudoinverse matrices and weighted normal pseudosolutions with singular weights,” Ukr. Math. J., Vol. 63, No. 1, 98–124 (2011).

    Article  MathSciNet  Google Scholar 

  7. I. V. Sergienko, E. F. Galba, and V. S. Deineka, “Existence and uniqueness theorems in the theory of weighted pseudoinverses with singular weights,” Cybern. Syst. Analysis, Vol. 47, No. 1, 11–28 (2011).

    Article  MathSciNet  Google Scholar 

  8. I. V. Sergienko and E. F. Galba, “Weighted pseudoinversion with singular weights,” Cybern. Syst. Analysis, Vol. 52, No. 5, 708–729 (2016).

    Article  MathSciNet  Google Scholar 

  9. E. F. Galba and I. V. Sergienko, “Methods for cmputing weighted pseudoinverses and weighted normal pseudosolutions with singular weights,” Cybern. Syst. Analysis, Vol. 54, No. 3, 398–422 (2018).

    Article  Google Scholar 

  10. S. K. Mitra amd C. R. Rao, “Projections under seminorms and generalized Moore–Penroze inverses,” Linear Algebra and Appl., No. 9, 155–167 (1974).

  11. C. R. Rao and S. K. Mitra, Generalized Inverse of Matrices and Its Applications, Wiley, New York (1971).

    MATH  Google Scholar 

  12. N. A. Varenyuk, E. F. Galba, I. V. Sergienko, and A. N. Khimich, “Weighted pseudoinversion with indefinite weights,” Ukr. Math. J., Vol. 70, No. 6, 866–889 (2018).

    Article  MathSciNet  Google Scholar 

  13. E. F. Galba and N. A. Vareniuk, “Representing weighted pseudoinverse matrices with mixed weights on terms of other pseudoinverses,” Cybern. Syst. Analysis, Vol. 54, No. 2, 185–192 (2018).

    Article  Google Scholar 

  14. E. F. Galba and N. A. Vareniuk, “Expansion of weighted pseudoinverses with mixed weights into matrix power series and power products,” Cybern. Syst. Analysis, Vol. 55, No. 5, 760–771 (2019).

    Article  Google Scholar 

  15. N. A. Vareniuk, E. F. Galba, I.V. Sergienko, and N. I. Tukalevska, “Iterative methods for calculating weighted pseudoinverses with mixed weights based on their expansion into matrix power series,” Dopov. Nac. Akad. Nauk Ukr., No. 8, 19–25 (2020).

  16. L. S. Pontryagin, “Hermitian operators in spaces with indefinite metrics,” Izvestiya AN USSR, Ser. Matem., Vol. 8, 243–280 (1944).

  17. A. I. Maltsev, Fundamentals of Linear Algebra [in Russian], Gostekhizdat, Moscow–Leningrad (1948).

    Google Scholar 

  18. J. Bognar, Indefinite Inner Product Spaces, Springer Verlag, Berlin–Heidelberg–New York (1974).

    Book  Google Scholar 

  19. I. Gohberg, P. Lancaster, and L. Rodman, Matrices and Indefinite Scalar Products, Birkhauser–Basel–Boston–Stuttgart (1983).

  20. T. Ya. Azizov and I. S. Iohvidov, Linear Operators in Spaces with an Indefinite Metric, John Wiley and Sons, Ltd., Chichester (1989).

  21. P. Lancaster and P. Rozsa, “Eigenvectors of H-self-adjoint matrices,” Z. Angew. Math. und Mech., Vol. 64, No. 9, 439–441 (1984).

    Article  MathSciNet  Google Scholar 

  22. Kh. D. Ikramov, “On algebraic properties of classes of pseudopermutation and H-self-adjoint matrices,” Zh. Vych. Mat. Mat. Fiz., Vol. 32, No. 8, 155–169 (1992).

    Google Scholar 

  23. V. V. Voevodin and Yu. A. Kuznetsov, Matrices and Calculations [in Russian], Nauka, Moscow (1984).

    Google Scholar 

  24. P. Horn and C. Johnson, Matrix Analysis, Cambridge Univ. Press (1989).

  25. C. F. Van Loan, “Generalizing the singular value decomposition,” SIAM J. Numer. Anal., Vol. 13, No. 1, 76–83 (1976).

    Article  MathSciNet  Google Scholar 

  26. Y. Wei and D. Wang, “Condition numbers and perturbation of the weighted Moore–Penrose inverse and weighted linear least squares problem,” Appl. Math. Comput., Vol. 145, 45–58 (2003).

    MathSciNet  MATH  Google Scholar 

  27. Y. Wei, “A note on the sensitivity of the solution of the weighted linear least squares problem,” Appl. Math. Comput., Vol. 145, 481–485 (2003).

    MathSciNet  MATH  Google Scholar 

  28. A. N. Khimich and E. A. Nikolaevskaya, “Reliability analysis of computer solutions of systems of linear algebraic equations with approximate initial data,” Cybern. Syst. Analysis, Vol. 44, No. 6, 863–874 (2008).

    Article  Google Scholar 

  29. E. A. Nikolaevskaya and A. N. Khimich, “Error estimation for a weighted minimum-norm least squares solution with positive definite weights,” Comp. Math. Math. Physics, Vol. 49, No. 3, 409–417 (2009).

    Article  Google Scholar 

  30. A. N. Khimich, “Perturbation bounds for the least squares problem,” Cybern. Syst. Analysis, Vol. 32, No. 3, 434–436 (1996).

    Article  MathSciNet  Google Scholar 

  31. I. V. Sergienko, E. F. Galba, and V. S. Deineka, “Representations and expansions of weighted pseudoinverse matrices, iterative methods, and problem regularization. I. Positive definite weights,” Cybern. Syst. Analysis, Vol. 44, No. 1, 36–55 (2008).

    Article  Google Scholar 

  32. I. V. Sergienko, E. F. Galba, and V. S. Deineka, “Representations and expansions of weighted pseudoinverse matrices, iterative methods, and problem regularization. II. Singular weights,” Cybern. Syst. Analysis, Vol. 44, No. 3, 278–308 (2008).

    Article  Google Scholar 

  33. A. N. Khimich, A. V. Popov, and V. V. Polyanko, “Algorithms of parallel computations linear algebra problems with of irregularly structured matrices,” Cybern. Syst. Analysis, Vol. 47, No. 6, 973–985 (2011).

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. V. M. Glushkov Institute of Cybernetics, National Academy of Sciences of Ukraine, Kyiv, Ukraine

    N. A. Vareniuk, E. F. Galba, I. V. Sergienko & N. I. Tukalevska

Authors
  1. N. A. Vareniuk
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. E. F. Galba
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. I. V. Sergienko
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. N. I. Tukalevska
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to N. A. Vareniuk.

Additional information

Translated from Kibernetyka ta Systemnyi Analiz, No. 3, May–June, 2021, pp. 3–20.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Vareniuk, N.A., Galba, E.F., Sergienko, I.V. et al. Iterative Methods to Calculate Weighted Pseudoinverses with Mixed Weights. Cybern Syst Anal 57, 337–353 (2021). https://doi.org/10.1007/s10559-021-00359-5

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10559-021-00359-5

Keywords

Search

Navigation