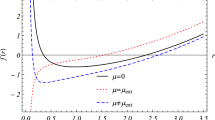
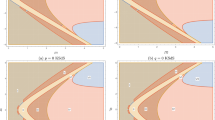
Abstract
The aim of present work is to extend the application of Weitzenböck Induced Matter Theory (WIMT) to a dyonic Reissner-Nordström Black Hole (RNBH), by proposing a condition compatible with a quantization relation between gravitational mass and both magnetic and electric charges from a geometric product defined as an invariant in 5D.
Similar content being viewed by others
Notes
A \(p\)-form is a tensor object which we call \(W\) in present footnote, this is \(p\) times cotangent and totally antisymmetric
$$\begin{aligned} W=\frac{1}{p!}\,w_{i_{1}\,\ldots\,i_{p}}\, \underrightarrow{e}^{i_{1}}\wedge \cdots\wedge\underrightarrow{e}^{i_{p}}, \end{aligned}$$in which wedge product is the complete anti-symmetrization of the tensorial product. The exterior covariant derivative is linked to a covariant derivative by
$$\begin{aligned} d(W)=\frac{1}{p!}\,w_{i_{1}\,\ldots\,i_{p}\,;k}\, \underrightarrow{e}^{k} \wedge\underrightarrow{e}^{i_{1}}\wedge\cdots\wedge \underrightarrow{e}^{i_{p}}, \end{aligned}$$each different covariant derivative ; defines a different exterior derivative. The adjoint operation denoted by ∗ is defined in a manifold of dimension \(m\) with the expression
$$\begin{aligned} {*W}=\frac{\sqrt{|g|}}{(m-p)!\,p!}\varepsilon _{j_{1}\,\ldots\,j_{p}i_{p+1}\,\ldots\,i_{n}}w^{j_{1}\,\ldots\,j_{p}}\, \underbrace{ \underrightarrow{e}^{i_{p+1}}\wedge\cdots\wedge\underrightarrow {e}^{i_{m}}}_{m-p}, \end{aligned}$$then adjoint operation interchanges tensor order from \(p\) to \(m-p\). Taking last three equations we must write the magnetic current of the Maxwell equations as in (3). For more details, the reader can see Grøn and Hervik (2007).
The 5D invariants proposed in Romero and Bellini (2015b), are
$$\begin{aligned} &^{(m)}\underrightarrow{\underrightarrow{J}} \bigl(\, ^{(e)}\overrightarrow{J},\overrightarrow{U} \bigr):= {}^{(e)}J^{a}\, ^{(m)}J_{ab} \,U^{b}= {}^{(m)}J_{a} \,^{(e)}J^{a}, \end{aligned}$$(12)$$\begin{aligned} &^{(m)}J:= {}^{(m)}\underrightarrow{J} ( \overrightarrow{U} )={}^{(m)}J_{a}\,U^{a}, \end{aligned}$$(13)$$\begin{aligned} &^{(e)}J:={}^{(e)}\underrightarrow{J} ( \overrightarrow{U} )={}^{(e)}J_{a}\,U^{a}, \end{aligned}$$(14)$$\begin{aligned} &\begin{aligned}[b] ^{(gem)}J^{2}&:= \bigl(^{ (e)}\underrightarrow{J} \, \wedge {}^{(m)} \underrightarrow{J} \bigr) \bigl(^{(e)}\overrightarrow{J}\,\wedge {}^{(m)}\overrightarrow{J} \bigr) \\ &= {}^{(gem)}J_{ab}\,^{(gem)}J^{ab}, \end{aligned} \end{aligned}$$(15)where \(^{(gem)}\underrightarrow{\underrightarrow{J}}= {}^{(ge)}\underrightarrow{J} \,\wedge {}^{(gm)}\underrightarrow{J}\) are the gravito-electro-magnetic 2-form, and \(U^{b}\) are the components of the penta-velocities of the observers.
For a general multi-tensor object
$$\begin{aligned} T & = A+B_{n} \underrightarrow{e}^{n}+C_{nm} \underrightarrow{e}^{n} \otimes \underrightarrow{e}^{m} \\ &\quad + D_{nmp}\underrightarrow{e}^{n} \otimes \underrightarrow{e}^{m} \otimes\underrightarrow{e}^{p}+\cdots \end{aligned}$$(20)where \(A, B_{n}, C_{nm}, D_{nmp}\) are arbitrary scalar functions in \(\mathfrak{F}(M)\). We must obtain a scalar defined by
$$\begin{aligned} T^{2} =&A^{2}+ B_{n} B_{n'} \overrightarrow{\overrightarrow {g}}\bigl(\underrightarrow{e}^{n}, \underrightarrow{e}^{n'}\bigr) \\ &{}+ C_{nm} C_{n'm'} \overrightarrow{\overrightarrow{g}} \bigl(\underrightarrow {e}^{n},\underrightarrow{e}^{n'}\bigr) \overrightarrow{\overrightarrow {g}}\bigl(\underrightarrow{e}^{m}, \underrightarrow{e}^{m'}\bigr) \\ &{}+ D_{nmp} D_{n'm'p'} \overrightarrow{\overrightarrow {g}} \bigl(\underrightarrow{e}^{n},\underrightarrow{e}^{n'}\bigr) \overrightarrow {\overrightarrow{g}}\bigl(\underrightarrow{e}^{m}, \underrightarrow{e}^{m'}\bigr) \\ &{}\times \overrightarrow{\overrightarrow{g}}\bigl(\underrightarrow {e}^{p},\underrightarrow{e}^{p'}\bigr) + \cdots \\ =& A^{2} + B_{n} B_{n'} g^{nn'}+ C_{nm} C_{n'm'} g^{nn'} g^{mm'} \\ &{}+ D_{nmp} D_{n'm'p'} g^{nn'} g^{mm'} g^{pp'}+\cdots , \end{aligned}$$(21)which is a generalized expression of a inner product for multi-tensorial objects of any kind. We must notice that such product coincides with the usual inner product for a pure vector or co-vector.
The reader can see the Sect. 3 of Romero and Bellini (2015b), where we demonstrated that
$$\begin{aligned} \bigl[^{(gm)}J_{A}\bigr]=\bigl(-\rho_{m} \, U^{5},0,0,0,\rho_{m} \, U^{1}\bigr), \quad \text{with} \ {}^{(gm)}J_{i}=0, \end{aligned}$$and
$$\begin{aligned} \bigl[^{(ge)}J_{A}\bigr]=( \rho_{e},0,0,0,\rho_{M}). \end{aligned}$$(26)The radius remains real for \(\frac{4m^{8}}{n^{2}-m^{2}}>\frac {{U^{5}}^{2}-{U^{1}}^{2}}{U^{5}}\), which reduces to \(\frac{4m^{8}}{n^{2}-m^{2}}>1\) over the horizon.
The Latin index \(n,m,p=0\ldots 4\) are associated to the 5D manifold taking values along the five coordinates, the Greek index \(\alpha,\beta=0\ldots3\) are associated to the effective 4D space-time taking values along the four effective coordinates obtained after the foliation.
References
Baez, J., Muniain, J.P.: Gauge Fields, Knots and Gravity. World Scientific, Singapore-New Jersey-London-Hong Kong (1994)
Casadio, R., Micu, O., Stojkovic, D.: J. High Energy Phys. 1505, 096 (2015)
De Sabbata, V., Kumar Datta, B.: Geometric Algebra and Applications to Physics. Taylor and Francis Group, New York-London (2007)
Dirac, P.A.M.: Proc. R. Soc. A 133, 60 (1931)
Dirac, P.A.M.: Phys. Rev. 74, 817 (1948)
Doran, C.J.L.: Geometric Algebra and Its Application to Mathematical Physics. A dissertation submitted for the degree of Doctor in Philosophy in the University of Cambridge (1994)
Grøn, Ø., Hervik, S.: Einstein’s General Theory of Relativity. With Modern Applications in Cosmology. Springer, Berlin (2007)
Nakahara, M.: Geometry, Topology and Physics. IOP Publishing Ltd., Bristol-Philadelphia (2003)
Romero, J.M., Bellini, M.: Eur. Phys. J. C 73, 2317 (2013)
Romero, J.M., Bellini, M.: Eur. Phys. J. C 74, 3043 (2014)
Romero, J.M., Bellini, M.: Can. J. Phys. 93, 445 (2015a)
Romero, J.M., Bellini, M.: Eur. Phys. J. C 75, 201 (2015b)
Shnir, Ya.M.: Magnetic Monopoles. Springer, Berlin-Heidelberg (2005)
Wesson, P.S., Ponce de León, J.: J. Math. Phys. 33, 3883 (1992)
Wesson, P.S., et al.: Int. J. Mod. Phys. A 11, 3247 (1996)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Romero, J.M., Bellini, M. Dyonic Reissner-Nordström black hole: extended Dirac quantization from 5D invariants. Astrophys Space Sci 359, 56 (2015). https://doi.org/10.1007/s10509-015-2504-3
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s10509-015-2504-3